An Efficient DCT-II Based Harmonic Wavelet Transform for Time-Frequency Analysis

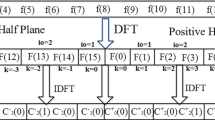
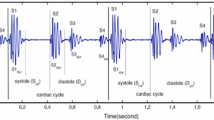
Abstract

DFT-based complex harmonic wavelet (CHW) is being used to directly compute frequency content with respect to time by employing discrete Fourier transform and inverse discrete Fourier transform. However, DFT coefficients suffer severe leakage of energy from one band to another band of frequency. To minimize the leakage between bands, a new basis function for harmonic wavelet transform using discrete cosine transform (DCTHWT) is proposed, which leads to a better representation of the time-frequency spectrum. The proposed DCTHWT bases are formulated by multiplying DCT-II matrix with a block diagonal matrix in which blocks are phase multiplied DCT-II matrices. The time-frequency analysis using the proposed DCTHWT is studied for different non-stationary input signals, and observed that leakages are minimized compared to the spectrogram, computed using DFT-based CHW. In addition to the aforesaid advantage, the proposed wavelet transform has features like admissibility, orthogonality, multiresolution and band-limited nature in the frequency domain. Further, the computational complexity of the proposed DCT-based harmonic wavelet transform is studied and observed that it has asymptotic gain of $50 \%$ compared to CHW and $33 \%$ compared to the modified CHW. Hence, the proposed DCT-based HWT is efficient in terms of computational complexity and have better time-frequency representation compared to DFT-based complex harmonic wavelet transform.

Appendix

1.1 A. Derivation of the Proposed DCTHWT Basis Function

The proposed DCTHWT equation in (15) is derived for $i_o >0$ in (13). Equation (13) is expressed excluding the constant term as

$$\begin{aligned} \begin{aligned} \psi _{[i_o, \tau ]}^{\prime }(n)&= \sum _{k=N_v}^{2N_v-1}\limits \cos \left( \frac{\pi (2n+1)k}{2N}\right) \cos \left( \frac{\pi (2k+1)\tau }{2N_v}\right) \\ {}&= \sum _{i_v=0}^{N_v-1}\limits \cos \left( \frac{\pi (2n+1)(N_v+i_v)}{2N}\right) \cos \left( \frac{\pi (2(N_v+i_v)+1)\tau }{2N_v}\right) \\ {}&= \sum _{i_v=0}^{N_v-1}\limits \cos \left( \frac{\pi (2n+1)(N_v+i_v)}{2N}\right) \cos \left( \pi \tau +\frac{\pi (2i_v+1)\tau }{2N_v}\right) \\ {}&= (-1)^{\tau }\sum _{i_v=0}^{N_v-1}\limits \cos \left( \frac{\pi (2n+1)(N_v+i_v)}{2N}\right) \cos \left( \frac{\pi (2i_v+1)\tau }{2N_v}\right) \\ {}&=\frac{(-1)^{\tau }}{2}\sum _{i_v=0}^{N_v-1}\limits \left( \cos (A+B) + \cos (A-B) \right) , \end{aligned} \end{aligned}$$

(26)

$$\begin{aligned} \text {where} \quad A=\frac{\pi (2n+1)(N_v+i_v)}{2N}, B=\frac{\pi (2i_v+1)\tau }{2N_v} . \end{aligned}$$

The first term in (26) is termed as $T_1$ and expressed as

$$\begin{aligned} \begin{aligned} T_1&=\sum _{i_v=0}^{N_v-1}\limits \cos (A+B)\\ {}&= \sum _{i_v=0}^{N_v-1}\limits \cos \left( \frac{\pi (2n+1)(N_v+i_v)}{2N} + \frac{\pi (2i_v+1)\tau }{2N_v}\right) \\ {}&= \sum _{i_v=0}^{N_v-1}\limits \cos \left( \frac{\pi N_v(2n+1)(N_v+i_v)+ \pi N(2i_v+1)\tau }{2NN_v}\right) \\ {}&= \sum _{i_v=0}^{N_v-1}\limits \cos \left( \alpha _1 + \beta _1 i_v\right) , \end{aligned} \end{aligned}$$

(27)

$$\begin{aligned} \text {where} \;\, \alpha _1= \frac{\pi N_v^{2}(2n+1)+\pi \tau N}{2NN_v}, \beta _1=\frac{\pi N_v(2n+1)+2 \pi \tau N}{2NN_v} . \end{aligned}$$

With the help of trigonometric identity, (27) is defined as

$$\begin{aligned} \begin{aligned} T_1&= \sum _{i_v=0}^{N_v-1}\limits \cos \left( \alpha + \beta i_v\right) \\ {}&= {\left\{ \begin{array}{ll} \cos \left( \alpha +(N_v-1)\frac{\beta }{2} \right) \frac{\sin \left( \frac{N_v\beta }{2}\right) }{\sin \left( \frac{\beta }{2}\right) } &{} \text {if}\;(\beta \ne 0)\\ N_v \cos (\alpha ) &{} \text {if}\;( \beta = 0) \end{array}\right. } \end{aligned} \end{aligned}$$

(28)

Equation (27) is derived with the help of (28) as

$$\begin{aligned} \begin{aligned} \sum _{i_v=0}^{N_v-1}\limits \cos (A+B)&= \cos \left( \alpha _1+(N_v-1)\frac{\beta _1}{2} \right) \frac{\sin \left( \frac{N_v\beta _1}{2}\right) }{\sin \left( \frac{\beta _1}{2}\right) }\\ {}&= \cos \left( C+\pi \tau \right) \frac{\sin \left( D+\pi \tau \right) }{\sin \left( \frac{(\pi \tau +D)}{N_v}\right) }\\ {}&=\frac{\cos (C)\sin (D)}{\sin \left( \frac{(\pi \tau +D)}{N_v}\right) } \end{aligned} \end{aligned}$$

(29)

$$\begin{aligned} \text {where} \;\, C=\frac{ \pi (2n+1)(3N_v-1)}{4N}+ \frac{\tau \pi }{2}, D=\frac{\pi (2n+1)N_v}{4N}-\frac{\pi \tau }{2} \end{aligned}$$

Similar to (29), the second term in (26) is obtained as

$$\begin{aligned} \sum _{i_v=0}^{N_v-1}\limits \cos (A- B)= \frac{\cos (C) \sin (D)}{\sin \left( \frac{D}{N_v}\right) } \end{aligned}$$

(30)

Combining the value of (29) and (30), $\psi '_{[i_o, \tau ]}(n)$ in (26) is obtained as

$$\begin{aligned} \begin{aligned} \psi _{[i_o, \tau ]}^{\prime }(n)&= \frac{(-1)^{\tau }}{2}\sum _{i_v=0}^{N_v-1}\limits \left[ \cos (A+B) + \cos (A-B)\right] \\ {}&= \frac{(-1)^{\tau }}{2}\left( \frac{\cos (C)\sin (D)}{\sin \left( \frac{(\pi \tau +D)}{N_v}\right) }+ \frac{\cos (C)\sin (D)}{\sin \left( \frac{D}{N_v}\right) }\right) \end{aligned} \end{aligned}$$

(31)

1.2 B. Derivation of $\sum _{m=1}^{n-2}\limits m 2^{m}$

The term $\sum _{m=1}^{n-2}\limits m 2^{m}$ is computed as

$$\begin{aligned} \begin{aligned} S&=\sum _{m=1}^{n-2} m 2^{m}\\ {}&=\left( 1\times 2 + 2\times 2^2 +3 \times 2^3 +4\times 2^4+\cdots +(n-2).2^{n-2}\right) \\{}& \begin{aligned}=\;&(2 +2^2+2^3+\cdots +2^{n-2})+(2^2+2^3+\cdots +2^{n-2})+\\&\cdots (2^k+2^{k+1}+\cdots +2^{n-2})+\cdots (2^{n-3}+2^{n-2})+2^{n-2}\end{aligned}\\ {}&\begin{aligned}=\;&2(2^{n-2}-1)+2^2(2^{n-3}-1)+\cdots +2^k(2^{n-k-1}-1)\\ {}&\cdots +2^{n-3}(2^2-1)+2^{n-2}(2-1)\end{aligned}\\ {}&=(n-1)2^{n-1}-(2 +2^2+2^3+\cdots +2^{n-2})\\ {}&=(n-1)2^{n-1}- 2(2^{n-2}-1)\\ {}&= n2^{n-1}- 2^{n}-2 \end{aligned} \end{aligned}$$

(32)