Design of an output feedback variable structure series vectorial compensator to enhance dynamic stability

Abstract

This paper presents an output feedback based Variable Structure Controller (VSC) is described for a series vectorial compensator (SVeC) in order to enhance the dynamic stability of electric power system. The input to the VSC is speed deviation and it is employed to design the switching surface of the proposed VSC. SVeC is a new series Flexible AC Transmission System (FACTS) device and this can regulate the line reactance by vary the duty ratio of PWM switches. The main aim is to check the effectiveness of the SVeC with VSC to enhance the stability in unique system. For this cause, proposed a novel SVeC current injection model incorporated in the test system. The mathematical model of VSC sliding controller gain proposed. The test system is derived using Heffron–Phillips model. The dynamic stability of the system with this proposed Variable Structure SVeC is analysed through eigenvalues and nonlinear time domain simulations for a unique system at nominal load. The performance of the proposed system is compared with SVeC with Feedback control, with PSS control and without control. From the results, it is concluded that the dynamic stability of the system is enhanced with proposed Variable Structure Feedback based SVeC controller compared with other methods.

Appendices

Appendices

$$ \begin{aligned} & H = 2.37\,\text{s}\;D = 0,\;K_{\rm A} = 400,\;R_{\text{S}} = 0.0\;\text{pu},\;R_{\text{e}} = 0.02\;\text{pu},\\ &\;T_{\text{d}} = 5.90s,\;T_{\rm A} = 0.2s\;\omega_{{\text{s}}} = 3.14\;{\text{rad/sec,}} \\ & X_{{\rm d}} = 1.70\;\text{pu},\;X_{\text{d}}^{^{\prime}} = 0.245\;\text{pu},\;X_{\text{e}} = 0.7\;\text{pu},\;X_{\text{q}} = 1.64\;\text{pu},\\ &\;V_{\inf } = 1.00\angle 0^{\circ} \;\text{pu},\;V_{\text{T}} = 1.72\angle 19.31^{\circ} \\ \end{aligned} $$

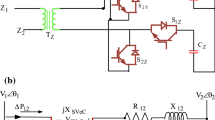
SVeC data

$$ K_{\text{SVeC}} = 4.0,\;T_{1} = 0.5,\;T_{2} = 0.1,\;T_{\text{SVeC}} = 25 $$

1.1 Computation of initial conditions in SMIB system

THETA1 = (pi*THETA)/180 = 0. 3370 (Voltage angle in radian).

THETA2 = (pi*0)/180 = 0 (Voltage angle in radian)

$$ V_{\text{i}} = 1.172;\;V_{\inf } = 1.0;\;X_{\text{eff}} = X_{\text{e}} = 0.7\;{\text{p}}.{\text{u}}; $$

$$ X_{\text{T}} = X_{\text{d}}^{^{\prime}} + X_{\text{eff}} = {0}{\text{.9450\;p}}.{\text{u}}; $$

$$ V_{1} = V_{\text{i}} *\exp (i*\text{THETA1}) = 1.1061 + i0.3876; $$

$$ V_{2} = V_{\inf } *\exp (i*\text{THETA2}) = 1; $$

$$ I_{\text{G}} = (V_{1} - V_{2} )/\left( {R_{\text{e}} + i*X_{\text{T}} } \right) = 0.4123 - i0.1035 $$

• Step 1: $\begin{aligned} E & = \left( {V_{1} + \left( {R_{\text{S}} + i*X_{\text{q}} } \right)*I_{\text{G}} } \right) = 1.2758 + i1.0637 \\ & = 1.6611\angle {0}{\text{.6950}} \\ \end{aligned}$

  $$ \delta = {0}{{.6950*180/3}}{.14} = {39}{{.8401}}\; \left( {{\text{Voltage}}\;{\text{angle}}\;{\text{in}}\;{\text{degrees}}} \right) $$

• Step 2: $I_{\text{dq}} = I_{\text{G}} *e^{{ - i\left( {\delta + \pi /2} \right)}} \;(\delta - {\text{angle in radian}}).$

  $\begin{aligned} I_{\text{d}} & = \text{real}\left( {I_{\text{dq}} } \right)\;{\text{and}}\;I_{\text{q}} = \text{imag}\left( {I_{\text{dq}} } \right) \\ V_{\text{dq}} & = V_{1} e^{{ - i\left( {\delta + 3.14/2} \right)}} \;(\delta - {\text{angle in radian}}) \\ \end{aligned}$

  $V_{\text{d}} = \text{real}\left( {V_{\text{dq}} } \right)\;{\text{and}}\;V_{\text{q}} = \text{imag}\left( {V_{\text{dq}} } \right)$

• Step 3: $E_{{d}}^{^{\prime}} = V_{\text{d}} + R_{\text{S}} I_{\text{q}} - X_{\text{q}} I_{\text{q}}$.

• Step 4: $E_{\text{q}}^{^{\prime}} = V_{\text{q}} + R_{\text{S}} I_{\text{q}} + X_{\text{d}}^{^{\prime}} I_{\text{d}}$.

• Step 5: $E_{\text{fd}} = E_{\text{q}}^{^{\prime}} + \left( {X_{\text{d}} - X_{\text{d}}^{^{\prime}} } \right)I_{\text{d}}$.

• Step 6: $V_{\text{ref}} = V_{\text{i}} + \left( {{\raise0.7ex\hbox{${E_{\text{fd}} }$} \!\mathord{\left/ {\vphantom {{E_{\text{fd}} } {K_{A} }}}\right.\kern-0pt} \!\lower0.7ex\hbox{${K_{A} }$}}} \right)$ and $T_{\text{M}} = E_{\text{q}}^{^{\prime}} I_{\text{q}} + \left( {X_{\text{q}} - X_{\text{d}}^{^{\prime}} } \right)I_{\text{d}} I_{\text{q}}$.

1.2 Calculation of K-constants in SMIB system

In SMIB system, considering 0.02 external resistance i.e. $R_{\text{e}} = 0.02$ [28] and calculate the K-constants using [28]

$$ \Delta_{\text{e}} = R_{\text{e}}^{2} + \left( {X_{\text{eff}} + X_{\text{d}}^{^{\prime}} } \right)\left( {X_{\text{eff}} + X_{\text{q}} } \right) = { 2}{\text{.2117}} $$

$$ \frac{1}{{K_{3} }} = 1 + \frac{{\left( {X_{\text{d}} - X_{\text{d}}^{\prime } } \right)\left( {X_{\text{q}} + X_{\text{e}} } \right)}}{{\Delta_{\text{e}} }} = 2.5394 $$

($\delta$-angle in radian)

$$ \begin{aligned} K_{4} & = V_{\infty } \frac{{\left( {X_{{\text{d}}} - X_{{\text{d}}}^{\prime } } \right)}}{{\Delta _{{\text{e}}} }}\left[ {\left( {X_{{\text{q}}} + X_{{\text{e}}} } \right)\sin \delta - R_{{\text{e}}} \cos \delta } \right] \\ K_{1} & = - \frac{1}{{\Delta _{{\text{e}}} }}\left[ {\begin{array}{*{20}l} {I_{{\text{q}}} V_{\infty } \left( {X_{{\text{d}}}^{\prime } - X_{{\text{q}}} } \right)\left\{ {\left( {X_{{\text{q}}} + X_{{\text{e}}} } \right)\sin \delta - R_{{\text{e}}} \cos \delta } \right\} + } \\ {V_{\infty } \left\{ {\left( {X_{{\text{d}}}^{\prime } - X_{{\text{q}}} } \right)I_{{\text{d}}} - E_{{\text{d}}}^{\prime } } \right\}\left\{ {\left( {X_{{\text{d}}}^{\prime } + X_{{\text{e}}} } \right)\cos \delta + R_{{\text{e}}} \sin \delta } \right\}} \\ \end{array} } \right] \\ \end{aligned} $$

($\delta$-angle in radian)

$$ \begin{aligned} K_{2} & = \frac{1}{{\Delta _{{\text{e}}} }}\left[ {\begin{array}{*{20}l} {I_{{\text{q}}} \Delta _{{\text{e}}} - I_{{\text{q}}} \left( {X_{{\text{d}}}^{\prime } - X_{{\text{q}}} } \right)\left( {X_{{\text{q}}} + X_{{\text{e}}} } \right) - } \\ {R_{{\text{e}}} \left( {X_{{\text{d}}}^{\prime } - X_{{\text{q}}} } \right)I_{{\text{d}}} + R_{{\text{e}}} E_{{\text{q}}}^{\prime } } \\ \end{array} } \right] \\ K_{5} & = \frac{1}{{\Delta _{{\text{e}}} }}\left[ {\begin{array}{*{20}l} {\frac{{V_{{\text{d}}} }}{{V_{{\text{i}}} }}X_{{\text{q}}} \left\{ {R_{{\text{e}}} V_{\infty } \sin \delta + V_{\infty } \cos \delta \left( {X_{{\text{d}}}^{\prime } + X_{{\text{e}}} } \right)} \right\} + } \\ {\frac{{V_{{\text{q}}} }}{{V_{{\text{i}}} }}X_{{\text{d}}}^{\prime } \left\{ {R_{{\text{e}}} V_{\infty } \cos \delta - V_{\infty } \left( {X_{{\text{e}}} + X_{{\text{q}}} } \right)\sin \delta } \right\}} \\ \end{array} } \right] \\ \end{aligned} $$

$$ K_{6} = \frac{1}{{\Delta_{\text{e}} }}\left[ {\frac{{V_{\text{d}} }}{{V_{\text{i}} }}R_{\text{e}} X_{\text{q}} - \frac{{V_{\text{d}} }}{{V_{\text{i}} }}X_{\text{d}}^{^{\prime}} \left( {X_{\text{q}} + X_{\text{e}} } \right)} \right] + \frac{{V_{\text{d}} }}{{V_{\text{T}} }} $$

1.3 Calculation of ‘A’ and ‘b’ matrices in state space model for SMIB system

The state-space model of the system is represented as [28]

$$ \dot{x} = Ax + Bu $$

where

$$ A = \left[ {\begin{array}{*{20}l} { - \frac{1}{{K_{3} T_{\text{d}} }}} & { - \frac{{K_{4} }}{{T_{do}^{^{\prime}} }}} & 0 & {\frac{1}{{T_{do}^{^{\prime}} }}} \\ 0 & 0 & {\omega_{\text{S}} } & 0 \\ { - \frac{{K_{2} }}{2H}} & { - \frac{{K_{1} }}{2H}} & { - \frac{{D\omega_{\text{S}} }}{2H}} & 0 \\ { - \frac{{K_{A} K_{6} }}{{T_{A} }}} & { - \frac{{K_{A} K_{5} }}{{T_{A} }}} & 0 & { - \frac{1}{{T_{A} }}} \\ \end{array} } \right] $$

$$ b = \left[ {\begin{array}{*{20}l} 0 & 0 \\ 0 & 0 \\ {\frac{1}{{\left( {2H} \right)}}} & 0 \\ 0 & {\frac{{K_{A} }}{{T_{A} }}} \\ \end{array} } \right] $$

1.4 Computation of initial conditions in SMIB system with SVeC

The calculation of effective reactance of the SMIB system with SVeC is $X_{\text{SVeC}} = 0.7044$ p.u (Consider the Duty Ratio from zero to 1)

$$ X_{\text{C1}} = 10\;{\text{p}}.{\text{u}} $$

$$ X_{\text{C}} = X_{{_{\text{C1}} }} /529.02\;\left( {{\text{Convert}}\;{\text{ to}}\;{\text{ p}}.{\text{u}}\;{\text{ values}}} \right) $$

$$ dX_{\text{SVeC}} = 10.4013\; \left( {{\text{According}}\;{\text{to}}\;{\text{the}}\;{\text{ref}}\;\left[ {{11}} \right]} \right) $$

$$ X_{\text{eff}} = X_{\text{e}} - X_{\text{SVeC}} = 1.4044\,{\text{p}}.{\text{u}} $$

$$ X_{\text{T}} = X_{\text{d}}^{^{\prime}} + X_{\text{e}} = 1.6474\;{\text{p}}.{\text{u}} $$

1.5 Calculation of K-constants and A and B matrices in state-space form for SMIB system with SVeC Δ_e = 5.0216

The equations for calculation of initial conditions with SVeC are similar to septs from 1 to 6, presented in Section “Calculation of K-constants in SMIB system”, and all are in p.u

$$ I_{\text{G}} = \left( {V_{1} - V_{2} } \right)/\left( {R_{\text{e}} + i*X_{\text{T}} } \right) = 0.2770 + 0.0716i $$

Calculation of K-constants and A and B matrices in state-space form for SMIB system with SVeC

$$ \Delta_{\text{e}} = {5}{\text{.0216}} $$

The equations for calculation of K-constants are presented in Section “Calculation of ‘A’ and ‘b’ matrices in state space model for SMIB system”.

The installation of SVeC in SMIB system results in addition of state variables corresponding to the SVeC controller $\Delta X_{\text{SVeC}} = \left[ {\begin{array}{*{20}l} {\Delta D_{\text{S}} } & {\Delta X_{\text{SVeC}} } \\ \end{array} } \right]^{T}$ in equations presented in Section “Computation of initial conditions in SMIB system with SVeC” to the ‘A’ matrix [11, 12]. The modified state variables with SVeC controller as

$$ \dot{x} = Ax + Bu $$

where

$$ \Delta x = \left[ {\begin{array}{*{20}l} {\Delta E_{\text{q}}^{^{\prime}} } & {\Delta \delta } & {\Delta \omega } & {\Delta E_{\text{fd}} } & {\Delta D_{\text{S}} } & {\Delta X_{\text{SVeC}} } \\ \end{array} } \right] $$

In $\Delta x$, the state variables, $\Delta D_{\text{S}}$ and $\Delta X_{\text{SVeC}}$ are the state variables of SVeC and the other variables are discussed in Sect. 2.1. The eigenvalues of the system matrix will be increased to two. The total eigenvalues of the system matrix with the installation of SVeC in SMIB system is six.

The equations corresponding to the SVeC controller are added in the HP model of SMIB system [15, 16]. Here

$K_{2} = \frac{{\partial P_{\text{e}} }}{{\partial E_{\text{q}}^{^{\prime}} }},\;K_{1} = \frac{{\partial P_{\text{e}} }}{\partial \delta }\;{\text{and}}\;K_{{D_{\text{S}} }} = \frac{{\partial P_{\text{e}} }}{{\partial D_{\text{S}} }}.$

Assuming stator resistance $R_{\text{S}} = 0$, the electrical power $\left( {P_{\text{e}} } \right)$ is.

$P_{\text{e}} = \frac{{E_{\text{q}}^{^{\prime}} V_{\infty } \sin \delta }}{{X_{\text{T}} }},$,where $X_{\text{T}} = X_{\text{d}}^{^{\prime}} + X_{\text{eff}}$ and $X_{\text{eff}} = X_{\text{e}} - X_{\text{SVeC}} \left( {D_{\text{S}} } \right)$ where $D_{\text{S}}$ is the duty ratio of the switches.

The system matrix A_sys for the corresponding model can be obtained as

$$ \left[ {\begin{array}{*{20}l} { - \frac{1}{{K_{3} T_{{{\text{do}}}}^{\prime } }}} & { - \frac{{K_{4} }}{{T_{{{\text{do}}}}^{\prime } }}} & 0 & {\frac{1}{{T_{{{\text{do}}}}^{\prime } }}} & 0 & 0 \\ 0 & 0 & {\omega_{{\text{s}}} } & 0 & 0 & 0 \\ { - \frac{{K_{{2}} }}{{2{\text{H}}}}} & { - \frac{{K_{{1}} }}{{2{\text{H}}}}} & { - \frac{{D\omega_{{\text{s}}} }}{{2{\text{H}}}}} & {\frac{{K_{{{\text{D}}_{{\text{s}}} }} }}{{2{\text{H}}}}} & 0 & 0 \\ { - \frac{{K_{{\text{A}}} K_{{6}} }}{{T_{{\text{A}}} }}} & { - \frac{{K_{{\text{A}}} K_{{5}} }}{{T_{{\text{A}}} }}} & 0 & { - \frac{1}{{T_{{\text{A}}} }}} & {\frac{{K_{{\text{A}}} }}{{T_{{\text{A}}} }}} & 0 \\ {\frac{{K_{{2}} T_{{1}} }}{{T_{{2}} }}\left( {\frac{{K_{{{\text{sVeC}}}} }}{{2{\text{H}}}}} \right)} & {\frac{{K_{{1}} T_{{1}} }}{{T_{{2}} }}\left( {\frac{{K_{{{\text{sVeC}}}} }}{{2{\text{H}}}}} \right)} & {\left( { - \frac{{K_{{{\text{sVeC}}}} }}{{T_{{2}} }} + - \frac{{K_{{{\text{sVeC}}T_{{1}} }} }}{{T_{{2}} }} - \frac{{D\omega_{{\text{s}}} }}{{2{\text{H}}}}} \right)} & 0 & { - \frac{1}{{T_{2} }}\left( {\frac{{ - K_{{{\text{sVeC}}T_{{1}} K_{{{\text{Ds}}}} }} }}{{2{\text{HT}}_{2} }}} \right)} & 0 \\ 0 & 0 & 0 & 0 & { - \frac{1}{{T_{{{\text{sVeC}}}} }}} & { - \frac{1}{{T_{{{\text{sVeC}}}} }}} \\ \end{array} } \right] $$

$$ B\_\text{SVeC} = \left[ {\begin{array}{*{20}l} 0 & 0 \\ 0 & 0 \\ \frac{1}{2H} & 0 \\ 0 & {\frac{{K_{A} }}{{T_{A} }}} \\ { - \frac{{K_{\text{SVeC}} T_{1} }}{{\left( {2HT_{2} } \right)}}} & 0 \\ 0 & 0 \\ \end{array} } \right] $$

$$ n_{\text{i}} = \text{rank}\left( {A\_\text{SVeC}} \right) = 6 $$

$$ m_{\text{i}} = {\text{rank}}\left( {{\text{B}\_\text{SVeC}}} \right) = 2m_{\text{i}} = {\text{rank}}\left( {{\text{B}\_\text{SVeC}}} \right) = 2 $$