Abstract
In this manuscript, we report the outcome of the topical workshop: paving the way to alternative NNLO strategies (https://indico.ific.uv.es/e/WorkStop-ThinkStart_3.0), by presenting a discussion about different frameworks to perform precise higher-order computations for high-energy physics. These approaches implement novel strategies to deal with infrared and ultraviolet singularities in quantum field theories. A special emphasis is devoted to the local cancellation of these singularities, which can enhance the efficiency of computations and lead to discover novel mathematical properties in quantum field theories.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
1 Introduction
Nowadays the calculation of observables relevant for high-energy physics (HEP) colliders is extremely important, in view of the future experiments that will provide new data with accuracy not reached so far. Therefore, insight and support from the theory side is necessary to understand the new findings. Clearly, the HEP community has to be ready to tackle this kind of problems and various approaches have to be implemented or reformulated. In particular, the perturbative framework applied to Quantum Field Theories (QFTs) has shown to be very important for providing highly precise predictions. The so-called Next-to-Leading Order (NLO) revolution was possible due to the emergence of novel techniques inspired by clever ideas. Likewise, predictions at NNLO are currently being calculated, but a fully established framework as at NLO is not yet complete. There are indeed several ideas and already working methods that can, for some processes, produce NNLO predictions to be compared with available experimental measurements.
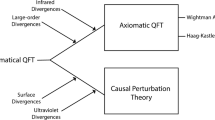
In the spirit of providing observables at NNLO and beyond, one encounters several obstacles that do not allow to easily perform an evaluation in the physical four space-time dimensions. For instance, the calculation of multi-loop Feynman integrals constitutes a challenge due to the presence of singularities. Hence, proper procedures to deal with infrared (IR) and ultraviolet (UV) divergences and with physical threshold singularities have to be devised and implemented. As seen in many applications, starting with an integrand free of singularities makes the evaluation more stable and leads to reliable numerical results. Such an integrand-level representation of physical observables, characterised by a point-by-point, or local cancellation of singularities, is one of the main topics of this manuscript and a valuable item in the HEP community wish-list.
This manuscript is one of the outcomes of the discussions and activities of the workshop “WorkStop/ThinkStart 3.0: paving the way to alternative NNLO strategies”, which took place on 4–6 November 2019 at the Galileo Galilei Institute for Theoretical Physics (GGI) in Florence. In this manuscript, we compare and summarise the several strategies, presented at the workshop, to locally cancel IR singularities and, thus, providing local integrand-level representations of physical observables in four space-time dimensions.Footnote 1 In order to analyse their features, we consider the NNLO correction to the scattering process \(e^{+}e^{-}\rightarrow \gamma ^{*}\rightarrow q{\bar{q}}\). The adopted techniques extend the ones summarised in Ref. [16], where thoughtful and complete descriptions of the NLO calculations for this process are provided. Although the full calculation of NNLO predictions requires several ingredients, we elucidate among the different frameworks their main features to perform such computations. Special emphasis is put on comparing the advantages and limitations of each strategy, in order to provide the reader with a better understanding of the techniques that are currently available.
It is clear that having a fully local representation of any physical observable allows for a smooth numerical evaluation and, thus, a more direct calculation of highly accurate predictions to be compared with the experimental data. In the context of this kind of calculations, taking care of the regularisation techniques applied to reach well-defined results is very important. Speaking in a wide sense, in this manuscript we consider two kinds of techniques, depending on the underlying dimensionality of the integration space: four vs. d dimensional implementations. Both alternatives are constrained to fulfil several conditions. In the following, we list a few of them.
-
A comparison between various versions of regularisation schemes that regulate singularities by treating the integration momenta in d dimensional space-time (dimensional schemes) and schemes that do not alter the space-time dimension (non-dimensional schemes) is carried out at NNLO, showing transition rules between both approaches at intermediate steps of the calculation.
-
The ultraviolet renormalisation, preserving all the symmetry properties of the amplitude, is under study in the different approaches. Some of these methods aim at an integrand-level renormalisation, which differs from the traditional integral-level framework. In other words, one is interested in extracting the usual UV counterterms directly from the bare amplitude rather than subtracting integrated counterterms. Once again, the main focus is put on the locality: subtracting the UV singularities directly from the amplitude leads to a local cancellation of non-integrable contributions in the UV region.
-
It is clear that multi-loop level calculations are, in general, contaminated not only by UV singularities. In fact, the presence of IR ones makes a direct integration more involved. This is because the standard IR singularities need to be canceled by the corresponding real corrections unless an IR counterterm is encountered.
A clear understanding of the aforementioned points will pave an avenue to provide a systematic procedure to generate NNLO calculations. On top of the d- or not to d-dimensional techniques, the treatment of \(\gamma _{5}\) might be elucidated to, thus, find agreement between the different schemes. Although this topic was not considered on of main the targets of this workshop, interesting discussions took place. In particular, at the closing discussion, which we summarise at the end of this manuscript.
Nevertheless, with the very interesting developments at the HEP colliders proposed for the near future, it is currently mandatory to consider higher-order predictions. Therefore, NNLO is no longer the ultimate goal and all methods need to overcome the obstacles of providing observables at \(\hbox {N}^3\)LO and \(\hbox {N}^4\)LO accuracy. Hence, for these reasons, presenting a collection of different methods has the intention of illustrating where we are and what we can do next. To this end, in the present manuscript, we comment on the features of the following regularisation/renormalisation schemes as well as methods that are only focused on the local cancellation of IR singularities:
-
Dimensional schemes: four dimensional helicity scheme (fdh) and dimensional reduction (dred)
-
Non-dimensional schemes: four-dimensional unsubtracted scheme (fdu), four dimensional regularisation/renormalisation (fdr), and implicit regularisation (ireg).
-
Subtraction methods: the \(q_{{\mathrm{T}}}\)-subtraction method, the antenna and the local analytic sector subtraction.
In the last section of this manuscript, we summarise the advantages and disadvantages of the above-mentioned methods. Furthermore, we provide a very brief summary of issues that were mentioned during the closing discussion session at the Workshop.
2 NNLO processes in FDH/DRED and FDR
The vast majority of higher-order calculations in QFT are done using conventional dimensional regularisation (cdr) to deal with ultraviolet (UV) and infrared (IR) singularities in intermediate expressions. As discussed in [\({\mathcal {O}}(\epsilon )\) terms in the real matrix element are compensated by similar modifications in the real-virtual corrections and adapted UV renormalisation, such that the physical cross section is scheme independent. This cancellation of the scheme dependence is best understood by treating the \(\epsilon \)-scalars that need to be introduced to consistently define fdh and dred as spurious physical particles. The UV and IR singularities of processes involving \(\epsilon \)-scalars cancel for physical processes after consistent UV renormalisation and combining double-virtual, real-virtual, and double-real parts. This leaves us with a finite contribution multiplied by \(n_\epsilon =2\epsilon \), the multiplicity of the \(\epsilon \)-scalars. Setting \(\epsilon \rightarrow 0\) in the final result the contribution of the \(\epsilon \)-scalars drops out or, equivalently, the scheme dependence cancels.
Since the contribution of \(\epsilon \)-scalars drops out for physical observables it is, of course, possible to leave them out from the very beginning. This is nothing but computing in cdr. However, including \(\epsilon \)-scalars sometimes offers advantages, as it is (from a technical point of view) equivalent to performing the algebra in four dimensions. We reiterate the statement that introducing \(\epsilon \)-scalars in diagrams and counterterms is nothing but a consistent procedure (also beyond leading order) to technically implement the often made instruction to “perform the algebra in four dimensions”.Footnote 2
Once we know how to transform from cdr to dimensional schemes where some degrees of freedom are kept in four dimensions, we ask the question whether the latter can be related to an entirely four-dimensional calculation using four-dimensional regularisation (fdr) [23,24,25].Footnote 3 It is clear that physical results must not depend on the regularisation scheme and, therefore, fdr (and fdh and dred) has to reproduce the results obtained in cdr, as long as the same renormalisation scheme (typically \({\overline{\textsc {ms}}}\)) is used. For that reason we investigate the possibility to relate individual parts of the calculation obtained in fdr to the corresponding ones in fdh and dred. Such a relation would deepen our understanding of the alternative schemes and tighten the argument that they are consistent (at least to the order investigated). On a more practical level, it opens up the possibility to compute individual parts in different schemes and consistently combine these results.
We start in Sect. 2.1 by presenting the new results for \(H\!\rightarrow \! b{\bar{b}}\) in dred and fdh and by discussing the results for \(\gamma \!\rightarrow \! q {{\bar{q}}}\). The corresponding NLO results and the \(N_F\) part of the NNLO results in fdr are presented in Sect. 2.2. In Sect. 2.3 we study the relation of the individual parts between the dimensional schemes and fdr.
2.1 FDH and DRED
The relation between cdr and other dimensional schemes such as fdh and dred has been investigated thoroughly in the literature [26, 27]. Before we consider the processes \(H\!\rightarrow \!b{\bar{b}}\) and \(\gamma ^*\!\rightarrow \!q{\bar{q}}\) we collect the renormalisation constants that are required. As is well known [28], in fdh and dred the evanescent coupling of an \(\epsilon \)-scalar to a fermion, \(a_e\!=\!\alpha _e/(4\pi )\), has to be distinguished from the gauge coupling \(a_s\!=\!\alpha _s/(4\pi )\). Identifying the renormalisation and regularisation scales, the relation between the bare couplings \(a_s^0\) and \(a_e^0\) to the \({\overline{\textsc {ms}}}\)-renormalised ones is given as
with \(S_{\epsilon }\!=\!e^{-\epsilon \gamma _{E}}(4\pi )^{\epsilon }\). In cdr we only need (2.1a) with \(n_\epsilon \!\rightarrow \!0\), whereas in fdh and dred \(n_\epsilon \!=\!2\epsilon \). The corresponding values in the \({\overline{\textsc {dr}}}\) scheme are simply obtained by setting \(n_\epsilon \!=\!0\) in (2.1). As the \(N_F\) part does not depend on \(n_\epsilon \) at the one-loop level, it is the same both in \({\overline{\textsc {ms}}}\) and \({\overline{\textsc {dr}}}\). For later purpose we further need the difference between (2.1b) and (2.1a) which is given by
For \(H\!\rightarrow \!b {\bar{b}}\) we also need the renormalisation of the Yukawa coupling \(y_{b}^{0} \) which is defined as the ratio of the bottom quark mass and the Higgs vacuum expectation value, see e.g. [29, 30]. Apart from its appearance through the Yukawa coupling we will set the bottom quark mass to zero. While the renormalisation of \(y_{b}^{0}\) is well known in cdr, for fdh/dred this constitutes an additional calculational step. Using the \({\overline{\textsc {ms}}}\) scheme we get
Similar to (2.2) we again have set equal the renormalised(!) couplings \(a_e\!=\!a_s\). The Yukawa renormalisation can be obtained from the UV divergences of an off-shell computation of the \(H\!\rightarrow \!b{\bar{b}}\) Green functions; however a technically simpler determination is also possible [31] by taking the on-shell form factor and subtracting the IR divergences obtained from the known general structure [26].
2.1.1 \(H\rightarrow b {{\bar{b}}}\) in FDH/DRED
In the following we consider one- and two-loop corrections to \(H\!\rightarrow \!b{{\bar{b}}}\) in fdh and dred. Our discussion follows closely [16] (for NLO) and [17] (for NNLO) where the corresponding calculations for the process \(e^+e^-\!\rightarrow \!\gamma ^*\!\rightarrow \! q{\bar{q}}\) are discussed. To start, we notice that disentangling the \(\epsilon \)-scalar contributions is actually simpler for \(H\!\rightarrow \!b{{\bar{b}}}\) than for \(\gamma ^*\!\rightarrow \!q {{\bar{q}}}\). This is due to the fact that the tree-level interaction is not mediated by a gauge boson and, accordingly, we therefore only have to split the gluon field in the one-loop contributions. Moreover, the major difference between fdh and dred is in the treatment of so-called ‘regular’ vector fields, see e.g. Table 1 of [16]. As in the present case only ‘singular’ vector fields contribute, the virtual correction is the same in both schemes, i.e.
see also Fig. 1. Using form factor coefficients \(F^{(n)}\) of loop-order n, the bare amplitude for \(H\!\rightarrow \! b {\bar{b}}\) can be written as
including the regularisation scale \(\mu _0\) and the Higgs mass \(M_H\). The tree-level amplitude \({\mathcal {A}}^{(0)}_{\text {bare}}\!=\! i\,y_{b}^{0}\, {\bar{u}}(p_b)v(p_{{\bar{b}}})\) results in the tree-level decay width \(\Gamma ^{(0)}\!=\!2\,M_H^2 y_b^2/(2 M_H)\).
2.1.2 NLO
The NLO virtual corrections are directly related the one-loop form factor for which we get in fdh/dred
The well-known cdr result which is given e.g. in [29, 30] is obtained by setting \(n_\epsilon \!\rightarrow \!0\). Identifying the (bare) couplings in (2.6) before making replacement (2.1) corresponds to so-called ‘naive’ fdh and it has been shown that this leads to inconsistent results at higher orders [32,33,34]. Instead, we first have to renormalise \(a_{s}^{0}\) and \(a_{e}^{0}\) and only then we are allowed to identify the renormalised couplings \(a_e\!=\!a_s\).
Integrating over the phase space and using the conventions of [16] we then obtain for the UV-renormalised virtual cross section
where we introduce the \(\epsilon \)-dependent prefactors
The subscript ds in (2.7) and in what follows indicates that the results for all dimensional schemes can be obtained from this expression. For the evaluation of the real contribution we use the setup and notation of [16] and arrive at
As for the virtual cross section, the result is the same in fdh and dred which is due to the absence of ‘regular’ gauge bosons at NLO for the considered process. The presence of a ‘singular’ gluon, however, leads to \(a_s\) contributions (which stem from the \({d}\)-dimensional gluon) and \(a_e\) contributions (which stem from the associated \(\epsilon \)-scalar gluon). Again, at NLO such a distinction is of course not strictly necessary; at NNLO, however, the different renormalisation of \(a_s\) and \(a_e\) has to be taken into account, see also Sect. 2.3.
Finally, since \(c_\Gamma \,\Phi _2 s^{-\epsilon }/\Phi _3 =1+\mathcal{O}(\epsilon ^3)\), the cancellation of singularities between (2.7) and (2.9) takes place and leaves us with the well known finite answer
In particular, the evanescent contributions \(\propto a_en_\epsilon \) drop out.
2.1.3 NNLO
In the following we provide separately the double-virtual, double-real, and real-virtual contributions to \(\Gamma \) in fdh/dred. All of these results are new and have not been published elsewhere before. For brevity reasons we keep the dependence on \(n_\epsilon \) explicit in the divergent terms, but drop finite \(n_\epsilon \) terms. Setting \(n_\epsilon \!\rightarrow \!0\ (2\epsilon ) \) then yields the cdr (fdh/dred) result. As before, we give the UV-renormalised results after having set \(a_e\!\equiv \!a_s\).
Writing the double virtual corrections as
we then obtain for the individual parts
We note that in (2.11) we could have pulled out additional prefactors such as \(c_\Gamma ^2\), in analogy to (2.7). This would of course modify the subleading poles and finite terms of (2.12) (as well as (2.13) and (2.14) below). The conclusions, however, we will draw in Sect. 2.3 are not affected by the choice of the prefactor.
As mentioned before, the one- and two-loop renormalisation of the Yukawa coupling is included in (2.12) in order to get consistent results. We would like to stress, that in fdh and dred there are finite terms associated with the \({\overline{\textsc {ms}}}\) renormalisation factors. The terms \(\propto n_\epsilon ^m/\epsilon ^n\) (\(m,n\!>\!0\)) that are potentially finite when setting \(n_\epsilon \!=\!2\epsilon \) cancel when combining the double-virtual with the real-virtual and double-real contribution, as will be shown below. However, if the double-real (and real-virtual) corrections are computed by doing the algebra in four dimensions, the \(n_\epsilon \) terms are not disentangled any longer and, therefore, all these terms need to be included to obtain a consistent result. Moreover, as no \(\epsilon \)-scalars are present at the tree level, (2.1) is sufficient for the renormalisation of \(a_s^0\) and \(a_e^0\).
Splitting the real-virtual and double-real contributions in a similar way as before, we then get
and
To get the double-real contribution we used the integrals of [35] and the FORM code of [36]. As for the process \(\gamma ^*\!\rightarrow \!q{\bar{q}}\) [17], the double-real corrections in dred/fdh are simply obtained by integrating the four-dimensional matrix element squared over the phase space. Their determination is therefore significantly simplified compared to the case of cdr.
Finally, combining these results, we can extend (2.10) to NNLO asFootnote 4
in agreement with [37]. As expected, the divergent \(n_\epsilon \) parts cancel in the final result which is nothing but the scheme-independence of the physical result.
2.1.4 \(\gamma \rightarrow q {{\bar{q}}}\) in FDH/DRED
The scheme dependence of the cross section \(e^+e^-\!\rightarrow \!\gamma ^*\!\rightarrow \!q{\bar{q}}\) at NNLO is discussed in detail in [17]. For this particular process the individual results in dred and fdh differ, as in dred there are \(\epsilon \)-scalar photons present in the tree-level process. While [17] mainly deals with dred, here we only recall a few points that are relevant for the comparison of fdh with fdr. In particular, we want to extend to NNLO the investigation of the interplay between fdh and fdr presented at NLO in [16].
2.1.5 NLO
Copying the results given in Section 2.3 of [16], we write the virtual and real cross sections as
where \(\sigma ^{(0)}\!=\!e^4/(4\pi )\,Q_q N_c/(3s)\) includes the electric charge and the colour number of the quark as well as the flux factor 1/(2s). Combining these two contributions we find the well-known regularisation-scheme independent physical cross section
2.1.6 NNLO
Moving on to NNLO, we first split the cross section into a double-virtual, a double-real, and a real-virtual part, i.e.
For the comparison with fdr in Sect. 2.3, we here focus on the \(N_F\) part of the respective contributions. The double-virtual part can be extracted from the dred result given in (3.8b) of [17] as
The other two contributions are given by
The combination of all three parts results in
in agreement with the literature [38, 39]. Note that the constant terms of (2.21) and (2.22) differ from the corresponding results in dred, as given in (3.20) and (3.17b) of [17].
2.2 FDR: four-dimensional regularisation/renormalisation
2.2.1 \(H\rightarrow b {{\bar{b}}}\) in FDR
2.2.2 NLO
Here we describe the fdr NLO calculation of the decay rate \(\Gamma _{H\rightarrow b {{\bar{b}}}(g)}\). The strong coupling constant does not appear at the tree-level and as in Sect. 2.1 we use the \({\overline{\textsc {ms}}}\) value with \(a=\alpha _s/{4 \pi }\). As for the unrenormalised bottom mass, it is denoted by \(m_0\) and again it is taken to be different from zero only in the Yukawa coupling. The one-loop relation between \(m_0\) and the physical pole mass m, defined as the value of the four-momentum at which the bottom quark propagator develops a pole, is obtained by evaluating the diagram of Fig. 2,
The unphysical mass \(\mu ^2\) in (2.24) is the fdr UV regulator, which in the present calculation is taken to coincide with the fdr IR regulator.Footnote 5 Once the decay amplitude is renormalised (namely expressed in terms of physical quantities only) all the \(\mu ^2\)s of UV origin get replaced by physical scales, and the remaining ones are IR regulators which cancel in the sum of virtual and real contributions. As a matter of fact, we will encounter two additional combinations besides \(L^{\prime \prime }\),
where for \(H\rightarrow b {\bar{b}}\) we have \(s=M_H^2\), the Higgs mass squared. The amplitude contributing to \(H\rightarrow b {{\bar{b}}}\) can be written as
where \(A^{(0)}_2\) is the tree level result and the one-loop correction of Fig. 1 reads
Inserting (2.24) in (2.26) produces the renormalised one-loop amplitude
Upon integration over the 2-body phase-space, the square of (2.28) gives the virtual part of the NLO corrections,
This can be translated to the \({\overline{\textsc {ms}}}\) scheme by expressing \(m^2\) in terms of the running \({\overline{\textsc {ms}}}\) bottom mass \({\underline{m}}^2(M^2_H)\) [40]
With the corresponding change in the Yukawa we obtain
which can be directly compared with (2.7). The real counterpart is obtained by squaring the diagrams of Fig. 3 and integrating over a 3-body phase-space in which all final-state particles acquire a small mass \(\mu \) [41],
Replacing \({{{\mathcal {R}}}}e(L')^2 = L^2+\pi ^2\), the sum of (2.31) and (2.32) gives the UV and IR finite decay rate up to the NLO accuracy
which agrees with (2.10).
2.2.3 NNLO, \(N_F\) part
The \(N_F\) parts contributing to the NNLO cross section in fdr are given in (4.19) of [25] in terms of the Yukawa coupling defined through the pole mass of the bottom quark. If we express these results in terms of the \({\overline{\textsc {ms}}}\) Yukawa couplings, as done for (2.31), we get
2.2.4 \(\gamma \rightarrow q {{\bar{q}}}\) in FDR
2.2.5 NLO
The computation of \(\gamma \rightarrow q {{\bar{q}}}\) in fdr at NLO has been discussed in [16]. Here we just list the results for the virtual and real corrections, as given in (5.2) and (5.35) of [16]. They read
where L is defined in (2.25), with s now the centre-of-mass energy squared.
2.2.6 NNLO, \(N_F\) part
The \(N_F\) parts contributing to the NNLO cross section in fdr are given in (5.13) of [25] and read
2.3 Relations between FDH and FDR
2.3.1 NLO
The relation between IR divergences of virtual (and therefore real) one-loop results obtained in fdh and fdr has been established in (5.41) of [16] for the process \(\gamma ^*\!\rightarrow \! q {\bar{q}}\) and reads
These relations are here confirmed by the results of \(H\!\rightarrow \!b {\bar{b}}\). In fact, (2.9) and (2.32) are related through (2.42) and so are (2.7) and (2.31) if the \({\overline{\textsc {ms}}}\) mass (2.30) is used. Moreover, if \(\epsilon \!=\!0\) in prefactors that are related to integration in \({d}\) dimensions, i.e. \(c_{\Gamma }(\epsilon \!=\!0)\), \(\Phi _{2}(\epsilon \!=\!0)\), and \(\Phi _{3}(\epsilon \!=\!0)\) in (2.8), the finite terms of the individual parts are the same in fdh and fdr, including the \(\pi ^2\) terms.
In order to find similar transition rules at NNLO, we also follow a second approach for the comparison between fdh and fdr which is slightly different. To start, we multiply a generic virtual one-loop result obtained in fdh by an \(\epsilon \)-dependent function \(\phi _1(\epsilon )\) and demand equality with the corresponding fdr result, i.e.
with
The coefficients \(b_{11}\) and \(b_{12}\) are so far unknown and can be obtained by inserting known fdh and fdr results. Before we can do this, however, we have to rescale powers of \(1/\epsilon \) by using
The scale factor \(\lambda _1\) is so far unknown and sets the size of the fdh \(1/\epsilon \)-pole with respect to the fdr logarithm L at NLO. Evaluating (2.43) by using for instance (2.16) and (2.37) we find after expanding, applying shift (2.45), and drop** \({\mathcal {O}}(\epsilon )\) terms
Equations (2.44)–(2.46) are equivalent to (2.42) in that they allow to translate virtual (and therefore real) one-loop results obtained in fdh to fdr and vice versa, including the finite terms. The validity of the rules has been checked explicitly for NLO corrections to \(H\!\rightarrow \!b{\bar{b}}\) and \(\gamma ^*\!\rightarrow \!q{\bar{q}}\).
2.3.2 NNLO, double-virtual
We start our NNLO comparisons with the IR divergences in the \(N_F\) part of the double-virtual contributions. As mentioned repeatedly, to get correct and unitary NNLO results in fdh it is crucial to distinguish evanescent couplings at NLO, see for instance (2.6) and the text below as well as (2.16). In fdr, on the other hand, unitarity is restored by treating UV-divergent one-loop subdiagrams appearing in two-loop amplitudes in the same way they are treated at NLO. This can be achieved by introducing ‘extra-integrals’, as explained in Appendix A of [25]. After taking this contribution into account, it is possible to compare the IR divergences of the double-virtual results obtained in fdh and fdr.
In order to find transition rules between the two schemes we follow the approach described in the previous section and adjust the involved quantities accordingly, i.e. we demand
with
and get after the rescaling
and the use of (2.12c) and (2.20) the two-loop coefficients
Similar to the one-loop case, we have reabsorbed the difference between the two schemes in the prefactor \(\phi _2(\epsilon )\), including the finite terms. Restricting ourselves to the \(N_F\) part of the double-virtual corrections for a single process, (2.48)–(2.50) can be seen as using four parameters \(\{\lambda _2, b_{21}, b_{22}, b_{23}\}\) to enforce four equalities between the \(L^n\) and \(\epsilon ^{-n}\) terms for \(n\in \{3,2,1,0\}\). What is remarkable is that the same relations hold for \(H\!\rightarrow \!b{\bar{b}}\) and \(\gamma ^*\!\rightarrow \!q{\bar{q}}\). Despite their apparent similarity, these two processes have a completely different behaviour regarding UV renormalisation. The question whether these rules also apply to the \(C_F^2\) and \(C_A\,C_F\) part of the aforementioned processes or to other processes can not be answered at the moment. Of course, the precise form of the subleading poles depends on whether or not prefactors like \(c_\Gamma ^2(\epsilon )\) are factored out in the fdh results. This explains the \(\pi ^2\) and \(\zeta _3\) terms in (2.50b) and (2.50c). Moreover, note that the two-loop scaling factor (2.50d) is different from the one-loop scaling (2.46c).
2.3.3 NNLO, real-virtual
Regarding the real-virtual corrections we first notice that for the considered processes the \(N_F\) part solely stems from the (sub)renormalisation of the bare couplings in the real one-loop result. In other words, it is given by the product of two one-loop quantities: the real NLO contribution (which contains double and single IR divergences) times a one-loop renormalisation constant (which contains a single UV divergence).
In fdh, the corresponding terms originate from inserting (2.1a) and (2.1b) in (2.9) and (2.17) as the Yukawa coupling \(y_b^0\) does not depend on \(N_F\) at NLO. Similar to the double-virtual contributions it is crucial to distinguish gauge and evanescent couplings in order to avoid the wrong UV-renormalisation of the \(a_e\) terms. Ignoring this distinction, however, leads to results in ‘naive’ fdh which are different from fdh:
In fdr, the corresponding results are given in (2.35) and (2.41) and read
Similar to fdh, they stem from the \(a_s\)-renormalisation in (2.32) and (2.38), see also (3.1) and (3.2) of [25]. In contrast to the double-virtual contributions, however, the different renormalisation of \(a_e\) and \(a_s\) is not taken into account via ‘extra-integrals’. Accordingly, (2.55) and (2.56) correspond to ‘naive’ fdh, i.e. (2.53) and (2.54), rather than fdh. Since only one-loop quantities are involved, the transition between ‘naive’ fdh and fdr is already known and given by e.g. (2.42) times the transition \(1/\epsilon _{{\textsc {uv}}}\leftrightarrow L\) for the UV divergence, i.e.
Note that the transition of the divergent \(\pi ^2\) terms depends on the \({d}\)-dependent prefactors that have been factored out in the fdh result, similar to the double-virtual contributions.
Regarding the finite terms it is clear that a transition between (‘naive’) fdh and fdr can not exist. The reason is that for the considered processes the \(N_F\) part of the real-virtual contribution in fdr is obtained via multiplying (2.32) and (2.38) by a pure UV divergence. As a consequence, (2.55) and (2.56) only contain pure divergences (which are parametrized as powers of L) and no finite terms. Therefore, the \(N_F\) part of the real-virtual contribution alone does not contain a finite part which is different from any dimensional scheme.
2.3.4 NNLO, double-real
In the previous section we have seen that a transition rule for real-virtual contributions between fdh and fdr does not exist. As the physical result has to be scheme independent, this is also the case for the double-real contributions. Given the fact that a transition rule exists for the double-virtual part, however, it is clear that (2.48) can also be used to translate the sum of the real-virtual and double-real components from fdh to fdr. This is due to the fact that the divergences are the same (apart from their sign) and that the finite term is given by the difference between the physical result and the finite part of the double-virtual contribution. We have checked this explicitly and find indeed
2.3.5 Unitarity restoration in FDH and FDR
As we have commented many times, fdh and fdr use different strategies to restore unitarity. In this paragraph, we report on an attempt towards a comparison of the two unitarity restoration methods.
Our starting point is ‘naive’ fdh in which no distinction is made between gauge and evanescent couplings, and we try to extract from fdr the contribution of the fdh evanescent \(a_e\) terms. This is achieved by interpreting the fdr ‘extra-integrals’ as UV-divergent dimensionally regulated integrals subtracted at the integrand level, as explained in Section 6 of [\(EEI_b\)).Footnote 7 These integrals contain now \(1/\epsilon \) poles of UV origin suitable to be combined with the ‘naive’ fdh expressions. For the regarded processes, their contribution corresponds to the evanescent \(a_e\) terms in (2.6) and (2.16), respectively, times the difference of the renormalisation constants \(\delta _Z\!=\!(Z_{\alpha _e}\!-\!Z_{\alpha _s})\), whose value in the \({\overline{\textsc {ms}}}\)-scheme is given in (2.2),
More precisely, (2.6) and (2.16) are reproduced if the contribution of (2.60) is added to the ‘naive’ FDH results. Note that, since \(\delta _Z\!=\!{\mathcal {O}}(\epsilon ^{\!-\!1})\) and \(n_\epsilon \!=\!2\epsilon \), the \(EEI_b\) are of \({\mathcal {O}}(\epsilon ^{\!-\!1})\) and that (2.60) refers to the full contributions, not only the \(N_F\) parts. Finally, it should be mentioned that the contributions in (2.60) have been extracted from off-shell diagrams, while the unitarity restoring fdr procedure to be used on-shell is slightly different [fdu framework to obtain the NNLO QED corrections to the \(N_f\) terms associated to \(\gamma ^{*}\!\rightarrow \! q {{\bar{q}}} (g)\).
We would like to highlight that the fdu/ltd framework has been extended and improved since the last WorkStop/ThinkStart meeting in 2016 [16]. Therefore, we briefly review the new features and properties that have been recently improved.
3.1 Dual representations from the LTD theorem
The ltd theorem is based on a clever application of Cauchy’s residue theorem (CRT) to the loop integrals. The original version was developed in Refs. [47, 49], where CRT was used to integrate out the energy component of each loop momenta. This procedure reduces loop amplitudes to collections of tree-level-like diagrams with a modification of the customary Feynman prescription. Thus, given a loop line associated to the momentum \(q_i\), we should use the rule
to replace the Feynman propagators when the momentum \(q_j\) is set on-shell. In this expression, \(\eta \) is a future-like vector that defines the explicit dependence of the prescription on the momentum flow, and the integration contours are closed on the lower half-plane such that only those poles with negative imaginary components are selected. It is important to notice that this dual representation is equivalent to the usual Feynman tree theorem (ftt) [63, 64], and the multiple cut information is encoded within the momentum-dependent dual prescription.
Recently, a new representation of dual integrals was achieved through the iterated calculation of residues, leading to what we call nested residues [54,55,56,57]. This strategy turns out to be more efficient computationally, since it allows a straightforward algorithmic implementation. Hence, in order to elucidate how ltd formalism works, let us consider an L-loop scattering amplitude with N external legs, \(\{p_j\}_N\) and n internal lines, in the Feynman representation,
This amplitude is naturally defined over the Minkowski space of the L loop momenta, \(\{\ell _s\}_L\). The singular structure is associated to the denominators introduced by the Feynman propagators,
with
where the \(q_{i},m_{i}\) and \(+\imath 0\) correspond to the loop momentum, its mass, and the infinitesimal Feynman prescription. Furthermore, we explicitly pull out the dependence on the energy component of the loop momentum \(q_{i,0}\) together with its on-shell energy,
that is expressed in terms of the spatial components of the loop momentum.
Besides, \(a_i\) and \(\{1,\ldots ,n \}\) in Eq. (3.2) correspond to the arbitrary positive integers, raising the powers of the propagators, and sets containing internal momenta of the form \(q_{i_j}=k_{j}+\ell _i \in i\), respectively. In the following, the \(a_j\) exponents will not be included in the notation because the treatment of the expressions is independent of their explicit values.
As in the previous ltd representation, the dual contributions are obtained by integrating out one degree of freedom per loop through the Cauchy residue theorem. Iterating this procedure, we can write [54],
starting from
All the sets in Eq. (3.6) before the semicolon contain one propagator that has been set on shell, while all the propagators belonging the sets that appear after the semicolon remain off shell. The sum over all possible on-shell configurations is implicit. Regarding the prescription, the contour choice is the same used in the original ltd formulation. It is worth mentioning that the ltd representation is independent of the coordinate system, and that there are some non-trivial cancellations when iterating the residue loop-by-loop. The last result implies that only those poles whose loci is always on the lower complex half-plane will lead to non-vanishing contributions; the others, called displaced poles will cancel at each iterative step [57]. Moreover, these contributions can be mapped onto the usual cut diagrams, thus allowing a graphical interpretation.
Finally, we would like to emphasise that the ltd representation corresponds to tree-level like objects integrated in the Euclidean space. In this way, the application of this novel representation of multi-loop scattering amplitudes allows to open loops into trees, aiming at finding a natural connection with the structures exhibited in the real emission contributions.
3.2 Local cancellation of IR singularities through kinematic map**s
Once the ltd theorem is applied to any multi-loop multi-leg scattering amplitude, we obtain a representation involving tree-level like objects and phase-space integrals. This leads to an important conceptual simplification to understand the origin of IR and threshold singularities, at integrand level. By analysing the intersection of the integration hyperboloids associated to the dual contributions for different cuts [fdu formalism has been successfully proven to deal with NLO corrections to physical observables, such as cross sections and decay rates [
with \(|{{{\mathcal {M}}}}^{(0)}_{n}|^2\) the Born squared amplitude and \(\mathcal{S}_0\) the IR-safe measure function that defines the physical observable. On the one hand, the virtual one-loop contribution is
where \({\mathrm{Re}}({{{\mathcal {M}}}}^{(1)}_{n} {{{\mathcal {M}}}}^{(0),*}_{n})\) corresponds to the interference between the one-loop and the Born amplitude, including also factors that might be related to self-energy contributions. On the other hand, we need to take into account the real-emission contribution,
which is characterised by the presence of an additional external particle. Notice that the measure function, \(\mathcal{S}_1(\{{p'}_i\})\), is extended to include the extra radiation and it must fulfil the reduction property \({{{\mathcal {S}}}}_1 \rightarrow {{{\mathcal {S}}}}_0\) in the IR limits. Moreover, the corresponding IR singularities can be disentangled and isolated into disjoint regions of the real-emission phase-space. Following a slicing strategy, we introduce a partition \({{{\mathcal {R}}}}_i\) and define
that fulfils \(\sum _ i \sigma _{R,i}^{(1)} = {\sigma }_R^{(1)}\) and that only one IR divergent configuration is allowed inside different \({{{\mathcal {R}}}}_i\).
Regarding the virtual contribution, the application of ltd to Eq. (3.9) will produce a sum of cuts leading to dual contributions, namely
with N the number of different internal lines. Each line is characterised by a momenta \(q_i\), which is set on shell in the different dual terms. The presence of this extra on-shell momenta allows to establish a connection with the real emission contributions, through a proper momentum map**. Explicitly, for the NLO case, we have a bijective transformation,
restricted to some partition \({{{\mathcal {R}}}}_i\). At this point, the dual contributions can be understood as local counterterms for the real corrections, whilst the development of the transformations \({{{\mathcal {T}}}}_i\) is guided by the structure of the partition \(\mathcal{R}_i\). This partition is based on the FKS or CS strategy, i.e. splitting the phase-space into disjoint regions containing at most one infrared singularity. Then, through a proper study of the cut structure, a connection among dual integrals and partitions is established, in such a way that a map** \({{{\mathcal {T}}}}_i\) exists and leads to local cancellations of IR singularities.
3.3 Multi-loop local UV renormalisation
The successful identification and cancellation of IR singularities lead to cross sections with only UV singularities. The standard procedure to remove those divergences requires renormalisation of the field wave-functions and couplings, this is what we aim to reach through the construction of local UV counterterms. Since all these elements have to cast only UV divergences in the ltd framework, it is important to study carefully the analytic properties of the UV integrands that will be added to the real radiation. At one-loop, it has been considered the regime of massless and massive particles propagating in the loop [42,43,44]. The transition between massless and massive renormalisation constants has found to be smooth and singularities are well understood in this framework. Let us point out a crucial difference between the standard renormalisation constant in dreg and ltd.
Wave-function renormalisation constants emerge from the computation of self-energy diagrams. In particular, massless bubble diagrams in dreg do not present any problem since the IR and UV divergences are considered as equal, therefore, the full integral vanishes. On the contrary, the same integral in the fdu/ltd framework will contribute to the IR and UV regions because they are treated separately, therefore, the integral cannot be removed even if the full integral is actually zero. We stress that integrands in the fdu/ltd are split into the IR and UV domains, and they have to be keep in this way in order to render the full integrands free of singularities in the fdu formalism.
Let us review the basic ideas of one-loop renormalisation constant; these ideas are implemented and improved for the two-loop case and beyond. The massive wave-function renormalisation constant, in the Feynman gauge, at one-loop can be obtained from,
which represent the unintegrated form. This integral is obtained by the standard Feynman rules procedure. It is important to remark that the limit of massless case is straightforward achieved from Eq. (3.14), so this expression is the most general of \(\Delta Z_2(p_1; M)\). Since \(\Delta Z_2(p_1; M)\) contains singularities associated to the UV domain, it is important to find the UV component of the Eq. (3.14), \(\Delta Z_2^{{{\mathrm{UV}}}}\), and subtract it in order to find the UV-free wave-function renormalisation constant, \(\Delta Z_2^{{{\mathrm{IR}}}}\). The UV part is extracted by performing an expansion of the integrand around the UV propagator \(G_F(q_{\mathrm{UV}}) = (q^2_{\mathrm{UV}}-\mu _{\mathrm{UV}}^2 + \imath 0)^{-1}\). In particular, for Eq. (3.14), it is found
Finally, \(\Delta Z_2^{\mathrm{IR}}\) is given by,
which contains only IR singularities and they are needed to cancel the remaining singularities at the cross section level.
We focus now on subtraction of UV singularities for two-loop amplitudes. In general, for the two-loop case, the integrand involved is cumbersome, therefore, we will use this document to emphasise the procedure to renormalise locally the UV behaviour of any two-loop amplitude. The procedure is valid if the integrand is free of infrared singularities. In this sense, it is mandatory to subtract first all IR divergences and then apply the following algorithm. This algorithm has been extensively discussed in [51,52,53], with applications of one- and two-loop scattering amplitudes.
Let us now consider a generic two-loop scattering amplitude free of IR singularities,
where the integrand is a function of the integration variables \(\ell _1\) and \(\ell _2\). UV divergences shall appear when the limit of \(|\vec {\ell }_1|\) and \(|\vec {\ell }_2|\) go to infinity. In the two-loop case, there are three UV limits to be considered. The limit when \(|\vec {\ell }_1|\) goes to infinity and \(|\vec {\ell }_2|\) remains fixed, the other way around, and when \(|\vec {\ell }_1|\) and \(|\vec {\ell }_2|\) go to infinity simultaneously. Based on the ideas developed at one-loop, the UV divergences can be extracted from the integrand by making the replacement,Footnote 9
for a given loop momentum \(\ell _j\) and expanding the expression up to logarithmic order around the UV propagator. This construction is represented by the \(L_{\lambda }\) operator. It is worth mentioning that the result shall generate a finite part after integration that has to be fixed to reproduce the correct value of the integral. Therefore, the first counterterms will be obtained by
where \(d_{j,{\mathrm{UV}}}\) is the fixing parameter which makes the finite part of integral to be zero in the \(\overline{{\mathrm{MS}}}\) scheme.
Now, after the complete subtraction of these counterterms, the remaining divergences shall occur when both \(|\vec {\ell }_1|\) and \(|\vec {\ell }_1|\) approach to infinity simultaneously. In this case, the following replacement is implemented,
on the subtracted integrand. Then, the application of the \(L_{\lambda }\) operation has to be made and the fixing parameter is again needed in order to build properly the counterterm, \({\mathcal {A}}^{(2)}_{{{\mathrm{UV}}}^2}\). Explicitly,
where \(d_{{{\mathrm{UV}}}^2}\) is the fixing parameter of the double limit.
Finally, the original amplitude can be renormalised by the subtraction of all UV counterterms, such thatFootnote 10
is free of IR and UV singularities.
Before closing this discussion, let us deepen into the multi-loop case. In this scenario, multiple ultraviolet poles will appear, since all loops have the possibility to tend to infinity at different speed. However, if the amplitude is free of IR singularities, the algorithm presented along this section is still valid. For an L-loop integral, there are \(2^L-1\) UV counterterms at most with the same number of fixing parameters. Therefore, after the proper knowledge of all UV counterterms, the renormalisation of the original L-loop amplitude is achieved and the four-dimensional representation of the integrand can be obtained.
3.4 \(\gamma ^{*}\rightarrow \,q{{\bar{q}}}\) at NNLO
Let us first consider the kinematics of decay of \(\gamma ^{*}\rightarrow q{\bar{q}}\), in which, to keep a simple structure at integrand level, we work with massless particles in the loop. We remark that this choice does not generate difficulties in the evaluation of integrals, within the ltd framework. The latter pattern is because of the way how integrands are expressed, which are inherit of the masses. Equivalently, their dependence is stored in the fixed energy components, \(q_{i,0}^{\left( +\right) }\). Hence, here and in the following processes, \(k=p_{1}+p_{2}+\cdots +p_{n}\), with \(p_{i}^{2}=0,k^{2}=s\) and \(n\le 4\). With this notation in mind, the squared tree-level amplitude can be cast as,
with \(Q_{q}=\frac{1}{3},-\frac{2}{3}\) and \(N_{c}\) the electric charge and the colour number of the quarks, respectively.
3.4.1 Virtual contributions
The contribution at one-loop, after only performing Dirac algebra and expressing scalar product in terms of denominators, becomes,
with,
As mentioned in the former sections, we would like to emphasise that within ltd and, therefore, fdu, scaleless integrals are not set directly to zero as conventionally carried out in dreg. The main reason to do this is to achieve a complete cancellation of singularities at integrand level by kee** as much as possible control on the local structure of the integrands. Thus, if we were in dreg, one finds that the second line in Eq. (3.24) vanishes and,
These relations amount to
where it is straightforward to identify the IR and UV singularities, which come from \(I_{111}^{\left( 1\right) }\) and \(I_{101}^{\left( 1\right) }\), respectively. This is indeed what is traditionally carried out by means of the tensor reduction.
In ltd, we keep scaleless integrals to perform a local UV renormalisation as well as IR subtraction from real corrections, following the lines of the fdu scheme. Thus, applying ltd to Eq. (3.24),
with
where,
We remark that the integrands of Eq. (3.28) are computed, without the loss of generality, in the center-of-mass frame, allowing us to have \(q_{3,0}^{\left( +\right) }=q_{1,0}^{\left( +\right) }\). Additionally, the integrands (3.29) are expressed in the multi-loop ltd representation, displaying structure depending only on physical singularities. A noteworthy comment on the structure of (3.29) is in order. The structure of these integrands can easily be related to one-, two- and three-point functions, which have been explicitly computed, independently on the number of loops, in [55]. Although there a simple recipe for the calculation of dual integrals through ltd, it as possible to profit of the explicit causal structure of multi-loop topologies. This is indeed what is carried out in the two- and three-point integrands, where their structure correspond to the Maximal-Loop and Next-to-Maximal-Loop topologies, respectively. A detailed discussion of the structure and the features of these topologies is presented in [55, 57, 68]. Interestingly from Eq. (3.29), the treatment of physical thresholds is straightforward because of the structure the latter hold. In this configuration, in fact, it is possible to obtained up to two “entangled causal” thresholds that are observed from the structure of \(\lambda _{i}^{\pm }\).
Hence, by following the idea presented in the one-loop case, we generate the two-loop contribution, in which we elaborate, for the sake of simplicity, on the two-loop integral,
with
Let us remark that the integrand obtained from the Feynman diagram depicted in Fig. 4c one only has five propagators, the additional ones, \(q_{6}\) and \(q_{7}\), are needed to express all scalar products in terms of denominators. This is done to simplify the structure of the integrand, but it is not mandatory and this step can be avoided. In fact, the explicit dependence on the energy component of the loop momenta can always pull out.
Hence, with the above considerations, the two-loop contribution turns out to be,
These two-loop integrals can be further reduced by means of the integration-by-parts identities (IBPs). Moreover, in order not to alter the local structure of the integrands, we do not make use of the zero sector symmetries and loop momentum redefinition. This is done to carefully combine and thus match virtual with real corrections.
3.4.2 Real contributions
In this section, we list the tree-level amplitudes that are needed to perform a cancellation of the IR singularities, \(\gamma ^{*}\rightarrow q{\bar{q}}g\) and \(\gamma ^{*}\rightarrow q{\bar{q}}q'{\bar{q}}'\).
\(\varvec{\gamma ^{*}\rightarrow q{\bar{q}}g}\)
with \(s_{123}=s_{12}+s_{23}+s_{13}\).
\(\varvec{\gamma ^{*}\rightarrow q{\bar{q}}q'{\bar{q}}'}\)
In this equation, we have not performed any additional collection of terms.
It is remarkable the simplicity of the full squared amplitudes displayed in (3.33) and (3.34). However, to keep track of the divergencies, within the ltd/fdu framework, one can consider individual Feynman diagrams and perform the match between real and virtual corrections. In fact, by kee** the ordering of the diagrams depicted in Fig. 5, one notices that, when squaring the amplitude, the interference terms account for contributions coming from the virtual diagrams. While the remaining diagrams account for the wave function corrections.
3.4.3 Map**s
As stated in the former sections, one of the distinctive features of the fdu approach is the real-virtual integrand-level combination through kinematical map**s. At NNLO, these map**s should relate the one- and two-loop amplitudes with the double-real emission terms. A similar strategy to the phase-space slicing method must be applied, but now there will be more singular regions, which might also overlap. In this way, a generic NNLO map** could be a complicated transformation with highly non-trivial dependencies.
However, when computing the NNLO QCD corrections proportional to \(N_f\) for the process \(\gamma ^* \rightarrow q {{\bar{q}}}\), a huge simplification takes place: only the double-virtual (i.e. two-loop) and the double-real emission contributes. Thus, we will need a transformation to generate a \(1 \rightarrow 4\) physical configuration starting from double-cuts, which are described by a physical \(1 \rightarrow 2\) process plus two additional on-shell momenta (i.e. the cut lines, \(q_i\) and \(q_j\)). A preliminar proposal for such map** is the following,
where momentum conservation is automatically fulfilled and the coefficients \(\alpha _i\) and \(\alpha _j\) must be adjusted to verify that \((p'_1)^2=p_1^2\) and \((p'_2)^2=p_2^2\). We can appreciate that the cut lines behave as real final state radiation, and we expect this transformation to link the IR singularities present in both contributions to achieve a fully local cancellation in four space-time dimensions.Footnote 11
3.5 Discussion
The ultimate goal of the ltd/fdu framework consists in achieving a fully local regularisation of both IR and UV singularities, thus leading to a four-dimensional representation of physical observables. The key observations are:
-
IR singularities cancel among virtual and real contributions, which is supported by the well-known KLN theorem;
-
and IR singularities inside the dualised virtual terms can be isolated into a compact region of the integration domain.
In this way, the singular regions in both contributions can be mapped to the same points, leading to a complete cancellation and skip** the need of introducing additional regularisation techniques (such as dreg). Alternatively, we can think that real emission is being used as an IR local counterterm for the dual contributions. Of course, renormalisation counterterms must be introduced, as well as potential initial-state radiation (ISR) subtraction terms.
Since the first proof-of-concept of the fdu framework, we have developed several new strategies to tackle the problem of obtaining integrable representations of IR-safe observables in four space-time dimensions. In particular, during last year, we have progressed a lot in understanding the location of IR and threshold singularities in the virtual amplitudes, as well as elucidating a novel dual representation through the application of nested residues. The path looks very promising to address some of the current limitations of our approach, namely a fully automated multi-loop local renormalisation and the cancellation of ISR singularities in a universal way. Other strategies, such as \(q_T\)-subtraction/resummation have shown to be perfectly adapted to attack these problems, although they still lack of locality. Thus, we believe that a conceptual combination of other methodologies might shed light to solve the current limitations to extend the ltd/fdu framework.
4 IREG: implicit regularisation
Envisaging beyond NLO calculations, let us generalise the procedure discussed in [16] within the non-dimensional ireg framework. We summarise the rules applicable to a general n-loop Feynman amplitude \({{{\mathcal {A}}}}_N^{(L)}\) with N external legs. Let \(k_l\) be the internal (loop) momenta (\(l= 1,\ldots , L\)) and \(p_i\) be the external momenta. After performing the usual Diracology and spacetime algebra in the physical dimension and internal symmetry contractions, the UV content of \({{{\mathcal {A}}}}_N^{(L)}\) can be cast in terms of well-defined basic divergent integrals (BDI’s), which are independent on the physical momenta. In order to define a massless renormalisation scheme, the explicit mass dependence in the BDI’s can be removed via regularisation independent identities which gives rise to a renormalisation scale. It was shown in [75] that BDI, as defined in ireg, comply with the Bogoliubov–Parasiuk–Hepp–Zimmerman (BPHZ) program [1, 76,77,78,79], which is a consistent renormalisation program applicable to arbitrary loop order in perturbation theory. Based on the topology of a Feynman graph, the subtraction of UV divergences is organised by the Zimmermann’s forest formula in a regularisation independent way. The forest formula can be cast into a counterterm language by means of Bogoliubov’s recursion formula, respecting locality, Lorentz invariance, unitarity and causality. Thus, in ireg, after subtracting, using the counterterms of lower \((n-1)^{th}\), the \(n^{th}\)-order counterterms can themselves be cast as BDI, without explicit evaluation.
Clearly care must be exercised as the symmetry content of the underlying model increases because finite regularisation dependent terms can lead to spurious symmetry breakings. In order to evaluate finite Green’s functions in a symmetry preserving fashion, the BPHZ program allied to quantum action principles can be used for an all-order proof of renormalisability of gauge field theories. By adopting a gauge invariant scheme a general proof can be constructed in a minimal subtraction scheme and then generalised to arbitrary gauge invariant schemes [80, 81]. A proof for all order abelian gauge invariance of ireg can be found in [82].
4.1 IREG procedure
The steps that accomplish the above mentioned issues are as follow.
-
1.
Perform the internal symmetry group and the usual Dirac algebra in the physical dimension avoiding symmetric integration in divergent amplitudes as such an operation is ambiguous [83]. The anticommutation \(\{\gamma _5,\gamma _\mu \}=0\) inside divergent amplitudes must not be used even in the physical dimension as they lead to spurious terms as well [22, 84, 85].
-
2.
Starting at one loop remove external momenta dependence from the divergent part of the amplitude by applying the identityFootnote 12
$$\begin{aligned}&\frac{1}{(k_{l}-p_{i})^2-\mu ^2}\nonumber \\&\quad =\sum _{j=0}^{n_{i}^{(k_{l})}-1}\frac{(-1)^{j}(p_{i}^2-2p_{i} \cdot k_{l})^{j}}{(k_{l}^2-\mu ^2)^{j+1}}\nonumber \\&\qquad +\frac{(-1)^{n_{i}^{(k_{l})}}(p_{i}^2-2p_{i} \cdot k_{l})^{n_{i}^{(k_{l})}}}{(k_{l}^2-\mu ^2)^{n_{i}^{(k_{l})}} \left[ (k_{l}-p_{i})^2-\mu ^2\right] }, \end{aligned}$$(4.1)in the propagators, where \(n_i\) is chosen so that the internal momentum \(k_l\) of the l-th loop renders the integral power counting ultraviolet finite. Logarithmically BDI’s appear asFootnote 13
$$\begin{aligned}&I_{log}(\mu ^2)\equiv \int _{k} \frac{1}{(k^2-\mu ^2)^{2}},\nonumber \\&I_{log}^{\nu _{1} \cdots \nu _{2r}}(\mu ^2)\equiv \int _k \frac{k^{\nu _1}\cdots k^{\nu _{2r}}}{(k^2-\mu ^2)^{r+2}}, \end{aligned}$$(4.2)with the definition \(\int _{k} = \int \frac{ d^{4} k}{(2 \pi )^{4}} \). The UV finite part in the limit where \(\mu \) approaches zero from above \(\mu \rightarrow 0\) has logarithmical dependence in the physical momenta which is the characteristic behaviour of the finite part of massless amplitudes.
-
3.
BDI’s with Lorentz indices \(\nu _{1} \cdots \nu _{2r}\) may be written as linear combinations of BDI’s without Lorentz indices plus well defined surface terms (ST’s), e.g.
$$\begin{aligned} \Upsilon _{0}^{(1)\mu \nu }= & {} \int _k\frac{\partial }{\partial k_{\mu }}\frac{k^{\nu }}{(k^{2}-\mu ^{2})^{2}}\nonumber \\= & {} 4\Bigg [\frac{g_{\mu \nu }}{4}I_{log}(\mu ^2)-I_{log}^{\mu \nu }(\mu ^2)\Bigg ], \end{aligned}$$(4.3)ST’s vanish if and only if momentum routing invariance (MRI) holds in the loops of Feynman diagrams. Moreover these requirements automatically deliver gauge invariant amplitudes [90,91,92,93,94] which has been demonstrated for abelian gauge theories to arbitrary loop order [82, 84] and verified for non-abelian gauge models [95,96,97]. Rephrasing it, unless MRI is verified, a symmetric integration leads to a finite definite value for the arbitrary surface term which potentially breaks (gauge) symmetry. By performing a general routing calculation it can be shown that setting ST’s=0 cancels routing dependent terms (which they systematically multiply), see e.g. [82]. This may explain why dimensional regularisation, where surface terms vanish in d dimensions, ensures MRI.Footnote 14
-
4.
An arbitrary positive (renormalisation group) mass scale \(\lambda \) appears via regularisation independent identities,
$$\begin{aligned} I_{log}(\mu ^2) = I_{log}(\lambda ^2) + \frac{i}{(4 \pi )^2} \ln \frac{\lambda ^2}{\mu ^2}, \end{aligned}$$(4.4)which enables us to write a BDI as a function of \(\lambda ^2\) plus logarithmic functions of \(\mu ^2/\lambda ^2\). The BDI can be absorbed in the renormalisation constants [100] and renormalisation functions can be computed using the regularisation independent identity:
$$\begin{aligned} \lambda ^2\frac{\partial I_{log}(\lambda ^2)}{\partial \lambda ^2}= -\frac{i}{(4 \pi )^2}. \end{aligned}$$(4.5)
At two-loop order a similar program can be devised, which allows to express the UV divergent content in terms of BDI in one loop momentum only. As an example, consider an UV divergent two-loop massless scalar integral
Following the algorithm proposed in [75], one identifies the different regimes in which the internal momenta can go to infinity (\(k_{1}\rightarrow \infty \), \(k_{2}\) fixed; \(k_{2}\rightarrow \infty \), \(k_{1}\) fixed; \(k_{1}\rightarrow \infty \), \(k_{2}\rightarrow \infty \)); for each case, uses identity (4.1) in the internal momenta that goes to infinity regarding all other momenta as external. This procedure allows to automatically identify the UV-counterterms required by Bogoliubov’s recursion formula in terms of BDI’s. Explicitly,
where the function \({\bar{H}}_{1}\) contains terms generated by integrating in \(k_{2}\), and similarly to \({\bar{H}}_{2}\). In this example, the first two terms are going to be canceled by 1-loop counterterms while the last one will contribute to the 2-loop counterterm. Further contributions to the 2-loop counterterm are also automatically identified, which will be of the form
or integrals in \(k_{1}\) (\(k_{2}\)) with no dependence on the scale \(\lambda \). The above integrals give rise to BDI’s of two-loop order defined by
This approach can be extended to tensorial and/or arbitrary loop order integrals, as sketched by the steps below
-
1.
At higher loop order the divergent content can be expressed in terms of BDI in one loop momentum after performing \(n-1\) integrations. The order of such integrations is chosen systematically to display the counterterms to be subtracted in compliance with the Bogoliubov’s recursion formula [1, 75,76,77,78,79]. The general form of the terms of a Feynman amplitude after l integrations is
$$\begin{aligned}&I^{\nu _{1}\ldots \nu _{m}}\!=\!\!\int \limits _{k_{l}}\!\frac{A^{\nu _{1}\ldots \nu _{m}}(k_{l},q_{i})}{\prod _{i}[(k_{l}-q_{i})^{2}-\mu ^{2}]}\ln ^{l-1}\!\left( \!-\frac{k_{l}^{2}-\mu ^{2}}{\lambda ^{2}}\right) \!, \end{aligned}$$(4.10)where \(l=1, \ldots , n\) and \(q_{i}\) is an element (or combination of elements) of the set \(\{p_{1},\ldots ,p_{L},k_{l+1},\ldots ,k_{n}\}\). \(A^{\nu _{1}\ldots \nu _{m}}(k_{l},q_{i})\) represents all possible combinations of \(k_{l}\) and \(q_{i}\) compatible with the Lorentz structure.
-
2.
Apply relation (4.1) in (4.10) by choosing \(n_{i}^{(k_{l})}\) such that all divergent integrals are free of \(q_{i}\). Therefore, the divergent integrals are cast as a combination of
$$\begin{aligned} I_{log}^{(l)}(\mu ^2)&\equiv \int \limits _{k_{l}} \frac{1}{(k_{l}^2-\mu ^2)^{2}} \ln ^{l-1}{\left( -\frac{k_{l}^2-\mu ^2}{\lambda ^2}\right) },\quad \end{aligned}$$(4.11)$$\begin{aligned} I_{log}^{(l)\nu _{1} \cdots \nu _{2r}}(\mu ^2)&\equiv \int \limits _{k_{l}} \frac{k_{l}^{\nu _1}\cdots k_{l}^{\nu _{2r}}}{(k_{l}^2-\mu ^2)^{r+1}} \ln ^{l-1}{\left( -\frac{k_{l}^2-\mu ^2}{\lambda ^2}\right) }, \end{aligned}$$(4.12)The surface terms derived from higher loop BDI’s are obtained through the identity
$$\begin{aligned} \Upsilon _{2i}^{(l)\nu _{1}\cdots \nu _{2j}}&\equiv \int _k\frac{\partial }{\partial k_{\nu _{1}}}\frac{k^{\nu _{2}}\cdots k^{\nu _{2j}}}{(k^{2}-\mu ^{2})^{1+j-i}}\ln ^{l-1}\nonumber \\&\quad \times \Bigg [-\frac{(k^{2}-\mu ^{2})}{\lambda ^{2}}\Bigg ]. \end{aligned}$$(4.13)For instance,
$$\begin{aligned} I_{log}^{(l)\,\mu \nu }(\mu ^2)&=\sum _{j=1}^{l}\left( \frac{1}{2}\right) ^j\!\frac{(l-1)!}{(l-j)!}\!\nonumber \\&\quad \times \left\{ \frac{g^{\mu \nu }}{2}I_{log}^{(l-j+1)}(\mu ^2)-\frac{1}{2}\Upsilon _{0}^{(l)\,\mu \nu }\right\} . \end{aligned}$$(4.14) -
3.
A renormalisation group scale is encoded in BDI’s. At \(\hbox {n}^{th}\)-loop order a relation analogous to (4.4) is obtained via the regularisation independent identity
$$\begin{aligned} I_{log}^{(l)}(\mu ^2)&=I_{log}^{(l)}(\lambda ^2) -\frac{b}{l}\ln ^{l}\left( \frac{\mu ^2}{\lambda ^2}\right) \nonumber \\&\quad - b \sum _{j=1}^{l-1}\frac{(l-1)!}{(l-j)!}\ln ^{l-j}\left( \frac{\mu ^2}{\lambda ^2}\right) , \end{aligned}$$(4.15)where \(\lambda ^{2}\ne 0,\;\; b \equiv \frac{i}{(4\pi )^2}\).
-
4.
BDI’s can be absorbed in renormalisation constants. A minimal, mass-independent scheme amounts to absorb only \(I_{log}^{(l)}(\lambda ^2)\). To evaluate RG constants, BDI’s need not be explicitly evaluated as their derivatives with respect to the renormalisation scale \(\lambda ^2\) are also BDI’s. For example [82],
$$\begin{aligned} \lambda ^2\frac{\partial I_{log}^{(n)}(\lambda ^2)}{\partial \lambda ^{2}}&=-(n-1)\, I_{log}^{(n-1)}(\lambda ^2)- b \,\, \alpha ^{(n)},\nonumber \\ \lambda ^2\frac{\partial I_{log}^{(n)\,\mu \nu }(\lambda ^2)}{\partial \lambda ^{2}}&=-(n-1)I_{log}^{(n-1)\,\mu \nu }(\lambda ^2) -\frac{g_{\mu \nu }}{2} \, b \, \beta ^{(n)}. \end{aligned}$$(4.16)where \(n \ge 2\), \(\alpha ^{(n)} = (n-1) !\) and \(\beta ^{(n)}\) may be obtained from \(\alpha ^{(n)}\) via relation (4.14).
4.2 Disentangling UV and IR divergences: a two-loop example
We consider the process \(\gamma ^{*}\rightarrow q{\bar{q}}\) at two-loop level. In order to illustrate the method, we evaluate the contribution that contains a quark loop of flavour d and external quarks of flavour b as depicted in Fig. 6. The amplitude can be written as
where \(\int _q = \int d^4 q/(2 \pi )^4\) and we have shifted the momentum variables such that \(q_1 \rightarrow q_1'=-(q_1 -p_2)\) and relabelled \(q_1'\) back to \(q_1\). N in the numerator stands for

\(Q=-1/3\), (a, b) are colour indices of the external quarks, \(C_{F}\) is the quadratic Casimir for the fundamental representation and \(\mu \) is a fictitious mass for the gluon propagator.
The integration over \(q_2\) is performed according to the ireg rules, by first applying Eq. (4.1) to separate its divergent content, which is expressed as an internal \(I_{log}(\lambda ^2)\), from the finite contribution encoded as \(Z_0(p^2, m_1^2, m_2^2)\) plus possible local terms, both multiplying the \(q_1\) integrand, as follows
where

Here
and we have used relation (4.4). Notice that \(Z_{0}\) is scale \(\lambda \) dependent in the first term in the square brackets of (4.20), and independent on it in the second term in the brackets.
According to the features of BDI mentioned in the beginning with respect to the Bogoliubov’s recursion formula, the term proportional to \(I_{log} (\lambda ^2)\) in (4.20) corresponds to a subdivergence and is exactly cancelled by the counterterm graph corresponding to the one mass-independent renormalisation of the down-quark loop.
Thus \( \widetilde{{{\mathcal {M}}}}^\mu _{ab}\), the amplitude which defines the two-loop order UV divergence after subtracting the subdivergences, is obtained by substituting \({{{\mathcal {I}}}}^\mu \) by

to define \(\widetilde{{{{\mathcal {M}}}}}^\mu _{ab}\) as depicted in Fig. 7.
In the next section we address the two-loop order specific UV divergences and their removal.
4.2.1 Virtual contribution: UV part
In this particular example, after the removal of the one-loop subdivergence, the divergent part of the amplitude is either UV or IR divergent, there is no overlap of UV and IR divergent contributions. The two-loop UV renormalisation constant can be extracted as a BDI from the UV divergent part of \({\widetilde{\Sigma }}^\mu \) (remember definition in (4.19)):

Using the rules of IReg, we isolate a BDI as a UV bare bone object free of masses by taking the limit where \(q_1 \gg m_d\) in \(Z_0 (q_1^2,m_d^2,\lambda ^2)\) to yield

The integrals above are only UV-logarithmic divergent, therefore, the BDI’s can be easily extracted as
where we made use of Eqs. (4.14) and (4.15) while setting ST’s to zero. Finally, the two-loop UV counterterm corresponding to this amplitude reads
Upon subtracting it from \(\widetilde{{{{\mathcal {M}}}}}^\mu _{ab}\), defined by (4.19) with (4.22), one obtains the renormalised amplitude. The next task is to identify the infrared divergences in the renormalised amplitude.
4.2.2 Virtual contribution: IR part
In Table 1, we summarise the results which are relevant to isolate the IR divergences of different natures as \(\ln ^n\mu \) as \(\mu \rightarrow 0\) in a non-dimensional regularisation scheme.Footnote 15 The IR divergent part is parametrized as \(\ln \) of the parameter \(\mu ^2\). Notice that this is a fictitious mass introduced in propagators to regularise the IR div. We have made use of FeynCalc [101,102,103] and Package-X [104] to compute the integrals. Moreover in order to express the function \(Z_0 (k^2, m_1^2, \lambda ^2)\) in a propagator like form we have used \(Z_0 = \int (\partial Z_0 / \partial m_1^2) d m_1^2\), with
Notice that since one is dealing with massive quarks, the integral in the Feynman parameter x of (4.21) is more involved as opposed to the example of the electron self-energy we performed in section 4.1 of [16].
Putting all the results displayed in Table 1 in the amplitude we finally obtain for its infrared content
with
The expression in (4.28) corresponds to the IR-divergent part of the amplitude that is going to be cancelled when adding the adequate real contributions to the process \(\gamma ^{*}\rightarrow q{\bar{q}}\), leading to scales and finite terms that must still be obtained.
4.3 Discussion
One of the main goals of the ireg scheme is to represent the UV-divergent content of a given multi-loop Feynman integral in terms of well-defined Basic Divergent Integrals (BDI) which do not need to be explicitly evaluated. Such program is compatible with the BPHZ theorem, assuring locality, causality and Lorentz invariance. Further understanding of the relation between ireg and dimensional schemes was achieved recently [97], with prospects to map BDI’s into poles in \(\epsilon ^{-n}\) for a UV n-loop calculation in general.
Regarding symmetries, the method complies with abelian gauge symmetry at arbitrary loop level, and non-abelian theories have also been tested up to two-loop level. A general proof based on the quantum action principle is still lacking, however, some of the main ingredients were proved to be fulfilled by the method in recent years [22]. Besides, a more general picture of dimension-specific objects and their properties (such as the \(\gamma _{5}\) matrix) in the context of four-dimensional schemes has emerged. In particular, it was shown, quite surprisingly, that consistent four-dimensional methods such as ireg need to deal with \(\gamma _{5}\) problems, in a way similar to dimensional schemes.
Finally, from the point of view of infrared divergences, some proof-of-principle computations are still lacking for a NNLO calculation. In particular, the knowledge of the double-real and virtual-real contributions for the process studied in this work is underway.
5 Local analytic sector subtraction: the Torino scheme
This section is devoted to the Local Analytic Sector subtraction scheme that has been recently proposed [105] for NLO and NNLO QCD calculations with coloured particles in the final state only. In order to present the NLO implementation of Local Analytic Sector subtraction we start by introducing a generic differential cross section with respect to an IR-safe observable X
where d is the space-time dimension set equal to \(4-2\epsilon \), with \(\epsilon <0\), and n is the number of final state, coloured partons involved in the Born process. The symbol \(d\Phi _i\) identifies the i-body phase space, while \(V_n\) is the UV-renormalised one loop correction and \(R_n\) is the tree level squared amplitude for a single real radiation. Finally, \(\delta _i(X) \equiv \delta (X-X_i)\) sets the observable X to be computed in the i-body kinematics. In dimensional regularisation the virtual matrix element features up to a double pole in \(\epsilon \), while the real correction, finite in \(d=4\), is characterised by up to two singular limits of IR nature in the radiation phase space. By integrating R in d dimensions over the phase space, its implicit singularities become manifest as \(1/\epsilon \) poles.
Although the sum on the r.h.s. of Eq. (5.1) is finite in \(d=4\) thanks to the KLN theorem [69, 70], it is unfeasible to estimate the differential distribution in this form. The complexity of a typical collider process requires to exploit numerical algorithms to treat the phase space integration. Indeed the integration over the unresolved phase space has to be performed in \(d=4\), therefore the IR singularities must be canceled prior to the integration: this goal can be achieved via a subtraction method.
The subtraction procedure consists in adding ad subtracting in Eq. (5.1) a counterterm that reproduces the singular behaviour of the real matrix element, and can be integrated analytically in the single-radiative phase space. Such a counterterm and its integrated counterpart can be defined in full generality as
with \(d\Phi _{\mathrm{rad}}= d\Phi _{n+1}/d\Phi _{n}\) being the factorised single-radiative phase space. The subtracted differential cross section then reads
where both terms in squared brackets are separately finite and integrable in \(d=4\).
The specific implementation of the counterterm is not unique and requires various technical aspects, which characterise the different subtraction methods. Several solutions to the IR subtraction problem are indeed already available and well tested at NLO, such as the schemes by Frixione-Kunszt-Signer [74, 106] (FKS) and Catani-Seymour (CS) [73, 107]. At NNLO the variety of subtraction procedures is much richer [5, 9, 15, 44, 108,109,110,111,112], but despite this considerably wide range of sophisticated schemes, many of them rely on demanding numerical calculations or involved integration procedures. In order to overcome such bottlenecks, the local analytic sector subtraction scheme provides an alternative NLO subtraction method that aims at combining the most advantageous aspects of the FKS and the CS schemes, complementing a minimal subtraction structure with an efficient integration strategy. These key aspects are suitable for a natural generalisation to NNLO.
We stress that all matrix elements entering the NLO and the NNLO corrections, are assumed to be treated with conventional dimensional regularisation, and renormalised within the \(\overline{\mathrm{MS}}\) scheme. Accordingly, the IR kernels that enter the counterterms have been then computed with the same regularisation approach. The radiative phase space parameterisation and the consequent integration strategy have been conveniently chosen according to dimensional regularisation. Changing the regularisation scheme would mean re-thinking the counterterm definition (and integration), as well as the precise correspondence between different contributions to the NNLO computation.
5.1 NLO subtraction
The key characteristic of the Local Analytic Sector subtraction at NLO is the locality of the counterterm \({{\overline{K}}}_{n+1}\) as well as the analytic procedure adopted to compute its integrated counterpart \(I_n\), as introduced in Eq. (5.2). The counterterm has indeed to mimic the infrared behaviour of the real matrix element locally in the phase space, and at the same time, it has to be simple enough to allow for analytic integration in the single-unresolved phase space. These two fundamental features are also crucial for the NNLO generalisation, as will be explained in Sect. 5.2. To present the method in its core structure at NLO, we introduce the following notation: we set the squared centre-of-mass energy to be s, the centre-of-mass four momentum to be \(q^\mu =\big (\sqrt{s}, \vec {0} \, \big )\), and \(k_i^\mu \) the i-th final state momentum. Moreover, the Lorentz invariants \(s_{ab}=2 k_a \cdot k_b\), the energy fraction \(e_{i}=s_{qi}/s\) and the angular variable \(w_{ij}=s \, s_{ij} /{s_{qi} \, s_{qj}}\) are also mentioned below. The singular soft and collinear behaviour of the real matrix element is extracted by the relevant projector operators,
To appropriately define the desired counterterms, we partition the real-radiation phase space into sectors by means of sector functions \({\mathcal {W}}_{ij}\) (\(i, j = 1, \dots , n ; \, i\ne j\)), inspired by the FKS scheme [74]. In analogy to FKS, the sectors functions are designed to fulfil a set of fundamental properties:
- 1.:
-
they have to be a unitary partition of the phase space: \(\sum _{i, j\ne i} {\mathcal {W}}_{ij}=1\),
- 2.:
-
they have to select a minimum number of singularities in each sector: \({\mathbf{S}}_{i} {\mathcal {W}}_{ib} \ne 0, \, {\mathbf{S}}_{i} {\mathcal {W}}_{ab} =0 , \, \forall i \ne a \, ; \, {\mathbf{C}}_{ij} {\mathcal {W}}_{ij} \ne 0, \, {\mathbf{C}}_{ij} {\mathcal {W}}_{ji} \ne 0 \, ; \, {\mathbf{C}}_{ij} {\mathcal {W}}_{ab} =0 , \, \forall ab \notin \{i,j\}\),
- 3.:
-
the sum over sectors sharing the same singular configurations has to be one: \({\mathbf{S}}_{i} \sum _{k\ne i} {\mathcal {W}}_{ik} =1 , {\mathbf{C}}_{ij} \big ( {\mathcal {W}}_{ij} + {\mathcal {W}}_{ji} \big )=1\).
These constraints must hold for any explicit definition of \({\mathcal {W}}_{ij}\). An efficient realisation of such sector functions is given by the following Lorentz invariants ratios,
Then, sector by sector, the singular regimes of the real matrix element are collected into a candidate counterterm \(K_{n+1}\)
where the last term in round brackets avoids the double subtraction of the mixed soft-collinear divergences. As it is evident from the expression above, the introduction of sectors enables a minimal definition for \(K_{ij}\): a remarkable feature that can be generalised also at NNLO.
Given the factorised structure of the real matrix element under unresolved limits, each contribution appearing in Eq. (5.6) can be written as a universal singular kernel and an appropriate Born-like matrix element (for a review see for instance [\(n+1\) phase space into a single radiative phase space \(d\Phi _{\mathrm{rad}}\) and a remaining n-body resolved phase space, so that the counterterm can be integrated only over the former. Moreover, with a momentum map** we force the Born kinematics to be on-shell and momentum conserving in the entire phase space.
There is ample freedom in the map** procedure, that can be exploited to simplify the integration procedure. To this aim, we introduce a Catani-Seymour final state map** [73], and define a set of n on-shell momenta \(\{{{\bar{k}}}\}^{(abc)}\) by choosing three final-state momenta \(k_a, k_b, k_c\) and combining them as

The notation  states that we are eliminating the momenta \(k_i\), with \(i=a,b,c\), from the initial set of \(n+1\) momenta. By choosing a, b, c according to the specific counterterm contribution, its integration can be carried out analytically with standard techniques. The phase space factorises consequently in terms of the Catani-Seymour parameters \(y=s_{ab}/s_{abc} , \, z=s_{ac}/(s_{ac}+s_{bc})\), where \(0\le y \le 1\) and \(0\le z \le 1\), as
states that we are eliminating the momenta \(k_i\), with \(i=a,b,c\), from the initial set of \(n+1\) momenta. By choosing a, b, c according to the specific counterterm contribution, its integration can be carried out analytically with standard techniques. The phase space factorises consequently in terms of the Catani-Seymour parameters \(y=s_{ab}/s_{abc} , \, z=s_{ac}/(s_{ac}+s_{bc})\), where \(0\le y \le 1\) and \(0\le z \le 1\), as
with \(\phi \) being the azimuthal angle between \(\vec k_a\) and an arbitrary three-momentum taken as reference direction. We stress that, referring to Eq. (5.9), the integration involves only the variables occurring after the semicolon, while the remaining variables indicate a functional dependence. Thus, the single radiative phase space can be written as
As already mentioned, we are free to choose partons a, b, c differently for each contribution to the counterterm. In particular, for the soft limit we set \(a=i\) and \(b=l, c=m\), where i identifies the soft parton, and l, m the emitters. For the hard-collinear component, the natural choice is \(a=i\), \(b=j\) and \(c=r\), with i, j being the collinear partons, and r an on-shell spectator different from i and j. In the remapped kinematics (remapped quantities are identified with a bar) the contributions to Eq. (5.6) are then given by
Here \({\mathcal {I}}_{lm}^{(i)}= \delta _{f_i g} \, s_{lm}/(s_{il} \, s_{im})\) is the eikonal kernel relative to parton i, \(B_{lm}\) is the colour-correlated Born matrix element, \(P_{ij}^{\mu \nu }\) is the spin-dependent Altarelli–Parisi splitting function, \(B_{\mu \nu }\) is the spin-correlated Born matrix element, \(C_{f_j}\) is the quadratic Casimir relevant for the colour representation of parton j and \({\mathcal {N}}_1= 8\pi \alpha _{s}(\mu ^2 e^{\gamma _E}/(4\pi ))^\epsilon \) is a normalisation factor. It is important to notice that the remapped contributions in Eq. (5.11) are not uniquely defined. Any definition of the barred counterterm is indeed acceptable, provided it fulfils a set of consistency relations. Such relations ensure \({{\overline{K}}}_{n+1}\) to reproduce the correct behaviour of \(R_{{n+1}}\) in all singular regions of the real phase space. These constraints reduce to the following set of relations
which are verified by the definitions in Eq. (5.11).
Before integrating over the unresolved phase space, the sum over sectors appearing in Eq. (5.6) can be organised according to
where the combinations in square brackets have been reduced to one, thank to the \({\mathcal {W}}_{ij}\) properties, preventing the sectors to affect the integration procedure. We are then left with the evaluation of the integrated counterterm \(I_{n}\). To maximally facilitate this crucial step, we choose to parametrise the phase space according to the kinematic map** adopted for each contribution, as done in the Catani–Seymour scheme. Considering for instance the soft contribution, the integration proceeds trivially,
where the factor \({\mathcal {N}}_1\) is omitted for brevity. Similar approach can be also applied for the hard-collinear component, choosing \(a=i, b=j, c=r\) in Eq. (5.9) [5.2 NNLO subtraction To generalise the subtraction method to NNLO [114]. In the first stages of the method implementation [105] some key elements were missing to provide an efficient subtraction method at NNLO: the treatment of the real-virtual singularities and the integration of the double unresolved counterterm. The lack of such ingredients obviously affects the possibility to test the method for arbitrary processes. Efforts are ongoing to tackle the above mentioned missing ingredients, towards a general validation of the scheme [115]. At NNLO the subtraction pattern manifests a non-trivial degree of complexity, due to the increased number of contributions to a generic observable X, where \(VV_{n}\) is the UV-renormalised double-virtual matrix element, \(RV_{n+1}\) is the real-virtual correction and \(RR_{n+2}\) is the double-real configuration. As a consequence, more counterterms are needed to cancel all the singularities arising from the unresolved radiation, and delicate cancellations have also to occur amongst the counterterms themselves, to enable a minimal and transparent pattern. To account for the double-real singularities, we introduce \({\overline{K}}^{(1)}\) that encodes the single-unresolved configurations, and the combination \({\overline{K}}^{(2)}-{\overline{K}}^{(12)}\) which cures the double-unresolved limits. In particular, \({\overline{K}}^{(2)}\) collects the homogenous limits, i.e. those configurations where the two unresolved partons become soft/collinear at the same rate, while \({\overline{K}}^{(12)}\) mimics the ordered limits, where one (one pair of) parton is more unresolved than the others. Finally, the unresolved regions of the real-virtual phase space are caught by \({\overline{K}}^{{{(\mathrm RV)}}}\). Each counterterm has to be integrated over the corresponding unresolved phase space, as prescribed by the definitions where \(d\Phi _{{\mathrm{rad}},2}=d\Phi _{n+2}/d\Phi _n\), \(d\Phi _{{\mathrm{rad}},1}=d\Phi _{n+2}/d\Phi _{n+1}\) and \(d\Phi _{\mathrm{rad}}=d\Phi _{n+1}/d\Phi _n\). The subtraction pattern at NNLO then reads As anticipated, the last line is finite in the whole phase space by construction, and therefore it can be evaluated in \(d=4\) dimensions. In the second line, the combination \(RV_{n+1}- {\overline{K}}^{{{(\mathrm RV)}}}\) is free of phase space divergences, but both terms manifest explicit pole in \(\epsilon \), that do not cancel in the sum. Those poles are subtracted in a non-trivial way: \(I^{(1)}\) exposes the same \(1/\epsilon \) poles as RV, due to a straightforward consequence of the KLN theorem, while we can properly define \({\overline{K}}^{(12)}\), such that its integrated counterpart reproduces the same explicit poles as \({\overline{K}}^{{{(\mathrm RV)}}}\). We stress that, in order to have the second line in Eq. (5.15) finite in \(d=4\), the integrated counterterm \(I^{(12)}\) has to play a double role. First, it has to cancel the explicit poles of the real-virtual counterterm. Second, it has to feature the same phase space singularities as \(I^{(1)}\) (up to a sign). This is in principle not guaranteed by the KLN and indeed requires a delicate interplay between the definition of \({\overline{K}}^{(12)}\) and \({\overline{K}}^{{(\mathrm RV)}}\). Finally, in the first line the combination \(I^{{(\mathrm RV)}}+I^{(2)}\) returns the explicit singularities of the double virtual matrix element. Provided that proper counterterms are defined to satisfy the cancellations just described, the three lines in Eq. (5.15) are finite in \(d=4\) and can be computed separately with numerical algorithms. To identify the singular configurations contributing to this perturbative order we introduce the relevant projector operators Nested compositions of these limits with the one in Eq. (5.4) also contribute to the divergent behaviour of the double real matrix element, and in particular the mixed action of NLO limits onto NNLO singular configurations gives rise to the strongly-ordered terms, collected by \(\overline{K}^{(12)}\). We start by analysing the real-virtual contribution to the NNLO computation. The (unintegrated) counterterm \({\overline{K}}^{{(\mathrm RV)}}\) must be defined in such a way that it embeds all of the phase space singularities of the real-virtual matrix-element RV. To do so, we partition the phase space into NLO sectors by means of sector functions \({\mathcal {W}}_{ij}\), (the same functions used for NLO subtractions). In each sector \({\mathcal {W}}_{ij}\) we then identify a finite quantity by subtracting from RV all its singular limits The contributing limits are understood to feature the kinematics map** discussed in Sect. 5.1. Note that Eq. (5.17) is a symbolic statement, which can be embedded in an efficient subtraction procedure only after providing an explicit expression for the barred projectors. We then introduce the real-virtual counterterm: The subtraction of the real-virtual singularities proceeds sector by sector. Once the proper counterterm has been subtracted from RV, the combination \((RV-{\overline{K}}^{{(\mathrm RV)}}) \, {\mathcal {W}}_{ij}\) is free of phase-space singularities by construction. We then have to add the counterterm back in its integrated form, i.e. we need to compute \(I^{(\mathrm{RV})}\). Before tackling the integration problem, we get rid of the sector functions as done at NLO (analogously to what we have presented in Eq. (5.13) upon replacing R with RV), obtaining As discussed at NLO, the quantities \(\overline{\mathbf{C}}_{ij} \, RV, \, \overline{\mathbf{S}}_{i} \, RV, \, \overline{\mathbf{S}}_{i} \overline{\mathbf{C}}_{ij} \, RV\) are in general constrained by a set of consistency relations forcing the barred limits reproduce the correct behaviour of RV. This requirement implies the relations given in Eq. (5.12), provided we substitute R with RV. The implementation of such relations mostly relies on the peculiar properties of the map** that are applied to the singular kernels of the real-virtual matrix. The freedom in defining the barred projectors implies that \(\overline{\mathbf{C}}_{ij} \, RV, \, \overline{\mathbf{S}}_{i} \, RV, \, \overline{\mathbf{S}}_{i} \overline{\mathbf{C}}_{ij} \, RV\) can benefit from extra terms that are not present in the off-shell singular regimes, provided the consistency relations are still satisfied. This feature can be exploited to implement further properties of \({\overline{K}}^{{(\mathrm RV)}}\), as the cancellation of its explicit poles against the one stemming from \(I^{(12)}\). Such a cancellation is not protected by the KLN theorem, and represents a specific trait of our method. The core structure of barred operators contributing to \(\overline{K}^{\mathrm{(RV)}}\) is designed to mimic the singular kernels known from the literature [116, 117]. To provide an example, we focus on the collinear contribution. The singular behaviour of the real-virtual matrix element reads [116, 117] where \(\beta _0=(11\,C_A-4\,T_R\,N_f)/3\), \(B_{\mu \nu }\) and \(V_{\mu \nu }\) are respectively the Born and the virtual spin-correlated matrix element, while \(P_{ij}^{\mu \nu }\) and \(P_{ij}^{(1) \mu \nu }\) are the spin-dependent Altarelli–Parisi (AP) kernel at tree level and one-loop accuracy. The one loop splitting function can be more easily treated by identifying its spin-averaged and a spin-dependent component as where for each \(X_{ij} \in \{ M_{ij}\,P_{ij}, \, N_{ij}, \, M_{ij}\,Q^{\mu \nu }_{ij}, \, O^{\mu \nu }_{ij} \}\) one has with \(\delta _{f_i \{q {{\bar{q}}}\}}=\delta _{f_i q}+\delta _{f_i {{\bar{q}}}}\) and \(\delta _{ \{f_i f_j\} \{q {{\bar{q}}}\} }=\delta _{f_i q} \, \delta _{f_j{{\bar{q}}}}+\delta _{f_i{{\bar{q}}}} \, \delta _{f_j q}\). The functions \(P_{ij}\) and \(Q_{ij}^{\mu \nu }\) are the spin components of the AP splitting functions at tree-level, written for instance in Eqs. (2.28-2.29) of Ref. [105]. For the gq splitting, relevant for the process \(e^+e^- \rightarrow jj\), we have where z is the collinear energy fraction of the emitted gluon. The structure in Eq. (5.20) provides the core structure of the corresponding barred limit, once the virtual and the Born spin-correlated matrices have been mapped. By choosing the (ijr) map**, the variable z appearing in Eq. (5.23) coincides with the Catani–Seymour parameter z, introduced in Eq. (5.9). Adopting this parameterisation, the terms in Eq. (5.20) that are proportional to virtual matrix-elements, as well as those coming from UV renormalisation (proportional to \(\beta _0\)), can be integrated with standard techniques. With regards to the \(P^{(1)\mu \nu }_{ij}\) contribution, the spin-dependent kernels \(Q_{ij}^{\mu \nu }\) and \(O_{ij}^{\mu \nu }\) vanish when integrated over the azimuth, while \(N_{ij}\) can be trivially integrated. The most involved integrals are due to the \(P_{ij}M_{ij}\) term, whose main structure is of the type where \(n, m \in \{-1,0,1\}\). For these values, the integral over z is well defined and gives that can be expanded in \(\epsilon \) powers, using for example the HypExp code [118, 119]. The integration over the remaining radiation phase space variables \(\phi \) and y is straightforward. All the other splitting configurations (\(g \rightarrow gg\), \(g \rightarrow q{{\bar{q}}}\)) feature the same degree of complexity as \(q \rightarrow gq\). Similar conclusions also hold for the core structure of the soft-collinear barred limit, that gives at most polynomials in the z and y variables. Moving to the soft contribution, we can consider as the core structure the expression in Eq.(3.30) of Ref. [117]. The integration of its contributions can be performed with standard machinery, except for the tripole-colour-correlated component which is slightly more involved. It is worth noting that neither the \(I^{(2)}\) counterterm, nor the double-virtual matrix element manifest such a peculiar colour structure. Thus, the cancellation of singularities proportional to tripole-colour-correlated matrix elements is a crucial step of the method, whose treatment is detailed in Ref. [115]. The methods developed to treat the NLO phase space singularities of the real-virtual matrix element can be generalised at NNLO to subtract the divergences of the double-real correction. At this perturbative order, sector functions and phase space parametrisation combine in a more involved way to enable the analytic integrations of the relevant singular kernels. The partition of phase space requires new sectors functions \({\mathcal {W}}_{abcd}\), that include as many different indices as the maximum number of partons that can become unresolved simultaneously. The indices run over the \(n+2\) legs of the double-real matrix element. In order to account for all NNLO singular configurations, to select a minimal set of them in each sector, and to avoid double counting, the four indices are chosen such that \(a\ne b\) and \(c\ne d\). Furthermore, c and d are allowed to equal b but not a (\(c,d\ne a\)). Three topologies arise from the possible choices of indices, As we have done for RV, we require such sector functions to be a unitary partition of phase space and sum to one when considering sectors that share the same singular configurations. The former condition is satisfied by defining The latter requirement can be trivially verified once an explicit form for \({\mathcal {W}}_{abcd}\) has been implemented. One possibility is choosing \(\sigma _{abcd}\) to be a generalisation of the NLO \(\sigma _{ab}\) in Eq. (5.5) as We stress that the choice of sector functions is not unique. For example, given the structure of Eq. (5.28), the exponent \(\alpha \) can be conveniently modulated. Also different structures could be envisaged, e.g. the energy fraction and the angular separation relative to the first pair of indices (a, b in Eq. (5.28)) could feature two different exponents. The sectors in Eq. (5.27) together with the definition in Eq. (5.28) can be easily checked to fulfil the relation which provides an example of the sum rules mentioned above. Analogous relations hold for the remaining projector operators listed in Eq. (5.16) and for their nested combinations. The collection of all singular configurations contributing to a given sector gives, As already discussed, this set of limits is a direct consequence of our choice of sector functions. Modifications in the definition in Eq. (5.28) lead to adjustments in the lists of contributing primary limits reported in Eqs. (5.30)–(5.32). Sector by sector, we subtract from the double-real matrix element all its singular configurations (avoiding double counting), obtaining a finite object that provides our candidate counterterm. In the \({\mathcal {W}}_{ijkl}\) sector the subtracted double-real matrix element reads Similar relations hold for the other topologies. In Eq. (5.33) we recognise the contribution of sector \({\mathcal {W}}_{ijkl}\) to the integrand function in the last line of Eq. (5.15). It is then necessary to disentangle the single-, the double- and the mixed-unresolved counterterms by reorganising the listed limits in Eqs. (5.30)–(5.32) according to their kinematics. In particular, the first two parentheses in Eq. (5.33) contain single-unresolved operators that we label collectively \(\overline{\mathbf{L}}_{ij}^{(1)}\), as already done for RV, while the remaining combinations feature pure double-unresolved limits, that are collected by \(\overline{\mathbf{L}}_{ijkl}^{(2)}\). The relation in Eq. (5.33) can be then rewritten in the more compact form as with \(\overline{\mathbf{L}}_{ij}^{(1)} \, \overline{\mathbf{L}}_{ijkl}^{(2)}\) giving rise to the strongly-ordered singularities. The explicit expression for the \(\overline{ \mathbf{L}}\) operators in the ijkl sector reads A fundamental requirement for the above described structure is that it must account for all and only the actual phase space singularities of RR. This statement implies that whatever \(\overline{ \mathbf{L}}\) is, it must match the RR behaviour under the singular limits listed in Eq. (5.16). For \(\overline{\mathbf{L}}_{ij}^{(1)}\) this means to impose the equivalent set of relations introduced in Eq. (5.12) upon considering RR instead of RV. For \(\overline{\mathbf{L}}_{ijkl}^{(2)}\) and \(\overline{\mathbf{L}}_{ijkl}^{(12)}\) the number of consistency relations is much larger: as a general statement, a counterterm contribution obtained nesting n primary projectors has to fulfil n consistency relations. For this reason, finding counterterm definitions that simultaneously satisfy all the constraints is highly non-trivial. Assuming the existence of consistent definitions for all the barred operators in Eq. (5.34) the counterterms can then be defined as Each term has to be integrated over its proper phase space, as defined in Eq. (5.14), and features different characteristics, therefore we will discuss separately their properties and the corresponding integration procedure. The single unresolved \({{\overline{K}}}^{(1)}\) and the mixed double unresolved \({{\overline{K}}}^{(12)}\) have been already analysed in Ref. [105], therefore we only summarise the main aspects of their treatment. Once \({{\overline{K}}}^{(1)}\) and \({{\overline{K}}}^{(12)}\) have locally subtracted the singularities of RR sector by sector in the double-unresolved phase space, both the counterterms have to be integrated over a single radiative phase space, as prescribed by Eq. (5.14). Their integrated counterparts are then combined with the real virtual matrix element and with \(\overline{K}^{{(\mathrm RV)}}\) (see the second line of Eq. (5.15)), which are split into NLO sectors. For this purpose, the sector functions appearing in Eq. (5.36), as defined in Eqs. (5.27)–(5.28), must factorise into NLO functions under single-unresolved limits. The generic expression of this property reads where Considering as an example the pure-soft content of \(\overline{K}^{(1)}\) and \({{\overline{K}}}^{(12)}\), the factorisation of NNLO sector functions into NLO sectors guarantees the following equalities where we have exploited the sector function sum properties introduced at NLO, which hold also for \({\mathcal {W}}_{ij}^{(\alpha )}\). The kinematic map** of sector functions, namely \(\overline{{\mathcal {W}}}_{kl}\), enables to factorise the structure of NLO sectors out of the radiative phase space, and integrate analytically only the singular kernels. By adopting the Catani–Seymour map**s already discussed in Eq. (5.9), and parametrising \(d\Phi _{{\mathrm{rad}}, 1}^{(abc)}=d\Phi _{\mathrm{rad}}^{(abc)}\) with the (iab) map** we can easily compute where the proportionality symbol understands constants and symmetry factors that are the same in the two lines above. The soft function \(J^{s}\big ({{\bar{s}}}_{ab}^{(iab)}\big )\) is defined as the integral over the single phase space of the Lorenz invariants occurring in the soft kernel and it can be easily computed to all orders in \(\epsilon \), From the explicit expressions of \(I^{(1), \, {\mathrm{s}}}\) and \(I^{(12), \, {\mathrm{s}}}\) on the r.h.s. of Eq. (5.42) it is evident that the two counterterm share the same phase space singularities, which then cancel in the combination \(I^{(1), \, {\mathrm{s}}}-I^{(12), \, {\mathrm{s}}}\). Analogous considerations apply to the hard-collinear component, so that \(I^{(1)}-I^{(12)}\) is free of implicit poles. Applying a similar procedure also for the collinear component, the complete single-unresolved integrated counterterm reads Notice that after the integration, the barred variables can be relabelled to the same real kinematics \(\{{{\bar{k}}}\}\), and that the sum over h, q runs over the NLO partons, and barred momenta and invariants refer to the NLO kinematics. In order to integrate the NNLO kernels in the double-unresolved phase space we need to implement NNLO map** that can simplify the integration procedure. To this purpose, we introduce double Catani–Seymour map**s [5.7), and able to reduce the initial set of \(n+2\) momenta to n on-shell momenta without breaking total momentum conservation. The double map** is defined as We then introduce the Catani–Seymour parameters to factorise the \(({n+2})\)-body phase space as The double unresolved phase space can be written as an integral over the variables \((\phi , y, z, x', y', z')\) as where \(y'\) and \(z'\) are the variables relative to the secondary-radiation phase space, and \(x'\) parametrises the azimuth between subsequent emissions. As an example, we consider the contribution to the double soft barred limit \(\overline{\mathbf{S}}_{ij} \, RR\) stemming from the \(q {{\bar{q}}} \) configuration. The core structure of such a limit embeds the NNLO soft current in Eq.(96) of Ref. [113], which reads with i, j referring to the unresolved partons, and c, d to the emitting particles. To integrate this current we choose to parametrise the double-unresolved phase space according to the (ijcd) map**. In this parametrisation, the denominators appearing in Eq. (5.49) read while in the numerators only polynomials in the Catani–Seymour parameters appear. Note that also the dependence on the azimuth is completely factorised and occurs only in the numerator through \(s_{jd}\) and \(s_{id}\), since and \(s_{id}=(y'+z-y' z) (1-y)\, {{\bar{s}}}_{cd}^{(ijcd)} -s_{jd} \). The phase space integral assumes the following form where the numerator reads in full generality Now we sketch the integration procedure by considering one variable at a time: the integration over \(\phi \) is trivial, the one over y returns a simple Beta function \(B(m_1-1-2\epsilon , m_2+1-2\epsilon )\), with \(m_1,m_2 \in {\mathbb {Z}}\), and the azimuth contribution is \(B(1/2-\epsilon ,1/2-\epsilon ) \, \delta _{k \, 0}\). The trivial dependence on \(z'\) in the numerator enables a straightforward integration that returns \(B(n_1+1-\epsilon , n_2+1-\epsilon )\), with \(n_1,n_2\) being integers or semi-integers. The z variable features instead a less-trivial structure, which however can be integrated according to The remaining integration over \(y'\) is tackled by applying recursively the hypergeometric function properties until we obtain \({}_2 F_1 (-\epsilon ,-2\epsilon ,1-2\epsilon , 1-y')\). The series expansion of such class of hypergeometric functions is known at all orders in \(\epsilon \) in terms of Spence functions. At this point the poles in \(\epsilon \) can be extracted using the plus prescription and the remaining integration over y can be carried out with standard techniques. We stress that the \(q{{\bar{q}}}\) case is particularly simple, since no denominators containing the azimuth appear in the current structure after the parametrisation. For the gg case (and for the collinear contributions) the integration is much more involved. However, in our approach it can be carried out with standard techniques [115]. Similar integrals have been computed in the context of other NNLO schemes, for instance by means of integration by parts identities and differential equations machinery [120, 121]. Given a general strategy to define double-unresolved and real-virtual counterterms (see Sect. 5.4) we have to identify the IR singularities of the double-virtual matrix element, that we assume to be already UV renormalised. From the studies carried on in the context of IR factorisation [122,123,124], the infrared poles of gauge theory scattering amplitudes are known to organise according to where \({\mathcal {A}}\) is a generic n-parton amplitude, \({\mathcal {H}}\) is finite for \(\epsilon \rightarrow 0\) and \({\mathbf {Z}}\) is a color operator with a universal form. In Eq. (5.55) all the color indices are understood, to simplify the notation. The operator \({\mathbf {Z}}\) obeys a renormalisation group equation that can be solved in terms of the anomalous dimension \({\varvec{\Gamma }}\) as described by the following expression The operator \({\varvec{\Gamma }}\), in turn, manifests a universal behaviour regulated by the dipole formula [122,123,124,125] where the \(\sigma _{ij}\) is a phase factors that equals 1 if i, j are both in the initial or in the final state, and vanishes otherwise. The function \({\widehat{\gamma }}_k\) is a universal quantity related to the cusp anomalous dimension, and the jet anomalous dimensions \(\gamma _i\) are related to the anomalous dimensions of quark and gluon fields. Finally, \({\mathbf {T}}_a\) are color operators [73, 126]. By expanding \({\varvec{\Gamma }}\) at two-loop order, and then deriving the expression of \({\mathbf {Z}}\), it is straightforward to obtain the singular part of the squared amplitude up to \(\alpha ^2_s\) by means of Eq. (5.55). As a result, the double virtual matrix element features infrared poles that obey the following general structure [123] [127]: with \(C_{f_i}\) being the Casimir eigenvalue for the leg i and \(b_0=(11C_A-4T_RN_f)/3\) being the one loop \(\beta \)-function coefficient. The quantity \(H_{ij}\) is a process-dependent finite contribution that derives from the virtual matrix element, while \(B_{ab}\) and \(B_{abcd}\) are respectively the single and double colour-correlated Born matrix elements: From Eq. (5.58) it is evident that such a structure can be implemented in the subtraction procedure only given the knowledge of the necessary anomalous dimensions, which however can be found in the literature. Moreover, also the process-dependent quantity \(H_{ij}\) has to be considered as an external input of the scheme. The validation of the subtraction scheme has been performed for the two jet production in \(e^+e^-\) annihilation, considering for the moment only the \(T_R C_F\) contribution [105]. The virtual \(e^+e^- \rightarrow q_1 {{\bar{q}}}_2 \), real-virtual \(e^+e^- \rightarrow q_1 {{\bar{q}}}_2 g_{[34]}\), and double real \(e^+e^- \rightarrow q_1 {{\bar{q}}}_2 q'_3 {{\bar{q}}}'_4\) contributions to the inclusive cross-section are known analytically from Refs. [36, 128, 129] We can now compute the local counterterms and their integrated counterparts, showing the cancellation of the singularities presented above. The double real matrix element presents single phase space singularities corresponding to the single collinear limit only. The double-unresolved singularities arise from the configurations where both the emitted quarks are soft, or they are collinear to one of the hard Born-level fermion. The relevant limits in the unbarred kinematics are \(\overline{\mathbf{C}}_{ij} RR, \, \overline{\mathbf{S}}_{ij} RR, \, \overline{\mathbf{C}}_{ijk} RR , \, \overline{\mathbf{S}}_{ij} \overline{\mathbf{C}}_{ijk} RR\), on top of the NLO limits, relevant for the real-virtual counterterm. Here \(\{i,j\}=\{3,4\}\), and \(\{ijk\} = \{134, 234\}\), and \(r=\{1,2,3,4\}, r\ne i,j,k\). The resulting complete set of counterterms is then given by5.3 Real-virtual contribution
5.3.1 Momentum map**s and integration procedure for the real-virtual counterterm
5.4 Double-real contribution
5.4.1 Double-real: single- and mixed double- unresolved counterterms
5.4.2 Double-real: pure double-unresolved counterterm

5.5 Double virtual contribution
5.6 Application: \(T_R C_F\) contribution to \(e^+e^- \rightarrow j j\) at NNLO
Let us stress that the spin-dependent component of the double-collinear Altarelli–Parisi splitting function vanishes upon integration. Finally, the composite limit \({\mathbf{S}}_{ij} {\mathbf{C}}_{ijk} RR\) coincides with the double soft contribution \({\mathbf{S}}_{ij} RR\), given the fact that k and r have to be different from i, j, and in this specific process they can only coincide with 1 and 2. Summing all the contributions, as prescribed by Eq. (5.62), we easily obtain the double-unresolved integrated counterterm
The next contribution is due to the single unresolved configurations, which are entirely reproduced by the collinear limit \(\overline{\mathbf{C}}_{34}\). The expression of \(T_R C_F\) contribution to \(I^{(1)}\) can be directly read from Eq. (5.44) returning
here \(\{h,q\}= \{1, 2, [34]\}\). The mixed-double unresolved counterterm is given by
Finally, the real-virtual counterterm reads
and its integrated counterpart that results
We can check verify that all the expected cancellations take place. The subtracted double real matrix element is finite by construction, the difference \({\overline{K}}^{(RV)} + I^{(12)}\) has to be finite sector-by-sector, and indeed we have
which is clearly free of explicit poles. The real-virtual matrix element has to be finite for \(\epsilon \rightarrow 0\) when combined with \(I^{(1)}\), thanks to the KLN theorem. Indeed we have
The comparison between Eqs. (5.70) and (5.71) makes evident that the phase space singularities of the two objects cancel in the combination \(RV \, \overline{{\mathcal {W}}}_{hq} + I_{hq}-({\overline{K}}_{hq}^{{(\mathrm RV)}} + I^{(12)}_{hq})\). Finally, the double-virtual poles are cancelled by the sum \(I^{(2)}+I^{{(\mathrm RV)}}\), as one can easily deduce by looking at the expressions in Eqs. (5.65)–(5.69).
5.7 Discussion
In this manuscript, we have reviewed the main aspects of the local analytic sector subtraction scheme.
We have recently computed all the integrated counterterms that are necessary to have a fully general subtraction method (for massless, final-state QCD).
It is evident from the discussion above that the scheme benefits from an optimised partition and parametrisation of the phase space, which allows for the analytic integration of the singular kernels arising both from the double real and the real-virtual matrix element.
Currently, the subtraction scheme is fully validated at NLO for a generic process with final state partons. With regards to NNLO, we have computed the \(C_F T_R\) contribution to the inclusive \(e^+e^- \rightarrow jj\) cross-section. Much work is in progress to further check the scheme in less trivial processes, towards its the generalisation to any final state QCD process.
Beyond this, the next steps will concern the extension of the scheme to the treatment of initial state radiation. Very recently, a preliminary successful study has been carried out at NLO. Extending it to NNLO is expected to be time-consuming, but not to present conceptual novelties. However we foresee to be able to propose a similar structure as the one for final-state radiation. The generalisation to the massive case is expected to be more involved, especially concerning the integration procedure.