Efficient sixth-order finite difference method for the two-dimensional nonlinear wave equation with variable coefficient

Abstract

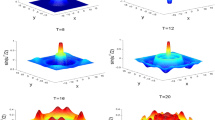
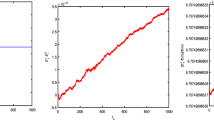
In this study, firstly, a sixth-order finite difference operator and correction technique of truncation error remainder are employed to construct a nonlinear high-order finite difference scheme and a linearized high-order finite difference scheme for solving the numerical solution of two-dimensional nonlinear wave equations with variable coefficients. Both new schemes have sixth-order accuracy in space and fourth-order accuracy in time. Then, the Richardson extrapolation technique is applied to obtain a numerical solution of sixth-order accuracy in both time and space. Meanwhile, the stability of the corresponding difference scheme for the linear wave equation is proved by Fourier analysis. In addition, two proposed sixth-order schemes are extended to solve the coupled sine-Gordon equations. Finally, some numerical experiments are presented to confirm the effectiveness and accuracy of the proposed schemes.

Appendix A

Taking the Taylor series expansion yields

$$\begin{aligned} {u_{i \pm 1}}&= {u_i} \pm h{\left( {\frac{{\partial u}}{{\partial x}}} \right) _i} + \frac{{{h^2}}}{2}{\left( {\frac{{{\partial ^2}u}}{{\partial {x^2}}}} \right) _i} \pm \frac{{{h^3}}}{6} {\left( {\frac{{{\partial ^3}u}}{{\partial {x^3}}}} \right) _i} \nonumber \\&\quad + \frac{{{h^4}}}{{24}}{\left( {\frac{{{\partial ^4}u}}{{\partial {x^4}}}} \right) _i} \pm \frac{{{h^5}}}{{120}}{\left( {\frac{{{\partial ^5}u}}{{\partial {x^5}}}} \right) _i} \nonumber \\&\quad + \frac{{{h^6}}}{{720}}{\left( {\frac{{{\partial ^6}u}}{{\partial {x^6}}}} \right) _i} \pm \frac{{{h^7}}}{{5040}} {\left( {\frac{{{\partial ^7}u}}{{\partial {x^7}}}} \right) _i} + O\left( {{h^8}} \right) \end{aligned}$$

(A.1)

$$\begin{aligned} {u_{i \pm 2}} =&{u_i} \pm 2h{\left( {\frac{{\partial u}}{{\partial x}}} \right) _i} + 2{h^2} {\left( {\frac{{{\partial ^2}u}}{{\partial {x^2}}}} \right) _i} \pm \frac{{8{h^3}}}{6} {\left( {\frac{{{\partial ^3}u}}{{\partial {x^3}}}} \right) _i} \nonumber \\&\quad + \frac{{16{h^4}}}{{24}} {\left( {\frac{{{\partial ^4}u}}{{\partial {x^4}}}} \right) _i} \pm \frac{{32{h^5}}}{{120}} {\left( {\frac{{{\partial ^5}u}}{{\partial {x^5}}}} \right) _i} \nonumber \\&\quad + \frac{{64{h^6}}}{{720}}{\left( {\frac{{{\partial ^6}u}}{{\partial {x^6}}}} \right) _i} \pm \frac{{128{h^7}}}{{5040}}{\left( {\frac{{{\partial ^7}u}}{{\partial {x^7}}}} \right) _i} + O\left( {{h^8}} \right) \end{aligned}$$

(A.2)

Adding ${u_{i + 1}}$ and ${u_{i - 1}}$ in (A.1) and ${u_{i + 2}}$ and ${u_{i - 2}}$ in (A.2) gives

$$\begin{aligned} {u_{i\mathrm{{ + }}1}}\mathrm{{ + }}{u_{i - 1}}&= 2{u_i} + {h^2}{\left( {\frac{{{\partial ^2}u}}{{\partial {x^2}}}} \right) _i} + \frac{{{h^4}}}{{12}}{\left( {\frac{{{\partial ^4}u}}{{\partial {x^4}}}} \right) _i}\nonumber \\&\quad + \frac{{{h^6}}}{{360}}{\left( {\frac{{{\partial ^6}u}}{{\partial {x^6}}}} \right) _i} + O\left( {{h^8}} \right) \end{aligned}$$

(A.3)

$$\begin{aligned} {u_{i\mathrm{{ + 2}}}}\mathrm{{ + }}{u_{i - 2}}&= 2{u_i} + 4{h^2}{\left( {\frac{{{\partial ^2}u}}{{\partial {x^2}}}} \right) _i} + \frac{{16{h^4}}}{{12}}{\left( {\frac{{{\partial ^4}u}}{{\partial {x^4}}}} \right) _i} \nonumber \\&\quad + \frac{{64{h^6}}}{{360}}{\left( {\frac{{{\partial ^6}u}}{{\partial {x^6}}}} \right) _i} + O\left( {{h^8}} \right) \end{aligned}$$

(A.4)

Put (A.4)–(A.3) $\times 16$, we obtain

$$\begin{aligned} {\left( {\frac{{{\partial ^2}u}}{{\partial {x^2}}}} \right) _i}&= \frac{1}{{12{h^2}}}\left[ {16\left( {{u_{i\mathrm{{ + }}1}}\mathrm{{ + }}{u_{i - 1}}} \right) - \left( {{u_{i\mathrm{{ + 2}}}}\mathrm{{ + }}{u_{i - 2}}} \right) - 30{u_i}} \right] \nonumber \\&\quad + \frac{{{h^4}}}{{90}}{\left( {\frac{{{\partial ^6}u}}{{\partial {x^6}}}} \right) _i} + O\left( {{h^6}} \right) \end{aligned}$$

(A.5)

In the same way, we can get (A.4)–(A.3) $\times 64$

$$\begin{aligned} {\left( {\frac{{{\partial ^2}u}}{{\partial {x^2}}}} \right) _i}&= \frac{1}{{60{h^2}}}\left[ {64\left( {{u_{i\mathrm{{ + }}1}}\mathrm{{ + }}{u_{i - 1}}} \right) - \left( {{u_{i\mathrm{{ + 2}}}}\mathrm{{ + }}{u_{i - 2}}} \right) - 126{u_i}} \right] \nonumber \\&\quad - \frac{{{h^2}}}{{15}}{\left( {\frac{{{\partial ^4}u}}{{\partial {x^4}}}} \right) _i} + O\left( {{h^6}} \right) \end{aligned}$$

(A.6)

Define the following difference operator:

$$\begin{aligned} {\bar{\delta }} _x^2{u_i} =&\frac{1}{{12{h^2}}}\left[ {16\left( {{u_{i\mathrm{{ + }}1}}\mathrm{{ + }}{u_{i - 1}}} \right) - \left( {{u_{i\mathrm{{ + 2}}}}\mathrm{{ + }}{u_{i - 2}}} \right) - 30{u_i}} \right] \end{aligned}$$

(A.7)

$$\begin{aligned} {\tilde{\delta }} _x^2{u_i} =&\frac{1}{{60{h^2}}}\left[ {64\left( {{u_{i\mathrm{{ + }}1}}\mathrm{{ + }}{u_{i - 1}}} \right) - \left( {{u_{i\mathrm{{ + 2}}}}\mathrm{{ + }}{u_{i - 2}}} \right) - 126{u_i}} \right] \end{aligned}$$

(A.8)

(A.5) and (A.6) can be rewritten as:

$$\begin{aligned} {\left( {\frac{{{\partial ^2}u}}{{\partial {x^2}}}} \right) _i} =&{\bar{\delta }} _x^2{u_i} + \frac{{{h^4}}}{{90}} {\left( {\frac{{{\partial ^6}u}}{{\partial {x^6}}}} \right) _i} + O\left( {{h^6}} \right) \end{aligned}$$

(A.9)

$$\begin{aligned} {\left( {\frac{{{\partial ^2}u}}{{\partial {x^2}}}} \right) _i} =&{\tilde{\delta }} _x^2{u_i} - \frac{{{h^2}}}{{15}}{\left( {\frac{{{\partial ^4}u}}{{\partial {x^4}}}} \right) _i} + O\left( {{h^6}} \right) \end{aligned}$$

(A.10)

Substituting (A.9) into (A.10), we have

$$\begin{aligned} \left( {1 + \frac{{{h^2}}}{{15}}{\bar{\delta }} _x^2} \right) {\left( {\frac{{{\partial ^2}u}}{{\partial {x^2}}}} \right) _i} = {\tilde{\delta }} _x^2{u_i} + O\left( {{h^6}} \right) \end{aligned}$$