Abstract
We construct convex bodies that can be “captured by nets.” More precisely, for each dimension \(n \ge 2\), we construct a family of Riemannian n-spheres, each with a stable geodesic net, which is a stable 1-dimensional integral varifold. Small perturbations of a stable geodesic net must lengthen it. These stable geodesic nets are composed of multiple geodesic loops based at the same point, and also do not contain any closed geodesic. All of these Riemannian n-spheres are isometric to convex hypersurfaces of \(\mathbb {R}^{n+1}\) with positive sectional curvature.





Similar content being viewed by others
Data availability
There is no data involved in this mathematical paper that could be made available.
Notes
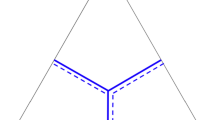
The \(\theta \) graph is the graph with two vertices that are connected by three edges.
We also highlight a counterexample by W. Ziller, of a closed homogeneous 3-manifold with positive sectional curvature and a stable closed geodesic [14, Example 1].
The proof of this assertion will be deferred the end of the paper, when we prove our main result, Theorem 1.1.
Note that this definition differs from the usual definition of the index form of a unit-speed geodesic by a factor of \(1/\ell \). However, this convention will simplify our subsequent formulas.
This is a consequence of basic Euclidean geometry.
To establish this convergence, one may begin by expressing \(\Theta \) as the convolution of two Gaussians, \(\Theta _1\) and \(\Theta _2\). The associativity of convolution will then yield \(f * \Theta = (f * \Theta _1) * \Theta _2\). The \(C^\infty \) function \(f * \Theta _1\) will bear all derivatives, while its convolution with \(\Theta _2\) will take care of convergence, as the variances of all these Gaussians tend to zero.
References
Pitts, J.T.: Regularity and singularity of one dimensional stationary integral varifolds on manifolds arising from variational methods in the large. In: Symposia Mathematica, volume 14, pages 465–472. Academic Press London-New York (1974)
Allard, W.K., Almgren, F.J.: The structure of stationary one dimensional varifolds with positive density. Invent. Math. 34(2), 83–97 (1976)
Nabutovsky, A., Rotman, R.: Shapes of geodesic nets. Geom. Topol. 11(2), 1225–1254 (2007)
Rotman, R.: Flowers on Riemannian manifolds. Math. Z. 269(1–2), 543–554 (2011)
Liokumovich, Y., Staffa, B.: Generic density of geodesic nets (2021)
Hass, J., Morgan, F.: Geodesic nets on the 2-sphere. Proc. Am. Math. Soc. 124(12), 3843–3850 (1996)
Adelstein, I., Vargas Pallete, F.: The length of the shortest closed geodesic on positively curved 2-spheres. Math. Z. pages 1–13 (2020)
Lawson Jr, H.B., Simons, J.: On stable currents and their application to global problems in real and complex geometry. Annal. Math. pages 427–450 (1973)
Shen, Y.-B., Xu, H.-Q.: On the nonexistence of stable minimal submanifolds in positively pinched Riemannian manifolds. In: Geometry And Topology Of Submanifolds X, pages 274–283. World Scientific (2000)
Howard, R.: The nonexistence of stable submanifolds, varifolds, and harmonic maps in sufficiently pinched simply connected Riemannian manifolds. Mich. Math. J. 32(3), 321–334 (1985)
Hu, Z.-J., Wei, G.-X.: On the nonexistence of stable minimal submanifolds and the lawson–simons conjecture. In: Colloquium Mathematicum, volume 96, pages 213–223. Instytut Matematyczny Polskiej Akademii Nauk (2003)
Synge, J.L.: On the connectivity of spaces of positive curvature. Q. J. Math. 1, 316–320 (1936)
Howard, R., Wei, S.W.: On the existence and nonexistence of stable submanifolds and currents in positively curved manifolds and the topology of submanifolds in Euclidean spaces. Geometry and Topology of Submanifolds and Currents. Contemp. Math. 646:127–167 (2015)
Ziller, W.: Closed geodesics on homogeneous spaces. Math. Z. 152(1), 67–88 (1976)
zeb. Is it possible to capture a sphere in a knot? MathOverflow. https://mathoverflow.net/q/8091 (version: 2009-12-08)
Petrunin, A.: Which convex bodies can be captured in a knot? MathOverflow. https://mathoverflow.net/q/360066 (version: 2020-06-24)
Milnor, J.: Morse Theory. (AM-51), Volume 51. Princeton university press, (2016)
Staffa, B.: Bumpy metrics theorem for geodesic nets (2021)
Lebedeva, N., Matveev, V., Petrunin, A., Shevchishin, V.: Smoothing 3-dimensional polyhedral spaces. ar**v preprint ar**v:1411.0307, (2014)
Jost, J.: Riemannian Geometry and Geometric Analysis, 7th edn. Springer, Germany (2017)
Golubitsky, M., Guillemin, V.: Stable map**s and their singularities, vol. 14. Springer Science & Business Media, Germany (2012)
Aparecida Soares Ruas, M.: Old and new results on density of stable map**s. In: José Luis Cisneros-Molina, Lê Dũng Tráng, and José Seade, (eds.) Handbook of Geometry and Topology of Singularities III, pages 1–80, Cham, (2022). Springer International Publishing
Mather, J.N.: Stability of \(c^\infty \) map**s: V, transversality. Adv. Math. 4(3), 301–336 (1970)
Mather, J.N.: Stability of \(c^\infty \) map**s: Ii. infinitesimal stability implies stability. Annal. Math. 89(2), 254–291 (1969)
Cheeger, J.: Finiteness theorems for riemannian manifolds. Am. J. Math. 92(1), 61–74 (1970)
Ghomi, M.: Optimal smoothing for convex polytopes. Bull. London Math. Soc. 36(4), 483–492 (2004)
Minkowski, H.: Volumen und Oberfläche. In: Ausgewählte Arbeiten zur Zahlentheorie und zur Geometrie, pages 146–192. Springer (1989)
Bonnesen, T., Fenchel, W.: Theory of convex bodies. BCS Associates (1987)
Nesterov, Y.: Lectures on convex optimization. volume 137. Springer (2018)
Acknowledgements
The author would like to thank his academic advisors Alexander Nabutovsky and Regina Rotman for suggesting this research topic, and for valuable discussions. The author would also like to thank Isabel Beach for useful discussions.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
A Linear-Algebraic Lemma
In this section we will prove Lemma 4.5, restated as follows.
Lemma 4.5. Let X be an n-dimensional vector space. Let \(x_1, \dotsc , x_n \in X\) be nonzero vectors that span an \((n-1)\)-dimensional subspace, satisfying the property that any \(n-1\) of them are linearly independent. Then there exist \((n-1)\)-dimensional subspaces of X, \(\Pi _1,\dotsc , \Pi _n\) such that \(x_i \in \Pi _i\) for all i and \(\bigcap _{i=1}^n \Pi _i = \{0\}\).
Proof
Choosing the \(\Pi _i\)’s generically should already work, but for concreteness we construct them explicitly. Let \(\Pi _n = \text {span}\{x_1,\dotsc ,x_n\}\), and choose some \(v \in X {\setminus } \Pi _n\). For \(1 \le i \le n - 1\), let \(\Pi _i = \text {span}\{x_i, x_{i+1}, \dotsc , x_{i + n - 3}, v\}\), where the indices of the \(x_i\) are taken cyclically modulo n. Thus, \(\dim \Pi _i = n - 1\). (Fig. 4(c) illustrates this choice of \(\Pi _i\)’s when \(n = 3\), the \(x_i\)’s are the vertices of an equilateral triangle in \(\mathbb {R}^2 \times \{0\}\) that is centred at the origin, and \(v = (0,0,1)\)).
Then \(\dim (\Pi _1 + \Pi _2) = \dim \text {span}\{x_1,\dotsc ,x_{n-1}, v\} = n\) because the \(n-1\) vectors \(x_1, \dotsc , x_{n-1}\) are linearly independent. And \(\text {span}\{x_2, \dotsc , x_{n-2}, v\} \subset \Pi _1 \cap \Pi _2\) but equality holds because both sides have dimension \(n-2\), by the dimension formula. Similarly, \(\dim ((\Pi _1 \cap \Pi _2) + \Pi _3) = \dim \text {span}\{x_2,\dotsc ,x_n, v\} = n\) because the \(n-1\) vectors \(x_2, \dotsc , x_n\) are linearly independent. Similarly again \(\text {span}\{x_3, \dotsc , x_{n-2}, v\} \subset (\Pi _1 \cap \Pi _2) \cap \Pi _3\) but equality holds because both sides have dimension \(n-3\), by the dimension formula. Continuing inductively, one can show that \(\bigcap _{i=1}^{n-1}\Pi _i= \text {span}\{v\}\). But then \(\bigcap _{i=1}^n\Pi _i= \{0\}\). \(\square \)
The Persistence of Stable Geodesic Bouquets After Perturbing the Metric
In this section we will prove Proposition 4.7, restated as follows.
Proposition 4.7. Let \((N,g_0)\) be a compact and flat Riemannian manifold, whose interior contains a stable geodesic bouquet \(G_0\) that is injective. Assume also that no two tangent vectors of the loops of \(G_0\) at the basepoint are parallel. Then for any Riemannian metric g on N that is sufficiently close to \(g_0\) in the \(C^\infty \) topology, (N, g) also contains a stable geodesic bouquet with the same number of loops, and in which no two tangent vectors of its loops at the basepoint are parallel.
Let us outline the proof strategy and prove a lemma about the perturbation of Morse functions.
Consider the space of immersions modulo reparametrizations \(\hat{\Omega }_{k}N\) that was defined in Sect. 2, where G has k loops. Every other Riemannian metric g on N induces a functional \(\text {length}_{g}: \hat{\Omega }_{k}N \rightarrow \mathbb {R}\) that gives the length of each immersion in (N, g). By hypothesis, \(\text {length}_{g_0}\) has a local minimum at \([G] \in \hat{\Omega }_{k}N\). Intuitively, we would like to prove that if g is sufficiently close to \(g_0\), then \(\text {length}_{g}\) would also have a local minimum close to [G].
To formalize this, we will define a smooth and compact finite-dimensional manifold B, as well as a family of embeddings \(\varphi _{g}: B \rightarrow \hat{\Omega }_{k}N\) parametrized by metrics g sufficiently close to \(g_0\). These embeddings may be considered as “finite-dimensional approximations of portions of \(\hat{\Omega }_{k}N\),” and they are modelled on similar constructions in the Morse theory of path space and geodesics [17, Section 16]. Specifically, the image of \(\varphi _{g}\) consists of immersions formed by “broken geodesics.”
We will show that \(L_{g_0} = {\text {length}_{g_0}} \circ \varphi _{g_0}\) has only one local minimum corresponding to [G], which will be non-degenerate. Next, we will prove that \(L_{g} = {\text {length}_{g}} \circ \varphi _{g}\) will also have a unique non-degenerate local minimum y which will correspond to the desired stable geodesic bouquet in (N, g). That y exists and is unique will be shown using the theory of stable map**s, which are, roughly speaking, \(C^\infty \) maps between manifolds that are “equivalent up to changes in coordinates” to all other maps that are sufficiently close in the \(C^\infty \) topology. Formal definitions are available in [21] and the survey [22], but we will only concern ourselves with the relevant implications, summarized in the following lemma.
Lemma B.1
Let B be a compact smooth manifold with a Morse function \(f : B \rightarrow \mathbb {R}\) that has a unique critical point in the interior of B that has index zero. Then every function \(\tilde{f}: B \rightarrow \mathbb {R}\) that is sufficiently close to f in the \(C^\infty \) topology is also Morse and has a unique critical point in the interior of B with index zero.
Proof
Since f is a proper Morse function, [21, Chapter III, Proposition 2.2] and [23, Theorem 4.1] imply that f is infinitesimally stable. The main implication for us will be [24, Theorem 2], which guarantees that for all \(\tilde{f}: B \rightarrow \mathbb {R}\) sufficiently close to f in the \(C^\infty \) topology, \(\tilde{f} = h_1 \circ f \circ h_2\) for some diffeomorphisms \(h_1 : \mathbb {R}\rightarrow \mathbb {R}\) and \(h_2 : B \rightarrow B\). Moreover, as \(\tilde{f}\) approaches f, \(h_1\) and \(h_2\) both approach the identity. As a result, \(\tilde{f}\) has a unique critical point in the interior of B that is a non-degenerate local minimum. \(\square \)
Now we are ready to prove Proposition 4.7.
Proof of Proposition 4.7 A result of J. Cheeger [25, Corollary 2.2] implies that for some constant \(\rho > 0\) and some open neighbourhood U of \(g_0\), in the space of Riemannian metrics on N with the \(C^\infty \) topology, the injectivity radius \(\text {inj}(N,g)\) exceeds \(\rho \) for all \(g \in U\). Let us now construct B as follows. Subdivide each edge of G into arcs of which lengths (with respect to \(g_0\)) are shorter than \(\rho /2\). This yields an embedded graph \(G_0^+\) with a great number of vertices \(x_0, \dotsc , x_m\), where \(x_0\) is the basepoint of G. Consider a constant \(\delta \in (0,\rho /10)\), and let \(B(x_i)\) be the closed ball of radius \(\delta \) in (N, g) that is centred at \(x_i\). Later on we will shrink the value of \(\delta \) even further. Define B to be the product \(B(x_0) \times B(x_1)^\perp \times \cdots \times B(x_m)^\perp \), where \(B(x_i)^\perp \) consists of the points \(y \in B(x_i)\) such that \(y - x_i\) is orthogonal to \(G_0\) at \(x_i\). (This makes sense because \((N, g_0)\) is flat and \(\left\Vert y - x_i\right\Vert < \rho \).)
For each \(g \in U\) and every pair of vertices \(x_i\) and \(x_j\) that are adjacent in \(G_0^+\), the fact that \(\text {inj}(N, g) > \rho \) implies that a unique minimizing geodesic in (N, g) connects each \(y_i \in B(x_i)\) and \(y_j \in B(x_j)\). In this manner, we may define an embedding \(\varphi _g: B \rightarrow \hat{\Omega }_{k}N\) that sends \((y_0,\dotsc ,y_m)\) to the equivalence class of the immersion \(\mathcal {B}_k \rightarrow N\) composed of those minimizing geodesics connecting \(y_i\) and \(y_j\) whenever \(x_i\) and \(x_j\) are adjacent. Observe that \(\varphi _{g_0}(x_0,\dotsc ,x_m) = [G_0]\). The stability of \(G_0\) implies that \(x = (x_0,\dotsc ,x_m)\) is a non-degenerate critical point of \(L_{g_0}\), because each \(x_i\) for \(i \ne 0\) is restricted to \(B(x_i)^\perp \) and cannot be displaced a nonzero distance along a vector field that is tangent to \(G_0\).
The Morse lemma guarantees that x is an isolated critical point, which means that we may decrease \(\delta \) and thereby shrink B until x remains as the only critical point of \(L_{g_0}\). Thus, \(L_{g_0}\) is a Morse function. We can choose U to be small enough such that for all \(g \in U\), \(L_g\) is \(C^\infty \)-close enough to \(L_{g_0}\) for us to apply Lemma B.1, which would imply that \(L_g\) is also Morse and has a unique critical point y in the interior of B with index 0.
For any \(g \in U\), let \(G \in \varphi _g(y)\) be the piecewise smooth immersion \(\mathcal {B}_k \rightarrow N\) that is formed by glueing together many geodesic arcs. Let us prove that it is a stationary geodesic bouquet, which requires us to verify that the sum of the outgoing unit tangent vectors at the basepoint sum to zero, and that adjacent arcs meeting away from the basepoint must form an angle of \(\pi \). The former criterion must be satisfied, otherwise we could have reduced the value of \(L_g(y)\) by perturbing \(y_0\) in \(B(x_0)\). To verify the latter criterion, observe that we may shrink U to guarantee that if adjacent arcs meet at a vertex \(y_i \in B(x_i)^\perp \), for \(i \ne 0\), then the two arcs must lie on different sides of the “disk” \(B(x_i)^\perp \). The two arcs must meet at angle \(\pi \) at \(y_i\); otherwise, we could have perturbed \(y_i\) in some direction along \(B(x_i)^\perp \) to reduce \(L_g(y)\). Therefore, G is a stationary geodesic bouquet, whose geodesic loops intersect the discs \(B(x_i)^\perp \) transversally at the points \(y_i\).
We may adapt [17, Theorem 16.2] to prove that the Hessian of \(L_g\) at y, denoted by \(\text {Hess}_y L_g\), has the same index as \(\text {Hess}_{[G]} {\text {length}_g}\), which must then be zero. Therefore, G is stable. \(\square \)
Smoothing the Double of a Convex Polytope
In this section we will prove Proposition 4.6, restated as follows.
Proposition 4.6. Let \(\textbf{X}\) be a convex n-polytope such that \(\mathcal {D}_{\mathrm {}}\textbf{X}\) contains a stable geodesic bouquet G. Then for any neighbourhood N of G in \(\mathcal {D}_{\textrm{sm}}\textbf{X}\) that is also a compact submanifold, there exists a sequence of embeddings \(\{\varphi _i: N \rightarrow M_i\}_{i = 1}^\infty \) into smooth convex hypersurfaces \(M_i\) of \(\mathbb {R}^{n+1}\) with strictly positive curvature, such that the pullback metrics \(g_i\) along \(\varphi _i\) from \(M_i\) converge to the flat metric on N in the \(C^\infty \) topology.
There are well-known methods to approximate a given convex body by a sequence of smooth convex hypersurfaces [26,27,28]. Nevertheless, we will implement our own version of this approximation to ensure that the Riemannian metrics of these hypersurfaces will converge in the \(C^\infty \) topology. These hypersurfaces will be the level sets of smooth convex functions \( R^{n+1} \rightarrow \mathbb {R}\), which will guarantee that the level sets will be smooth and convex. However, in general the sectional curvature of convex hypersurfaces may vanish at some points. To guarantee strictly positive sectional curvature, we will consider the level sets of functions that satisfy a stronger notion of convexity borrowed from convex optimization, defined as follows.
Definition C.1
(Strong convexity [29]) Given a constant \(\kappa \ge 0\) and an open convex set \(U \subset \mathbb {R}^n\), we say that a function \(f : U \rightarrow \mathbb {R}\) is \(\kappa \)-strongly convex if, for all \(x_0, x_1 \in U\) and \(\lambda \in [0,1]\) we have
We note that \(\kappa \)-strong convexity implies continuity. Moreover, if f is smooth, then \(\kappa \)-strong convexity is equivalent to \(\left\langle (\text {Hess}_p f)v,v\right\rangle _{} \ge \kappa \left\Vert v\right\Vert ^2\) for all \(p \in U\) and \(v \in T_p\mathbb {R}^n\) [29]. As a result, the following lemma shows that a \(\kappa \)-strongly convex function (for any \(\kappa > 0\)) has level sets with strictly positive sectional curvature.
Lemma C.2
Let U be an open subset of \(\mathbb {R}^n\) and \(h: U \rightarrow \mathbb {R}\) be a smooth function whose Hessians are positive definite. Let z be a regular value. Then \(h^{-1}(z)\) is a smooth hypersurface whose sectional curvatures are positive.
Proof
Let \(M = h^{-1}(z)\). Let \(p \in M\). Since z is a regular value, the gradient of h at p, denoted by \(\text {grad}_p h\), does not vanish. Let \(u, v \in T_pM\) be orthonormal. The Gauss equation implies that
where A is the second fundamental form associated with the normal vector \(\text {grad}_p h\). But \(A(u,v) = -\left\langle \frac{\partial }{\partial u} \text {grad}_p h,v\right\rangle _{} = -\left\langle (\text {Hess}_p h)u,v\right\rangle _{}\). Similar computations imply that \(\left\Vert \text {grad}_p h\right\Vert ^2K(u,v)\) is a \(2 \times 2\) minor of \(\text {Hess}_p h\). However, by hypothesis, \(\text {Hess}_p h\) is positive definite, so the minor is positive. \(\square \)
The functions whose levels sets will yield our desired convex hypersurfaces will be the convolutions of the squared-distance function \(f(x) = {\text {dist}}_{}(x,\textbf{X}\times \{0\})^2\) with Gaussians. To prove the strong convexity of the convolution, it will help to study the Hessian of f where it exists. In particular, given a convex n-polytope \(\textbf{X}\) and a point \(x \in \textbf{X}\), let \(C(x) \subset \mathbb {R}^{n+1}\) be the set of points whose closest point in \(\textbf{X}\times \{0\}\) is (x, 0). Then f will coincide with the squared-distance function from (x, 0) over C(x). As shown in the next lemma, C(x) will have the shape of an affine convex cone, that is, the translation of some convex cone.
Lemma C.3
For any convex n-polytope \(\textbf{X}\) for \(n \ge 2\), C(x) is an affine convex cone for all \(x \in \textbf{X}\). Moreover, if x is a vertex of \(\textbf{X}\) then C(x) has nonempty interior.
Proof
Note that \(C(x) = C_0(x) \times \mathbb {R}\), where \(C_0(x)\) is the set of points in \(\mathbb {R}^n\) whose closest point in \(\textbf{X}\) is x. By translating \(\textbf{X}\) through \(\mathbb {R}^n\), we may assume that x is at the origin. Hence it suffices to show that \(C_0(x)\) is a convex cone with apex at x which has nonempty interior when x is a vertex of \(\textbf{X}\).
For each \(y \in C_0(x)\), the fact that \(\textbf{X}\) is convex and that the closest point in \(\textbf{X}\) to y is x (the origin) implies that the hyperplane through the origin that is orthogonal to the vector y separates y from the interior of \(\textbf{X}\). Let \(H_y\) be the closed half-space that is bounded by this hyperplane and that contains \(\textbf{X}\). Then clearly \(H_{\lambda y} = H_y\) for any \(\lambda \ge 0\). In addition, for any \(z \in C_0(x)\) and \(\lambda \in [0,1]\), \(H_{\lambda y + (1-\lambda )z} \supset H_y \cap H_z \supset \textbf{X}\). Therefore, the closest point to \(\lambda y + (1-\lambda )z\) in \(\textbf{X}\) is also the origin, and \(\lambda y + (1-\lambda )z \in C_0(x)\). That is, \(C_0(x)\) is a convex cone.
If x is a vertex of \(\textbf{X}\), then there are at least n supporting hyperplanes of \(\textbf{X}\) meeting at x, such that the outward-pointing normal vectors are linearly independent. Hence the parallelepiped spanned by those vectors has positive volume: its volume is equal to the absolute value of the nonzero determinant of the matrix whose columns are those vectors. Therefore, \(C_0(x)\), which contains this parallelepiped, has nonempty interior. \(\square \)
Let us proceed to prove the strong convexity of certain convolutions \(f * \Theta \), where f is strongly convex over some affine convex cone, as will be the case in our situation. Let \(B_r(z) \subset \mathbb {R}^n\) denote the open ball of radius r and centred at z. For an affine convex cone \(C \subset \mathbb {R}^n\) with apex v, define its projective inradius as \(\sup _{B_r(z) \subset C}r/\left\Vert z - v\right\Vert \), where the supremum is taken over open balls of positive radius.
Lemma C.4
Let \(f: \mathbb {R}^n \rightarrow \mathbb {R}\) be a convex function that is \(\kappa \)-strongly convex when restricted to some affine convex cone C with nonempty interior, for some \(\kappa > 0\). Let \(\Theta : \mathbb {R}^n \rightarrow \mathbb {R}\) be a smooth and radially symmetric Gaussian probability density function such that \(\Theta (y) = \theta (\left\Vert y\right\Vert )\) is a function of \(\left\Vert y\right\Vert \). Let v be the apex of C and let \(\rho \) be its projective inradius. Then for any \(r > 0\), the convolution \(f * \Theta \) is \(\hat{\kappa }\)-strongly convex when restricted to \(B_r(0)\), for \(\hat{\kappa }= \kappa \omega _n \theta (\frac{1 + r}{\rho }+ \left\Vert v\right\Vert )\), where \(\omega _n\) is the volume of a unit ball in \(\mathbb {R}^n\).
Proof
Choose any \(x_0, x_1 \in B_r(0)\) and \(\lambda \in [0,1]\). Choose some \(z \in C\) such that \(B_{1 + r}(z) \subset C\) and \((1 + r)/\left\Vert z - v\right\Vert = \rho \). Note that for all \(y \in B_1(z)\), we have \(x_i + y \in B_{1 + r}(z)\) for \(i = 0,1\). Thus, the convolution \(\hat{f} = f * \Theta \) satisfies
so it remains to estimate \(\kappa \int _{B_1(z)} \Theta (-y) \,dy\). However, since \(\Theta (-y) = \theta (\left\Vert y\right\Vert )\) is a decreasing function of \(\left\Vert y\right\Vert \), and the supremum of the norm of points in \(B_1(z)\) is \(\left\Vert z\right\Vert + 1\),
where the last inequality holds because \(\left\Vert z\right\Vert \le \left\Vert z - v\right\Vert + \left\Vert v\right\Vert = (1 + r)/\rho + \left\Vert v\right\Vert \). \(\square \)
Now we are ready to prove Proposition 4.6.
Proof of Proposition 4.6 Define the function \(f: \mathbb {R}^{n+1} \rightarrow \mathbb {R}\) by \(f(x) = {\text {dist}}_{}(x,\overline{\textbf{X}})^2\), where \(\overline{\textbf{X}}= \textbf{X}\times \{0\}\). We will smooth \(\mathcal {D}_{\mathrm {}}\textbf{X}\) by considering the level sets of the convolution \(f * \Theta \), where \(\Theta \) is a radially symmetric Gaussian probability density function. Let v be a vertex of \(\overline{\textbf{X}}\); Lemma C.3 guarantees that C(v) is an affine convex cone with nonempty interior; thus, it has a nonzero projective inradius. Over the interior of C(v), f coincides with the square of the distance to (v, 0), so its Hessian is twice of the identity matrix. As a result, f is 2-strongly convex over the interior of C(v). Let \(\hat{f}\) denote the restriction of \(f * \Theta \) to some fixed large ball containing \(\overline{\textbf{X}}\). Then Lemma C.4 guarantees that \(\hat{f}\) is \(\kappa \)-strongly convex for some \(\kappa > 0\). By Lemma C.2, the level sets of \(\hat{f}\) have strictly positive sectional curvature.
Choose the variance of \(\Theta \) to be sufficiently small and choose a regular value \(r^+\) in the image of \(\hat{f}\) such that \(M^+ = \hat{f}^{-1}(r^+)\) lies in a tubular neighbourhood of \(\overline{\textbf{X}}\). Let \(h: M^+ \rightarrow \overline{\textbf{X}}\) denote the projection of the tubular neighbourhood, but restricted to \(M^+\). Let us find a smooth map \(\varphi : N \rightarrow M^+\) that “approximately lifts” the map \(N \hookrightarrow \mathcal {D}_{\mathrm {}}\textbf{X}\xrightarrow {\pi } \textbf{X}\xrightarrow {\text {id}\times \{0\}} \overline{\textbf{X}}\) over h. That is, the following diagram “nearly commutes”:

We will then pull back metrics on \(M^+\) over \(\varphi \) to get the desired metrics on N.
In some sense, we will break N up into simpler pieces and define \(\varphi \) over each piece. For each sufficiently small \(\delta > 0\) and convex polytope \(\textbf{Y}\), let \(\textbf{Y}(\delta ) = \{y \in \textbf{Y}~|~ {\text {dist}}_{}(y,\partial \textbf{Y}) \ge \delta \}\). For each face F of \(\textbf{X}\), let \(\textbf{P}_F^\delta \subset \textbf{X}\) denote a prism based at \(F(\delta )\) with height \(\delta \). (That is, \(\textbf{P}_F^\delta \) is isometric to \(F(\delta ) \times [0,\delta ]\).) Given that N is disjoint from the \((n-2)\)-skeleton of \(\mathcal {D}_{\mathrm {}}\textbf{X}\), for sufficiently small \(\delta \) we know that N is contained inside the image of \((\textbf{X}(\delta ) \cup \bigcup _F \textbf{P}_F^\delta ) \times \{0,1\}\) in \(\mathcal {D}_{\mathrm {}}\textbf{X}\), which we denote by \(N'\).
If the radius of the tubular neighbourhood and the variance of \(\Theta \) are much smaller than \(\delta \), then \(h^{-1}(\pi (N') \times \{0\})\) is almost isometric to \(N'\). This assertion can be verified separately over each \(\textbf{X}(\delta ) \times \{i\}\) and each \(\textbf{P}_F^\delta \times \{i\}\). Thus, we can define \(\varphi \) over \(N'\) by map** it to \(h^{-1}(\pi (N') \times \{0\})\), and then restrict to N.
Our conclusion, that we can choose a sequence of such embeddings \(\varphi \) whose pullback metrics on N converge to the flat metric in the \(C^\infty \) topology, follows from the property that as the variance of \(\Theta \) tends to 0, the functions \(f * \Theta \) converge in the \(C^\infty \) topologyFootnote 6 after being restricted to some fixed compact neighbourhood of \(\overline{\textbf{X}}\). \(\square \)
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Cheng, H.Y. Stable Geodesic Nets in Convex Hypersurfaces. J Geom Anal 34, 56 (2024). https://doi.org/10.1007/s12220-023-01489-2
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s12220-023-01489-2