Analytic approach to the non-pre-emptive Markovian priority queue

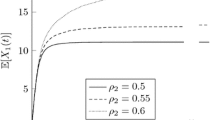
Abstract

A new approach is developed for the joint queue-length distribution of the two-level non-pre-emptive M/M/c (i.e. Markovian) priority queue that allows explicit and exact results to be obtained. Marginal distributions are derived for the general multi-level problem. The results are based on a representation of the joint queue-length probability mass function as a single-variable complex contour integral, which reduces to a real integral on a finite interval arising from a cut on the real axis. Both numerical quadrature rules and exact finite sums, involving Legendre polynomials and their generalization, are presented for the joint and marginal distributions. A high level of accuracy is demonstrated across the entire ergodic region. Relationships are established with the waiting-time distributions. Asymptotic behaviour in the large queue-length regime is extracted.

Appendices

Appendix A Joint asymptotics

To determine the large-$\ell$ behaviour of the joint integral (89), we use the symmetry properties and periodicity of the integrand to write it as an integral over the interval $\theta \in [0,2\pi )$. We then promote the integration variable $\theta$ to the complex plane and deform the contour from $\theta = 0$ to $\theta = 2\pi$ into three straight legs: (i) from 0 to $0 + i\infty$, (ii) from $0 + i\infty$ to $2\pi + i\infty$, and (iii) from $2\pi + i\infty$ to $2\pi$, making small excursions on the vertical legs around the points $\theta = \, \textrm{acos}(\alpha ), \, \textrm{acos}(\beta )$. The contribution from the horizontal contour vanishes, while the vertical contours cancel apart from the excursions around the singular points. As a result, we obtain the decomposition $J_{\text {scl}}(\ell ,m) = J_{\text {scl}}^\alpha (\ell ,m) + J_{\text {scl}}^\beta (\ell ,m)$ where, on writing $\theta = it$, we have

$$\begin{aligned} J_{\text {scl}}^{\alpha ,\beta }(\ell ,m) = (\beta -\alpha )^{m+1}\oint _{C_{\alpha ,\beta }} \frac{dt}{2\pi i}\, \frac{\sinh (t)e^{-\ell t}}{(\cosh (t)-\alpha )^{m+1}(\cosh (t) - \beta )} , \end{aligned}$$

(A1)

where $C_\alpha$ is an infinitesimal anti-clockwise circle about the point $t = {{\,\textrm{acosh}\,}}(\alpha )$, and likewise for $C_\beta$ about the point $t = {{\,\textrm{acosh}\,}}(\beta )$.

It is clear that $J_{\text {scl}}^\beta (\ell ,m) = d_{\ell +1}(\beta )$. To evaluate $J_{\text {scl}}^\alpha (\ell ,m)$, we set $\alpha \equiv \cosh (s)$ so that

$$\begin{aligned} \begin{aligned} J_{\text {scl}}^\alpha (\ell {-}1,m)&= \left( \frac{\beta -\alpha }{2}\right) ^{m+1}\!\!e^{-\ell s}\oint _{C_\alpha } \frac{dt}{2\pi i}\, \frac{e^{-\ell (t-s)}}{\sinh ^{m+1}((t-s)/2)} \\&\quad {}\times \frac{\sinh (t)}{\sinh ^{m+1}((t+s)/2)[\cosh (t)-\beta ]} \\&= \left( \frac{\beta -\alpha }{2}\right) ^{m+1}\frac{e^{-\ell s}}{\ell }\oint _{C_0} \frac{dt}{2\pi i}\,\frac{e^{-t}}{\sinh ^{m+1}(t/(2\ell ))} \\&\quad {}\times \frac{\sinh (s+t/\ell )}{\sinh ^{m+1}(s+t/(2\ell ))[\cosh (s+t/\ell )-\beta ]} \;. \end{aligned} \end{aligned}$$

(A2)

Taking the limit yields

$$\begin{aligned} J_{\text {scl}}^\alpha (\ell {-}1,m) \mathrel {\mathop {\sim }_{\ell \rightarrow \infty }} \frac{(\beta -\alpha )^{m+1}\ell ^me^{-\ell s}}{\sinh ^m(s)[\cosh (s)-\beta ]} \oint _{C_0}\frac{dt}{2\pi i}\, \frac{e^{-t}}{t^{m+1}} = -\frac{\ell ^m}{m!}\left( \frac{\alpha -\beta }{\sqrt{\alpha ^2-1}}\right) ^m d_\ell (\alpha ) ,\nonumber \\ \end{aligned}$$

(A3)

where $\mathcal {C}_0$ is an infinitesimal anti-clockwise circle about the origin. The result (123) in the main text follows. To see how to use it in determining the asymptotics of the joint PMF, let us make the decomposition $P_{\text {cut}}(\ell ,m) = P^{\alpha }(\ell ,m) + P^{\beta }(\ell ,m)$, where $P^{\alpha }(\ell ,m)$ denotes the component of the cut contribution to the joint PMF that arises solely from $J_{\text {scl}}^{\alpha }(\ell ,m)$, and where $P^{\beta }(\ell ,m)$ arises solely from $J_{\text {scl}}^{\beta }(\ell ,m)$. One can show that the $\beta$-component exactly cancels the pole term, $P^{\beta }(\ell ,m) = -P_{\text {pol}} (\ell ,m)$, which leads to the representation

$$\begin{aligned} \begin{aligned} P(\ell ,m)&= P_{\text {pol}}(\ell ,m) + P_{\text {cut}}(\ell ,m) \\&= P^{\alpha }(\ell ,m) \\&= (1-r)r^{m-1} r_{\text {hi}}^{\ell /2}\left[ \sqrt{r_{\text {hi}}}{\cdot }J_{\text {scl}}^{\alpha }(\ell ,m-1) - r{\cdot }J_{\text {scl}}^{\alpha }(\ell -1,m)\right] \;. \end{aligned} \end{aligned}$$

(A4)

It is straightforward to evaluate $J_{\text {scl}}^\alpha (\ell ,m)$ for $m = 0$ and confirm that the expected result for $P_{\text {xhi}}(\ell ) = P(\ell ,0)$ is recovered. We obtain $P_{\text {xhi}}(\ell ) = (1-r)r_{\text {hi}}^{\ell /2}{\cdot }d_\ell (\alpha )$ which, given (49) and (109), reproduces the form cited above (120). A by-product of this discussion is an alternative representation of the joint integral, given by

$$\begin{aligned} J_{\text {scl}}(\ell ,m) = d_{\ell +1}(\beta ) + \oint _{C_{\alpha }}\frac{dw}{2\pi i}\, \frac{d_{\ell +1}(w)}{w-\beta }\left( \frac{\beta -\alpha }{w-\alpha }\right) ^{m+1} . \end{aligned}$$

(A5)

The large-m asymptotics for fixed $\ell$ follow directly from (81) as

$$\begin{aligned} P_{\text {cut}}(\ell ,m) \mathrel {\mathop {\sim }_{m\rightarrow \infty }} \left[ \mathscr {C}_2^{\ell ,m}{\cdot }a(\ell +1) - \mathscr {C}_1^{\ell ,m}{\cdot }\ell \right] {\cdot }\frac{1}{\pi } \int _0^1 du\, \frac{\sqrt{u}}{(u+a)^{m+1}(u+b)} ,\nonumber \\ \end{aligned}$$

(A6)

where the asymptotics of the integral follow from (64) and (68) for non-vanishing and vanishing values of b, respectively. The results obtained here are consistent with those presented in [10], when specialized to the case of a common service rate.

Appendix B Laguerre series for the waiting time

Let us write $p_n \equiv P_\mathscr {L}(n)$ so that

$$\begin{aligned} p_n = \frac{1}{n!}{\cdot }\left. \frac{d^n}{dp^n} g_{\text {lo}}(z)\right| _{z=0} = \oint _{\mathcal {C}}\frac{dz}{2\pi i}\, \frac{g_{\text {lo}}(z)}{z^{n+1}} , \end{aligned}$$

(B7)

where the closed anti-clockwise traversed integration contour $\mathcal {C}$, initially taken to be a circle centred on the origin of radius $0< \eta < 1/r$, is deformed into the Bromwich contour $\Gamma$ on which $z = \delta +iy$ with $-\infty< y < +\infty$, for some $1< \delta < 1/r$ so that $\mathop {\textrm{Re}} \nolimits z > 1$. Then, we consider the Binomial transform of the $p_n$ sequence

$$\begin{aligned} \begin{aligned} \gamma _n&\equiv \sum _{k=0}^n \left( {\begin{array}{c}n\\ k\end{array}}\right) (-1)^k p_k \\&= \int _\Gamma \frac{dz}{2\pi i}\, g_{\text {lo}}(z)\sum _{k=0}^n \left( {\begin{array}{c}n\\ k\end{array}}\right) (-1)^k \frac{1}{z^{k+1}} \\&= \int _\Gamma \frac{dz}{2\pi i}\, \frac{g_{\text {lo}}(z)}{z}{\cdot } (1-1/z)^n \;. \end{aligned} \end{aligned}$$

(B8)

We now recall the generating function for the Laguerre polynomials $L_n(x)$, given by

$$\begin{aligned} \sum _{n=0}^\infty \tau ^n L_n(x) = \frac{1}{1-\tau }e^{-\tau x/(1-\tau )} . \end{aligned}$$

(B9)

On setting $\tau = 1 - 1/z$ and $x = r_{\text {lo}}t$, this reads

$$\begin{aligned} \sum _{n=0}^\infty \left( 1 - 1/z\right) ^nL_n(r_{\text {lo}}t) = z e^{-r_{\text {lo}}t(z-1)} , \end{aligned}$$

(B10)

from which it follows that

$$\begin{aligned} \sum _{n=0}^\infty \gamma _n L_n(r_{\text {lo}}t) = \int _\Gamma \frac{dz}{2\pi i}\, g_{\text {lo}}(z) e^{-r_{\text {lo}}t(z-1)} . \end{aligned}$$

(B11)

Now, (125) can be written as

$$\begin{aligned} P_\mathscr {L}(k) = \int _0^\infty dt'\, P'_\mathscr {W}(t') \frac{t^{\prime k}}{k!}e^{-t'} , \end{aligned}$$

(B12)

where $t' = r_{\text {lo}}t$ and $P'_\mathscr {W}(t')dt' = P_\mathscr {W}(t)dt$. We also have

$$\begin{aligned} L_n(t) = \sum _{k=0}^n \left( {\begin{array}{c}n\\ k\end{array}}\right) \frac{(-1)^k}{k!} t^k . \end{aligned}$$

(B13)

These may be combined to yield

$$\begin{aligned} \int _0^\infty dt'\, e^{-t'}L_n(t') P'_\mathscr {W}(t') = \sum _{k=0}^n \left( {\begin{array}{c}n\\ k\end{array}}\right) (-1)^k \int _0^\infty dt'\, P'_\mathscr {W}(t') \frac{t^{\prime k}}{k!}e^{-t'} = \gamma _n .\nonumber \\ \end{aligned}$$

(B14)

The completeness relation [37]

$$\begin{aligned} \sum _{n=0}^\infty L_n(t)L_n(t') = e^{(t+t')/2}{\cdot }\delta (t-t') , \end{aligned}$$

(B15)

$\delta (t)$ being the Dirac delta function, implies the convergent Laguerre-series representation

$$\begin{aligned} P'_\mathscr {W}(t') = \sum _{n=0}^\infty \gamma _n L_n(t') , \end{aligned}$$

(B16)

and we conclude, via (B11), that

$$\begin{aligned} P_\mathscr {W}(t)dt = r_{\text {lo}}dt{\cdot }\int _\Gamma \frac{dz}{2\pi i}\, g_{\text {lo}}(z) e^{-r_{\text {lo}}t(z-1)} . \end{aligned}$$

(B17)

The Bromwich contour $\Gamma$, with $\Re z > 1$, may now be deformed as $\Gamma \mapsto \mathcal {C}_{\text {pol}} \cup \mathcal {C}_{\text {cut}}$ so that it wraps around the pole and cut of $g_{\text {lo}}(z)$. This serves to reproduce (130).

The convergence claim for (B16) follows from the theorem that the formal expansion

$$\begin{aligned} \gamma (t) \equiv \sum _{k=0}^\infty \gamma _k L_k(t) \end{aligned}$$

(B18)

converges in $L_2$ if and only if $\Vert \gamma \Vert ^2_{L_2} < \infty$, where

$$\begin{aligned} \Vert \gamma \Vert ^2_{L_2} \equiv \int _0^\infty dt\, e^{-t}|\gamma (t)|^2 = \sum _{k=0}^\infty |\gamma _k|^2 . \end{aligned}$$

(B19)

In the present application, we have $\gamma (t) = P'_{\mathscr {W}}(t)$ for which, trivially,

$$\begin{aligned} \Vert P'_\mathscr {W}\Vert _{L_2}^2 = \int _0^\infty dt\, e^{-t}\left[ P'_\mathscr {W}(t)\right] ^2 < \int _0^\infty dt\, e^{-t} = 1 . \end{aligned}$$

(B20)

One should note that, while the coefficients $\gamma _n$ of the Laguerre series (B16) are constructed as (potentially) numerically problematic alternating combinatorial sums, the last equality in (B8) shows that they can be efficiently computed by means of the very same iterative quadrature method derived for the queue-length probabilities $p_n$. Explicitly,

$$\begin{aligned} \begin{aligned} \gamma _n&= \Theta (r^2 - r_{\text {hi}}) \left[ 1 - \frac{r(1-r)}{r_{\text {lo}}}\right] {\cdot }\frac{(1-r)^{n+1}}{r} \\&\quad {}+ \frac{1-r}{2\pi r}\int _0^1 du\,\sqrt{u(1-u)}{\cdot } \frac{(u+c)^n}{(u + a)^{n+1}(u + b)} , \end{aligned} \end{aligned}$$

(B21)

where $c \equiv (x_- - 1)/x_{\text {dif}} = (1-r)a + rb$.