Inverse estimation of the time-dependent wall temperature in stagnation region of an annular jet on a cylinder rod using Levenberg–Marquardt method

Abstract

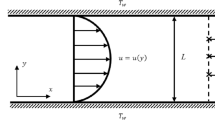
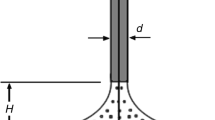
For the first time, a numerical solution code, based on Levenberg–Marquardt method is presented for solving non-linear problem of inverse heat transfer in axisymmetric stagnation flow im**ing on a cylinder rod to determine time-dependent wall temperature by temperature distribution at a specific point in the fluid region. Also, the effect of noisy data on the final result has been studied. For this purpose, the numerical solution of the dimensionless temperature and the convective heat transfer in a radial incompressible flow on a cylinder axis is carried out as a direct problem. In the direct problem, the free stream is steady with an initial flow strain rate of $\overline{k}$. Using similarity variable and appropriate transformations, momentum and energy equations are converted into semi-similar equations. The new equation systems are then discretized using an implicit finite difference method and solved by applying the tridiagonal matrix algorithm (TDMA). The wall temperature is then estimated by applying the Levenberg–Marquardt parameter estimation approach. This technique is an iterative approach based on minimizing the least-square summation of the error values, the error being the difference between the estimated and measured temperatures. Results of the inverse analysis indicate that the Levenberg–Marquardt algorithm is an efficient and acceptably stable technique for estimating wall temperature in axisymmetric stagnation flow. The maximum value of the sensitivity coefficient is related to the estimation of polynomial wall temperature and its value is 0.1952 also the minimum value of the sensitivity coefficient is 8.62 × 10^–6 which is related to the triangular wall temperature. The results show that the parameter estimation error in calculating the triangular and trapezoidal wall temperature is greater than the others because the maximum value of RMS error is obtained for these two cases, which are 0.451 and 0.479, respectively, the reason for the increase in error in estimating these functions is the existence of points where the first derivative of the function does not exist. This method also exhibits considerable stability for noisy input data.

Appendix: Numerical solution method

Before solving the third-order differential Eq. (9), a change of the variable is applied as bellow:

$$ \xi = f^{\prime} \to \xi^{\prime} = f^{\prime \prime} \to \xi^{\prime \prime} = f^{\prime \prime \prime } $$

This formulation is necessary to eliminate the need for third-order difference and to obtain a tridiagonal system of linear algebraic equations at further stage of the analysis.

The first step in solving the system of non-linear ordinary differential Eqs. (9) and (12) is to convert them into a system of quasi-linear differential equations.

$$ \begin{aligned} & \frac{{\partial \xi^{({\text{k}} + 1)} }}{\partial \tau } + M_{0} \xi^{{{\prime \prime }({\text{k}} + 1)}} + M_{1} \xi^{{{\prime }({\text{k}} + 1)}} + M_{2} \xi^{({\text{k}} + 1)} + M_{3} f^{({\text{k}})} = M_{4} \\ & \frac{{\partial \theta^{({\text{k}} + 1)} }}{\partial \tau } + L_{0} \theta^{{{\prime \prime }({\text{k}} + 1)}} + L_{1} \theta^{{{\prime }({\text{k}} + 1)}} + L_{2} \theta^{({\text{k}} + 1)} + L_{3} f^{({\text{k}} + 1)} = L_{4} \\ \end{aligned} $$

where

$$ \begin{aligned} & M_{0} = - \frac{\eta }{{\text{Re}}} \\ & M_{1} = - \frac{1}{{\text{Re}}} - \frac{{f^{(k)} }}{{\text{Re}}} \\ & M_{2} = 2\xi^{(k)} \\ & M_{3} = - \frac{{\xi^{{{\prime }(k)}} }}{{\text{Re}}} \\ & M_{4} = \xi^{2(k)} - \frac{{f^{(k)} }}{{\text{Re}}}\xi^{{{\prime }(k)}} + 1 \\ & L_{0} = - \frac{\eta }{{{\text{Re}} \,\Pr }} \\ & L_{1} = - \frac{1}{{{\text{Re}} \,\Pr }} - f \\ & L_{2} = \frac{{{{{\text{d}}T_{\rm w} (\tau )} \mathord{\left/ {\vphantom {{{\text{d}}T_{\rm w} (\tau )} {{\text{d}}\tau }}} \right. \kern-\nulldelimiterspace} {{\text{d}}\tau }}}}{{T_{\rm w} (\tau ) - T_{\infty } }} \\ & L_{3} = - \theta^{{{\prime }(k)}} \\ & L_{4} = - f\theta^{{{\prime }(k)}} \\ \end{aligned} $$

where k and k + 1 are the iteration indices.

In the numerical analysis, we replace the boundary conditions $\xi^{({\text{k}} + 1)} (\infty ,\tau ) = 1$, $\theta^{({\text{k}} + 1)} (\infty ,\tau ) = 0$ by

$$ \xi^{({\text{k}} + 1)} (\eta_{\text{e}} ,\tau ) = 1,\;\;\theta^{({\text{k}} + 1)} (\eta_{\text{e}} ,\tau ) = 0. $$

where $\eta_{e}$ is a sufficiently large value of $\eta$.

Then, the problem has been written in a finite difference form. The interval $1 \le \eta \le \eta_{{\text{e}}}$ has been divided into (N − 1) equal intervals and denotes the values of the dependent variables at $\eta_{\rm i} = 1 + (i - 1)h$ with the subscript i (= 1, 2,...,N) where $h = {{(\eta_{{\text{e}}} - 1)} \mathord{\left/ {\vphantom {{(\eta_{e} - 1)} {(N - 1)}}} \right. \kern-\nulldelimiterspace} {(N - 1)}}$. Substituting, as usual, the expressions

$$ \begin{gathered} \xi^{{\prime \prime }} = \frac{{\xi_{{\rm i} + 1} - 2\xi_{\rm i} + \xi_{{\rm i} - 1} }}{{h^{2} }},\quad \xi^{{\prime }} = \frac{{\xi_{{\text{i}} + 1} - \xi {}_{{\text{i}} - 1}}}{2h}, \hfill \\ \theta^{{\prime \prime }} = \frac{{\theta_{{\rm i} + 1} - 2\theta_{\rm i} + \theta_{{\text{i}} - 1} }}{{h^{2} }},\quad \theta^{{\prime }} = \frac{{\theta_{{\text{i}} + 1} - \theta {}_{{\text{i}} - 1}}}{2h} \hfill \\ \frac{{\partial \xi_{\rm i} }}{\partial \tau } = \frac{{\xi_{\rm i} - \xi_{\rm i}^{{{\text{old}}}} }}{\Delta \tau },\quad \frac{{\partial \theta_{\rm i} }}{\partial \tau } = \frac{{\theta_{\rm i} - \theta_{\rm i}^{{{\text{old}}}} }}{\Delta \tau } \hfill \\ \end{gathered} $$

In to equations and using the boundary conditions, at every time step, we obtain a system of linear algebraic equations in a tridiagonal form:

$$ \begin{aligned} & A_{2,2} \xi_{2}^{({\text{k}} + 1)} + A_{2,3} \xi_{3}^{({\text{k}} + 1)} = C_{2} - A_{2,1} \\ & A_{{\text i},{\text i} - 1} \xi_{{\text i} - 1}^{({\text{k}} + 1)} + A_{{\rm i},{\rm i}} \xi_{\rm i}^{({\text{k}} + 1)} + A_{{\rm i},{\rm i} + 1} \xi_{{\rm i} + 1}^{({\text{k}} + 1)} = C_{\rm i} \quad (i = 3,4, \ldots ,{\text{N}} - 2) \\ & A_{{\text{N}} - 1,{\text{N}} - 2} \xi_{{\text{N}} - 2}^{({\text{k}} + 1)} + A_{{\text{N}} - 1,{\text{N}} - 1} \xi_{{\text{N}} - 1}^{({\text{k}} + 1)} = C_{{\text{N}} - 1} - A_{{\text{N}} - 1,{\text{N}}} \\ & A_{2,2}^{{\prime }} \theta_{2}^{({\text{k}} + 1)} + A_{2,3}^{{\prime }} \theta_{3}^{({\text{k}} + 1)} = C_{2}^{{\prime }} - A_{2,1}^{{\prime }} \\ & A^{\prime}_{{\text{i}},{\text{i}} - 1} \theta_{{\text{i}} - 1}^{({\text{k}} + 1)} + A_{{\text{i}},{\text{i}}}^{{\prime }} \theta_{\rm i}^{({\text{k}} + 1)} + A_{{\text{i}},{\text{i}} + 1}^{{\prime }} \theta_{{\text{i}} + 1}^{({\text{k}} + 1)} = C_{\rm i}^{{\prime }} \quad ({\text{i}} = 3,4, \ldots ,{\text{N}} - 2) \\ & A_{{\text{N}} - 1,{\text{N}} - 2}^{{\prime }} \theta_{{\text{N}} - 2}^{({\text{k}} + 1)} + A_{{\text{N}} - 1,{\text{N}} - 1}^{{\prime }} \theta_{{\text{N}} - 1}^{({\text{k}} + 1)} = C_{{\text{N}} - 1}^{{\prime }} - A_{{\text{N}} - 1,{\text{N}}}^{{\prime }} \\ \end{aligned} $$

where

$$ \begin{aligned} & A_{{\text{i}},{\text{i}} - 1} = \frac{{M_{0} }}{{h^{2} }} - \frac{{M_{1} }}{2h} \\ & A_{{\text{i}},{\text{i}}} = \frac{1}{\Delta \tau } - \frac{{2M_{0} }}{{h^{2} }} + M_{2} \\ & A_{{\text{i}},{\text{i}} + 1} = \frac{{M_{0} }}{{h^{2} }} + \frac{{M_{1} }}{2h} \\ & C_{\rm i} = M_{4} + \frac{{\xi_{\rm i}^{{({\text{old}})}} }}{\Delta \tau } - M_{3} f_{\rm i}^{(k)} \\ & A_{{\text{i}},{\text{i}} - 1}^{{\prime }} = \frac{{L_{0} }}{{h^{2} }} - \frac{{L_{1} }}{2h} \\ & A_{{\text{i}},{\text{i}}}^{{\prime }} = \frac{1}{\Delta \tau } - \frac{{2L_{0} }}{{h^{2} }} + L_{2} \\ & A_{{\text{i}},{\text{i}} + 1}^{{\prime }} = \frac{{L_{0} }}{{h^{2} }} + \frac{{L_{1} }}{2h} \\ & C_{\rm i}^{{\prime }} = L_{4} + \frac{{\theta_{\rm i}^{{({\text{old}})}} }}{\Delta \tau } - L_{3} f_{\rm i} \\ \end{aligned} $$

In the above relations, the superscript (old) represents the calculated value of $\xi$ and $\theta$ in the previous time step.

These systems are composed of (N-2) equations for (N-2) unknowns $\xi_{\rm i}^{({\text{k}} + 1)} ,\theta_{\rm i}^{({\text{k}} + 1)}$. It can be solved quite easily by usual swee** method. Once all of $\xi_{\rm i}^{({\text{k}} + 1)}$ are determined, $f_{\rm i}^{({\text{k}} + 1)}$ is obtained from $\xi = f^{\prime}$, namely:

$$ f_{\rm i}^{({\text{k}} + 1)} = \int\limits_{1}^{{\eta_{\rm i} }} {\xi_{\rm i}^{({\text{k}} + 1)} {\text{d}}\eta } $$

executing a numerical integration. The values of $\xi_{\rm i}^{({\text{k}} + 1)}$ and $f_{\rm i}^{({\text{k}} + 1)}$ obtained here are used to replace $\xi_{\rm i}^{({\text{k}})}$ and $f_{\rm i}^{(k)}$ for the next cycle. The convergence is considered achieved if $\left| {\xi_{\rm i}^{(k + 1)} - \xi_{\rm i}^{(k)} } \right| \le \varepsilon$ and $\left| {\theta_{\rm i}^{(k + 1)} - \theta_{\rm i}^{(k)} } \right| \le \varepsilon$ for all points, where $\varepsilon$ is a prescribed accuracy criterion.