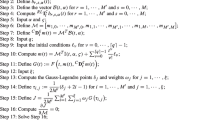SpringerLink
Log in

Extended barycentric rational schemes for functions of singularities

  • Published:
Calcolo Aims and scope Submit manuscript

Abstract

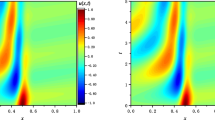
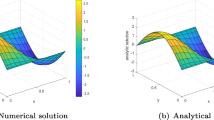
In this paper, we propose two kinds of extended barycentric rational schemes via (non-conformally) scaled transformations for approximating functions of singularities, which are bulit upon applying the barycentric interpolation formula of the second kind at two kinds of mapped nodes: (i) equispaced nodes and (ii) (shifted) Chebyshev nodes. While the weights in interpolation formula are selected inspired by the works of Berrut, Floater and Hormann. Ample numerical tests show that the extended barycentric rational schemes are efficient and can achieve higher convergence rates as the scaled parameter and the degree of the local approximation polynomial increase. Moreover, from the barycentric formula, it is easy to derive the difference matrices at these mapped nodes, which leads to an accurate Levin method for dealing with highly oscillatory integrals with the integrands of algebraic singularities. Numerical experiments are carried out to illustrate the effectiveness and accuracy of the proposed schemes.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Baltensperger, R., Berrut, J.-P., Noël, B.: Exponential convergence of a linear rational interpolant between transformed Chebyshev points. Math. Comp. 68(227), 1109–1121 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  2. Bernstein, S.: Sur la meilleure approximation de \(|x|\) par des polynomes de degrés donnés. Acta Math. 37, 1–57 (1914)

    Article  MathSciNet  MATH  Google Scholar 

  3. Bernstein, S.: Sur la meilleure approximation de \(|x|^{p}\) par des polynômes de degrés très élevés. Izv. Akad. Nauk SSSR Ser. Mat. 2, 169–190 (1938)

    MATH  Google Scholar 

  4. Berrut, J.-P.: Rational functions for guaranteed and experimentally well-conditioned global interpolation. Comput. Math. Appl. 15(1), 1–16 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  5. Berrut, J.-P., Klein, G.: Recent advances in linear barycentric rational interpolation. J. Comput. Appl. Math. 259, 95–107 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  6. Berrut, J.-P., Trefethen, L.N.: Barycentric Lagrange interpolation. SIAM Rev. 46(3), 501–517 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  7. Bos, L., De Marchi, S., Hormann, K.: On the Lebesgue constant of Berrut’s rational interpolant at equidistant nodes. J. Comput. Appl. Math. 236(4), 504–510 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  8. Bos, L., De Marchi, S., Hormann, K., Klein, G.: On the Lebesgue constant of barycentric rational interpolation at equidistant nodes. Numer. Math. 121(3), 461–471 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  9. Dupuy, M.: Les études du professeur marcantoni sur les applications du calcul matriciel a la compensation des grands réseaux. Bulletin géodésique 9(1), 241–250 (1948)

    Article  Google Scholar 

  10. Floater, M.S., Hormann, K.: Barycentric rational interpolation with no poles and high rates of approximation. Numer. Math. 107(2), 315–331 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  11. Hale, N., Trefethen, L.N.: Chebfun and numerical quadrature. Sci. Chin. Math. 55, 1749–1760 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  12. Higham, N.: The numerical stability of barycentric Lagrange interpolation. IMA J. Numer. Anal. 24(4), 547–556 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  13. Güttel, S., Klein, G.: Convergence of linear barycentric rational interpolation for analytic functions. SIAM J. Numer. Anal. 50(5), 2560–2580 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  14. Kong, D., **ang, S.: Fast linear barycentric rational interpolation for singular functions via scaled transformations. (2021). ar**v:2101.07949

  15. Levin, D.: Procedures for computing one- and two-dimensional integrals of functions with rapid irregular oscillations. Math. Comp. 38(158), 531–538 (1982)

    Article  MathSciNet  MATH  Google Scholar 

  16. Olver, S.: Moment-free numerical integration of highly oscillatory functions. IMA J. Numer. Anal. 26(2), 213–227 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  17. Olver, S.: Shifted GMRES for oscillatory integrals. Numer. Math. 114, 607–628 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  18. Salzer, H.E.: Lagrangian interpolation at the Chebyshev points \(x_{n,\nu } \equiv \cos (\nu \pi /n), \nu = 0(1)n\); some unnoted advantages. Comput. J. 15(2), 156–159 (1972)

    Article  MathSciNet  MATH  Google Scholar 

  19. Stahl, H.R.: Best uniform rational approximation of \(x^\alpha \) on \([0, 1]\). Acta Math. 190(2), 241–306 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  20. Trefethen, L.N.: Approximation Theory and Approximation Practice, Extended Edition. Society for Industrial and Applied Mathematics (2019)

  21. Wang, H., Huybrechs, D., Vandewalle, S.: Explicit barycentric weights for polynomial interpolation in the roots or extrema of classical orthogonal polynomials. Math. Comp. 83(290), 2893–2914 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  22. Wang, H., **ang, S.: On the convergence rates of Legendre approximation. Math. Comp. 81, 861–877 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  23. Klein, G.: An extension of the Floater-Hormann family of barycentric rational interpolants. Math. Comp. 82(284), 2273–2292 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  24. Engquist, B., Fokas, A., Hairer, E., Iserles, A.: Highly Oscillatory Problems. Cambridge University Press, Cambridge (2009)

    Book  MATH  Google Scholar 

  25. Deaño, A., Huybrechs, D., Iserles, A.: Computing highly oscillatory integrals. Society for Industrial and Applied Mathematics (2017)

  26. Higham, N.J.: Functions of matrices: theory and computation. Society for Industrial and Applied Mathematics (2008)

  27. Wang, Y., **ang, S.: Levin methods for highly oscillatory integrals with singularities. Sci. Chin. Math. 65(3), 603–622 (2022)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors are grateful for the referees’ helpful suggestions and insightful comments, which helped improve the manuscript significantly. This work was supported by the National Natural Science Foundation of China (No. 12271528). The first author is partly supported by the Fundamental Research Funds for the Central Universities of Central South University (No. 2020zzts031).

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Central South University, Changsha, 410083, Hunan, People’s Republic of China

    Desong Kong, Shuhuang **ang, Li Li & Ran Li

Authors
  1. Desong Kong
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Shuhuang **ang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Li Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Ran Li
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Shuhuang **ang.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kong, D., **ang, S., Li, L. et al. Extended barycentric rational schemes for functions of singularities. Calcolo 59, 35 (2022). https://doi.org/10.1007/s10092-022-00480-7

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10092-022-00480-7

Keywords

Mathematics Subject Classification

Search

Navigation