Stability analyses of compressible flat plate boundary layer flow over a mechanically compliant wall

Abstract

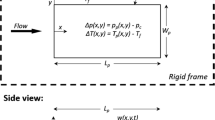
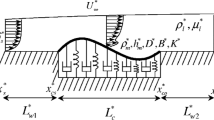
Sustained flight at hypersonic speeds is characterized by high pressure and aerothermal loads imposed on the structure of the aerodynamic vehicle. A consequence of lightening the structural design permits fluid–structure interaction phenomena that can significantly alter the flow and initiate unsteady structural responses. We investigate the coupling between high-speed laminar boundary layer flows over a mechanically compliant panel and analyze the dynamic system response of the coupled system to boundary layer instabilities by means of local convective linear stability analysis. The resulting non-dimensional interaction parameters describing the compliant system are shown to affect the boundary layer instabilities in the infinitely thin panel limit, and the transition from the rigid limit is described by two distinctly different responses: (a) a piston-like, one-dimensional panel deflection, or (b) a synchronization with flexural waves. Compliance is shown to non-monotonically change convective wave growth rates and induce uncertainty in the integrated N-factors.

Eigenvalue problem

The quadratic eigenvalue problem described in Eq. 5 can be rewritten as a first-order system following

$$\begin{aligned} \begin{bmatrix} L^1 &{}\quad L^0 \\ I &{}\quad 0 \end{bmatrix} {\hat{z}}= \alpha \begin{bmatrix} -L^2 &{}\quad 0\\ 0 &{}\quad I \end{bmatrix} {\hat{z}} \quad \text {with} \quad {\hat{z}} = \begin{bmatrix} \alpha {\hat{q}}\\ {\hat{q}} \end{bmatrix}, \end{aligned}$$

(18)

where I denotes the identity matrix and 0 is a zero matrix. Similarly, the quartic eigenvalue problem for the compliant system can be rewritten as a first-order system following

$$\begin{aligned} \begin{bmatrix} L^1 &{}\quad L^0 &{}\quad L_2 &{}\quad 0\\ 0 &{}\quad 0 &{}\quad 0 &{}\quad I\\ 0 &{}\quad 0 &{}\quad I &{}\quad 0 \\ I &{}\quad 0 &{}\quad 0 &{}\quad 0 \end{bmatrix} {\hat{z}} = \alpha \begin{bmatrix} 0 &{}\quad 0 &{}\quad 0 &{}\quad -L_4\\ 0 &{}\quad 0 &{}\quad I &{}\quad 0 \\ I &{}\quad 0 &{}\quad 0 &{}\quad 0 \\ 0 &{}\quad I &{}\quad 0 &{}\quad 0\\ \end{bmatrix} {\hat{z}} \quad \text {with} \quad {\hat{z}}=\begin{bmatrix} \alpha {\hat{q}}\\ {\hat{q}} \\ \alpha ^2 {\hat{q}} \\ \alpha ^3 {\hat{q}} \end{bmatrix}. \end{aligned}$$

(19)

The components to the submatrices $[L^0,L^1,L^2,L^4]$ follow the pattern

$$\begin{aligned} L^i = \begin{bmatrix} L^i_{11} &{}\quad L^i_{12} &{}\quad L^i_{13} &{}\quad L^i_{14} &{}\quad L^i_{15}\\ L^i_{21} &{}\quad L^i_{22} &{}\quad L^i_{23} &{}\quad L^i_{24} &{}\quad L^i_{25}\\ L^i_{31} &{}\quad L^i_{32} &{}\quad L^i_{33} &{}\quad L^i_{34} &{}\quad L^i_{35}\\ L^i_{41} &{}\quad L^i_{42} &{}\quad L^i_{43} &{}\quad L^i_{44} &{}\quad L^i_{45}\\ L^i_{51} &{}\quad L^i_{52} &{}\quad L^i_{53} &{}\quad L^i_{54} &{} \quad L^i_{55}\\ \end{bmatrix} \end{aligned}$$

(20)

with the following nonzero components:

$$\begin{aligned} L^0_{11}&= D_2 + \frac{1}{\mu } \frac{\partial \mu }{\partial T} \frac{\partial T}{\partial T} D_1 - i \left( \beta W - \omega \right) \frac{{{Re}}}{\mu T} -\beta ^2 \\ L^0_{12}&= -\frac{{{Re}}}{\mu T} \frac{\partial U}{\partial y} \\ L^0_{14}&= \frac{1}{\mu } \frac{\partial \mu }{\partial y} \frac{\partial U}{\partial y} D_1 + \frac{1}{\mu } \frac{\partial \mu }{\partial T} \frac{\partial ^2 U}{\partial y^2} + \frac{1}{\mu } \frac{\partial ^2 \mu }{\partial y^2} \frac{\partial T}{\partial y} \frac{\partial U}{\partial y} \\ L^0_{22}&= D_2 + \frac{1}{\mu }\frac{\partial \mu }{\partial T} \frac{\partial T}{\partial y} D_1-i \left( \beta W -\omega \right) \frac{{{Re}}}{l_2 \mu T} - \frac{\beta }{l_2} \\ L^0_{23}&= \frac{{{Re}}}{l_2 \mu } D_1 \\ L^0_{24}&= \frac{i}{l_2 \mu } \frac{\partial \mu }{\partial T} \frac{\partial U}{\partial y} \\ L^0_{25}&= i \beta \frac{l_1}{l_2} D_1 + i \beta \frac{l_0}{l_2 \mu } \frac{\partial \mu }{\partial T} \frac{\partial T}{\partial y} \\ L^0_{32}&= D_1 - \frac{1}{T} \frac{\partial T}{\partial y} \\ L^0_{33}&= i \gamma M^2 \left( \beta W - \omega \right) \\ L^0_{34}&= -\frac{i}{T} \left( \beta W -\omega \right) \\ L^0_{35}&= i \beta \\ L^0_{41}&= 2 {{Pr}}\gamma M^2 \frac{\partial U}{\partial y} D_1 \\ L^0_{42}&= 2 i \left( \gamma -1\right) M^2 Pr \beta \frac{\partial W}{\partial y} - \frac{{{Pr}}{{Re}}}{\mu T} \frac{\partial T}{\partial y} \\ L^0_{43}&= i \left( \beta W -\omega \right) \left( \gamma -1\right) M^2 \frac{{{Pr}}{{Re}}}{\mu } \\ L^0_{44}&= D_2 + \frac{2}{k} \frac{\partial k}{\partial y} D_1 -i \left( \beta W -\omega \right) \frac{{{Pr}}{{Re}}}{\mu T} + \left( \gamma -1 \right) \frac{{{Pr}}}{\mu } \frac{\partial \mu }{\partial y} \left[ \left( \frac{\partial U}{\partial y} \right) ^2 + \left( \frac{\partial W}{\partial y} \right) ^2 \right] \\&\quad + \frac{1}{k} \frac{\partial ^2 k}{\partial y^2} - \beta ^2 \\ L^0_{45}&= 2 {{Pr}}\left( \gamma -1 \right) M^2 \frac{\partial W}{\partial y} D_1 \\ L^0_{52}&= i \beta l_1 D_1 + i \frac{\beta }{\mu } \frac{\partial \mu }{\partial y} \frac{\partial T}{\partial y} - \frac{{{Re}}}{\mu T} \frac{\partial W}{\partial y} \\ L^0_{53}&= -i \beta \frac{Re}{mu} \\ L^0_{54}&= \frac{1}{\mu } \frac{\partial \mu }{\partial T} \frac{\partial W}{\partial y} D_1 + \frac{1}{\mu } \frac{\partial \mu }{\partial T} \frac{\partial ^2 W}{\partial y^2} + \frac{1}{\mu } \frac{\partial ^2 \mu }{\partial T^2} \frac{\partial T}{\partial y} \frac{\partial W}{\partial y} \\ L^0_{55}&= D_2 + \frac{1}{\mu } \frac{\partial \mu }{\partial T} \frac{\partial T}{\partial y} D_1 -i \left( \beta W -\omega \right) \frac{{{Re}}}{\mu T} - l_2 \beta ^2 \\ L^1_{11}&= -i U \frac{{{Re}}}{\mu T} \\ L^1_{12}&= i l_1 D_1 + \frac{i}{\mu } \frac{\partial \mu }{\partial T} \frac{\partial T}{\partial y} \\ L^1_{13}&= -i \frac{Re}{\mu } \\ L^1_{15}&= -\beta l_1 \\ L^1_{21}&= i \frac{l_1}{l_2} D_1 + \frac{i}{\mu } \frac{\partial \mu }{\partial T} \frac{\partial T}{\partial y} \frac{l_0}{l_2} \\ L^1_{22}&= -i U \frac{{{Re}}}{l_2 \mu T} \\ L^1_{24}&= -i U \frac{{{Re}}}{l_2 \mu T} \\ L^1_{31}&= i \\ L^1_{33}&= i \gamma M^2 U \\ L^1_{34}&= -i \frac{U}{T}\\ L^1_{42}&= 2 i \left( \gamma -1\right) M^2 {{Pr}}\frac{\partial U}{\partial y} \\ L^1_{43}&= i \left( \gamma -1\right) M^2 \frac{{{Pr}}{{Re}}}{\mu } \\ L^1_{44}&= -i U \frac{{{Pr}}{{Re}}}{\mu T} \\ L^1_{51}&= -\beta l_1 \\ L^1_{55}&= -i U \frac{{{Re}}}{\mu T} \\ L^2_{11}&= \frac{i}{\mu } \frac{\partial \mu }{\partial T} \frac{\partial T}{\partial y} \frac{l_0}{l_2} \\ L^2_{22}&= - \frac{1}{l_2} \\ L^2_{44}&= -1 \\ L^2_{55}&= -1, \end{aligned}$$

where $D_1$ and $D_2$ denote the discrete first and second derivative operator with respect to wall-normal coordinate y and $l_i = i + k/\mu$. These submatrices arise from sorting the governing perturbation equations (Eqs. 1–3 in conjunction with the perturbation ansatz Eq. 4) in the following order:

$$\begin{aligned} \begin{bmatrix} \text {continuity} \\ \text {x-momentum equation} \\ \text {y-momentum equation} \\ \text {z-momentum equation} \\ \text {energy equation} \\ \end{bmatrix} \end{aligned}$$

(21)

In terms of the boundary conditions of the rigid system, we enforce $[{\hat{u}}_w, {\hat{u}}_{\infty }]=0$ in the x-momentum equation, $[{\hat{v}}_w, {\hat{v}}_{\infty }]=0$ in the y-momentum equation, $[{\hat{w}}_w, {\hat{w}}_{\infty }]=0$ in the z-momentum equation, and $[{\hat{T}}_w, {\hat{T}}_{\infty }]=0$ in the energy equation. For the compliant system, we substitute $[{\hat{v}}_w, {\hat{v}}_{\infty }]=0$ with Eq. 10, which changes the following components of above submatrices $L^i$ at the wall:

$$\begin{aligned} L^0_{22}&= \omega ^2 + i \beta ^4 \mu \phi \\ L^0_{23}&= \omega \\ L^2_{22}&= 2 i \beta ^2 \mu \phi \\ L^4_{22}&= i \mu \phi .\\ \end{aligned}$$