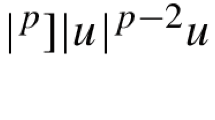Abstract
This note studies the Schrödinger–Choquard equation with an inhomogeneous combined source term and a fractional Laplacian operator
Indeed, for \(b\ne 0\) and \(0<s<1\), one obtains a sharp threshold of global existence versus finite time blow-up dichotomy for mass-super-critical and energy sub-critical radial solutions.
Similar content being viewed by others
References
Boulenger, T., Himmelsbach, D., Lenzmann, E.: Blow-up for fractional NLS. J. Funct. Anal. 271, 2569–2603 (2016)
Cho, Y., Hwang, G., Kwon, S., Lee, S.: On finite time blow-up for the mass-critical Hartree equations. Proc. R. Soc. Edinb. Sect. A 145(3), 467–479 (2015)
Cho, Y., Hwang, G., Kwon, S., Lee, S.: Profile decomposition and blow-up phenomena of mass-critical fractional Schrödinger equations. Nonlinear Anal. 86, 12–29 (2013)
Cho, Y., Ozawa, T.: Sobolev inequalities with symmetry. Commun. Contemp. Math. 11, 355–365 (2009)
Dinh, V.D.: On blowup solutions to the focusing mass-critical nonlinear fractional Schrödinger equation. Commun. Pure Appl. Anal. 18, 689–708 (2019)
Dinh, V.D.: A study on blowup solutions to the focusing \(L^2\)-supercritical nonlinear fractional Schrödinger equation. J. Math. Phys. 59, 071506 (2018)
D’avenia, P., Siciliano, G., Squassina, M.: On fractional Choquard equations. Math. Model. Methods Appl. Sci. 25(8), 1447–1476 (2015)
Elgart, A., Schlein, B.: Mean field dynamics of Boson Stars. Commun. Pure Appl. Math. 60(4), 500–545 (2007)
Feng, B., Wang, Y.: Sharp thresholds of blow-up and global existence for the Schrödinger equation with combined power-type and Choquard-type nonlinearities. Bound. Value Probl. 2019, 195 (2019)
Feng, B., Zhang, H.: Stability of standing waves for the fractional Schrödinger–Hartree equation. J. Math. Anal. App. 460(1), 352–364 (2018)
Gill, T.S.: Optical guiding of laser beam in nonuniform plasma. Pranama J. Phys. 55, 845–852 (2000)
Guo, B., Huo, Z.: Global well-posedness for the fractional nonlinear Schrödinger equation. Commun. Partial Differ. Equ. 36(2), 247–255 (2010)
Guo, B., Huo, Z.: Well-posedness for the nonlinear fractional Schrödinger equation and inviscid limit behavior of solution for the fractional Ginzburg-Landau equation, ract. Calc. Appl. Anal. 16(1), 226–242 (2013)
Guo, Z., Sire, Y., Wang, Y., Zhao, L.: On the energy-critical fractional Schrödinger equation in the radial case. Dyn. Parttial Differ. Equ. 15, 265–282 (2018)
Guo, Z., Wang, Y.: Improved Strichartz estimates for a class of dispersive equations in the radial case and their applications to nonlinear Schrödinger and wave equations. J. Anal. Math. 124(1), 1–38 (2014)
Gurevich, B., Jeffrey, A., Pelinovsky, E.N.: A method for obtaining evolution equations for nonlinear waves in a random medium. Wave Motion 17(287), 287–295 (1993)
Iomin, A.: Fractional Schrödinger equation in gravitational optics. Mod. Phys. Lett. A (To appear)
Laskin, N.: Fractional quantum mechanics and Levy path integrals. Phys. Lett. A. 268, 298–304 (2000)
Laskin, N.: Fractional Schrödinger equation. Phys. Rev. E. 66, 056108 (2002)
Lieb, E.: Analysis, Graduate Studies in Mathematics, vol. 14, 2nd edn. American Mathematical Society, Providence (2001)
Lieb, E.H., Yau, H.-T.: The Chandrasekhar theory of stellar collapse as the limit of quantum mechanics. Commun. Math. Phys. 112, 147–174 (1987)
Liu, C.S., Tripathi, V.K.: Laser guiding in an axially nonuniform plasma channel. Phys. Plasmas 1, 3100–3103 (1994)
Nirenberg, L.: On elliptic partial differential equations. Ann. Scuola Norm. Sup. Pisa Cl. Sci. 13, 116–162 (1955)
Peng, C., Zhang, Y., Ma, C.: Blow-up dynamics of \(L^2\)-critical inhomogeneous fractional nonlinear Schrödinger equation. Math. Methods Appl. Sci. 41, 1–10 (2019)
Peng, C., Zhao, D.: Global existence and blowup on the energy space for the inhomogeneous fractional nonlinear Schrödinger equation. Discrete Contin. Dyn. Syst. B 24(7), 3335–3356 (2019)
Saanouni, T.: Remarks on the inhomogeneous fractional nonlinear Schrödinger equation. J. Math. Phys. 57, 081503 (2016)
Saanouni, T.: Strong instability of standing waves for the fractional Choquard equation. J. Math. Phys. 59, 081509 (2018)
Saanouni, T.: A note on the fractional Schrödinger equation of Choquard type. J. Math. Anal. Appl. 470(2), 1004–1029 (2019)
Shen, Z., Gao, F., Yang, M.: Ground states for nonlinear fractional Choquard equations with general non-linearities. Math. Methods Appl. Sci. 39(14), 4082–4098 (2016)
Sulem, C., Sulem, P.L.: Self-focusing and wave collapse. In: Sulem, C., Sulem, P.L. (eds.) The Nonlinear Schrödinger Equation. Applied Mathematical Sciences, vol. 139, Springer, New York (1999)
Wei, Y.: Comment on Fractional quantum mechanics and Fractional Schrödinger equation. Physical Review B: Condensed Matter and Materials Physics (1998–2015), vol. 93. American Physical Society (2016). https://doi.org/10.1103/physreve.93.066103ff.ffhal-01588657f
Zhu, S.: On the blow-up solutions for the nonlinear fractional Schrödinger equation. J. Differ. Equ. 261, 1506–1531 (2016)
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix
Appendix
In this section, one proves Lemma 2.5. Let \(\epsilon >0\) and \(1+\frac{b\chi _{b>0}}{N-2s}<p< p^*\). Take \((u_n)\) a bounded sequence of \(H^s_{rd}.\) Without loss of generality, one assumes that \((u_n)\) converges weakly to zero in \(H^s.\) The purpose is to prove that \(\Vert u_n\Vert _{L^{2p}(|x|^{b}\,\mathrm{{d}}x)}\rightarrow 0.\)
-
A.
First case \(-2s<b<0\). Using Hölder estimate, if \((q,q')\) satisfies \(q'|b|<N\), one gets
$$\begin{aligned} \int _{|x|\le \epsilon }|x|^{b}|u_n|^{2p}\,\mathrm{{d}}x\le & {} \Vert u_n\Vert _{2pq}^{2p}\Vert |x|^{b}\Vert _{q'}\\\le & {} C\Vert u_n\Vert _{2pq}^{2p}\int _0^\epsilon \frac{\mathrm{{d}}t}{t^{-q'b-N+1}}\\\le & {} C\Vert u_n\Vert _{2pq}^{2p}\epsilon ^{N+q'b}. \end{aligned}$$Now, since \(p<p^*\), one has \(N+\frac{Nb}{N-p(N-2s)}>0\). Taking \(q:=\frac{N}{p(N-2s)}\) and using Sobolev injections, it follows that there exists \(\mu >0\) such that
$$\begin{aligned} \int _{|x|\le \epsilon }|x|^{b}|u_n|^{2p}\,\mathrm{{d}}x\le & {} C\Vert u_n\Vert _{H^s}^{2p}\epsilon ^{N+q'b}\\\le & {} C\epsilon ^{N+\frac{Nb}{N-p(N-2s)}}\\\le & {} C\epsilon ^\mu . \end{aligned}$$On the other hand, by Sobolev injections,
$$\begin{aligned} \int _{|x|\ge \epsilon }|x|^{b}|u_n|^{2p}\,\mathrm{{d}}x \le \epsilon ^{b}\Vert u_n\Vert _{2p}^{2p}. \end{aligned}$$Since \(\epsilon \) is arbitrary, one obtains \(\int _{\mathbb R^N}|x|^{b}|u_n|^{2p}\,\mathrm{{d}}x\rightarrow 0\) as \(n\rightarrow \infty .\) The proof is complete.
-
B.
Second case \(b\ge 0\). Write, using Compact Sobolev injections
$$\begin{aligned} \int _{|x|\le \epsilon }|x|^{b}|u_n|^{2p}\,\mathrm{{d}}x\le C\Vert u_n\Vert _{2p}^{2p}\rightarrow 0. \end{aligned}$$Moreover, with Strauss inequality and Rellich Theorem,
$$\begin{aligned} \int _{\epsilon \le |x|\le \frac{1}{\epsilon }}|x|^{b}|u_n|^{2p}\,\mathrm{{d}}x\le C\Vert u_n\Vert _{L^\infty (\epsilon \le |x|\le \frac{1}{\epsilon })}^{2(p-1)}\int _{\epsilon \le |x|\le \frac{1}{\epsilon }}|u_n|^2\,\mathrm{{d}}x\rightarrow 0. \end{aligned}$$Now, with Strauss inequality
$$\begin{aligned} \int _{|x|\ge \frac{1}{\epsilon }}|x|^{b}|u_n|^{2p}\,\mathrm{{d}}x&=\int _{|x|\ge \frac{1}{\epsilon }}|x|^{b-(p-1)(N-2s)}(|x|^{\frac{N-2s}{2}}|u_n|)^{2(p-1)}|u_n|^2\,\mathrm{{d}}x\\\le & {} C\int _{|x|\ge \frac{1}{\epsilon }}|x|^{b-(p-1)(N-2s)}|u_n|^2\,\mathrm{{d}}x\\\le & {} C\epsilon ^{(p-1)(N-2s)-b}. \end{aligned}$$The proof is achieved because \({b-(p-1)(N-2s)}<0.\)
Rights and permissions
About this article
Cite this article
Saanouni, T., Alharbi, M.G. Fractional Choquard Equations with an Inhomogeneous Combined Non-linearity. Mediterr. J. Math. 19, 108 (2022). https://doi.org/10.1007/s00009-022-02023-4
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00009-022-02023-4