1 Introduction

As is well known, Krasnoselskii's fixed point theorem has a wide range of applications to nonlinear integral equations of mixed type (see [1]). It has also been extensively employed to address differential and functional differential equations. His theorem actually combines both the Banach contraction principle and the Schauder fixed point theorem, and is useful in establishing existence theorems for perturbed operator equations. Since then, there have appeared a large number of articles contributing generalizations or modifications of the Krasnoselskii fixed point theorem and their applications (see [2]-[21]).

The study of asymptotically nonexpansive map**s concerning the existence of fixed points have become attractive to the authors working in nonlinear analysis. Goebel and Kirk [22] introduced the concept of asymptotically nonexpansive map**s in Banach spaces and proved a theorem on the existence of fixed points for such map**s in uniformly convex Banach spaces. In 1971, Cain and Nashed [23] generalized to locally convex spaces a well known fixed point theorem of Krasnoselskii for a sum of contraction and compact map**s in Banach spaces. The class of asymptotically nonexpansive map**s includes properly the class of nonexpansive map**s as well as the class of contraction map**s. Recently, Vijayaraju [21] proved by using the same method some results concerning the existence of fixed points for a sum of nonexpansive and continuous map**s and also a sum of asymptotically nonexpansive and continuous map**s in locally convex spaces. Very recently, Agarwal et al. [1] proved some existence theorems of a fixed point for the sum of a weakly-strongly continuous map** and a nonexpansive map** on a Banach space and under Krasnoselskii-, Leray Schauder-, and Furi-Pera-type conditions.

Motivated and inspired by Agarwal et al. [1] and Vijayaraju [21], in this article we will prove some new generalized forms of the Krasnoselskii theorem on fixed points for the sum A + B of a weakly-strongly continuous map** and an asymptotically nonexpansive map** in Banach spaces. These results encompass a number of previously known generalizations of the theorem.

2 Preliminaries

Let M be a nonempty subset of a Banach space X and T : M → X be a map**. We say that T is weakly-strongly continuous if for each sequence {x n } in M which converges weakly to x in M, the sequence {Tx n } converges strongly to Tx. The map** T is nonexpansive if ||Tx -Ty|| ≤ ||x - y|| for all x, y ∈ M, and T is asymptotically nonexpansive (see [22]) if there exists a sequence {k n } with k n ≥ 1 for all n and limn→∞k n = 1 such that ||Tnx - Tny|| ≤ k n ||x - y|| for all n ≥ 1 and x, y ∈ M.

Definition 2.1. [21] If B and A map M into X, then B is called a uniformly asymptotically regular with respect to A if, for each ε > 0 there exists n0 ∈ ℕ, such that

for all n ≥ n0 and all x ∈ M.

Now, let us recall some definitions and results which will be needed in our further considerations. Let X be a Banach space, Ω(X) is the collection of all nonempty bounded subsets of X, and is the subset of Ω(X) consisting of all weak compact subsets of X. Let B r denote the closed ball in X countered at 0 with radius r > 0. In [24], De Blasi introduced the following map** ω : Ω(X) → [0, ∞) defined by

for all M ∈ Ω(X). For completeness, we recall some properties of ω(·) needed below (for the proofs we refer the reader to [24]).

Lemma 2.2. [24] Let M1 and M2 ∈ Ω(X), then we have

(i) If M1 ⊆ M2, then ω(M1) ≤ ω(M2).

(ii) ω(M1) = 0 if and only if M1 is relatively weakly compact.

(iii) , where is the weak closure of M1.

(iv) ω(λM1) = |λ|ω(M1) for all λ ∈ ℝ.

(v) ω(co(M1)) = w(M1).

(vi) ω(M1 + M2) ≤ ω(M1) + ω(M2).

(vii) If (M n )n≥1is a decreasing sequence of nonempty, bounded and weakly closed subsets of X with limn→∞ω(M n ) = 0, then and , i.e., is relatively weakly compact.

Throughout this article, a measure of weak noncompactness will be a map** ψ : Ω(X) → [0, ∞) which satisfies the assumptions (i)-(vii) cited in Lemma 2.2.

Definition 2.3. [25] Let M be a closed subset of X and I, T : M → M be two map**s. A map** T is said to be demiclosed at the zero, if for each sequence {x n } in M, the conditions x n → x0 weakly and Tx n → 0 strongly imply Tx0 = 0.

Lemma 2.4. [26]-[29] Let X be a uniformly convex Banach space, M be a nonempty closed convex subset of X, and let T : M → M be an asymptotically nonexpansive map** with F(T) ≠ ∅. Then I - T is demiclosed at zero, i.e., for each sequence {x n } in M, if {x n } converges weakly to q ∈ M and {(I - T)x n } converges strongly to 0, then (I - T)q = 0.

Definition 2.5. [1, 13] Let X be a Banach space and let ψ be a measure of weak noncompactness on X. A map** B : D(B) ⊆ X → X is said to be ψ-contractive if it maps bounded sets into bounded sets and there is a β ∈ [0, 1) such that ψ(B(S)) ≤ βψ(S) for all bounded sets S ⊆ D(B). The map** B : D(B) ⊆ X → X is said to be ψ-condensing if it maps bounded sets into bounded sets and ψ(B(S)) < ψ(S) whenever S is a bounded subset of D(B) such that ψ(S) > 0.

Let be a nonlinear operator from into X. In the next section, we will use the following two conditions:

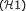
If (x n )n∈ℕis a weakly convergent sequence in , then has a strongly convergent subsequence in X.

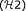
If (x n )n∈ℕis a weakly convergent sequence in , then has a weakly convergent subsequence in X.

Remark 2.6. 1. Operators satisfying 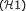or 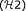are not necessarily weakly continuous (see [12, 19, 30]).

  1. 2.

    Every w-contractive map** satisfies .

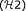  2. 3.

    A map** 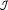satisfies 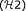if and only if it maps relatively weakly compact sets into relatively weakly compact ones (use the Eberlein-Š mulian theorem [31]).

  3. 4.

    A map** 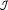satisfies 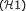if and only if it maps relatively weakly compact sets into relatively compact ones.

  4. 5.

    The condition 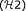holds true for every bounded linear operator.

The following fixed point theorems are crucial for our purposes.

Lemma 2.7. [12] Let M be a nonempty closed bounded convex subset of a Banach space X. Suppose that A : M → X and B : X → X satisfying:

(i) A is continuous, AM is relatively weakly compact and A satisfies ,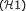

(ii) B is a strict contraction satisfying ,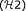

(iii) Ax + By ∈ M for all x, y ∈ M.

Then, there is an x ∈ M such that Ax + Bx = x.

Lemma 2.8. [20] Let M be a nonempty closed bounded convex subset of a Banach space X. Suppose that A : M → X and B : M → X are sequentially weakly continuous such that:

(i) AM is relatively weakly compact,

(ii) B is a strict contraction,

(iii) Ax + By ∈ M for all x, y ∈ M.

Then, there is an x ∈ M such that Ax + Bx = x.

Lemma 2.9. [1] Let X be a Banach space and let ψ be measure of weak noncompactness on X. Let Q and C be closed, bounded, convex subset of X with Q ⊆ C. In addition, let U be a weakly open subset of Q with 0 ∈ U, and a weakly sequentially continuous and ψ-condensing map**. Then either

(2.1)

or

(2.2)

here ∂ Q U is the weak boundary of U in Q.

Lemma 2.10. [1] Let X be a Banach space and B : X → X a k-Lipschitzian map**, that is

In addition, suppose that B verifies 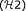. Then for each bounded subset S of X, we have ψ(BS) ≤ kψ(S);

here,ψ is the De Blasi measure of weak noncompactness.

Lemma 2.11. [15, 32] Let X be a Banach space with C ⊆ X closed and convex. Assume U is a relatively open subset of C with 0 ∈ U, bounded and a condensing map**. Then, either F has a fixed point in or there is a point u ∈ ∂U and λ ∈ (0,1) with u = λF(u); here and ∂U denote the closure of U in C and the boundary of U in C, respectively.

Lemma 2.12. [15, 32] Let X be a Banach space and Q a closed convex bounded subset of X with 0 ∈ Q. In addition, assume F : Q → X a condensing map** with if is a sequence in ∂Q× [0, 1] converging to (x, λ) with X = λF(x) and 0 < λ < 1, then λ j F (x j ) ∈ Q for j sufficiently large, holding. Then F has a fixed point.

3 Main results

Now, we are ready to state and prove the main result of this section.

Theorem 3.1. Let M be a nonempty bounded closed convex subset of a Banach space X. Let A : M → X and B : M → M satisfy the following:

(i) A is weakly-strongly continuous, and AM is relatively weakly compact,

(ii) B is an asymptotically nonexpansive map** with a sequence (k n ) ⊂ [1, ∞) satisfying ,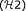

(iii) if (x n ) is a sequence of M such that ((I - B)x n ) is weakly convergent, then the sequence (x n ) has a weakly convergent subsequence,

(iv) I - B is demiclosed,

(v) Bnx + Ay ∈ M for all x, y ∈ M and n = 1, 2,...,

(vi) B is uniformly asymptotically regular with respect to A.

Then, there is an x ∈ M such that Ax + Bx = x.

Proof. Suppose first that 0 ∈ M and let for all n ∈ ℕ. By hypothesis (v), we have

Since B is asymptotically nonexpansive, it follows that

(3.1)

Hence, a n Bn is contraction on M. Therefore, by Lemma 2.7, there is an x n ∈ M such that

(3.2)

for all n ∈ ℕ. This implies that

(3.3)

since a n → 1 as n → ∞ and M is bounded and Bnx + Ay ∈ M for all x, y ∈ M. Since B is uniformly asymptotically regular with respect to A, it follows that

(3.4)

From (3.3) and (3.4), we obtain

(3.5)

Now, it is noted that

(3.6)

Using (3.3) and (3.5) in (3.6), we get

(3.7)

Using the fact that AM is weakly compact and passing eventually to a subsequence, we may assume that {Ax n } converges weakly to some y ∈ M. By (3.7), we have

(3.8)

By hypothesis (iii), the sequence {x n } has a subsequence which converges weakly to some x ∈ M. Since A is weakly-strongly continuous, converges strongly to Ax.

Hence, we observe that

(3.9)

Hence, by the demiclosedness of I - B, we have Ax + Bx = x.

To complete the proof, it remains to consider the case 0 ∉ M. In such a case, let us fix any element x0 ∈ M and let M0 = {x - x0, x ∈ M }. Define two map**s A0 : M0 → X and B0 : M0 → M by and , for x ∈ M. By the result of the first case for A0 and B0, we have an x ∈ M such that A0(x - x0) + B0(x - x0) = x - x0. Hence Ax + Bx = x. □

Corollary 3.2. Let M be a nonempty bounded closed convex subset of a uniformly convex Banach space X. Let A : M → X and B : M → M satisfy the following:

(i) A is weakly-strongly continuous,

(ii) B is an asymptotically nonexpansive map** with a sequence (k n ) ⊂ [1, ∞),

(iii) Bnx + Ay ∈ M for all x, y ∈ M, and n = 1, 2,...,

(iv) B is uniformly asymptotically regular with respect to A.

Then, there is an x ∈ M such that Ax + Bx = x.

Our next result is the following:

Theorem 3.3. Let M be a nonempty bounded closed convex subset of a Banach space X. Suppose that A : M → X and B : M → M are two weakly sequentially continuous map**s that satisfy the following:

(i) AM is relatively weakly compact,

(ii) B is an asymptotically nonexpansive map** with a sequence (k n ) ⊂ [1, ∞),

(iii) if (x n ) is a sequence of M such that ((I - B)x n ) is weakly convergent, then the sequence (x n ) has a weakly convergent subsequence,

(iv) Bnx + Ay ∈ M for all x, y ∈ M, and n = 1, 2,...,

(v) B is uniformly asymptotically regular with respect to A.

Then, there is an x ∈ M such that Ax + Bx = x.

Proof. Without loss of generality, we may assume that 0 ∈ M. Let for all n ∈ ℕ. By hypothesis (iv), we have

Since B is asymptotically nonexpansive, it follows that

(3.10)

Hence, a n Bn is a contraction on M. By Lemma 2.8, there is a x n ∈ M such that

(3.11)

for all n ∈ ℕ. This implies that

(3.12)

Since B is uniformly asymptotically regular with respect to A, it follows that

(3.13)

From (3.12) and (3.13), we obtain

(3.14)

Now, it is noted that

(3.15)

Using (3.12) and (3.14) in (3.15), we get

(3.16)

Using the fact that AM is weakly compact and passing eventually to a subsequence, we may assume that {Ax n } converges weakly to some y ∈ M. Hence, by (3.16)

(3.17)

By hypothesis (iii), the sequence {x n } has a subsequence which converges weakly to some x ∈ M. Since A and B are weakly sequentially continuous, converges weakly to Ax, and converges weakly to Bx. Hence, Ax + Bx = x. □

Theorem 3.4. Let Q and C be closed bounded convex subset of a Banach space X with Q ⊆ C. In addition, let U be a weakly open subset of Q with 0 ∈ U, and B : X → X are two weakly sequentially continuous map**s satisfying the following:

(i) is a relatively weakly compact,

(ii) B is an asymptotically nonexpansive map** with a sequence (k n ) ⊂ [1, ∞),

(iii) if (x n ) is a sequence of M such that ((I - B)x n ) is weakly convergent, then the sequence (x n ) has a weakly convergent subsequence,

(iv) Bnx + Ay ∈ C for all , and n = 1, 2,...,

(v) B is uniformly asymptotically regular with respect to A.

Then, either

(3.18)

or

(3.19)

here, ∂ Q U is the weak boundary of U in Q.

Proof. Let for all n ∈ ℕ. We first show that the map** F n = a n A+a n Bn is ψ-contractive with constant a n . To see that, let S be a bounded subset of . Using the homogeneity and the subadditivity of the De Blasi measure of weak noncompactness, we obtain

Kee** in mind that A is weakly compact and using Lemma 2.10, we deduce that

This proves that F n is ψ-contractive with constant a n . Moreover, taking into account that 0 ∈ U and using assumption (iv), we infer that F n map into C. Next, we suppose that (3.19) does not occur, and F n does not have a fixed point on ∂ Q U (otherwise we are finished since (3.18) occurs). If there exists a u ∈ ∂ Q U, and λ ∈ (0, 1) with u = λF n u then u = λa n Au + λa n Bnu. It is impossible since λa n ∈ (0, 1). By Lemma 2.9, there exists such that

for all n ∈ ℕ. This implies that

(3.20)

Since B is uniformly asymptotically regular with respect to A, it follows that

(3.21)

From (3.20) and (3.21), we obtain

(3.22)

Now, it is noted that

(3.23)

Using (3.20) and (3.22) in (3.23), we get

(3.24)

Since AM is weakly compact and passing eventually to a subsequence, we may assume that {Ax n } converges weakly to some . Thus, we have

(3.25)

By hypothesis (iii), the sequence {x n } has a subsequence which converges weakly to some . Since A and B are weakly sequentially continuous, converges weakly to Ax, and converges weakly to Bx. Hence, Ax + Bx = x. □

Theorem 3.5. Let U be a bounded open convex set in a Banach space X with 0 ∈ U. Suppose and B : X → X are continuous map**s satisfying the following:

(i) is compact, and A is weakly-strongly continuous,

(ii) B is an asymptotically nonexpansive map** with a sequence (k n ) ⊂ [1, ∞), and I - B is demiclosed,

(iii) if (x n ) is a sequence of such that ((I - B)x n ) is weakly convergent, then the sequence (x n ) has a weakly convergent subsequence,

(iv) B is uniformly asymptotically regular with respect to A.

Then, either

(3.26)

or

(3.27)

Proof. Suppose (3.27) does not occur and let for all n ∈ ℕ. The map** F n := a n A + a n Bn is the sum of a compact and a strict contraction. This implies that F n is a condensing map** (see [13]). By Lemma 2.11, we deduce that there is an such that

for all n ∈ ℕ. This implies that

(3.28)

Since B is uniformly asymptotically regular with respect to A, it follows that

(3.29)

From (3.28) and (3.29), we obtain

(3.30)

Now, it is noted that

(3.31)

Using (3.28) and (3.30) in (3.31), we get

(3.32)

Since AM is weakly compact and passing eventually to a subsequence, we may assume that {Ax n } converges weakly to some . This implies that

(3.33)

By hypothesis (iii), the sequence {x n } has a subsequence which converges weakly to some . Since A is weakly-strongly continuous, converges strongly to Ax.

Consequently

(3.34)

By the demiclosedness of I - B, we have Ax + Bx = x. □

Corollary 3.6. Let U be a bounded open convex set in a uniformly convex Banach space X with 0 ∈ U. Suppose and B : X → X are continuous map**s satisfying the following.

(i) is compact, and A is weakly-strongly continuous,

(ii) B is an asymptotically nonexpansive map** with a sequence (k n ) ⊂ [1, ∞),

(iii) B is uniformly asymptotically regular with respect to A.

Then, either

(3.35)

or

(3.36)

Theorem 3.7. Let Q be a closed convex bounded set in a Banach space X with 0 ∈ Q. Suppose A : Q → X and B : X → X are continuous map**s satisfying the following:

(i) A(Q) is compact, and A is weakly-strongly continuous,

(ii) B is an asymptotically nonexpansive map** with a sequence (k n ) ⊂ [1, ∞), and I - B is demiclosed,

(iii) if (x n ) is a sequence of such that ((I - B)x n ) is weakly convergent, then the sequence (x n ) has a weakly convergent subsequence,

(iv) if is a sequence of ∂Q × [0, 1] converging to (x, λ) with X = λAx + λBnx and 0 ≤ λ < 1, then λ j Ax j + λ j Bnx j ∈ Q for j sufficiently large,

(v) B is uniformly asymptotically regular with respect to A.

Then, A + B has a fixed point in Q.

Proof. We first define F n := a n A + a n Bn, where for all n ∈ ℕ. Since F n is the sum of a compact map** and a strict contraction map**, it follows that F n is a condensing map**. For any let fixed n, we have is a sequence of ∂Q × [0, 1] converging to (y, λ) with y = λF n (y) and 0 ≤ λ < 1. Then y = a n λAy + a n λBny. From assumption (iv), it follows that a n λ j Ay j + a n λ j Bny j ∈ Q for j sufficiently large. Applying Lemma 2.12 to F n , we deduce that there is an x n ∈ Q such that

As in Theorem 3.5 this implies that

(3.37)

By hypothesis (iii), the sequence {x n } has a subsequence which converges weakly to some x ∈ Q. Since A is weakly-strongly continuous, converges strongly to Ax.

It follows that

(3.38)

Hence, by the demiclosedness of I - B, we have Ax + Bx = x. □

Corollary 3.8. Let Q be a closed convex bounded set in a uniformly convex Banach space X with 0 ∈ Q. Suppose A : Q → X and B : X → X are continuous map**s satisfying the following:

(i) A(Q) is compact and A is weakly-strongly continuous,

(ii) B is an asymptotically nonexpansive map** with a sequence (k n ) ⊂ [1, ∞),

(iii) if is a sequence of ∂Q × [0, 1] converging to (x, λ) with X = λAx + λBnx and 0 ≤ λ < 1, then λ j Ax j + λ j Bnx j ∈ Q for j sufficiently large,

(iv) B is uniformly asymptotically regular with respect to A.

Then, A + B has a fixed point in Q.