Multivariable control of the undesirable axial vibrations of the horizontal drill string

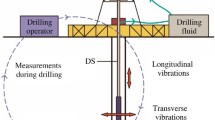
Abstract

Although new technologies in energy area such as the wind turbines, solar systems and etcetera are introduced as some alternatives for the oil and gas energy sources, the latter ones are still the most important sources of energy in the world. So, researches have been continued to investigate in these fields to optimize all the steps from the extraction to producing the new products. After our previous article about the horizontal drilling dynamics, modeling the system with mode summation method, modes' convergence analysis, evaluation of the stability situation of the system with changing the parameters and identifying the instability; in this article designing an effective controller for that system is on agenda. Preventing the instability condition can lead to the increasing of the extraction and life time of the components. For the fourth mode in which the instability is observed, the controller is designed and this controller is examined in the case of five modes. It is shown that the controller performs efficiently in suppression of the undesirable axial vibrations of the horizontal drilling process. In addition, through a parametric study of the dynamic system, the number of inputs is decreased and the magnitude of them is reduced to have a more suitable design for using in the realistic applications.

Appendix A. Designing GCCF control method

For designing the controller, method of the pole placement is used; in which, more than one input exists to control the system. So, approaches such as the Bass-Gura and Ackerman methods that are used for the problem with one input are not suitable and it is necessary to use the Generalized Controllability Canonical Form (GCCF) control method.

If the configuration of the equations is in the state space form of Eq. (A-1), equations can be changed to a new form with the suitable transformation into Eq. (A-2). The parameters in new form are described in Eqs. (A-1) and (A-4).

$$ \dot{\underline {x} } = A\underline {x} + Bu $$

(A-1)

$$ \dot{\underline {z} } = A_{G} \underline {z} + B_{G} v $$

(A-2)

$$ A_{G} = \left[ {\begin{array}{*{20}c} {A_{1} } & 0 & \cdots & 0 \\ 0 & {A_{2} } & \ddots & \vdots \\ \vdots & \ddots & \ddots & 0 \\ 0 & \cdots & 0 & {A_{r} } \\ \end{array} } \right]_{n \times n} $$

(A-3)

$$ B_{G} = \left[ {\begin{array}{*{20}c} {\left\{ {b_{1} } \right\}} & 0 & \cdots & 0 \\ 0 & {\left\{ {b_{2} } \right\}} & \ddots & \vdots \\ \vdots & \ddots & \ddots & 0 \\ 0 & \cdots & 0 & {\left\{ {b_{r} } \right\}} \\ \end{array} } \right]_{n \times r} $$

(A-4)

r is the number of inputs. For each matrix, control invariant is used as mentioned in Eqs. (A-5)-(A-8), as:

$$ \mathop \sum \limits_{i = 1}^{r} \gamma_{i} = n $$

(A-5)

$$ \gamma_{1} \ge \gamma_{2} \ge \gamma_{3} \ge \ldots \ge \gamma_{r} \ge 0 $$

(A-6)

$$ \left[ {A_{i} } \right] = \left[ {\begin{array}{*{20}c} 0 & 1 & \cdots & 0 \\ 0 & 0 & \ddots & \vdots \\ \vdots & \ddots & \ddots & 1 \\ 0 & \cdots & 0 & 0 \\ \end{array} } \right]_{{\gamma_{i} \times \gamma_{i} }} $$

(A-7)

$$ \left\{ {b_{i} } \right\} = \left[ {\begin{array}{*{20}c} 0 \\ 0 \\ \vdots \\ 1 \\ \end{array} } \right]_{{\gamma_{i} \times 1}} $$

(A-8)

To transfer from Eq. (A-1) into Eq. (A-2), there are three steps as follows:

$$ 1) \underline {x} = T\underline {z} \to \dot{\underline {z} } = \left( {T^{ - 1} {\text{AT}}} \right)\underline {z} + \left( {T^{ - 1} B} \right)u $$

(A-9)

$$ 2) u = F\omega \to \dot{\underline {z} } = \left( {T^{ - 1} {\text{AT}}} \right)\underline {z} + \left( {T^{ - 1} {\text{BF}}} \right)u $$

(A-10)

$$ 3) \omega = v - Hz \to \dot{\underline {z} } = \left( {T^{ - 1} {\text{AT}} - T^{ - 1} {\text{BFH}}} \right)\underline {z} + \left( {T^{ - 1} {\text{BF}}} \right)v = A_{G} \underline {z} + B_{G} v $$

(A-11)

So, the aim is to find H, T and F. Controllability matrix is defined by Eq. (A-12) and if it has n independent columns, the system has the ability to be controlled.

$$ \overline{{M_{c} }} = \left[ {\begin{array}{*{20}c} B & {AB} & {A^{2} B} \\ \end{array} \begin{array}{*{20}c} \ldots & {A^{n - r} B} \\ \end{array} } \right]_{{n \times \left( {n - r + 1} \right)r}} $$

(A-12)

Combination of the independent vectors is set in Eq. (A-13) as:

$$ \widehat{{M_{c} }} = \left[ {\begin{array}{*{20}c} {b_{1} } & {Ab_{1} } & \ldots & {A^{{\gamma_{1} - 1}} b_{1} } & \vdots & {b_{2} } & {Ab_{2} } & \ldots & {A^{{\gamma_{2} - 1}} b_{2} } & \vdots & \ldots & \vdots & {b_{r} } & {Ab_{r} } & \ldots & {A^{{\gamma_{r} - 1}} b_{r} } \\ \end{array} } \right] $$

(A-13)

If the inverse of the above matrices is obtained, and end row of the block for each control invariant is extracted and named $e_{1}$ to $e_{r}$, then the inverse matrix of T is achieved as:

$$ T^{ - 1} = \left[ {\begin{array}{*{20}c} {e_{1} } \\ {e_{1} A} \\ \vdots \\ {e_{1} A^{{\gamma_{1} - 1}} } \\ \vdots \\ {e_{r} } \\ {e_{r} A} \\ \vdots \\ {e_{r} A^{{\gamma_{r} - 1}} } \\ \end{array} } \right] $$

(A-14)

With specifying T, the matrices F and H are calculated as below:

$$ F = (B_{G}^{T} T^{ - 1} B)^{ - 1} $$

(A-15)

$$ H = B_{G}^{T} \left( {T^{ - 1} AT - A_{G} } \right) $$

(A-16)

At the end, with feedback, the system that the invariants are transformed to the zero, is changed to the desired matrix $A_{d}$. The structure of $A_{d}$ is similar to $A_{G}$ but the sub matrix ($A_{i}$) must be changed to the desired dynamics equations.

$$ v = - {\Gamma z} \to \dot{\underline {z} } = \left( {A_{G} - B_{G} {\Gamma }} \right)\underline {z} = A_{d} \underline {z} $$

(A-17)

$$ {\Gamma } = B_{G}^{T} \left( {A_{G} - A_{d} } \right) $$

(A-18)

For simplifying the above transformations, all of them can be collected and rewritten through new feedback ($K$) as:

$$K=F(\Gamma +H){T}^{-1}$$

(A-19)

According to Eqs. (23)-(28) and Eq. (31), state matrix can be obtained as below:

$$\left[\begin{array}{c}\dot{{x}_{3}}\\ \dot{{x}_{4}}\\ \begin{array}{c}\dot{{x}_{5}}\\ \dot{{x}_{6}}\\ \begin{array}{c}\dot{{x}_{7}}\\ \begin{array}{c}\dot{{x}_{8}}\\ \dot{e}\end{array}\end{array}\end{array}\end{array}\right]=\left[\begin{array}{ccccccc}0& 1& 0& 0& 0& 0& 0\\ -{{\omega }_{2}}^{2}& 0& 0& 0& 0& 0& 0\\ 0& 0& 0& 1& 0& 0& 0\\ 0& 0& -{{\omega }_{3}}^{2}& 0& 1& 0& 0\\ 0& 0& 0& 0& 0& 1& 0\\ 0& 0& 0& 0& -{{\omega }_{4}}^{2}& 0& 0\\ 0& 0& 0& 0& 0& 0& 0\end{array}\right]\left[\begin{array}{c}{x}_{3}\\ {x}_{4}\\ {x}_{5}\\ {x}_{6}\\ {x}_{7}\\ {x}_{8}\\ e\end{array}\right]+\left[\begin{array}{cccc}0& 0& 0& 0\\ 1& 0& 0& 0\\ 0& 0& 0& 0\\ 0& 1& 0& 0\\ 0& 0& 0& 0\\ 0& 0& 1& 0\\ 0& 0& 0& 1\end{array}\right]\left[\begin{array}{c}{u}_{1}\\ {u}_{2}\\ {u}_{3}\\ {u}_{4}\end{array}\right]$$

(A-20)

$$ A = \left[ {\begin{array}{*{20}c} 0 & 1 & 0 & 0 & 0 & 0 & 0 \\ { - \omega_{2}^{2} } & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & 0 & 0 & 0 \\ 0 & 0 & { - \omega_{3}^{2} } & 0 & 1 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 0 & { - \omega_{4}^{2} } & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ \end{array} } \right] $$

(A-21)

$$ B = \left[ {\begin{array}{*{20}c} 1 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 \\ 0 & 0 & 0 & 0 \\ 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 \\ \end{array} } \right] $$

(A-22)

$$ \widehat{{M_{c} }} = \left[ {\begin{array}{*{20}c} 0 & 1 & 0 & 0 & 0 & 0 & 0 \\ 1 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & 0 & 0 & 0 \\ 0 & 0 & 1 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & 0 & { - \omega_{4}^{2} } & 0 \\ 0 & 0 & 0 & 0 & 1 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 1 \\ \end{array} } \right] $$

(A-23)

$$ \gamma_{1} = 2,\gamma_{2} = 2,\gamma_{3} = 2,\gamma_{4} = 1 $$

(A-24)

According to the control theory, the T matrix is achieved as:

$$ \widehat{{M_{c} }}^{ - 1} = \left[ {\begin{array}{*{20}c} 0 & 1 & 0 & 0 & 0 & 0 & 0 \\ 1 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & 0 & 0 & 0 \\ 0 & 0 & 1 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & 0 & 1 & 0 \\ 0 & 0 & 0 & 0 & {\frac{1}{{ - \omega_{4}^{2} }}} & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 1 \\ \end{array} } \right] $$

(A-25)

$$ \left[ {\begin{array}{*{20}c} {e_{1} } \\ {e_{2} } \\ {e_{3} } \\ {e_{4} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} 1 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 1 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & {\frac{1}{{ - \omega_{4}^{2} }}} & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 1 \\ \end{array} } \right] $$

(A-26)

$$ T^{ - 1} = \left[ {\begin{array}{*{20}c} 1 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 1 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & {\frac{1}{{ - \omega_{4}^{2} }}} & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 1 \\ \end{array} } \right] $$

(A-27)

$B_{G}$ is equal to $B$ because it is in standard form and $A_{G}$ can be written as below:

$$ A_{G} = \left[ {\begin{array}{*{20}c} {\left[ {\begin{array}{*{20}c} 0 & 1 \\ 0 & 0 \\ \end{array} } \right]} & 0 & \cdots & 0 \\ 0 & {\left[ {\begin{array}{*{20}c} 0 & 1 \\ 0 & 0 \\ \end{array} } \right]} & {} & \vdots \\ \vdots & {} & {\left[ {\begin{array}{*{20}c} 0 & 1 \\ 0 & 0 \\ \end{array} } \right]} & 0 \\ 0 & \cdots & 0 & 0 \\ \end{array} } \right] $$

(A-28)

The form of $A_{d}$ is similar to $A_{G}$, with this difference that instead of the zero poles, it has desirable poles. For this target, Butterworth method is used to determine these poles as:

$$ n = 1 \to z_{1} = - 1 \to s + 1 = 0 \to A_{4} = \left[ { - 1} \right] $$

(A-29)

$$ n = 2 \to z_{1} = \frac{\sqrt 2 }{2}\left( { - 1 \pm j} \right) \to s^{2} + \sqrt 2 z + 1 = 0 \to A_{1 \to 3} = \left[ {\begin{array}{*{20}c} 0 & 1 \\ { - \sqrt 2 } & { - 1} \\ \end{array} } \right] $$

(A-30)

So, the $A_{d}$ matrix is written as below:

$$ A_{d} = \left[ {\begin{array}{*{20}c} {\left[ {\begin{array}{*{20}c} 0 & 1 \\ { - \sqrt 2 } & { - 1} \\ \end{array} } \right]} & 0 & \cdots & 0 \\ 0 & {\left[ {\begin{array}{*{20}c} 0 & 1 \\ { - \sqrt 2 } & { - 1} \\ \end{array} } \right]} & {} & \vdots \\ \vdots & {} & {\left[ {\begin{array}{*{20}c} 0 & 1 \\ { - \sqrt 2 } & { - 1} \\ \end{array} } \right]} & 0 \\ 0 & \cdots & 0 & { - 1} \\ \end{array} } \right] $$

(A-31)

$$ F = \left[ {\begin{array}{*{20}c} 1 & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 \\ 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 1 \\ \end{array} } \right] $$

(A-32)

$$ H = \left[ {\begin{array}{*{20}c} { - \omega_{2}^{2} } & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & { - \omega_{3}^{2} } & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & { - \omega_{4}^{2} } & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ \end{array} } \right] $$

(A-33)

$$ {\Gamma } = \left[ {\begin{array}{*{20}c} {\sqrt 2 } & 1 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & {\sqrt 2 } & 1 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & {\sqrt 2 } & 1 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 1 \\ \end{array} } \right] $$

(A-34)

$$ K = \left[ {\begin{array}{*{20}c} { - \omega_{2}^{2} } & 1 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & { - \omega_{3}^{2} } & 1 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & {1.000} & 1 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 1 \\ \end{array} } \right] $$

(A-35)