Numerical investigation of Auger current density in a InGaN/GaN multiple quantum well solar cell under hydrostatic pressure

Abstract

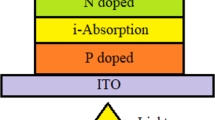
This study used a numerical model to analyze the Auger current in c-plane InGaN/GaN multiple-quantum well solar cells (MQWSC) under hydrostatic pressure. Finite difference techniques were employed to acquire energy eigenvalues and their corresponding eigenfunctions of ${\text{InGaN/GaN}}$ MQWSC. Besides, the hole eigenstates were calculated via a $6 \times 6$ k.p method under applied hydrostatic pressure. Our calculations demonstrated that the hole-hole-electron (CHHS) and electron–electron-hole (CCCH) Auger coefficients had the largest contribution to the total Auger coefficient (i.e., 76 and 20%, respectively). Also, it was found that a pressure change of up to 10 GPa increases the carrier density in the quantum well and barriers. Based on the result, such a change could decrease the exciton binding energy, raise the quantum confinement of carriers, and decrease the Auger current in the multiple-quantum well and barrier regions. The solar cell’s performance is better when the Auger current is lower; thus, hydrostatic pressure plays a positive role in the performance of solar cells.

Appendices

Appendix A: Numerical method

The discretization of Schrodinger and Poisson equations has been performed using the finite difference method (FDM). A centered second-order scheme is used for this purpose. Therefore, a continuous term such as $\frac{d}{dz}\left( {f\frac{d\psi }{{dz}}} \right)$ is discretized according to [58]:

$$ \frac{d}{dz}\left( {f\frac{d\psi }{{dz}}} \right) = \frac{{\frac{{(f_{i + 1} + f_i )}}{2} \times \frac{{(\psi_{i + 1} - \psi_i )}}{\Delta z}}}{\Delta z} $$

(11)

The Schrodinger equation becomes: $H\psi_i = E\psi_i$. The nonzero elements of the matrix H are:

$$ H(i,j) = \left\{ \begin{gathered} \frac{\hbar^2 }{{2m_0 \Delta z^2 }}\frac{1}{2}\left( {\frac{1}{m^* \left( i \right)} + \frac{1}{{m^* \left( {i - 1} \right)}}} \right)\begin{array}{*{20}c} {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\,} & {\,} \\ \end{array} } & {\,} \\ \end{array} } & {\,} \\ \end{array} } & {\begin{array}{*{20}c} {if} & {j = i + 1} \\ \end{array} } \\ \end{array} \hfill \\ \frac{\hbar^2 }{{2m_0 \Delta z^2 }}\left( {\frac{1}{2}\left( {\frac{1}{m^* \left( i \right)} + \frac{1}{{m^* \left( {i - 1} \right)}}} \right) + \frac{1}{2}\left( {\frac{1}{m^* \left( i \right)} + \frac{1}{{m^* \left( {i + 1} \right)}}} \right)} \right) \hfill \\ - \frac{\hbar^2 }{{2m_0 \Delta z^2 }}\frac{1}{2}\left( {\frac{1}{m^* \left( i \right)} + \frac{1}{{m^* \left( {i + 1} \right)}}} \right)\begin{array}{*{20}c} {\begin{array}{*{20}c} {\,} & {\,} \\ \end{array} } & {\,} & {\,} & {j = i + 1} \\ \end{array} \hfill \\ \end{gathered} \right. + E_c (i)\begin{array}{*{20}c} {\,} & {j = i} \\ \end{array} $$

(12)

It is straightforward to obtain the matrix system related to the Poisson equation.

The above eigenvalues system and linear system are coupled and should be solved using an iterative method. The convergence is obtained when the difference on the Fermi level associated with two consecutive iterations is smaller than $10^{ - 4} eV$. The boundary conditions related to the Schrodinger equation are:

$$ \psi_n (z = 0) = \psi_n (z = L) = 0\begin{array}{*{20}c} {\,} & {\,} \\ \end{array} $$

(13)

where L is the total height of the structure. The boundary conditions related to the Poisson equation are:

$$ \left. {\frac{d(V_H + V_P )}{{dz}}} \right|_{z = 0} = \left. {\frac{d(V_H + V_P )}{{dz}}} \right|_{z = L} = 0 $$

(14)

The details of the self-consistent solution of the Schrodinger–Poisson equation are as follows:

• 1. Consider the optional value for $n_{2D}$.

• 2. Solve the Poisson equation.

• 3. Solve the Schrodinger equation and obtain the wave functions and their energy subbands.

• 4. Using the following equations and Eq. 5, the electron density and the Fermi energy are obtained as follows.

  $$ E_F = E_0 + {{(\pi \hbar^2 n_{2D} )} / {m^* }} $$

  (15)
  $$ E_0 = {{(9\pi \hbar^2 e^2 n_{2D} )} / {(8\varepsilon_0 \sqrt {8m^* } \varepsilon_{GaN} )}} $$

  (16)

• 1.5. If it is $E_{F\left( n \right)} - E_{F\left( {n - 1} \right)} < 10^{ - 4} eV$, the self-consistent program will end; otherwise,$E_{F\left( n \right)} - E_{F\left( {n - 1} \right)} > 10^{ - 4} eV$. Put new ${n}_{2D}$ in Schrodinger’s equation and continue the program until the condition $E_{F\left( n \right)} - E_{F\left( {n - 1} \right)} < 10^{ - 4} eV$ is established.

The same grid mesh is used for Poisson and Schrödinger’s equation during the calculations. For mesh refinement in the numerical calculation written in the MATLAB software, commands ‘initmesh’ and ‘refinemesh’ are performed as follows in different regions of structure InGaN/GaN.

fem. mesh = initmesh (fem,'hmax',[0.05e-9],'hmaxfact',1,'hgrad',1.3, 'zscale',1.0);

fem. mesh = refinemesh (fem, ‘mcase’,0);

where there are FEM commands in solving wave functions, hmax is the maximum edge size (= 0.05e-9m), hgrad is the mesh growth rate (= 1.3), and zscale is a region to be refined.

Appendix B: Electric fields

Assuming no free charge, electric displacements at the interface between $\left( j \right)$ th and $\left( {j + 1} \right)$ th layers and between $\left( {j + 1} \right)$ th and $\left( {j + 2} \right)$ th layers can be expressed as follows [20]:

$$ \varepsilon_{j + 1} \varepsilon_0 F_{j + 1} = \varepsilon_j \varepsilon_0 F_j + P_j - P_{j + 1} $$

(17)

$$ \varepsilon_{j + 2} \varepsilon_0 F_{j + 2} = \varepsilon_{j + 1} \varepsilon_0 F_{j + 1} + P_{j + 1} - P_{j + 2} $$

(18)

Substituting from Eq. (17) gives an expression for Eq. (18):

$$ \varepsilon_{j + 2} \varepsilon_0 F_{j + 2} = \varepsilon_j \varepsilon_0 F_j + P_j - P_{j + 2} $$

(19)

where $\varepsilon_j$ denotes the dielectric constants and $P_j$ is the polarization in jth layer. In the following, the field in any layer is related to the field in a particular layer as:

$$ F_k = \frac{1}{\varepsilon_k \varepsilon_0 }\left( {\varepsilon_j \varepsilon_0 F_j + P_j - P_k } \right)\begin{array}{*{20}c} {\,} & {k = j + 2} \\ \end{array} $$

(20)

We focus on the case where there is no voltage difference across MQW either because thermodynamic equilibrium prevails or an applied field exists that compensates for any built-in field. The condition in which the volt value dropped across MQW is zero is as follows:

$$ \sum_k {L_k F_k } = L_j F_j + \sum_{k \ne j} {L_k F_k } $$

(21)

Here, $L_k$ and $L_j$ are the kth and jth layer's thickness. Substituting from Eq. (19) gives an expression for electric field in any layer:

$$ F_j = \frac{{\sum_{k \ne j} {\left( {P_k - P_j } \right)\left( {{{L_k } / {\varepsilon_k }}} \right)} }}{{\varepsilon_k \varepsilon_0 \sum_k {\left( {{{L_k } / {\varepsilon_k }}} \right)} }} $$

(22)

In the case of an MQW with only two types of layers ($L_w$ and $L_b$), the fields are:

$$ F_w = \frac{{\left( {P_b - P_w } \right)L_b }}{{\varepsilon_0 \left( {\varepsilon_w L_w + \varepsilon_b L_b } \right)}},\;F_b = \frac{{\left( {P_w - P_b } \right)L_w }}{{\varepsilon_0 \left( {\varepsilon_w L_w + \varepsilon_b L_b } \right)}} = - F_w \frac{L_w }{{L_b }} $$

(23)

where the difference in polarization density is equal to surface polarization density in InGaN/GaN, which is calculated as follows [42, 59]:

$$ \sigma_b = |P_b - P_w | = |P_{In_m Ga_{(1 - m)} N}^{PZ} + P_{In_m Ga_{(1 - m)} N}^{SP} - P_{GaN}^{SP} - P_{GaN}^{PZ} | $$

(24)

where

$$ P_{GaN}^{PZ} = - 0.918 \in + 9.541 \in^2 $$

(25)

$$ P_{InN}^{PZ} = - 1.373 \in + 7.559 \in^2 $$

(26)

$$ P_{(In_m Ga_{(1 - m)} N)}^{SP} = 0.042m - 0.034(1 - m) + 0.038m(1 - m) $$

(27)

$$ P_{In_m Ga_{(1 - m)} N}^{PZ} = mP_{InN}^{PZ} + (1 - m)P_{GaN}^{PZ} $$

(29)

Here, $\in$ is the basal strain whose relation is presented in the main text.