The dynamics about asteroid (162173) Ryugu

Abstract

The dynamical environment around the asteroid (162173) Ryugu is analyzed in detail using a constant-density polyhedron model based on the measurements from the Hayabusa2 mission. Six exterior equilibrium points (EPs) are identified along the ridge line of Ryugu, and their topological classifications fall into two distinctive categories. The initial periodic orbit (PO) families are computed and analyzed, including distant retrograde/prograde orbit (DRO/DPO) families and fifteen PO families emanating from the exterior EPs. The fifteen PO families are further divided into three categories: seven converge to an EP, seven reach Ryugu’s surface, and one exhibits cyclic behavior during its progression. The existence of initial PO families converging to an EP is analyzed using the bifurcation of a degenerate EP. Connection between these families and similar ones in the circular restricted three-body problem (CRTBP) is also examined. Bifurcated PO families are identified and computed from the initial PO families, including ten families from the DROs, fifteen from the DPOs, and twenty-five associated with the EPs. The bifurcated families are separately analyzed and categorized in terms of their corresponding initial families. Connections established by the same bifurcation points between different bifurcated families are identified. A comparison is made for the dynamical environments of Ryugu and Bennu to evaluate the similarities and differences in the evolution of EPs and the progression of PO families in top-shaped asteroids.

1 Introduction

Space missions to near-Earth asteroids (NEAs) represent a significant frontier in deep space exploration. Recent asteroid-specific sample-return missions, including JAXA’s Hayabusa2 mission to asteroid (162173) Ryugu (Watanabe et al. 2019; Saiki et al. 2020) and NASA’s OSIRIS-REx mission to asteroid (101955) Bennu (Scheeres et al. 2019), provide valuable insights into the composition, structure, and dynamics of these objects. More missions targeting the NEAs are scheduled in the near future, promising to further deepen our understanding of the formation and evolution of the solar system.

It is crucial to understand the dynamical environment about an asteroid for its mission design and the associated scientific research. Given the irregular shapes of observed NEAs, the dynamics in the vicinity of an asteroid is closely linked with the asteroid’s gravity field. In the previous research, three prevailing models are investigated and applied to accurately approximate the gravitation from an irregular-shaped asteroid, including the spherical/ellipsoidal harmonics model (Lundberg and Schutz 1988; Romain and Jean-Pierre 2001), the mass concentration model (Mascon) model (Geissler et al. 1996), and the polyhedron model (Werner 1994). In this work, a constant-density polyhedron model is utilized. The exterior gravitation due to a constant-density polyhedron is analytically derived by Werner (1994) with a concise expression of summations, and more details about the derivation are included in the author’s subsequent work (Werner and Scheeres 1996). Closed-form expressions for the spherical harmonic coefficients for the potential of a constant-density polyhedron are also presented (Werner 1997). Additionally, the potential of homogeneous-density polyhedron is also derived using line integrals by Petrović (1996), and the singularity in such a gravity field is further investigated (Tsoulis and Petrović 2001). Analytical computation of the gravity tensor using line integrals and its algorithmic implementation are also provided (Tsoulis 2012). In the case of Bennu, errors resulting from the constant-density assumption are measured to be within a few percent when compared with the real measurements obtained from the OSIRIS-REx mission (Scheeres et al. 2020). Characterized by no truncation error or convergence issue, the accuracy of this model primarily arises from the shape determination of a celestial body and its discretization. Although the discretization with a large number of triangular facets entails substantial computation, the polyhedron model is capable of achieving a sufficiently high precision and is widely applied.

With the dynamical model around an asteroid, one typical dynamical behavior to be calculated is the equilibrium points (EPs). In the previous research, the EPs have been computed in various asteroid models, ranging from simplified ones like a rotating straight segment (Romanov and Doedel 2014), a uniform disk (Fukushima 2010), and a uniformly rotating triaxial ellipsoid (Scheeres 1994), to more sophisticated shape models of NEAs, such as (433) Eros (Scheeres et al. 2000), (4769) Castalia (Scheeres et al. 1996), and (4179) Toutatis (Takahashi et al. 2013). The evolution of EPs in different scenarios is also investigated, including the annihilation of EPs under different spin rates (Jiang and Baoyin 2018) and the bifurcation of EPs during homotopy deformation of asteroids (Liu et al. 2021). In addition, the numerical methods to compute the EPs are also studied (Tardivel 2014; Brown and Scheeres 2023), and the method proposed in Tardivel (Tardivel 2014) offers the additional advantage of yielding the ridge line around an asteroid.

In addition to EPs, periodic orbits (POs) represent another important dynamical behavior to investigate the dynamical environment around an asteroid. For the PO family around an asteroid, the methods for its computation, bifurcation detection, and stability determination can be traced to the similar research on the circular restricted three-body problem (CRTBP). In Hénon’s research, the PO searching method based on the symmetry of an orbit is elaborated and the qualitative behavior and structure of achieved PO families are thoroughly discussed and summarized (Hénon 2003). Using standard numerical continuation and bifurcation methods, Doedel et al. compute the typical PO families and their bifurcations in the CRTBP, presenting an impressive bifurcation diagram of PO families in this model (Doedel et al. 2003). Furthermore, the stability of the PO families in the CRTBP is investigated by means of stability parameter and stability diagram proposed by Broucke (1969).

The aforementioned methods are further refined and adapted to investigate the POs in the irregular gravity fields of asteroids. Typical PO families being investigated can be classified into two categories: the distant retrograde/prograde PO families and the PO families around EPs. Families in the first category are mapped out around multiple asteroids with different gravity models, including (433) Eros (Scheeres et al. 2000) and (4769) Castalia (Hu and Scheeres 2008). Bifurcations of the families around the two asteroids are also identified and the associated bifurcated resonant families are computed (Chappaz 2011). The stable and unstable regions for retrograde PO families around the asteroids are determined as well (Lan et al. 2017). For the PO families in the second category, more mathematical description is provided using the manifold theory. PO families in this category can be systematically achieved from the analysis on the linearized dynamics about EPs. Early asteroid-related research employing this method is conducted by Scheeres et al. to investigate the POs and trajectories on the stable and unstable manifolds associated with EPs (Scheeres et al. 1996). Based on the linearized dynamics about EPs in the polyhedon model, the initial conditions of POs and the associated manifolds around EPs are derived by Jiang et al. (Jiang et al. 2014). Similar semi-analytic solutions of POs around EPs are derived by Soldini et al. (2020) in high-precision Mascon model to achieve 1:1 resonant PO families. In addition, it is noteworthy that the grid searching method combined with a predictor–corrector algorithm is also utilized to map out the PO families around an asteroid (Yu and Baoyin 2012; Yu et al. 2015; Hou et al. 2018). Despite requiring significant computation, the method has been validated to effectively generate abundant two- and three-dimensional PO families.

With a specific focus on Ryugu, this work presents a detailed analysis of the dynamical environment in the vicinity of Ryugu using the constant-density polyhedron model. Contributions are made from two major aspects. Firstly, the previous research on the dynamics of Ryugu is largely conducted within the three-body regime. In the study by Giancotti et al. (2014), candidate PO families meeting the requirements of Hayabusa2 mission are calculated in the Hill’s problem with the existence of solar radiation pressure acceleration. Additional perturbations, including the eclipse and $J_2$ perturbation of Ryugu, are included by Villegas-Pinto et al. (2020) to investigate their influence on three typical PO families in the Hill’s problem. Both works are conducted in the framework of three-body problem, where the irregular gravity field of Ryugu was not considered. In contrast, this work is based on an up-to-date high-resolution shape model of Ryugu, which is constructed from the optical navigation camera-telescopic images from Hayabusa2 mission (Watanabe et al. 2019) and made publicly available by the Japan Planetarium Association (2019). The achieved PO families in this work are dominated by Ryugu’s irregular gravity field and situated in close proximity to the asteroid, within a range of approximately 4 km. Furthermore, in comparison with the limited 1:1 resonant PO families around Ryugu’s EPs presented by Soldini et al. (2020), this work provides a comprehensive collection of PO families in the close vicinity of the asteroid. Secondly, while multiple similarities are observed between the two top-shaped asteroids, Ryugu and Bennu, they are still characterized by their unique attributes and remained to be explored individually. Detailed analysis on the dynamical environment around Bennu was performed in the recent work of Brown and Scheeres (2023a, 2023b). By comparing the results in the previous research, this work offers a summary on the similarities and differences in the dynamical environment of Ryugu and Bennu and provides further insight into this category of asteroids.

This paper is organized as follows. We start by introducing the constant-density polyhedron model and the corresponding dynamics defined in a body-fixed frame. Subsequently, the ridge line and EPs around Ryugu are presented, with the topological classification and linear stability of the EPs being discussed. In the following section, the pseudo-arclength continuation method to calculate a full PO family is summarized and the initial PO families of the aforementioned two PO categories are demonstrated. The bifurcated PO families from the initial PO families are further calculated, and the associated findings are discussed in a separate section. Based on the achieved results of Ryugu, a detailed comparison on the dynamical environment between Ryugu and Bennu is provided. Conclusions are drawn in the last section of this paper.

2 Dynamic model

In this section, the constant-density polyhedron model is introduced for the accurate modeling of Ryugu’s gravity field. Subsequently, the equations of motion of a point mass relative to a polyhedral Ryugu are derived in a body-fixed frame.

2.1 Polyhedron model

The polyhedron model is developed to numerically model the gravity field of small irregular celestial bodies. In this model, a polyhedron whose surface consists of planar triangles is employed to accurately approximate the shape of a small irregular body and enable the calculation of its gravity field. In this work, we employ the algorithm proposed by Werner and Scheeres (1996), which provides the closed-form expressions for the exterior gravitation due to a constant-density polyhedron. The gravitational potential U, gravitational force $\nabla U$, gravity gradient matrix $\nabla \nabla U$, and Laplacian $\nabla ^2 U$ of such a constant-density polyhedron at its external point are provided in Eq. (1) (Werner and Scheeres 1996):

$$\begin{aligned} U&=\frac{1}{2} G \sigma \sum _{e=1}^{n_e} \varvec{r}_e \cdot \varvec{E}_e \cdot \varvec{r}_e \cdot L_e-\frac{1}{2} G \sigma \sum _{f=1}^{n_f} \varvec{r}_f \cdot \varvec{F}_f \cdot \varvec{r}_f \cdot \omega _f \end{aligned}$$

(1a)

$$\begin{aligned} \nabla U&=-G \sigma \sum _{e=1}^{n_e} \varvec{E}_e \cdot \varvec{r}_e \cdot L_e+G \sigma \sum _{f=1}^{n_f} \varvec{F}_f \cdot \varvec{r}_f \cdot \omega _f \end{aligned}$$

(1b)

$$\begin{aligned} \nabla \nabla U&=G \sigma \sum _{e=1}^{n_e} \varvec{E}_e \cdot L_e-G \sigma \sum _{f=1}^{n_f} \varvec{F}_f \cdot \omega _f \end{aligned}$$

(1c)

$$\begin{aligned} \nabla ^2 U&=-G \sigma \sum _{f=1}^{n_f} \omega _f={\text {tr}}(\nabla \nabla U)= \left\{ \begin{array}{cc} 0 &{} \text { outside the body } \\ -4 \pi G \sigma &{} \text { inside the body } \end{array}\right. \end{aligned}$$

(1d)

where G is the gravitational constant and $\sigma$ is the celestial body’s density. $n_e$ and $n_f$ denote the number of edges and faces of the polyhedron, respectively. $\varvec{r}_e$ represents the vector from an external point to any point on an edge e, and $\varvec{r}_f$ represents the vector from an external point to any point in a face f. $\varvec{E}_e$ and $\varvec{F}_f$ are dyads defined by the normal vectors of an edge e and a face f. $L_e$ and $\omega _f$ are factors of integration related with an edge e and a face f, respectively. The expressions of $\varvec{E}_e$, $\varvec{F}_f$, $L_e$, and $\omega _f$ are included in Eq. (2):

$$\begin{aligned} \varvec{E}_e&=\hat{\varvec{n}}_{f}\left( \hat{\varvec{n}}_e^{f}\right) ^\textrm{T} + \hat{\varvec{n}}_{f^{\prime }}\left( \hat{\varvec{\varvec{n}}}_e^{f^{\prime }}\right) ^\textrm{T} \end{aligned}$$

(2a)

$$\begin{aligned} \varvec{F}_f&=\hat{\varvec{n}}_f \hat{\varvec{n}}_f^\textrm{T} \end{aligned}$$

(2b)

$$\begin{aligned} L_e&=\ln \frac{r_{i_e}+r_{j_e}+l_e}{r_{i_e}+r_{j_e}-l_e} \end{aligned}$$

(2c)

$$\begin{aligned} \omega _f&=2 \arctan \frac{\varvec{r}_{i_f} \cdot \varvec{r}_{j_f} \times \varvec{r}_{k_f}}{r_{i_f} r_{j_f} r_{k_f}+r_{i_f}\left( \varvec{r}_{j_f} \cdot \varvec{r}_{k_f}\right) +r_{j_f}\left( \varvec{r}_{k_f} \cdot \varvec{r}_{i_f}\right) +r_{k_f}\left( \varvec{r}_{i_f} \cdot \varvec{r}_{j_f}\right) } \end{aligned}$$

(2d)

where $\hat{\varvec{n}}_f$ denotes the unit normal vector of a face f. For an edge e (with a length of $l_e$) and its two associated faces f and $f^{\prime }$, $\hat{\varvec{n}}_e^{f}$ and $\hat{\varvec{\varvec{n}}}_e^{f^{\prime }}$ represent the unit edge-normal vectors perpendicular to $\hat{\varvec{n}}_f$ and $\hat{\varvec{n}}_{f^{\prime }}$. The set of vectors from an external point to the vertices of an edge e and a face f are, respectively, denoted by $\{\varvec{r}_{i_e}, \varvec{r}_{j_e}\}$ and $\{\varvec{r}_{i_f}, \varvec{r}_{j_f}, \varvec{r}_{k_f}\}$. The norm of these vectors is denoted by the associated unbold characters, i.e., $r_{i_e}$, $r_{j_e}$, $r_{i_f}$, $r_{j_f}$, and $r_{k_f}$.

2.2 Equations of motion

To derive the equations of motion of a mass particle in an asteroid’s gravity field approximated by the constant-density polyhedron model, a body-fixed frame $\mathcal {B}$: $\{\hat{\varvec{x}}, \hat{\varvec{y}}, \hat{\varvec{z}} \}$ is defined firstly. The origin of this $\mathcal {B}$-frame coincides with the barycenter of an asteroid and the frame rotates in such a fashion that the asteroid is fixed in the frame. Specifically, the positive direction of z axis, $+\hat{\varvec{z}}$, is kept aligned with the asteroid’s angular velocity vector $\varvec{\omega }$, i.e., $\varvec{\omega } = \omega \hat{\varvec{z}}$. Since the mass distribution remains constant in this frame, the directions of $\hat{\varvec{x}}$ and $\hat{\varvec{y}}$ can be suitably oriented provided that the right-hand rule is satisfied. In addition, the following normalized units are selected: Length is scaled by the radius of the sphere that has the same volume as the asteroid of interest. Time is scaled so that the synodic period of the asteroid is 2$\pi$. Based on the normalized units above, the equations of motion of a mass particle in the $\mathcal {B}$-frame can be stated as:

$$\begin{aligned} \dot{\varvec{X}} = \varvec{f}(\varvec{X}) =\left[ \begin{array}{c} \varvec{v} \\ -2 \varvec{\omega } \times \varvec{v} -\nabla V(\varvec{r}) \end{array} \right] \end{aligned}$$

(3)

where $\varvec{r}$ and $\varvec{v}$ denote, respectively, the position and velocity vector of the particle, and $V(\varvec{r})$ is the amended potential which includes both rotational and gravitational effects. Considering the gravitational potential $U(\varvec{r})$ of the polyhedron model, $V(\varvec{r})$ can be expressed as:

$$\begin{aligned} V(\varvec{r}) = -\frac{1}{2}\Vert \varvec{\omega } \times \varvec{r}\Vert ^2 - U(\varvec{r}) \end{aligned}$$

(4)

From the time-invariant dynamics defined by Eq. (3), an energy integral can be derived for the system:

$$\begin{aligned} \mathcal {C} =\frac{1}{2} \dot{\varvec{r}} \cdot \dot{\varvec{r}}+ V(\varvec{r}) \end{aligned}$$

(5)

which is referred to as the Jacobi integral or Jacobi constant (Scheeres 2016). The surface $\mathcal {C} = V(\varvec{r})$ is known as the zero-velocity surface, which represents the boundary of a particle’s possible motion with a fixed value of energy. In addition, the intersection of the zero-velocity surface with a plane is called a zero-velocity curve. The zero-velocity surfaces with different values of Jacobi constant around Ryugu are illustrated in Fig. 1. When the values of Jacobi constant are relatively small, the zero-velocity surfaces restrict a particle’s motion to two possible regions: one interior region in the close vicinity of Ryugu and an exterior region expanding infinitely in x and y directions. As the Jacobi constant increases, the two regions of possible motion are connected and the forbidden region keeps shrinking. The whole space becomes reachable when the value of Jacobi constant is sufficiently large.

Note that while previous studies on asteroid dynamics have considered the perturbation of the solar radiation pressure (SRP), it is excluded from this study and its corresponding modeling is absent in Eq. (3). This consideration stems from the observation that, for the dynamics of boulders and large particles in the vicinity of irregularly shaped asteroids (such as Ryugu and Bennu), the influence of the SRP is less dominant compared to the irregular gravitational field of the asteroid (Scheeres et al. 2016; McMahon et al. 2020). In contrast, for particles at the centimeter scale and even smaller, the effect of the SRP cannot be disregarded (Villegas-Pinto et al. 2020; Amarante et al. 2021). As this research focuses on the dynamics of boulders and large particles, the SRP is not explored.

Fig. 1

Zero-velocity surfaces with different values of the Jacobi constant

3 Ridge line and equilibrium points

In this section, the ridge line is introduced for the calculation of equilibrium points around Ryugu in the body-fixed frame. The topological classification and linear stability of the achieved equilibrium points are presented.

3.1 Ridge line

An equilibrium point (EP) is a special-case orbit which remains stationary in the body-fixed frame, with zero acceleration and velocity. An algorithm was proposed by Tardivel (2014) to solve for the EPs around an asteroid using the following condition defined in cylindrical coordinates:

$$\begin{aligned} \nabla V(\varvec{r}_\textrm{cyl}) = \nabla V(h,\theta ,z) = \varvec{0} \end{aligned}$$

(6)

where the three coordinates, h, $\theta$, and z, are, respectively, defined as the radial distance, azimuth, and height. It is remarked that $\varvec{r}_\textrm{cyl}$ denotes a vector with cylindrical coordinates ($h,\theta ,z$). From the algorithm, a useful concept, $zh^*$ set, is subsequently defined as:

$$\begin{aligned} zh^*=\left\{ \varvec{r}_\textrm{cyl} \in \textbf{R}^3 \mid V_{h}(\varvec{r}_\textrm{cyl})=0, V_z(\varvec{r}_\textrm{cyl})=0\right\} \end{aligned}$$

(7)

where $V_{h}(\varvec{r}_\textrm{cyl})$, $V_{\theta }(\varvec{r}_\textrm{cyl})$, and $V_z(\varvec{r}_\textrm{cyl})$ represent the first-order partial derivatives of V with respect to the corresponding cylindrical coordinates at the subscripts. The $zh^*$ set is proved as a closed smooth one-dimensional manifold and further referred to as the ridge line (Tardivel 2014). When a ridge line is parameterized by $\theta$, the $V_{\theta }(\varvec{r}_\textrm{cyl})$ along a ridge line is continuous. The set of points on a ridge line satisfying $V_{\theta }(\varvec{r}_\textrm{cyl}) = 0$ are the EPs around an investigated asteroid. It is further proved that, when excluding the existence of degenerate and resonant EPs, an even number of EPs are located on a ridge line (Tardivel 2014). In this case, the topological classification of these EPs alternates between limited different types when $\theta$ changes monotonically along a ridge line (Tardivel 2014).

3.2 Equilibrium points and their stability

Six EPs are identified around Ryugu by solving Eq. (6) using Tardivel’s algorithm based on the ridge line. The six EPs are numbered in an ascending order in terms of their azimuth coordinates. The locations and Jacobi constant values of the EPs are listed in Table 1. Due to the asymmetry of Ryugu’s shape, the six EPs are located close to the xy-plane with a small amplitude in z direction. The EPs are plotted in the left plot of Fig. 2 along with the ridge line around Ryugu and the zero-velocity curves on the xy-plane. To further illustrate the energy levels around the EPs and the ridge line, the amended potential on the xy-plane is shown in three dimensions in the right plot of Fig. 2. The amended potential of each point on the ridge line is the local maximum potential along the corresponding radial direction. Furthermore, the six EPs’ locations correspond to the local extrema of the amended potential along the ridge line. EP1 and EP3 are the EPs with the highest energy level, while the energy of the three adjacent EPs, i.e., EP4, EP5, and EP6, is relatively close and lower.

Table 1 Locations of equilibrium points around Ryugu in the body-fixed frame

Fig. 2

Equilibrium points and ridge line around Ryugu in the body-fixed frame. Left: The equilibrium points (solid magenta points), ridge line (the magenta curve), and amended potential V on xy-plane (the surface plot). Right: The equilibrium points (solid magenta points), ridge line (the magenta curve), and amended potential V in three dimensions (the surface plot)

The linear stability of an EP can be determined using the linearized dynamics about the EP based on Eq. (3). A Jacobian matrix $\varvec{A}$ can be derived from the linearized dynamics for small perturbations, which can be expressed as:

$$\begin{aligned} \varvec{A} = \left[ \begin{array}{ll} \varvec{0}_{3\times 3} &{} \varvec{I}_{3\times 3} \\ -\nabla \nabla V(\varvec{r}) &{} -2[\varvec{\omega }] \end{array} \right] \text{ where } [{\varvec{\omega }}] = \left[ \begin{array}{ccc} 0 &{} -1 &{} 0 \\ 1 &{} 0 &{} 0 \\ 0 &{} 0 &{} 0 \end{array} \right] \end{aligned}$$

(8)

By analyzing the eigenvalues of the resulting Jacobian matrix $\varvec{A}$, the topological structure of the EP can be achieved and its linear stability can be determined. According to Jiang et al. (2014), the topological structure of non-degenerate and non-resonant EPs around a rotating asteroid can be categorized into six categories, which are enumerated in Table 2. Each category corresponds to a different combination of the following three types of eigenvalue pairs: (1) a pair of conjugate pure imaginary numbers $\pm \text {i} \beta \, (\beta \in \textbf{R}^{+})$, which is defined as a center; (2) a pair of opposite real numbers $\pm \alpha \; (\alpha \in \textbf{R}^{+})$, which is defined as a saddle; (3) a pair of conjugate complex numbers $\pm \sigma \pm \text {i} \tau \; (\sigma , \tau \in \textbf{R}^{+})$, which is defined as a spiral. Among all the possible combinations shown in Table 2, only the EPs falling into the first category are linearly stable. In addition, each center eigenvalue pair individually corresponds to a two-dimensional center manifold, which ensures the existence of a periodic orbit (PO) family emanating from the EP (Scheeres 2016).

Table 2 Topological classification of non-degenerate and non-resonance equilibrium points (Jiang et al. 2014)

Regarding the six EPs around Ryugu, the eigenvalues $\lambda _i\;(i=1,\ldots ,6)$ of their Jacobian matrices are provided in Table 3. The topological classifications of the EPs are discovered to alternate between the first two categories in Table 2. EP1, EP3, and EP5 belong to the category center $\times$ center $\times$ center and allow the existence of three PO families. In comparison, EP2, EP4, and EP6 are included in the category saddle $\times$ center $\times$ center and allow the existence of two PO families.

Table 3 Eigenvalues of Jacobian matrices of the EPs around Ryugu

Note that there are also six EPs identified around Ryugu in the work by Soldini et al. (2020). Different from the constant-density polyhedron model applied in this research, a Mascon model of Ryugu uniformly packed by 1.4 million spherical particles is employed to compute Ryugu’s gravity field. The topological classifications of each EP generated by the two models are identical as well. Minor discrepancies are observed in the EPs’ locations between the two models, which should result from the slight differences in Ryugu’s topology in the respective shape models applied by the two studies.

4 Initial periodic orbit families

Two specific types of PO families, the distant retrograde/prograde PO families and the PO families emanating from EPs, are investigated for the dynamical environment around Ryugu. These PO families are collectively referred to as the initial PO families in this paper, as the PO families bifurcated from them are subsequently calculated and demonstrated in the following section. In this section, the methodology to continue a PO family is introduced, including a predictor–corrector scheme based on pseudo-arclength continuation method and the initialization of a continuation process. The achieved initial PO families around Ryugu are subsequently illustrated in different categories and the associated findings are discussed.

4.1 Methodology to continue a PO family

4.1.1 Pseudo-arclength continuation

In this study, the pseudo-arclength continuation method (Keller 1977) is applied to compute a complete initial PO family around Ryugu. Consider a system of finite-dimensional equations:

$$\begin{aligned} \varvec{f}(\varvec{X}) = {\textbf {0}}, \;\varvec{f}: \textbf{R}^{n+1} \rightarrow \textbf{R}^{n} \end{aligned}$$

(9)

where ${\varvec{{f}}}$ is assumed to be sufficiently smooth and the number of equations is one less than the number of variables. Generically, given a locally unique solution branch and a solution $\varvec{X}_0$ on the branch, the classic Newton’s method can be utilized to solve Eq. (9) for an adjacent solution $\varvec{X}_1$ on this branch. In the pseudo-arclength continuation method, the following extended equations are employed:

$$\begin{aligned} \left\{ \begin{array}{l} \varvec{f}(\varvec{X}_0) = {\textbf {0}} \\ \dot{\varvec{X}}_0^\textit{T} \cdot (\varvec{X}_1 - \varvec{X}_{0}) = \Delta s \end{array} \right. \end{aligned}$$

(10)

where $\dot{\varvec{X}}_0$ is the unit tangent to the solution branch at $\varvec{X}_{0}$, the superscript $\textit{T}$ denotes matrix transpose, and $\Delta s$ is the pseudo-arclength continuation step size. It is proved that the above continuation method works near a regular solution $\varvec{X}_{0}$, i.e., the null space of the Jacobian matrix $\nabla \varvec{f} (\varvec{X}_0)$ is one-dimensional. In this case, the Jacobian of Eq. (10) evaluated at $\varvec{X}_{0}$ is non-singular (Seydel 2009). According to the implicit function theorem (Keller 1977), such a fact guarantees the existence of a locally unique solution branch through $\varvec{X}_{0}$ and this branch can be parameterized locally by $\Delta s$. In addition, for a sufficiently small $\Delta s$ and a sufficiently accurate initial approximation, the Newton’s method is proved to converge for solving Eq. (10) (Seydel 2009).

4.1.2 Predictor–corrector scheme

Based on the pseudo-arclength continuation method, a predictor–corrector scheme using the Newton’s iteration is designed to continue an initial PO family. In this scheme, the predictor offers the initial estimation of a family member and the associated tangent vector along a family branch. The corrector solves for the accurate state of a family member using the information obtained from the predictor. Details of these two segments are as follows.

The predictor provides an initial guess for Newton’s iterations. For the continuation of POs, two critical values should be provided: One is the estimated initial state $\tilde{{\varvec{{X}}}}_0$ of a PO, and another is the estimated period $\tilde{T}$ for the PO. These two values can be approximated by:

$$\begin{aligned} \left\{ \begin{array}{l} \tilde{\varvec{X}}_0^{(i+1)} = \varvec{X}_0^{(i)} + \frac{\text {d}\varvec{X}}{\text {d}s}|_{\varvec{X} = \varvec{X}_0^{(i)}} \cdot \Delta s \\ \tilde{\textit{T}}^{(i+1)} = \textit{T}^{(i)} + \frac{\text {d}{} \textit{T}}{\text {d}s}|_{\textit{T} = \textit{T}^{(i)}} \cdot \Delta s \end{array} \right. \end{aligned}$$

(11)

where the superscripts i and (i+1) denote the ith and (i+1)th members in a PO family, respectively. $\varvec{X}_0^{(i)}$ and $\textit{T}^{(i)}$ are the accurate initial state and period of the ith family member. $\frac{\text {d}\varvec{X}}{\text {d}s}|_{\varvec{X} = \varvec{X}_0^{(i)}}$ and $\frac{\text {d}{} \textit{T}}{\text {d}s}|_{\textit{T} = \textit{T}^{(i)}}$ are two family tangents along a pseudo-arc s for state $\varvec{X}_0^{(i)}$ and period $\textit{T}^{(i)}$, respectively. The logic behind the predictor in Eq. (11) is to generate an initial guess for a subsequent member based upon the previously achieved one in a PO family.

The corrector corresponds to the iteration in a numerical root-finding algorithm. For the continuation of a PO family, a Newton’s method-based iteration process is applied to solve for the accurate state $\varvec{X}_0$ and period $\textit{T}$ from three types of equations (or constraints), which can be expressed by:

$$\begin{aligned} \left\{ \begin{array}{l} \phi _t (\varvec{X}_0^{(i+1)}) |_{{t=\textit{T}}^{(i+1)}} - \varvec{X}_0^{(i+1)} = {\textbf {0}} \\ {}[\varvec{f}(\varvec{X}_0^{(i)})]^\textrm{T} \cdot (\varvec{X}_0^{(i+1)} - \varvec{X}_0^{(i)}) = 0 \\ \left[ \frac{\text {d}\varvec{X}}{\text {d}s}|_{\varvec{X}_0 = \varvec{X}_0^{(i)}}\right] ^\textrm{T} \cdot \Delta \varvec{X}_0 + \frac{\text {d}{} \textit{T}}{\text {d}s}|_{\textit{T}=\textit{T}^{(i)}} \cdot \delta T - \delta s = 0 \end{array} \right. \end{aligned}$$

(12)

The first equation in Eq. (12) is a boundary value condition, which is established upon the periodicity of a PO. $\phi _t (\varvec{X}_0^{(i+1)}) |_{{t=\textit{T}}^{(i+1)}}$ denotes the final state integrated with the initial state $\varvec{X}_0^{(i+1)}$ for a time span of $\textit{T}^{(i+1)}$. The second equation is a Poincaré phase constraint (Seydel 2009), which can be interpreted geometrically that $\varvec{X}_0^{(i+1)}$ should lie in a hyperplane which is orthogonal to the tangent vector $\varvec{f}(\varvec{X}_0^{(i)})$ of an existing PO determined by $\varvec{X}_0^{(i)}$. The third equation is a pseudo-arclength constraint, which corresponds to the appended equation in Eq. (10). Equation (12) can be written in a simpler fashion as a vector function:

$$\begin{aligned} \mathcal {F} (\varvec{X}_0^{(i+1)}, \textit{T}^{(i+1)}) = {\textbf {0}} \end{aligned}$$

(13)

and the iteration function for the above equation is given as:

$$\begin{aligned}{} & {} {[}{\varvec{X}_0^{(i+1)}}_{(j+1)}, {\textit{T}^{(i+1)}}_{(j+1)}] = [{\varvec{X}_0^{(i+1)}}_{(j)}, {\textit{T}^{(i+1)}}_{(j)}] \nonumber \\{} & {} \quad - [\nabla \mathcal {F} ({\varvec{X}_0^{(i+1)}}_{(j)}, {\textit{T}^{(i+1)}}_{(j)})]^{\dagger } \; \mathcal {F} ({\varvec{X}_0^{(i+1)}}_{(j)}, {\textit{T}^{(i+1)}}_{(j)}) \end{aligned}$$

(14)

where the extra subscripts j and (j+1) denote the jth and (j+1)th iterations, respectively. The symbol $\dagger$ denotes the pseudo-inverse of the non-square Jacobian matrix $\nabla \mathcal {F}$ evaluated at $[{\varvec{X}_0^{(i+1)}}_{(j)}, {\textit{T}^{(i+1)}}_{(j)})]$. The non-square Jacobian matrix in Eq. (14) can be derived analytically as:

$$\begin{aligned} \nabla \mathcal {F} ({\varvec{X}_0^{(i+1)}}, \textit{T}^{(i+1)}) = \left[ \begin{array}{ll} \varvec{\Phi }({\textit{T}^{(i+1)}}) - {\varvec{{I}}}_{6 \times 6} &{} \varvec{f}(\varvec{X}^{(i+1)}_{\textit{T}^{(i+1)}}) \\ {[}\varvec{f}(\varvec{X}^{(i)}_0)]^\textit{T} &{} 0 \\ \left[ \frac{\text {d}\varvec{X}}{\text {d}s}|_{\varvec{X}_0 = \varvec{X}_0^{(i)}}\right] ^\textrm{T} &{} \frac{\text {d}{} \textit{T}}{\text {d}s}|_{\textit{T}=\textit{T}^{(i)}} \end{array} \right] \end{aligned}$$

(15)

where $\varvec{\Phi }({\textit{T}^{(i+1)}})$ is the state transition matrix (STM) evaluated at $\textit{T}^{(i+1)}$. The STM of the dynamics in Eq. (3) can be acquired from the integration of the following equation:

$$\begin{aligned} \dot{\varvec{\Phi }}(t) = \varvec{A}\;\varvec{\Phi }(t), \text {with}\; \varvec{\Phi }(t_0) = \varvec{I}_{6\times 6} \end{aligned}$$

(16)

Once the accurate values of ${\varvec{X}_0^{(i+1)}}$ and $\textit{T}^{(i+1)}$ are solved from Newton’s iterations, the associated family tangents can be readily achieved by calculating the null space of Eq. (15), and further applied to estimate the information of a next family member using Eq. (11). The value $\nabla ^2 U$ in Eq. (1d) is applied to determine whether a PO collides with the asteroid’s surface. When a negative $\nabla ^2 U$ is detected in orbit propagation, both the orbit propagation and continuation process will be terminated.

4.1.3 Initialization of continuation

To initialize the predictor–corrector scheme, the family tangents $\frac{\text {d}\varvec{X}}{\text {d}s}|_{\varvec{X} = \varvec{X}_0^{(0)}}$ and $\frac{\text {d}{} \textit{T}}{\text {d}s}|_{\textit{T} = \textit{T}^{(0)}}$ have to be estimated and provided together with the state $\varvec{X}_0^{(0)}$ and period $\textit{T}^{(0)}$. For the distant retrograde/prograde orbit families, the related initialization can resort to their existence in the Kepler problem (Batkhin 2013). The continuation of these families normally starts with a nearly circular member located sufficiently far away from an asteroid. In this case, ${\varvec{{X}}}_0^{(0)}$ is given as:

$$\begin{aligned} {\varvec{{X}}}_0^{(0)} = [a_p\;\;0\;\;0\;\;0\;\;\left( \pm \sqrt{\frac{1}{a_p}}-a_p\right) \;\;0]^{T} \end{aligned}$$

(17)

where $a_p$ is the amplitude of the first member in the family. The sign in front of the term $\sqrt{\frac{1}{a_p}}$ is a positive sign for the prograde family, a negative sign for the retrograde family. For the PO computations related to Ryugu, $a_p$ is set to 8 in the normalized unit to ensure a sufficiently large distance from the asteroid. With a sufficiently large $a_p$, the period value ${\textit{T}}^{(0)}$ is set to $2\pi$. Moreover, $\frac{\text {d}\varvec{X}}{\text {d}s}|_{\varvec{X} = \varvec{X}_0^{(0)}}$ is selected as $\frac{\varvec{X}_0^{(0)}}{||\varvec{X}_0^{(0)}||}$, and $\frac{\text {d}{} \textit{T}}{\text {d}s}|_{\textit{T} = \textit{T}^{(0)}}$ is set to zero.

For the PO family originating from an EP, the initialization to continue such a PO family has recourse to its associated center manifold (Koon et al. 2000). Let the center manifold be characterized by a pair of conjugate imaginary eigenvalues $\pm \text {i}\lambda \; (\lambda \in \textbf{R}^{+})$ and a normalized eigenvector $\nu _c$. In this case, ${\varvec{{X}}}_0^{(0)}$ is selected as the coordinate of the EP. The period value ${\textit{T}}^{(0)}$ is estimated by $\frac{2\pi }{\lambda }$. In addition, $\frac{\varvec{X}_0^{(0)}}{||\varvec{X}_0^{(0)}||}$ is selected as the real part of vector $\nu _{c}$. Empirically, $\frac{\text {d}{} \textit{T}}{\text {d}s}|_{\textit{T} = \textit{T}^{(0)}}$ can be given as zero when a pseudo-arclength step $\delta s$ is sufficiently small (Gómez et al. 1991). For the PO computations related to Ryugu, $\delta s$ is set to 10$^{-4}$ in the normalized unit.

4.2 Numerical simulation of initial PO families

Utilizing the predictor–corrector scheme based on the pseudo-arclength continuation method, seventeen initial PO families are calculated and summarized in Table 4, including the distant retrograde orbit (DRO) family, distant prograde orbit (DPO) family, and fifteen PO families emanating from Ryugu’s exterior EPs. The DRO and DPO families are illustrated in Sect. 4.2.1, and the fifteen PO families associated with the EPs are provided in Sect. 4.2.2. Since the two topological classifications of Ryugu’s exterior EPs, $\textit{center}$ $\times$ $\textit{center}$ $\times$ $\textit{center}$, and $\textit{saddle}$ $\times$ $\textit{center}$ $\times$ $\textit{center}$, allow two and three PO families emanating from the EPs, respectively, the following naming rule is employed to differentiate these PO families. The PO families emanating from the same EP are sorted by their orbital periods in a descending order, and the jth PO family emanating from the ith EP is denoted by EiCj. Additionally, the fifteen PO families associated with Ryugu’s EPs are divided into three categories according to their structures and the properties observed in the continuation processes. The PO families in each category will be separately discussed in the corresponding sections.

Table 4 Initial PO families

Furthermore, different terms are utilized to describe the PO families from different aspects. “Progression” denotes the alteration of family members as a continuation process takes place. “Lyapunov” (with quotation marks) is employed when describing orbits that have similarities with a part of a Lyapunov family around the homogenous triaxial ellipsoid model (Romanov and Doedel 2012), or to describe orbits very close to the xy-plane. “Vertical” (with quotation marks) is used when describing orbits that are similar to a portion of a Vertical family around the homogenous triaxial ellipsoid model (Romanov and Doedel 2012), or to describe orbits with a notable out-of-plane amplitude. When both “Lyapunov” and “vertical” members are identified in a family, the family would be labeled as “Combined.”

4.2.1 Distant retrograde/prograde orbit families

The DRO and DPO families around Ryugu are illustrated in Fig. 3. The continuation of both families starts at 4 km away from the Ryugu’s surface (which corresponds to $a_p = 8$ in Eq. (17)) and terminates when their last family members reach the asteroid’s surface. Note that for demonstration purposes, only a portion of both families is presented in Fig. 3. Both families can be continued to quite far from Ryugu in the employed body-fixed frame. For both families illustrated in Fig. 3, their first family member is represented in black, the last member in red, and the intervening members in light blue. The POs in both families are nearly circular and located very close to the xy-plane when their amplitudes are sufficiently large. However, as both families gradually approach Ryugu, their family members in the vicinity of the asteroid exhibit an out-of-plane amplitude. A maximum out-of-plane amplitude of 35 m is observed when the DRO family reaches the surface (see Fig. 3c). In comparison, the vertical shifting of the DPO family is more evident as shown in Fig. 3d. It is noteworthy that the out-of-plane shifting of both families results from the asymmetric shape of Ryugu compared to the Kepler problem. The influence of the asymmetric gravitational field intensifies in the asteroid’s close vicinity as it is function of the local topographical features, e.g., craters and ridges.

Fig. 3

Distant retrograde and prograde orbit families around Ryugu. In both families, the first family member is represented in black, the last member in red, and the intervening members in light blue.Top left: DRO family around Ryugu. Top right: DPO family around Ryugu. Bottom left: Out-of-plane members in the DRO family. Bottom right: Out-of-plane members in the DPO family

The variation of Jacobi constant $\mathcal {C}$ and orbital period T of the DRO and DPO families is shown in Fig. 4. In both plots of Fig. 4, a black pentagon represents the first family member’s $\mathcal {C}$ and T values, while a red pentagon signifies the corresponding values of the last family member. The energy level of the DRO family is much higher than that of the DPO family, while the orbital period of the DRO family is smaller than that of the DPO family. The two families exhibit opposing period variations as their members approach Ryugu’s surface. The members in the DRO family show a decreasing tendency in orbital periods during the progression, while the DPO family displays an increasing tendency. In addition, the steep segment of the $\mathcal {C}$–T curve in Fig. 4b corresponds to the out-of-plane members in the DPO family.

Fig. 4

$\mathcal {C}$–T variation of the distant retrograde and prograde orbit families around Ryugu. The $\mathcal {C}$ and T values of the first and last family members are represented by black and red pentagons, respectively. Left: $\mathcal {C}$–T variation of the DRO family. Right: $\mathcal {C}$–T variation of the DPO family

4.2.2 Orbit families emanating from equilibrium points

Fifteen initial orbit families emanating from the EPs are identified around Ryugu. Their general properties are summarized in Table 5, including their minimum/maximum Jacobi constant $\mathcal {C}$ and orbital period T. It is noteworthy that each EP allows the existence of both a “Lyapunov” and a “vertical” family originating from itself. Furthermore, there is an additional “Combined” family emanating from each EP of the type center $\times$ center $\times$ center. The three “Combined” families are characterized by a much larger orbital period in comparison with that of the other families and exhibit more complex progression processes during the continuation. In addition, the fifteen families are further classified into three categories in terms of their individual structures and the properties observed in their continuation. Category A includes the PO families finally converging to an EP during continuation. Category B contains the PO families whose continuation terminates when reaching Ryugu’s surface. In Category C, the progression of a PO family exhibits a cyclic property. Such a cyclic property is evident in the $\mathcal {C}$–T curve of this family, where the family’s progression corresponds to a back-and-forth movement along that curve. Detailed discussions on each category are provided in the subsequent content.

Table 5 Properties of initial PO families emanating from EPs

Category A: Families converging to an EP

In this category, the continuation of a PO family starts from one EP and finally ends at another. Such a phenomenon is observed to occur between two pairs of adjacent EPs: EP2 and EP3, EP4 and EP5. Seven PO families fall into this category, including the families E2C1, E3C2, E4C1, E5C1, E5C2 and E5C3. Three pairs of these families, E2C1 and E3C2, E4C1 and E5C2, E4C2 and E5C3, are discovered to consist of identical members and illustrated in Fig. 5. Although the continuation sequence of the two families in each pair is opposite, the two families in one pair are characterized by a same structure. Among these families, the families E2C1 (E3C2) and E4C1 (E5C2) are of the “Lyapunov” type, while the family E4C2 (E5C3) of the “vertical” type. In Fig. 5c, a typical member in the family E4C2 (E5C3) is highlighted in magenta to showcase the orbit’s shape and the family’s extension in three dimensions.

Fig. 5

Six initial PO families converging to an EP. Family members are represented in light blue. Magenta is used to highlight a typical member in the family

It is remarked that the rigorous proof for the existence of the families connecting two EPs is still pending. Recently, Brown and Scheeres propose a possible explanation for this phenomenon utilizing the concept of “genesis event” (Brown and Scheeres 2023a). In their work, a “genesis event” refers to the situation when a degenerate EP around a rotating asteroid bifurcates into two adjacent EPs as the asteroid’s spin rate increases (Brown and Scheeres 2023b). The EPs’ evolution around Bennu is further investigated to showcase the existence and mechanism of such an event. In addition, Jiang et al. (2015) provided a comprehensive classifications for all the possible bifurcations of a degenerate EP in this event.

Fig. 6

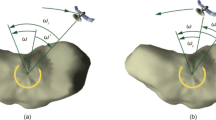
Genesis event of the EPs around Ryugu. Left: Locations of the EPs around Ryugu, with the reference ridge line in magenta to indicate the reference spin rate. Right: A zoom representation of the black box region in the left plot, where a degenerate EP bifurcates into EP4 and EP5. The two red arrows indicate the directions in which the branches of EP4 and EP5 extend locally

In this work, the evolution of EPs around Ryugu with respect to different spin rates is also investigated to shed light on the existence of the PO families in this category. To illustrate the variation in Ryugu’s spin rate and the corresponding synodic rotation period, a scaling factor $\kappa$ is defined to indicate the scaling of the rotation period with respect to its reference value. The locations of EPs are computed and illustrated in Fig. 6 when $\kappa$ decreases from 3 to 1. Reducing $\kappa$ to 1 results in achieving the reference EPs reported in Sect. 3.2, accompanied by the plotting of the reference ridge line in Fig. 6 to indicate the current reference spin rate. The degenerate EP emerges when $\kappa$ is decreased to 1.2822434 and the branches of EP4 and EP5 appear as $\kappa$ is further decreased. It is validated that the eigenvalues of the Jacobian matrix evaluated at the degenerate EP are composed of a pair of zeros and two pairs of center. When the degenerate EP bifurcates into EP4 and EP5, the zero eigenvalue pair evolves into a pair of saddle and a pair of center correspondingly for the two EPs. The rest two center eigenvalue pairs also changes continuously into the corresponding center pairs of EP4 and EP5 as the $\kappa$ decreases. The right plot of Fig. 6 is a zoom representation of the black box region in the left plot. Two red arrows indicate the directions in which the branches of EP4 and EP5 extend locally in the xy-plane. It is validated that the two direction vectors at the degenerate EP align with the vector composed of the position components of the eigenvector associated with the zero eigenvalues. In addition, a consistent variation is also observed for the components in the corresponding eigenvectors as $\kappa$ decreases.

Given the observation above, an intuitive explanation can be formulated for the presence of the PO families connecting EP4 and EP5 in Fig. 5. Let $\omega _c$ denote the spin rate at which EP4 and EP5 bifurcate from the degenerate EP. When the spin rate of Ryugu is further increased infinitesimally, EP4 and EP5 maintain an extremely close proximity to each other. In this scenario, the two center eigenvalue pairs of EP4 and EP5 just experience a minute change, remaining almost identical to the corresponding pairs of the degenerate EP. Likewise, the eigenvector to initialize the continuation of a PO family for a center eigenvalue pair of EP4 and its counterpart of EP5 is almost the same. Initialized by the same perturbation stemming from the same eigenvector, the two generated PO families are supposed to include the same members and can be considered as one single family. Moreover, such a family should also originate from both EP4 and EP5 as both EPs are the locations where the continuation starts. When the spin rate of Ryugu is increased continuously, the PO family evolves correspondingly and still connects EP4 and EP5. As two center eigenvalue pairs are directly associated with the degenerate EP, this fact explains straightforwardly the existence of two PO families connecting EP4 and EP5. Due to the perturbations resulting from the two different eigenvectors, one PO family becomes “Lyapunov” (see Fig. 5b) and another is “vertical” (see Fig. 5c).

However, it is noteworthy that the aforementioned explanation based on the degenerate EP’s bifurcation cannot account for the existence of family E2C1 and its identical counterpart E3C2. It is verified that no “genesis event” occurs between the EP2 and EP3 when the spin rate of Ryugu is varied. The EP2 and EP3 are classified as the “original” EPs which exist in the homogenous triaxial ellipsoid model (Romanov and Doedel 2012). To understand the existence of the family E2C1 (E3C2), it is possible to draw a connection between this family to the similar families identified in the CRTBP. In Van Anderlecht’s work (Van Anderlecht 2016), the orbits enabling transfers between the triangular libration points $L_4$ and $L_5$ are investigated, along with the PO families emanating from these two EPs. The PO families connecting $L_4$ (with a topology of $\textit{center}$ $\times$ $\textit{center}$ $\times$ $\textit{center}$) and $L_3$ (with a topology of $\textit{saddle}$ $\times$ $\textit{center}$ $\times$ $\textit{center}$) are discovered when changing the system’s mass ratio (Van Anderlecht 2016). It is verified that when the mass ratio $\mu$ falls within the range $\left[ 0.0009, 0.004\right]$, “Lyapunov” families originating from $L_4$ and converging to $L_3$ can be continued using the same initialization method in Sect. 4.1.3. These families should have an inherent connection with the family E2C1, as they connect the adjacent “original” EPs of the same topologies. In addition, a limited variation in the energy level is observed between the members in these PO families. Although more work should be carried out in this area, it is expected that the existence of such a family results from a combination of factors, including the topography of the adopted shape model, the EPs’ topology, the system’s dominant parameter, and the constraint imposed on the energy level.

Different from the aforementioned families in this category, the family E5C1 has no same counterpart among the PO families emanating from EP4, but still converges to the EP4. The progression of family E5C1 is shown in Fig. 7 and separated into four consecutive subprocesses. Each subprocess is depicted with three subplots to illustrate the progression of family members. Following the same coloring rule as in Fig. 3, in each subplot, the first member in the progression is represented in black, the last in red, and the intervening members in light blue. The color magenta is applied to highlight the typical shape a family member. As shown in the first subplot of Fig. 7a, the family originates from EP5 and expands horizontally in the xy-plane with “Lyapunov” members. At a certain point, the family members start acquiring an out-of-plane amplitude and expanding both horizontally and vertically. Upon reaching a local maximum in-plane amplitude, the family begins to contract horizontally and ultimately reaches a local minimum in-plane amplitude (see the second subplot in Fig. 7a). At this point, the out-of-plane amplitude of the family reaches its maximum. Subsequently, the family restarts expanding in both x and y directions as shown in the third subplot in Fig. 7a. It undergoes a second contraction when reaching another local maximum in-plane amplitude, and culminates with a “Lyapunov” member. Meanwhile, the out-of-plane amplitude of the family decreases consistently. The subprocess illustrated in Fig. 7a repeated twice more. The two repeated subprocesses are shown, respectively, in Fig. 7b, c. With each repetition, the family attaining progressively larger local in-plane and out-of-plane amplitudes. When the family completes the three aforementioned subprocesses, the family restarts a horizontal expansion (see the first subplot in Fig. 7d). At this time, however, no out-of-plane amplitude is acquired by the family member as the continuation proceeds. Upon reaching a local maximum in-plane amplitude, the family begins to contract and ultimately converges to EP4. It is noteworthy that the multi-revolution members in the family E5C1 can be classified as the “tadpole orbits” (Van Anderlecht 2016). Characterized by a long-period librational motion and a short-period epicyclical motion, the tadpole orbits are discovered around the two triangular libration points in the CRTBP. In addition, the topological category of the triangular libration points is also $\textit{center}$ $\times$ $\textit{center}$ $\times$ $\textit{center}$, which is the same as that of EP5.

Fig. 7

Progression of PO family E5C1. In each subprocess, the first family member is represented in black, the last member in red, and the intervening members in light blue. The typical family member observed in the progression is highlighted by magenta

The special progression pattern of PO family E5C1 is also reflected in its $\mathcal {C}$–T variation. In Fig. 8, three spikes are observed along the $\mathcal {C}$–T curve before the curve ultimately ends at the energy level of EP4. Note that no overlaps are observed near the spikes and each point on the $\mathcal {C}$–T curve corresponds to a distinct member of the PO family. Each spike, along with its front horizontal segment, corresponds to one subprocess depicted in Fig. 7. The four subprocesses are labeled as “SP” in Fig. 7 and their horizontal segments correspond to the “Lyapunov” family members. In addition, it is remarked that although the family members in the last subplot of Fig. 7d closely resemble the ones of PO family E4C1 (E5C2) in Fig. 5b, the former’s orbital periods are approximately 12 times of the latter’s. The progression pattern of PO family E5C1 should result from its abundant bifurcations, which is further discussed in Sect. 5.2.2.

Fig. 8

$\mathcal {C}$–T variation of PO family E5C1. The black and red pentagrams correspond to the $\mathcal {C}$ and T values of the first and last members in PO family E5C1, respectively

Category B: Families reaching Ryugu’s surface

In this category, the continuation of a PO family starts from an EP and terminates when a family member reaches Ryugu’s surface. While technically the continuation can proceed by allowing a PO going through the asteroid, the POs with intersections with Ryugu are neglected. Thus, the last member of these family corresponds to the first PO touching Ryugu’s surface in the continuation, and is highlighted in red in Fig. 9. It is worth noting that PO families in this category all emerge from four “original” EPs, i.e., the EPs with no “genesis event.” Considering the location and topological category, the “original” EPs, which include EP1, EP2, EP3, and EP6, should have connection with the four exterior EPs of the homogenous triaxial ellipsoid model discussed by Romanov and Doedel (2012). A same conclusion is also drawn in the analysis of the PO families around Bennu (Brown and Scheeres 2023a).

Seven PO families are included in this category and represented in Fig. 9, which includes three PO families emanating from EP1, PO family E2C2 and E3C3, and two PO families emanating from EP6. Only PO Families E1C2 and E6C1 are “Lyapunov,” and the rest PO families are all “vertical.” For the two vertical PO families of EP1, E1C1 and E1C3, although the structure of both families resembles each other and both families include a large portion of identical members, the progression of family E1C1 starts with almost-horizontal members. When a local maximum in-plane amplitude is reached, the family begins contracting in the x and y directions and expanding vertically. The family members related with the aforementioned process are highlighted in magenta in Fig. 9b. Such a phenomenon should resort to the continuation of E1C1 is initialized by an eigenvector with zero z and $\dot{z}$ elements. As the continuation proceeds, the continuation of the family switches onto the solution branch of family E1C3. The family E1C3 is initialized by an eigenvector with out-of-plane components, and the whole family is completely “vertical.” In addition, although the radial distance of the four “original” EPs with respect to Ryugu’s surface is close, only the members in “vertical” families of EP1 and EP3 can realize a full coverage of Ryugu’s z-amplitude. The structure of the two “vertical” families should have a underlying relation with the topology of their corresponding EPs, since both EP1 and EP3 belong to the category center $\times$ center $\times$ center.

Fig. 9

Initial PO families reaching Ryugu’s surface. The EPs from which these families emerge from are represented by magenta points. The last members in these families are represented in red and the intervening members in light blue

Category C: Families exhibiting a cyclic property

In this category, the continuation of a PO family exhibit a cyclic property. Only the family E3C1 belong to this category and its $\mathcal {C}$–T variation is utilized to showcase the cyclic nature in the family’s progression. As shown in Fig. 10, the whole continuation process is divided into six subprocesses (abbreviated to “SP” in the labels of the figure). Upon completing the 6th subprocess, the continuation would proceed by undergoing the six subprocesses in reverse order. Graphically, the continuation begins at the black pentagram on the $\mathcal {C}$–T curve, progresses to the red pentagram, and then retraces its path along the same $\mathcal {C}$–T curve back to the black pentagram. Different from the families in the Category A, the family E3C1 fails to connect two exterior EPs. Additionally, its family members do not reach the asteroid’s surface. Thus, this family stands out as a distinct category in this research.

Fig. 10

$\mathcal {C}$–T variation of PO family E3C1. The black and red pentagrams correspond to the $\mathcal {C}$ and T values of the first and last members in PO family E3C1, respectively

As shown in Fig. 10, each subprocess in the $\mathcal {C}$–T curve consists of a horizontal segment followed by a spike-shaped segment. The horizontal segments correspond to “Lyapunov” family members which resemble the POs depicted in the subplots of the first column in Fig. 7. Family members associated with the spike-shaped segments in the six subprocesses are represented in Fig. 11. In the 1st subprocess (see Fig. 11b), vertical family members are continued from a “Lyapunov” PO. As the out-of-plane amplitude of the vertical members increases, they contract in both x and y directions. Upon a maximum z amplitude is achieved, the vertical POs re-expand in x and y directions. Meanwhile, their out-of-plane amplitude keeps on decreasing until a horizontal PO is reached. As shown in Fig. 11c, e, the family progression in the 3rd and 5th subprocesses share a similar variation as the one in the 1st subprocess, which starts from a “Lyapunov” PO, transforms into a set of POs with a different shape, and finally changes back into a “Lyapunov” PO. The difference lies in the fact that, in the 3rd subprocess, multi-revolution vertical POs with a large z-direction coverage are acquired, while in the 5th subprocess, vertical tadpole POs with a limited z-direction coverage are achieved. The family progression of the 2nd, 4th, and 6th subprocesses shown in Fig. 11b, d, and f is similar to each other. In terms of the orbits’ projection on the xy-plane, the family progression starts from a “Lyapunov” PO, expands in the x and y directions, contracts when a maximum in-plane coverage is reached, and finally ends at another “Lyapunov” PO. The difference between these three subprocesses is that the out-of-plane amplitude of the family members in each subprocess grows as the corresponding orbital period increases. For the members in the 6th subprocess, a maximum z-amplitude of 0.3 km is reached. The existence of these subprocesses can be related with the PO family bifurcations of the family E3C1 and will be discussed in Sect. 5.2.2.

Fig. 11

Initial PO family E3C1. The first and last members in each subprocess are represented in black and the intervening members in light blue. The color red is used to highlight the member with the largest out-of-plane or smallest in-plane amplitude in a subprocess. A family member with a typical shape is represented in magenta

5 Bifurcated orbit families

In this section, the method to detect bifurcations of a PO family based on its linear stability is presented. The bifurcated orbit families from the initial PO families are subsequently illustrated in different categories and the associated findings are discussed.

5.1 Methodology

5.1.1 Linear stability of a periodic orbit

The bifurcation of a PO family is closely associated with the change of its stability properties. The linear stability of a PO can be determined from the eigenvalues of its monodromy matrix $\varvec{\Phi }_T$, which is the state transition matrix (STM) integrated over one orbital period T for the PO. It is proved that, for an autonomous Hamiltonian system, the eigenvalues of its associated monodromy matrix $\varvec{\Phi }_T$ can be grouped into a pair of unity eigenvalues and two reciprocal or conjugate pairs (Koon et al. 2000). By eliminating the two unity eigenvalues, the six-dimensional monodromy matrix can be reduced to a four-dimensional linearized Poincaré map $\varvec{\Phi }_T^{R}$ (Scheeres 2016), which further enables the determination of a PO’s stability using two parameters $\alpha$ and $\beta$ related to the Broucke stability diagram (Broucke 1969). Necessary concepts and equations to achieve $\varvec{\Phi }_T^{R}$ are provided in the subsequent content.

The reduction from $\varvec{\Phi }_T$ to $\varvec{\Phi }_T^{R}$ is realized through the utilization of the Jacobi constant constraint $\mathcal {C}$ in Eq. (5) and the Poincaré section constraint $\mathcal {S}$. A Poincaré section is the intersection of a PO in the phase space of a dynamical system with a lower-dimensional subspace which is transversal to the flow of the system (Koon et al. 2000). Let $p_{\text {PS}}$ denote the position coordinate corresponding to a Poincaré section and $v_{\text {PS}}$ denote the value of the coordinate. A typical Poincaré section can be expressed as:

$$\begin{aligned} \mathcal {S}(\varvec{X}) = \hat{\varvec{s}}^{T} \varvec{r} - v_{\text {PS}} \end{aligned}$$

(18)

where $\hat{\varvec{s}}$ is a 3 $\times$ 1 standard basis vector with the $p_{\text {PS}}$th element equal to one. The linearized Poincaré map $\varvec{\Phi }_T^{R}$ established on the Poincaré section constraint and Jacobi constant constraint can be given as:

$$\begin{aligned} \varvec{\Phi }_T^{R} = \varvec{I}^R \; {\varvec{D}}_{\mathcal {S}}\;\varvec{\Phi }_T \;([\varvec{I}^R]^\textrm{T} +\varvec{D}_{\mathcal {C}} ) \end{aligned}$$

(19)

where $\varvec{I}^R$ denotes a 4 $\times$ 6 matrix acquired by removing the $p_{\text {PS}}$th and $(p_{\text {PS}}$+3)th rows from an identity matrix $\varvec{I}_{6\times 6}$. ${\varvec{D}}_{\mathcal {S}}$ and ${\varvec{D}}_{\mathcal {C}}$ are two derivative matrices related to the two constraints:

$$\begin{aligned} \begin{aligned} {\varvec{D}}_{\mathcal {S}}&= \varvec{I}_{6 \times 6} - \frac{1}{\hat{\varvec{s}}^\textrm{T} \varvec{v}_T} \varvec{f}(\varvec{X}_T)\left[ \begin{array}{c} \hat{\varvec{s}} \\ {\textbf {0}}_{3 \times 1} \end{array}\right] ^\textrm{T} \\ {\varvec{D}}_{\mathcal {C}}&= -\frac{1}{\hat{\varvec{s}}^\textrm{T} \varvec{v}_0}\left[ \begin{array}{c} {\textbf {0}}_{3 \times 1} \\ \hat{\varvec{s}} \end{array}\right] \left( \left. \varvec{I}^R \; \frac{\partial \mathcal {C}}{\partial \varvec{X}}\right| _{\varvec{X}_0}\right) ^\textrm{T} \end{aligned} \end{aligned}$$

With the knowledge of matrix $\varvec{\Phi }_T^{R}$, the two parameters $\alpha$ and $\beta$ to determine a PO’s linear stability can be achieved from the following equations (Howard and MacKay 1987):

$$\begin{aligned} \alpha= & {} \text {Tr} \left( \varvec{\Phi }_T^{R} \right) \nonumber \\ \beta= & {} \frac{1}{2} \left( \alpha ^2 - \text {Tr} \left( \left[ \varvec{\Phi }_T^{R} \right] ^2 \right) \right) \end{aligned}$$

(20)

A PO is stable when the following inequalities are satisfied: $8|\alpha | \le 4\beta - 8 \le \alpha ^2 \le 16$ (Scheeres 2016).

5.1.2 Bifurcation detection method

At a bifurcation point, the behavior and properties of a PO family changes qualitatively (Seydel 2009). As mentioned previously, the bifurcation of a PO family can be identified when the linear stability of the family changes. The stability change can be further linked with the appearance of some specific eigenvalues of the matrix $\varvec{\Phi }_T^{R}$. In the work of Campbell (Campbell 1999), several typical bifurcations and their corresponding eigenvalue pairs are listed: $(+1,+1)$ for the tangent bifurcation, $(-1,-1)$ for the period-doubling (2T) bifurcation, $(e^{+\text {i}\frac{2\pi }{3}},e^{-\text {i}\frac{2\pi }{3}})$ or $(e^{+\text {i}\frac{4\pi }{3}},e^{-\text {i}\frac{4\pi }{3}})$for the period-tripling (3T) bifurcation (TB), $(e^{+\text {i}\frac{\pi }{4}},e^{-\text {i}\frac{\pi }{4}})$ or $(e^{+\text {i}\frac{3\pi }{4}},e^{-\text {i}\frac{3\pi }{4}})$ for the period-quadrupling (4T) bifurcation. To identify these different types of bifurcations from an achieved PO family, the intersections between the $\alpha$-$\beta$ curve of the family and the following curve/lines are solved using the bisection method:

$$\begin{aligned} c_{SH}= & {} \beta -\frac{1}{4}\alpha ^2 -2 \nonumber \\ c_{TB}= & {} \beta -2\alpha +2 \nonumber \\ c_{2T}= & {} \beta +2\alpha +2 \nonumber \\ c_{3T}= & {} \beta + \alpha -1 \nonumber \\ c_{4T}= & {} \beta -2 \end{aligned}$$

(21)

The curve and lines defined in the equation above compose the boundaries for the stability region in the $\alpha$-$\beta$ space. The subscripts of symbol c on the left-hand side of the equation differentiates the boundary related with different bifurcation points. The subscript “SH” represents the secondary Hopf bifurcation. Once a bifurcation point is detected, the corresponding PO member with an initial state $\varvec{X}_{\text {bf}}$ and period $T_{\text {bf}}$ can be utilized to initialize a continuation process to acquire the bifurcated PO family. The initialization follows the same fashion in Eq. (11). In this case, ${\varvec{{X}}}_0^{(0)}$ is selected as $\varvec{X}_{\text {bf}}$. The period value ${\textit{T}}^{(0)}$ is estimated by $nT_{\text {bf}}$ for the nT bifurcation and $T_{\text {bf}}$ for the tangent and Hopf bifurcation. In addition, $\frac{\varvec{X}_0^{(0)}}{||\varvec{X}_0^{(0)}||}$ is selected as the real part of the eigenvector $\nu _{c}$ associated with the typical aforementioned eigenvalues. Empirically, $\frac{\text {d}{} \textit{T}}{\text {d}s}|_{\textit{T} = \textit{T}^{(0)}}$ can be given as zero when a pseudo-arclength step $\delta s$ is sufficiently small (Gómez et al. 1991). For the continuation of bifurcated PO families around Ryugu, $\delta s$ is set to 10$^{-4}$ in the normalized unit for the tangent and Hopf bifurcation. For the PO families from nT bifurcations, $\delta s$ is set to 10$^{-6}$ for a better convergence performance of the Newton’s iteration.

5.2 Numerical simulation of bifurcated PO families

Using the pseudo-arclength continuation method elaborated in Sect. 4.1, the PO families bifurcated from the initial PO families are computed. The achieved bifurcated families are grouped in terms of the two kinds of initial PO families they emerge from and separately represented in the following content. The bifurcated families are named after their corresponding initial families, with a format of “DPO/DRO/EiCj-BmSn.” The string in front of the hyphen denotes the original family from which a bifurcated family originates, and the string behind the hyphen is composed by two parts. The first part “Bm” represents the type of bifurcation corresponding to the achieved family. For a tangent bifurcation, m = 1; for a nT bifurcation, m = n. The second part “Sn” represents the nth family detected for the “Bm” type of bifurcation during the progression of an initial family. To differentiate from the naming of bifurcated families, the letters in a bifurcated family are removed and only the numbers are left to denote a bifurcation point. For instance, the bifurcated family “E3C1-B4S1” is the first 4T bifurcation family identified from the progression of initial family E3C1, and “BP-3141” denotes the bifurcation point where the family progresses from.

Three special-case scenarios are encountered during the generation of the bifurcated families. Although the secondary Hopf bifurcation points are detected in the simulation, it is verified that the corresponding initial conditions cannot be applied to generate new bifurcated PO families (Campbell 1999). In addition, some PO families originating from the tangent bifurcation points are found to be a portion of its corresponding initial family. These bifurcated families are not illustrated in this work and only the one with a structure different from that of its initial family is reported. Lastly, some bifurcation states are discovered to be situated too close to the asteroid’s surface. The corresponding families are discarded when their progression is very limited, or they cannot be continued due to numerical issues.

5.2.1 Bifurcated PO families from distant retrograde/prograde orbit families

Families bifurcating from the DRO family

Ten bifurcated PO families from the DROs are identified and their general properties are summarized in Table 6. During the progression of the DRO family, the orbital periods of family members are decreasing as the family gradually approaches the asteroid’s surface. Hence, a same decreasing tendency is also observed in the orbital periods of the families belonging to a same bifurcation type, as shown in the row of $T_{{\textbf {min}}}$ and $T_{{\textbf {max}}}$ in Table 6. Moreover, according to the row of $\mathcal {C}_{{\textbf {min}}}$ and $\mathcal {C}_{{\textbf {max}}}$ in Table 6, the bifurcated families originating from the DROs exhibit high energy levels, which is evidenced by the presence of family members with positive Jacobi constants among all bifurcated families. It is worth noting that only one stable family, DRO-B2S3, is identified among the ten bifurcated families.

Table 6 Properties of bifurcated PO families originating from DROs

The four “Lyapunov” bifurcated PO families originating from DROs around Ryugu, DRO-B2S3, DRO-B2S4, DRO-B3S2, and DRO-B4S2, are shown in Fig. 12. Their associated bifurcation types can be identified directly from the number of revolutions achieved by their family members. The z-amplitude of all the families are restricted within 50 m. The first and last members of each family are highlighted in black and red, respectively, in Fig. 12. The continuation of the four families terminates when their respective family members reach specific equatorial regions. Among these families, three of them achieve their largest extension in the x and y directions when their family member reaches the asteroid’s surface.

Fig. 12

Lyapunov PO families bifurcated from DRO family. In each family, the first family member is represented in black, the last member in red, and the intervening members in light blue

The six “vertical” PO families bifurcating from DROs around Ryugu, DRO-B2S1, DRO-B2S2, DRO-B3S1, DRO-B3S3, DRO-B4S1, and DRO-B4S3, are shown in Fig. 13. Although the out-of-plane amplitude achieved by the members in the DRO family is quite limited, the six “vertical” bifurcated families are characterized by manifest extension in the z direction. Starting from a horizontal member, these six families progress spatially before finally reaching the asteroid’s surface. Two bifurcated families, DRO-B3S1 and DRO-B4S1, progress firstly in the $+z$ direction before advancing in the $-z$ direction and reaching Ryugu’s surface. According to Fig. 13, a larger z extension can be reached when a family progresses from a member with a larger coverage in x and y directions.

Fig. 13

Vertical PO families bifurcated from DRO family. In each family, the first family member is represented in black, the last member in red, and the intervening members in light blue

Families bifurcating from the DPO family

Fourteen bifurcated PO families from the DPO family are identified and their general properties are summarized in Table 7. The bifurcated families of the same bifurcation type demonstrate an increasing trend in orbital periods as the DPO family progresses toward Ryugu’s surface. Such a trend is opposite to the tendency observed in the bifurcated families originating from the DROs. These bifurcated orbit families are characterized by negative Jacobi constants, which indicates a low energy level in comparison with the bifurcated families of the DPOs. In addition, four of these families are completely unstable.

Table 7 Properties of bifurcated PO families originating from DPOs

Five “Lyapunov” bifurcated PO families originating from DPOs around Ryugu, DPO-B2S2, DPO-B3S2, DPO-B3S4, DPO-B4S2, and DPO-B4S4, are shown in Fig. 14. Similar to the “Lyapunov” bifurcated families from DROs, their associated bifurcation types can be identified from the number of revolutions achieved by their family members. However, it is observed that some of these families can reach multiple equatorial regions before the continuation terminates, such as family DPO-B3S4 and DPO-B4S4. Moreover, all of these families achieve their largest extension in the xy-plane upon their family members reach Ryugu’s surface.

Fig. 14

Lyapunov PO families bifurcated from DPO family. In each family, the first family member is represented in black, the last member in red, and the intervening members in light blue

Eight “vertical” PO families bifurcating from the DPOs are shown in Fig. 15. The family DPO-B4S6 is discovered to be a portion of family DPO-B4S5 and thus is not demonstrated in the figure. As demonstrated in the plots, most “vertical” bifurcated families reach the asteroid’s surface and achieve their largest expansion in z direction at the end of the continuation. By comparison, two distinctive families are family DPO-B3S3 and DPO-B4S5. These two families display a cyclic property in their family progression. As shown in Fig. 15c, the family DPO-B3S3 starts from a horizontal member, reaches its maximum z-amplitude, and progresses back to the xy-plane. Members in the family DPO-B4S5 gradually reach their largest expansion in z direction (see the red orbit in Fig. 15g) in the continuation and progress back to its initial member. In addition, a noteworthy family is DPO-B4S3, whose members display a rotational symmetry in their shape and simultaneously cover a wide range in the z direction.

Fig. 15

Vertical PO families bifurcated from DPO family. In each family, the first family member is represented in black, the last member in red, and the intervening members in light blue

5.2.2 Bifurcated PO families from the initial EP orbit families

PO families bifurcated from the initial PO families emanating from the EPs are listed in Table 8. For Ryugu, no obvious relationship is identified between the number of bifurcated families or bifurcation types and the existing categories defined in Sect. 4.2.2. Since the occurrence of bifurcation is directly linked to the stability change of a PO family, the stability of the initial PO families is also provided in Table 8. As shown in the table, there is no bifurcated families originating from the three initial PO families, E2C2, E3C3, and E6C1, which are completely stable or unstable. In addition, only one bifurcated family originating from the tangent bifurcation, E1C1-B1S2, is discovered to be different from its initial PO family, while the remaining families belonging to the tangent bifurcation are all validated to be a portion of their initial PO families and not separately illustrated in this work.

Table 8 Bifurcated PO families from the initial EP PO families

To visualize the relation between the solution branches of initial PO families emanating from the EPs and their bifurcation points, a solution map is depicted in Fig. 16. Such a solution map is inspired by the pioneering work of Hénon (1969), which investigated the planar PO families around the secondary in the Hill’s three-body problem. Unlike Hénon’s solution map, which includes only the solution branches of the planar PO families, our solution map incorporates both two- and three-dimensional PO families are included, along with their Jacobi constant. In this solution map, each PO’s centroid is computed, and the argument of a PO’s centroid $\theta$ is selected as the x-axis of the solution map, while the planar radius of a PO’s centroid r serves as the y-axis. The maximum absolute value of z-coordinate along a PO, denoted as $|z|_\text {max}$, is chosen as the z-axis. This representation enables the identification of whether an initial family is characterized by “vertical” members. In addition, Ryugu’s six exterior EPs and ridge line are included as well, represented by red solid spheres and a red dashed line, respectively. It is evident from the figure that the initial PO families originate from the EPs located on the ridge line. Bifurcation points, labeled as “BP” in the figure, are represented by magenta diamonds. It should be noted that the families E3C1 and E5C1, along with their bifurcation points, are excluded from Fig. 16 to avoid unclear illustration. The bifurcations of these two families are separately discussed in the following content.

Fig. 16

Solution map of initial PO families and their bifurcation points

Different from the other initial PO families, the PO families E3C1 and E5C1 are distinguished by a rich abundance of bifurcation points. Such a fact is evident from their stability diagrams in Fig. 17, where the blue curve represents the variation of $\alpha$ and $\beta$ values during the families’ progression. The curve and lines defined in Eq. (21) to delineate the boundaries of stability region are depicted with solid lines. It is noteworthy that connections are identified between the detected bifurcations in the two families and the subprocesses of the two families’ progression discussed in Sect. 4.2.2. For each spike shown in the $\mathcal {C}$–T plots in Figs. 8 and 10, the tip of a spike corresponds to a tangent bifurcation point of the family’s $\alpha$-$\beta$ curve in the stability diagram. These tangent bifurcation points are highlighted by magenta diamonds in Fig. 17. According to the stability diagram, it is highly probable that during the continuation of these two families, switches between different family branches take place multiple times, ultimately resulting in a prolonged progression of these two families.

Fig. 17

Stability diagrams of initial PO families E3C1 and E5C1

The bifurcated PO families originating from different initial EP orbit families are further illustrated. The bifurcated families demonstrated firstly are E2C1-B4S1 and E6C2-B4S1 in Fig. 18, which are associated with the saddle $\times$ center $\times$ center type of EP. These two families start from very close proximity of Ryugu and progress with limited members before they reach Ryugu’s surface. The first and last family members in these two families are highlighted, respectively, in black and red in the figure.

Fig. 18

Bifurcated PO families associated with EP2 and EP6. The first and last family members in these two families are highlighted in black and red, respectively

Fig. 19

Bifurcated PO families associated with EP1. In each family, the first family member is represented in black, the last member in red, and the intervening members in light blue. The typical family member observed in a family is highlighted by magenta

More bifurcated families are identified to be associated with the center $\times$ center $\times$ center type of EP. The bifurcated families associated with EP1 are demonstrated in Fig. 19. The same coloring rule used in Fig. 7 is applied here: the first member in the progression is represented in black, the last in red, and the intervening members in light blue. In addition, for certain families whose members are characterized by multiple revolutions, the intervening members are excluded for clearer illustration. Among these families, the families E1C1-B1S2 in Fig. 19a and E1C2-B4S1 in Fig. 19b exhibit a cyclic property during their progression. In addition, same portion of family members are observed between the two families and family E1C3-B4S1 in Fig. 19c. This phenomenon can be explained by the fact that these families pass through the same bifurcation points as they progress. To clearly explain the three families’ progression, the $\mathcal {C}$–T variation of the families are separately provided in Fig. 20. As shown in Fig. 20a, the family E1C1-B1S2 starts from BP-1112, passes BP-1111 and reaches EP1. It restarts from EP1, passes BP-1111 and BP-1112, reaches BP-1241, moves back to BP-1112 and repeats the aforementioned process. For the family E1C2-B4S1, as shown in Fig. 20b, it starts from BP-1241, passes BP-1112 and BP-1111, and reaches EP1. Subsequently, it passes the bifurcations points reversely to reach BP-1241 and starts its repetition. The family E1C3-B4S1 in Fig. 20c starts from BP-1341, passes BP-1241, and ultimately reach the surface of Ryugu. From the progression of the three families, it can be observed that when passing the same bifurcation point, a PO family is likely to switch onto the same family branch which also exists in another PO family.

Fig. 20

$\mathcal {C}$–T variation of three bifurcated PO families

For the remaining three bifurcated families associated with EP1, the PO family E1C3-B4S2 in Fig. 19d is the only bifurcated family associated with EP1 which is observed to progress from the very close proximity of Ryugu. The two bifurcated families, E1C1-B3S1 and E1C1-B4S1, are characterized by a prolonged orbital period around 100 h. As shown in Figs. 19e and 19f, these two families can extend to a relatively larger region around Ryugu, with family members marked by many revolutions. It is worth noting that, before reaching Ryugu’s surface, family members resembling the Lissajous orbit (Koon et al. 2000) are observed in both families and highlighted in magenta.

Given the abundance of bifurcations associated with families E3C1 and E5C1, not all of the bifurcated PO families are calculated. Instead, the first ten bifurcated PO families from the families E3C1 and E5C1 during their progression are computed. The achieved families are further categorized in terms of the structure of each family. Representatives of each category are selected and their typical family members are demonstrated in Fig. 21 and Fig. 22. It is remarked that these bifurcated families are characterized by orbital periods three or four times larger than those of their associated initial families. In addition, while the achieved bifurcated families possess more revolutions, no significant change in shape is observed for the members in the bifurcated families compared to those in the initial families.

The computed bifurcated PO families from the family E3C1 can be classified into four categories. The first one include families E3C1-B3S1 (see Fig. 21a) and E3C1-B4S2. The continuation of these families exhibit a cyclic property and the structure of these two vertical families are nearly symmetric about the xy-plane. In this category, family members progress from the xy-plane and expands in the z-direction until the member with largest z amplitude is reached. In the second category, three PO families, E3C1-B3S2 (see Fig. 21b), E3C1-B3S5, and E3C1-B4S4, are included. Families are in this category exhibit a similar variation fashion as the ones in the previous category, however, with the expansion and contraction appears very close to the xy-plane. In the third category, there are two families: E3C1-B3S3 and E3C1-B4S1(see Fig. 21c). POs in these two families can be classified as the tadpole orbits and some family members resemble the Lissajous orbit. The progression of these two families also exhibit a cyclic property. The families E3C1-B3S4, E3C1-B4S3 (see Fig. 21d), and E3C1-B4S5 belong to the fourth category. These families are continued until reaching Ryugu’s surface. As “vertical” families, a wide coverage in the x and y directions can also be reached by the associated family members.

Fig. 21

Selected bifurcated PO families associated with family E3C1. In each family, the first family member is represented in black, the last member in red, and the intervening members in light blue. The typical member observed in a family is highlighted by magenta

The computed bifurcated PO families from the family E5C1 can be classified into three categories. The six families in the first category include E5C1-B3S1, E5C1-B3S3 (see Fig. 22a), E5C1-B3S5, E5C1-B4S1 (see Fig. 22b), E5C1-B4S3, and E5C1-B4S5. As shown in Figs. 22a and 22b, the progression of families in this category is same as that of the first category associated with the bifurcated families of the family E3C1. These families are continued from the xy-plane and the z-amplitude of their members increases gradually. Upon reaching the maximum z-amplitude, the families begin contracting and converge back to its first member. In the second category, the families E5C1-B3S4 (see Fig. 22c) and E5C1-B4S4 are included. The two families can be classified as “Lyapunov” and also exhibit a cyclic property during their progression. As shown in Fig. 22c, the family initially expands in the xy-plane from a crescent-shaped member. During the expansion, family members with multiple revolutions are clearly identified. When a locally largest xy-coverage is achieved, the family starts contracting and eventually reaches its first family member. The families in the third category, E5C1-B3S2 and E5C1-B4S2 (see Fig. 22d), displays a different progression pattern. Although the progression of the two “Lyapunov” families has similarities to the that of the precious category, the two families finally converge to the EP4. As shown in Fig. 22d, instead of contracting to its initial member, the family contracts to a member with no obvious feature of multiple revolutions (the dark green PO in Fig. 22d). As the continuation proceeds, the family eventually converges to the EP4. It is noteworthy that, while no apparent multi-revolution feature is characterized by the family members converging to the EP4, their orbital periods are three or four times larger than those of their initial families. Such a fact indicates that the bifurcated families in this category do not overlap with the initial family E5C1.

Fig. 22

Selected bifurcated PO families associated with family E5C1. In each family, the first and last family member is represented in black and the intervening members in light blue. The typical member observed in a family is highlighted by magenta

6 Comparison of dynamical environment between Ryugu and Bennu

In this section, a comparison is made between Ryugu and Bennu regarding the dynamical environment around the two asteroids. The dynamical environment about Bennu in an asteroid body-fixed frame are thoroughly investigated in Brown and Scheeres’ works (Brown and Scheeres 2023b, a), which serves as the primary reference for this comparison. Different from the comparison made in the work by Scheeres et al. which focuses on the orbital and surface environments of both asteroids (Scheeres et al. 2019), in this work, the identified similarities and differences between the two asteroids are summarized from two major aspects: (1) the evolution of EPs and (2) the progression and structure of PO families.

6.1 Comparison of EP’s evolution

To provide a comparison of the EP’s evolution between Ryugu and Bennu, the EP’s evolution with respect to Ryugu’s different spin rates quantified by the scaling factor $\kappa$ is calculated and demonstrated in Fig. 23. Employing the same illustration approach as shown in Fig. 6a, in this figure, the scaling factor $\kappa$ is further decreased to achieve the EPs located very close to Ryugu’s surface. It is observed that six additional EPs emerge from three “genesis events.” The six EPs are indexed anticlockwise from EP7 to EP12 based on their appearance as the spin rate increases. According to their associated “genesis events,” they can be divided into three pairs, each comprising one EP of the type $\textit{spiral}$ $\times$ $\textit{spiral}$ $\times$ $\textit{center}$ and another EP of the type $\textit{saddle}$ $\times$ $\textit{center}$ $\times$ $\textit{center}$. Their topological properties remain unchanged as Ryugu’s spin rate varies. A similar unaltered topology is also observed for EP2, EP4, and EP6, which remain the type of $\textit{saddle}$ $\times$ $\textit{center}$ $\times$ $\textit{center}$ during the evolution. In contrast, the topology of EP1, EP3, and EP5 transforms from $\textit{center}$ $\times$ $\textit{center}$ $\times$ $\textit{center}$ to $\textit{spiral}$ $\times$ $\textit{spiral}$ $\times$ $\textit{center}$ when $\kappa$ is decreased to around 0.57.

Fig. 23

EPs’ evolution with respect to different spin rates of Ryugu. The scaling factor $\kappa$ is decreased from 3.0 to 0.5 to achieve the EPs very close to Ryugu’s surface. The ridge line computed at the reference spin rate (which corresponds to $\kappa =1.0$) is highlighted in magenta

By comparison with Fig. 1 and associated description in Brown and Scheeres’ work (Brown and Scheeres 2023b) which illustrating the evolution of Bennu’s EPs at different spin rates, the following findings are summarized. Although the EP branches are located diversely for each asteroid, several similarities are identified for the EPs between the two asteroids. Firstly, at relative low spin rates, there are four EPs around both asteroids. The four EPs’ topological classifications alternate between $\textit{center}$ $\times$ $\textit{center}$ $\times$ $\textit{center}$ and $\textit{saddle}$ $\times$ $\textit{center}$ $\times$ $\textit{center}$. In this scenario, the four EPs are located relatively far away from the asteroids and not strongly influenced by their irregular gravity field. Their existence can be interpreted in terms of the four external EPs in the homogenous triaxial ellipsoid model (Romanov and Doedel 2012). Secondly, as the asteroid’s spin rate increases, additional EPs emerge from the “genesis events” around the two asteroids. Four “genesis events” are observed around both asteroids, and the locations where these events occur should be attributed to the combined effects of each asteroid’s topology and spin rate. Furthermore, the EP branches situated very close to the asteroid’s surface are identified for both asteroids. These EPs are highly sensitive to the asteroid’s surface topology and are likely to change with the slight nuances on the surface. Thirdly, the same alteration and fixation of EPs’ topology is identified for both asteroids. For instance, the topology of a $\textit{center}$ $\times$ $\textit{center}$ $\times$ $\textit{center}$ EP is transformed to $\textit{spiral}$ $\times$ $\textit{spiral}$ $\times$ $\textit{center}$ as the spin rate increases. The EPs of the type “unstable center” (which includes $\textit{spiral}$ $\times$ $\textit{spiral}$ $\times$ $\textit{center}$ and $\textit{saddle}$ $\times$ $\textit{center}$ $\times$ $\textit{center}$) remain unchanged when the spin rate alters. In addition, for both asteroids, the topology of their EPs are identified to belong to the aforementioned three categories.

6.2 Comparison of PO families

The PO families around Bennu in a body-fixed frame are discussed extensively by Brown and Scheeres (2023a) and thus employed for the comparison of PO families between Bennu and Ryugu. Before delving into the comparison, it is remarked that, although the initial and bifurcated PO families are investigated using the same continuation method and stability analysis method in our research and theirs, a significant difference is that, in Brown and Scheeres’ research, some PO families are further continued even after their members reach Bennu’s surface. Such an approach results from the close proximity of Bennu’s EPs with respect to its surface. Instead of terminating the orbit continuation at the point of surface intersection and demonstrating seemingly unrelated PO families, their method to continue the families helps to reveal the underlying relation between the PO families and provide further insight into their structure. In contrast, with a slower spin rate, the EPs of Ryugu are located relatively further away from its surface. Such a fact enables sufficient expansion of the PO families and allows the appearance of typical progression patterns and structure. In addition, in this research, the initial families reaching Ryugu’s surface are also further continued beyond the point of surface intersection. However, no significant connections or progression patterns are observed from the extended continuation. Therefore, the corresponding continuation is terminated at Ryugu’s surface and the associated PO is defined as the last member of the family.

The first comparison between Bennu and Ryugu is made between their DRO/DPO and their bifurcated families. Since the continuation of these families around Ryugu is terminated upon reaching Ryugu’s surface, the comparison is limited to the corresponding portion. Similarities in multiple aspects are identified. Firstly, for both asteroids, their DRO families are characterized by a smaller out-of-plane amplitude than the corresponding DPO families when approaching the asteroid’s surface. Secondly, nearly symmetrical structure are observed in some portions of both the DPO/DRO and some bifurcated families (e.g., DRO-B4S3 in Fig. 13f and DPO-B4S3 in Fig. 15f) around both asteroids. Thirdly, the POs with similar shapes are identified in the bifurcated families of both asteroids, e.g., the typical shapes of the member reaching the asteroid’s surface in the family DRO-B2S1 in Figs. 13a and DPO-B3S4 in 15c. However, it is remarked that the extension of the bifurcated families from DRO/DPO around Ryugu is generally larger than those around Bennu. The aforementioned similarities in the DRO/DPO and their bifurcated families can be understood from the fact that the asteroid’s topology and the resulting irregular gravity field do not exert a strong influence to the DRO/DPO families until their members approach the close vicinity of the asteroid. The two asteroids can be even treated as identical homogenous triaxial ellipsoids when a PO is located sufficiently far away. Thus, similar family structures and orbit shape is observed in the corresponding families around both asteroids.

As for the comparison of the PO families emanating from the EPs, the similarities and differences are summarized in terms of the initial families and the associated bifurcated families. For the initial families, the candidates converging to an EP (which are classified in Category A in Sect. 4.2.2) are observed for both asteroids. For some families in this category, the evolution in the eigenstructure resulting from the “genesis events” can explain their presence. However, a counterpart of the family E2C1 (E3C2) in Fig. 5a cannot be found around Bennu. Similarly, the counterpart of the PO family connecting the EP1 and EP6 around Bennu cannot be found around Ryugu. In addition, a PO family whose progression is similar to that of the family E5C1 shown in Fig. 7 is not identified around Bennu. Initial families with abundant bifurcations, e.g., the families E3C1 and E5C1, are not found as well around Bennu.

When considering the bifurcated families associated EPs, it is noticeable that Ryugu has more families of this kind than Bennu, due to the existence of abundant bifurcation points in the families E3C1 and E5C1. Similarities are identified between these bifurcated PO families. Different bifurcated families connected by the same bifurcation points are observed around Bennu. Such a phenomenon is also spotted for Ryugu. As shown in Fig. 20, three bifurcated families associated with EP1 are found to pass the same bifurcation points and include a portion of identical family members. However, the cyclic property exhibited by some bifurcated PO families around Ryugu (e.g., the families E1C1-B1S2 and E1C2-B4S1) is not reported for Bennu. From the comparison, it is obvious that the PO families emanating from the asteroids’ EPs are more sensitive to the irregular gravity field of the asteroid. Thus, even the comparison is between the PO families, the effect from the EPs should not be neglected. The different topological classifications of EPs around the two asteroids determine different numbers of PO families emanating from them, and the different distances of ridge lines with respect to the two asteroids further influence the progression of the PO families.

7 Conclusion

The dynamical environment around the asteroid Ryugu is analyzed using a constant-density polyhedron model defined in a body-fixed rotating frame. Six exterior equilibrium points (EPs) fitting into two topological classifications are identified along the ridge line around Ryugu. The distant retrograde/prograde orbit (DPO/DRO) families around Ryugu are computed and the PO families bifurcating from both families are analyzed, with ten bifurcating from the DRO family and fourteen from the DPO family. Fifteen PO families emanating from Ryugu’s EPs are calculated and further classified into three categories based on their progression pattern. The first category consists of seven PO families converging to an EP, the second comprises seven PO families reaching Ryugu’s surface, and the third entails a PO family exhibiting a cyclic property during its progression. The presence of PO families in the first category could be explained by the bifurcation of a degenerate EP. The similarity between these families and analogous ones in the circular restricted three-body problem (CRTBP) is also examined. Twenty-five bifurcated PO families associated with Ryugu’s EPs are computed and analyzed. Different bifurcated families are discovered to be connected by same bifurcation points. The bifurcated families originating from two initial PO families rich in bifurcation points are further categorized.

By comparing the dynamical environments of Ryugu and Bennu, similarities and differences are identified in the evolutions of their EPs and the progression of their PO families. Four independent EPs are identified for both asteroids at relative low spin rates. The bifurcation of EP branches from a degenerate EP is observed for both asteroids as their spin rates increase. Although PO families with similar shapes are identified around both asteroids, the PO families emanating from the EPs of Ryugu fall into more categories, and more bifurcated families are detected around Ryugu.