Generating Functions for Local Symplectic Groupoids and Non-perturbative Semiclassical Quantization

Abstract

This paper contains three results about generating functions for Lie-theoretic integration of Poisson brackets and their relation to quantization. In the first, we show how to construct a generating function associated to the germ of any local symplectic groupoid and we provide an explicit (smooth, non-formal) universal formula $S_\pi$ for integrating any Poisson structure $\pi$ on a coordinate space. The second result involves the relation to semiclassical quantization. We show that the formal Taylor expansion of $S_{t\pi }$ around $t=0$ yields an extract of Kontsevich’s star product formula based on tree-graphs, recovering the formal family introduced by Cattaneo, Dherin and Felder in [6]. The third result involves the relation to semiclassical aspects of the Poisson Sigma model. We show that $S_\pi$ can be obtained by non-perturbative functional methods, evaluating a certain functional on families of solutions of a PDE on a disk, for which we show existence and classification.

Appendix: Kontsevich Graphs, Butcher Series and Networks

In this Appendix, we first recall basic definitions involving Kontsevich graphs and symbols as well as of Butcher series parameterized by rooted trees. In Sect. A.3, we introduce a certain type of graphs built from rooted trees which we call “networks of rooted trees” and define associated symbols. These networks make the bridge between certain Butcher series expressions for generating functions to the Kontsevich-trees generating function (33), as explained in Sect. 4.3.

1.1 Kontsevich trees and their symbols

A Kontsevich graph of type (n, m) is a graph (V, E) whose vertex set is partitioned in two sets of vertices $V=V^{a}\sqcup V^{g}$, the aerial vertices $V^{a}=\{1,\dots ,n\}$ and the terrestrial vertices $V^{g}=\{\bar{1},\dots ,\bar{m}\}$ such that

• all edges start from the set $V^{a}$,

• loops are not allowed,

• there are exactly two edges going out of a given vertex $k\in V^{a}$,

• the two edges going out of $k\in V^{a}$ are ordered, the first (“left”) one being denoted by $e_{k}^{1}$ and the second (“right”) one by $e_{k}^{2}$.

An aerial edge is an edge whose end vertex is aerial, and a terrestrial edge is an edge whose end vertex is terrestrial. We denote by $G_{n,m}$ the set of Kontsevich graphs of type (n, m).

Given a Poisson structure $\pi$ and a Kontsevich graph $\Gamma \in G_{n,m}$, one can associate a m-differential operator $B_{\Gamma }(\pi )$ on ${\mathbb {R}}^{n}$ in the following way: For $f_{1},\dots ,f_{m}\in C^{\infty }({\mathbb {R}}^{n})$, we define

$$\begin{aligned} B_{\Gamma }(\pi )(f_{1}\dots ,f_{m}):=\sum _{I:E_{\Gamma }\rightarrow \{1,\dots ,d\}}\big [\prod _{k\in V_{\Gamma }^{a}}(\prod _{\begin{array}{c} e\in E_{\Gamma }\\ e=(*,k) \end{array} }\partial _{I(e)})\pi ^{I(e_{k}^{1})I(e_{k}^{2})}\big ]\prod _{i\in V_{\Gamma }^{g}} \big (\prod _{\begin{array}{c} e\in E_{\Gamma }\\ e=(*,i) \end{array} }\partial _{I(e)}\big )f_{i}. \end{aligned}$$

The symbol $\hat{B}_{\Gamma }$ of $B_{\Gamma }$ is defined by

$$\begin{aligned} B_{\Gamma }(e^{p_{1}x},\dots ,e^{p_{m}x})=\hat{B}_{\Gamma }(p_{1},\dots ,p_{m})e^{(p_{1} +\dots +p_{m})x}. \end{aligned}$$

Example A.1

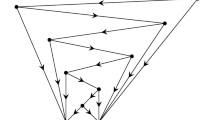
Figure 2b shows an example of a Kontsevich graph $\Gamma \equiv \Gamma _\rho$ of type (n, 2). The corresponding symbol is given by

$$\begin{aligned} \hat{B}_\Gamma (\pi )(p_1,p_2) = \pi ^{k_1k_2} \ \pi ^{j_1j_2} \ \partial _{k_2}\partial _{j_1}\pi ^{i_1i_2} \ \partial _{k_1}\pi ^{l_1l_2} \ p_{1i_1}p_{1l_1}p_{2i_2}p_{2l_2}p_{2j_2}. \end{aligned}$$

The order of the arrows (left vs. right arrows) is important because flip**, for example, the order of the first aerial vertex order would introduce a sign, since $\pi ^{ij}=-\pi ^{ji}$.

$T_{n,2}$ is a subset of $G_{n,2}$ that we now define:

Definition A.2

Let $\Gamma \in G_{m,n}$ be a Kontsevich graph. The interior of $\Gamma$ is the graph $\Gamma _{i}$ obtained from $\Gamma$ by removing all terrestrial vertices and terrestrial edges. A Kontsevich graph is a Kontsevich tree if its interior is a tree in the usual sense (i.e. it has no cycles). We denote by $T_{n,m}$ the set of Kontsevich’s trees of type (n, m).

We will not need a detailed presentation of Kontsevich weights $\Gamma \mapsto W_\Gamma \in {\mathbb {R}}$, the reader can find a rough account with the conventions that we follow in this paper in [2, App. A].

1.2 Rooted trees, elementary differentials and Butcher series

A graph is the data (V, E) of a finite set of vertices $V=\{v_{1},\dots ,v_{n}\}$ together with a set of edges E, which is a subset of $V\times V$. The number of vertices is called the degree of the graph and is denoted by $|\Gamma |$. We think of $(v_{1},v_{2})\in E$ as an arrow that starts at the vertex $v_{1}$ and ends at $v_{2}$. Two graphs are isomorphic if there is a bijection between their vertices that respects theirs edges. The set ${\bar{\Gamma }}$ of all isomorphic graphs to a given graph $\Gamma$ is called a topological graph. A symmetry of a graph is an automorphism of the graph (i.e. a relabeling of its vertices that leaves the graph unchanged). The group of symmetries of a given graph $\Gamma$ will be denoted by $E(\Gamma )$. Note that the number of symmetries of all graphs sharing the same underlying topological graph is equal; we define the symmetry coefficient $\sigma ({\overline{\Gamma }})$ of a topological graph ${\overline{\Gamma }}$ to be the number of elements in $E(\Gamma )$, where $\Gamma \in {\overline{\Gamma }}$.

A rooted tree is a graph that (1) contains no cycle, (2) has a distinguished vertex called the root, (3) whose set of edges is oriented toward the root. We will denote the set of rooted trees by RT and the set of topological rooted trees by [RT]. The set of topological rooted trees can be described recursively as follows: The single vertex graph $\bullet$ is in [RT] and if $t_{1},\dots ,t_{n}\in [RT]$ then so is

$$\begin{aligned} t=[t_{1},\dots ,t_{n}]_{\bullet }, \end{aligned}$$

where the bracket is to be thought as grafting the roots of $t_{1},\dots ,t_{n}$ to a new root, which is symbolized by the subscript $\bullet$ in the bracket $[\,,\dots ,\,]$. We can represent graphically topological rooted trees as the $\gamma \equiv \gamma (\rho )=[\bullet ,\bullet ]_\bullet$ depicted in Fig. 2a (without the extra orientation of edges present in that figure).

Remark A.3

Since we are dealing with topological rooted trees, the ordering in $[t_{1},\dots , t_{m}]_{\bullet }$ is not important (for instance, we do not distinguish between $[\bullet ,[\bullet ]_{\bullet }]_{\bullet }$ and $[[\bullet ]_{\bullet },\bullet ]_{\bullet }$).

Let $X=X^{i}(x)\partial _{x^i}$ be a vector field on ${\mathbb {R}}^{d}$. We define the elementary differential of X recursively as follows: For the single vertex tree, we define $D_{\bullet }^{u}X=X^{u}(x)$, and for $t=[t_{1},\dots ,t_{m}]$ in [RT], we define

$$\begin{aligned} D_{t}^{u}X(x)=D_{t_{1}}^{i_{1}}X(x)\cdots D_{t_{m}}^{i_{m}}X(x) \ \partial _{i_{1}}\dots \partial _{i_{m}}X^{u}(x), \end{aligned}$$

(57)

where we used the Einstein summation convention. Similarly, given a smooth function $H:{\mathbb {R}}^d \rightarrow {\mathbb {R}}$ we define recursively for $t=[t_{1},\dots ,t_{m}]$,

$$\begin{aligned} F^{H,X}_{t}(x) = D^{i_1}_{t_1}X(x)\cdots D^{i_m}_{t_m}X(x) \ \partial _{i_1}\dots \partial _{i_m}H (x), \ F^{H,X}_{\bullet }(x)=H(x). \end{aligned}$$

Following [1], one has that the n-th iterated Lie derivative is given by

$$\begin{aligned} L_X^n x^i = \sum _{t \in [ RT], \ |t|=n} \frac{|t|!}{t! \sigma (t)} D^i_t X, \end{aligned}$$

(58)

where the tree factorial is recursively defined as $t!= |t| t_1! \cdots t_k!$ for $t=[t_1,..,t_k]$, $\bullet ! =1$. The symmetry factor $\sigma (t)$ also admits a recursive formula, namely, $\sigma ([t_1^{n_1},..,t_1^{n_k}]) = n_1!\sigma (t_1)^{n_1} ... n_k!\sigma (t_k)^{n_k}$ where the trees $t_1,..,t_k$ are assumed different and the exponent $n_i$ denotes it is repeated $n_i$-times.

Given a map $a:[RT] \rightarrow {\mathbb {R}}$ and a vector field $X\equiv X^i(x)\partial _{x^i}$ on ${\mathbb {R}}^n$ the associated Butcher series is defined by

$$\begin{aligned} B(a, \epsilon X, x) = x + \sum _{t \in [RT]} \frac{\epsilon ^{|t|}}{\sigma (t)} \ a_t \ D_t X |_x. \end{aligned}$$

One can extend this assignment to $B(a, \epsilon X, H)\equiv H|_{y= B(a, \epsilon X, x)}$ for any function $H\equiv H(x)$ by expanding formally around $\epsilon =0$ and using the symbols $F^{H,X}_t$ defined above (see “S-series” in [14]). The reader is referred to the foundational work of Butcher [1] on elementary differentials and the use of trees and Butcher series in ordinary differential equations for more information (see also [14]).

Example A.4

Following [2, Thm. 21], the formal Taylor expansion around $t=0$ of the realization map $\alpha _{t\pi }(x,p)$ defined by Eq. (27) is given by the Butcher series of Eq. (38). The coefficients $t\mapsto c^B_t$ generalize Bernoulli numbers and can be computed by iterated integrals (see [2, Thm. 24]).

1.3 Networks of rooted trees and their symbols

The motivation for introducing networks of rooted trees comes from considering elementary differentials of the form $D_\gamma ^iX$, with $\gamma \in [RT]$ and $X=X^{p_1\alpha }$ a hamiltonian vector field in $M\times M^*$ as in (37) (M is a coordinate space), and substituting the Butcher series (39) in place of $\alpha :M\times M^*\cdot \cdot _{M\times 0}\dashrightarrow M$. The idea is that each derivative in the elementary differential acting on each term of $\alpha$ produces, by the Leibniz rule, a series of terms which can be arranged into these “network” graphs. To account for the two terms in the hamiltonian vector field $X^{p_1\alpha }$ we consider an extra orientation on the (“skeleton”) edges of $\gamma$, which tells us which term is acting.

With these considerations, a network of rooted trees $\rho \in NRT$ consists of the following data:

• a rooted tree $\gamma \equiv \gamma (\rho )\in RT$, called the skeleton of $\rho$, endowed with an additional orientation on its edges (besides the natural one towards the root);

• for each vertex v of $\gamma$, a rooted tree $\rho (v) \in [RT]$;

• for each additionally oriented edge e of $\gamma$ going from v to w, a pair of vertices $\rho (e)_1 \in \rho (v) \ ,\rho (e)_2 \in \rho (w)$.

We also consider the case in which there is an extra marked vertex $\rho ^* \in \rho (r(\gamma ))$, where $r(\gamma )\in \gamma$ is the root, and in this case we denote $\rho \in NRT^*$. The sum of all the vertices on the various $\rho (v), \ v\in \gamma ,$ yields the total number of vertices in the network, denoted $|\rho |$, and we say $\rho \in NRT_{|\rho |}$. An example of a network of rooted trees is illustrated in Fig. 2a.

Fig. 2

In a we have a network of rooted trees $\rho \in NRT_{4}$ and we depicted its skeleton $\gamma (\rho )\in [RT]_3$ together with the additional orientation on its edges. In b we have the associated Kontsevich graph $\Gamma _\rho$ of type (n, 2), following the assignment $\rho \mapsto \Gamma _\rho$ of Sect. 4.3. The terrestrial vertices are on a horizontal line and the aerial vertices are placed above them. The first (“left”) arrow stemming out of an aerial vertex has a solid black head, while the second (“right”) one has a hollow white one

Consider a vector field $V=V^j(x,p)\partial _{x^j}$ as in Eq. (39) and $p_2 \in M^*$. We now associate a symbol map

$$\begin{aligned} \rho \mapsto E_\rho \equiv E_\rho ^{p_2,V} \in C^\infty (T^*M), \end{aligned}$$

to each network, by giving symbolic rules. For each internal vertex in some $\rho (v), \ v\in \gamma$, we write $p_{2k}V^k$ if it is the root or $V^k$ otherwise. For each internal edge in each tree $\rho (v)$, we write $\partial _{x^k}$ on the left if the edge is arriving or on the right if it is departing towards the root. For each skeleton-oriented edge $e \in \gamma$ connecting $\rho (e)_1$ to $\rho (e)_2$, we write $\pm \partial _{p_j}$ on the left at $\rho (e)_1$ and $\partial _{x^j}$ also on the left at $\rho (e)_2$, where we take $-1$ if the orientation of e is towards the root of $\gamma$ or $+1$ otherwise. When $\rho \in NRT^*$ has an extra marked vertex $\rho ^*$ as above, the associated symbol requires an additional choice of ’decoration’ by $x^j$ or $p_j$ and is denoted

$$\begin{aligned} E_\rho ^{x^j} \ or \ E_\rho ^{p_j}. \end{aligned}$$

This symbol is computed with the same rules as before and by adding at $\rho ^*$ the extra symbol $-\partial _{p_j}$ or $\partial _{x^j}$ on the left, respectively for decorations $x^j$ or $p_j$.

Example A.5

Consider the network $\rho \in NRT_4$ of Fig. 2a. Following the above rules we obtain that the corresponding symbol is given by

$$\begin{aligned} E_\rho ^{p_2,V}(x,p)&= - p_{2k_1} \partial _{p_{j_1}}V^{k_1}|_{(x,p)} \ \partial _{p_{j_2}}V^{l_2}|_{(x,p)} \ \partial _{x^{j_1}}\partial _{x^{l_2}}V^{k_2}|_{(x,p)}\\&\qquad p_{2k_2} \ \partial _{x^{j_2}}V^{k_3}|_{(x,p)} p_{2k_3}. \end{aligned}$$

When $V=V_\pi = \pi ^{ij} p_j \partial _{x^i}$ as in Eq. (38), then

$$\begin{aligned} E_\rho ^{p_2,V}(x,p_1) = - \hat{B}_{\Gamma _\rho }(\pi )(p_1,p_2), \end{aligned}$$

where $\Gamma _\rho$ is the associated Kontsevich graph, computed following Sect. 4.3 and depicted in Fig. 2b, and its Kontsevich symbol was computed in Example A.1.