Energy-saving method of heat transfer enhancement during magneto-thermal convection in typical thermal cavities adopting aspiration

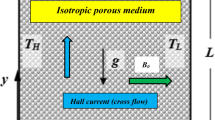
Abstract

In the area of energy-efficient system development, the paper presents a novel approach aiming to enhance heat transfer during magnetohydrodynamic convection in thermal cavities by implementing a technique of free aspiration. Through two small openings, the proposed free aspiration naturally allows a partial admission of fresh fluid into the cavity (from its immediate surroundings) and eventually vents the admitted fluid out the cavity without any pum** device. The efficacy of aspiration technique, whether viable under different problem conditions, is worked out for various classical configurations like differential heating, corner heating, and split heating. The mathematical formulations are derived by applying the Maxwell model (for magnetic fields) and the Brinkman–Forchheimer–Darcy model (for porous media). The evolved complex and coupled mathematical equations (involving the laws of continuity, momentum, and energy) are solved by an indigenously developed computing code. The study is conducted exhaustively addressing both a porous domain and a clear domain in terms of pertinent design parameters—Hartmann number (Ha = 0–100), porosity (ε = 0.1–1), Darcy number (Da = 10⁻⁷–10⁻³), Darcy–Rayleigh number (Ra_m = 0.1–10³), and Rayleigh number (Ra = 10³–10⁶). The obtained results conclusively bring out an improved trend of heat transfer for all three thermal cavities due to the aspiration (without any pum** means). Even with the existence of flow-hindering porous substance and magnetic force, the augmentation in heat transfer could be as high as seven times more compared to their respective non-aspiration cases.

Graphic abstract

1 Introduction

Many transport processes in engineering devices utilize magneto-thermal convection. Temperature gradient principally in a vertical direction drives fluid flow and associated convective heat transfer in thermal devices through natural or mixed convection. Natural or mixed convection prevails in multitudinous applications in technologies and industries. It also occurs spontaneously in many natural phenomena. In several technological as well as industrial applications, magnetic fields play a vital role in the process control of thermal devices. A few examples of magneto-convective applications are as follows: electromagnetic casting, liquid-metal cooling and solidification, crystal growth in molten liquids, smelting, plasma confinement, microfluidic devices, food cleaning, and drying technologies. Magnetohydrodynamic (MHD) thermal convection finds many emerging applications in the presence of other multiphysics (like concentration gradients, porous substance, nanoparticles, etc.). At the present date, biomedical applications and magneto-science are dealing with magnetic endoscopy, separation of cells, thermal therapy, treatment of cancer and tumor, etc.[1, 2]. Rashidi et al. [3] have reviewed the magnetohydrodynamics linking to biological applications.

Enhancing heat transport especially for low energy systems (or passive cooling devices) is always a major concern. The primary and essential issues of such systems to achieve superior thermal performance face many challenges. The growing demand for energy efficiency along with the miniaturization (of devices) necessitates serious research from the fundamentals. In the broad area of thermal convection, there exist many investigations conducted analytically, numerically, and experimentally under different scenarios. However, the contribution toward newer modifications for the augmentation of fluid flow and heat transfer in the cavity-like problems undergoing natural convection is relatively a few. In the present work, thermal convection occurring in a cavity-like system is presented with a new and fruitful modification utilizing a facility of free aspiration. The aspiration is the free partial admission of outside ambient fluid into the cavity and its venting out, similar to the process of human breathing. From the low- and high-pressure points in the cavity, the drawing and venting of fluid take place naturally through the appropriately positioned small openings (aspiration vents). An implementation of aspiration to the cavity is first presented in an earlier work [4] of us using a lid-driven porous cavity without any magnetic field. The scope and practical importance of aspiration were detailed therein, and its ability to enhance the heat transfer was found magnificent (up to ~ 339%) in that context of the lid-driven cavity [4]. The aspiration does not require any additional energy for the evolved partial free flow through the cavity; still, this technique is seemed to be very powerful to enhance the heat transfer [4]. The lack of work in this direction motivates us to undertake the present work to explore the efficacy of aspiration technique on different typical thermal cavities undergoing magnetohydrodynamic (MHD) natural convection. During free aspiration, the partial admission of fresh ambient fluid takes place and the same amount of hot fluid leaves from the cavity as venting. However, the aspiration is only feasible when the working fluids in cavities and their ambient fluids are the same.

In the present study, the cavities are provided with two small openings or aspiration vents (meant for ‘breathing in’ and ‘breathing out’ as indicated in Fig. 1). Under a steady-state condition, continuously through one vent the aspiration draws fluid into the cavity from its immediate surroundings and returns the same through another opening. In the absence of such works in the open literature, the open cavity or partially open cavity problems could be close proximate to the present problem. Full admission of ambient fluid is established in case of open cavity problems, which enters into and leaves out the cavity freely. For both fully open and partially open cavity problems, the ambient fluid is utilized as the working fluid. It is observed that few researchers have focused on heat transfer coupled with magnetohydrodynamic (MHD) flow in open cavities without/with the presence of a porous substance. Kolsi et al. [5] conducted a three-dimensional open channel flow of natural convection using nanofluid and magnetic fields. Utilizing the heatline visualization tool, Bondareva et al. [6] analyzed the effects of nanofluid, magnetic fields, and porous media during natural convection in an open cavity with corner heating considering cavity inclination and wall waviness. Using an open cavity and considering bottom heating, Cu–water nanofluid, and external magnetic fields at different inclinations, Mehrez et al. [7] presented convective heat transfer and associated entropy generation. Kefayati [8] analyzed an open cavity natural convection using a magnetic field and nanofluid. Mahmoudi et al. [9] also considered a magnetic field and nanofluid considering heat generation. Hussein et al. [10] also extended a similar study on natural convection in an open enclosure applying a magnetic field. Other classes of works on thermal convection from an open cavity under the effect of MHD in a confined area packed with fluid-saturated porous substance can be found in the literature as in Refs. [11,12,13,14,15].

Fig. 1

Schematic descriptions of computational domains: a differentially heated cavity (DHCA), b corner-heated cavity (CHCA), c split-heated cavity (SHCA) with aspiration

Many studies were extended to partially open cavities. Oztop et al. [16] considered a three-dimensional computational analysis in a partially open cavity and found that the edge of the openings influences heat transfer and entropy generation. They also analyzed natural convection in a partially heated open cavity packed with a porous substrate [17]. Gangawan et al. [18] examined buoyancy-induced convective heat transfer of different fluids in an open cavity heated partially. Other relevant works on the open cavity can be found in the literature [19,20,21]. The studies on thermal convective heat transfer on in a partially or fully open cavity packed with porous substrate have also been extended in different multi-physical applications. Some of these works are mixed convection in ventilated enclosures with partially filled two-layer porous media [22], fully packed porous cavities [23, 24], and porous cavities with different exit-port positions [25]. A comprehensive review of fluid flow and heat transport through a porous matrix has been reported with greater details by the many renowned authors [26,27,28,29].

Understandably, the enhancement of heat transfer for a system running on natural convection is a difficult task. Furthermore, the presence of a flow-hindering magnetic force and a porous substance during natural convection makes the task more challenging. The porous substance offers resistance to flow and thus reduces convective heat transport. When magnetic field is applied to a fluid, the electrical conducting property interacting with the magnetic field evolves the Lorentz force. This force usually dampens the convective flow and thereby reduces heat transport. The present work focuses on these issues from a fundamental point of view. The present investigation aims to implement free aspiration in thermal cavities undergoing magneto-thermal convection for augmenting the heat transfer. For wide applicability of the present investigation with the aspiration, some typical configurations of heating are chosen using the classical geometries of differentially heated cavity (DHC), corner-heated cavity (CHC), and split-heated cavity (SHC). These configurations are very familiar in miscellaneous applications starting daily life to industrial fields like cooling, heating, drying, etc. Many researchers have reported the importance, applications, and findings of these classical thermal cavities frequently referring to the famous and pioneering works on DHC [30, 31], CHC [32, 33], and SHC [34, 35]. The novel aspect in the context of the present work is the implementation of free aspiration to these cavities along with the consideration of constant external magnetic fields. Furthermore, the effect of aspiration is searched for in a fundamental way for both the cases of porous and clear domains considering broad ranges of the involved parameters like the Hartmann number, Darcy number, porosity, Darcy–Rayleigh number (for a porous domain), and the Rayleigh number (for a clear domain). The present study contributes to the area of enhanced heat transfer without adopting any mechanical means or additional energy input. The aspiration through small openings takes place naturally depending upon the hydrodynamic conditions inside the cavities.

2 Problem description and governing equations

Three classical thermal cavities (of a square shape, the length scale, and total heating length equal to H) are schematically presented in Fig. 1 as the chosen aspirated cavities. Based on typical positions of the heaters, these cavities are referred to as a differentially heated cavity with aspiration (DHCA, in short) in Fig. 1a, a corner-heated cavity with aspiration (CHCA) in Fig. 1b, and a split-heated cavity with aspiration (SHCA) in Fig. 1c. It is noteworthy to mention here that the middle–middle configuration of split heating through the sidewalls is more commonly used in practice. Moreover, this split heating arrangement is also found as a superior configuration for better heat transfer compared to other possible configurations along the sidewalls [38]. The aspiration takes place through the two vents. The vents (of width w = 0.1H) are located in the bottom wall (for a possible fractional inflow of cold fresh fluid) and the middle of the top wall (for fractional venting out of hot fluid). The isothermal conditions of heating (at temperature T_h) and cooling (at temperature T_a) are considered for all the cavities. The cooling temperature T_a is based on the temperature of the ambient fluid. The other boundaries of the cavities are assumed adiabatic. To assess the role of the proposed aspiration on the heat transfer augmentation, the thermal performance of the cavities with no aspiration has to be used as the reference. As such, the thermofluid flow in two sets of three cavities (without and with aspiration, i.e., DHC–DHCA, CHC–CHCA, and SHC–SHCA) is simulated for a range of different parametric variations. Another important point is to be noted here that, as the conditions of the same heating length and the same temperature difference are applied for all the cavities, it can reveal the impact of heater position on the convective flow and thermal performance of both the aspirated and non-aspirated cavities in the presence of magnetic fields and porous media. Further, for the completeness, the analysis of free aspiration is also extended for these cavities with a clear domain (without porous substance). Uniform magnetic fields (of magnitude B) are applied externally at different orientations (of angle γ). When it interacts with the electrical conducting property of the working fluid, the Lorentz force develops. This evolved Lorentz force usually hinders flow velocity, markedly affects flow structure, and eventually dampens global heat transfer from the cavities.

The usefulness of the aspiration technique to the different types of cavity-like thermal systems undergoing natural convection is assessed by applying certain assumptions and models [26,27,28,29] as given below:

• An identical two-dimensional square geometry is considered for all the heating systems.

• The buoyancy force is modeled assuming the Boussinesq approximation.

• The laminar flow under steady condition is simulated assuming fluid properties (density, viscosity, thermal diffusivity) constant.

• The porous medium has uniform pores and permeability. It is also assumed as a homogeneous porous substance for all porous cavities.

• To frame the momentum equations, the Brinkman–Forchheimer–Darcy model is adopted to account for the resistance against fluid motion offered by the porous substance. The enhanced frictional effect at higher velocities is also taken care of by this model through the Forchheimer term.

• The magnetic source located outside of the cavities produces uniform fields that negotiate with the electrically conducting fluid of the system. It results in the flow-dampening Lorentz force that usually retards fluid flow and thereby causes reduced heat transfer.

• The present steady-state modeling and assessment of aspiration technique assume a condition of local equilibrium of temperature of the fluid and porous substance. Accordingly, the derivation of the energy equation is performed, which is justified in the absence of any heat generating process.

• The small value of Reynolds number of magnetic induction is expected for the present study, and different insignificant contributing terms like viscous dissipation, pressure work, induced magnetic effect, Joule heating, and Hall effect are neglected.

The resulting dimensional equations addressing the presence of a porous substance, magnetic field, and aspiration are formulated by applying three basic conservation principles as written below:

$$ \frac{\partial u}{{\partial x}} + \frac{\partial v}{{\partial y}} = 0 $$

(1)

$$ \begin{aligned} & \frac{1}{{\varepsilon^{2} }}\left( {u\frac{\partial u}{{\partial x}} + v\frac{\partial u}{{\partial y}}} \right) = - \frac{1}{\rho }\frac{\partial p}{{\partial x}} + \frac{\nu }{\varepsilon }\left( {\frac{{\partial^{2} u}}{{\partial x^{2} }} + \frac{{\partial^{2} u}}{{\partial y^{2} }}} \right) - \left( {\frac{\nu }{K}u + \frac{{F_{{\text{C}}} \sqrt {u^{2} + v^{2} } }}{\sqrt K }u} \right) \\ & \quad + \,\frac{1}{\rho }\sigma B^{2} \left( {v\sin \gamma \cos \gamma - u\sin^{2} \gamma } \right) \\ \end{aligned} $$

(2)

$$ \begin{aligned} & \frac{1}{{\varepsilon^{2} }}\left( {u\frac{\partial v}{{\partial x}} + v\frac{\partial v}{{\partial y}}} \right) = - \frac{1}{\rho }\frac{\partial p}{{\partial y}} + \frac{\nu }{\varepsilon }\left( {\frac{{\partial^{2} v}}{{\partial x^{2} }} + \frac{{\partial^{2} v}}{{\partial y^{2} }}} \right) - \left( {\frac{\nu }{K}v + \frac{{F_{{\text{C}}} \sqrt {u^{2} + v^{2} } }}{\sqrt K }v} \right) \\ & \quad + \,\frac{1}{\rho }\sigma B^{2} \left( {u\sin \gamma \cos \gamma - v\cos^{2} \gamma } \right) + g\beta (T - T_{{\text{c}}} ) \\ \end{aligned} $$

(3)

$$ u\frac{\partial T}{{\partial x}} + v\frac{\partial T}{{\partial y}} = \alpha \left( {\frac{{\partial^{2} T}}{{\partial x^{2} }} + \frac{{\partial^{2} T}}{{\partial y^{2} }}} \right) $$

(4)

Equations (1)–(4) are transformed into their dimensionless forms to carry out the present simulation and investigation. The final forms of the governing equations are given below:

$$ \frac{\partial U}{{\partial X}} + \frac{\partial V}{{\partial Y}} = 0 $$

(5)

$$ \begin{aligned} & \frac{1}{{\varepsilon^{2} }}\left( {U\frac{\partial U}{{\partial X}} + V\frac{\partial U}{{\partial Y}}} \right) = - \frac{\partial P}{{\partial X}} + \frac{\Pr }{\varepsilon }\left( {\frac{{\partial^{2} U}}{{\partial X^{2} }} + \frac{{\partial^{2} U}}{{\partial Y^{2} }}} \right) - \frac{\Pr }{{{\text{Da}}}}U - \frac{{F_{{\text{C}}} \sqrt {U^{2} + V^{2} } }}{{\sqrt {\varepsilon^{3} {\text{Da}}} }}U \\ & \quad + \,{\text{Ha}}^{2} \Pr \left( {V\sin \gamma \cos \gamma - U\sin^{2} \gamma } \right) \\ \end{aligned} $$

(6)

$$ \begin{aligned} & \frac{1}{{\varepsilon^{2} }}\left( {U\frac{\partial V}{{\partial X}} + V\frac{\partial V}{{\partial Y}}} \right) = - \frac{\partial P}{{\partial Y}} + \frac{\Pr }{\varepsilon }\left( {\frac{{\partial^{2} V}}{{\partial X^{2} }} + \frac{{\partial^{2} V}}{{\partial Y^{2} }}} \right) - \frac{\Pr }{{{\text{Da}}}}V - \frac{{F_{{\text{C}}} \sqrt {U^{2} + V^{2} } }}{{\sqrt {\varepsilon^{3} {\text{Da}}} }}V \\ & \quad + {\text{Ha}}^{2} \Pr \left( {U\sin \gamma \cos \gamma - V\cos^{2} \gamma } \right) + {\text{Ra}}_{{\text{m}}} \frac{\Pr }{{{\text{Da}}}}\theta \\ \end{aligned} $$

(7)

$$ U\frac{\partial \theta }{{\partial X}} + V\frac{\partial \theta }{{\partial Y}} = \left( {\frac{{\partial^{2} \theta }}{{\partial X^{2} }} + \frac{{\partial^{2} \theta }}{{\partial Y^{2} }}} \right) $$

(8)

Here, the pressure (P), temperature ($\theta$), and velocity components (U, V) in (X, Y) coordinates are non-dimensional variables. The involved dimensionless parameters are the Prandtl number (Pr), Hartmann number (Ha), modified Rayleigh or Darcy–Rayleigh number (Ra_m), and the Darcy number (Da). F_C, ε, and γ are, respectively, the Forchheimer coefficient, porosity, and the inclination angle of magnetic fields with the horizontal axis. The details of these quantities are

$$ \begin{aligned} & (X,Y) = (x,y)/H,\quad (U,V) = (u,v)/(\alpha {\text{ /H}}) \, \\ & \theta = (T - T_{{\text{a}}} )/(T_{{\text{h}}} - T_{{\text{a}}} ),\quad P = (p - p_{{\text{a}}} )/\rho (\alpha /H{)}^{{2}} \, \\ & {\Pr} = \nu /\alpha ,{\text{ Da}} = K/H^{{2}} ,\quad {\text{Ha }} = BH\sqrt {\sigma /\rho \nu } \, \\ & F_{{\text{C}}} \, = \, 1.75/\sqrt {150} {,}\quad {\text{Ra}}_{{\text{m}}} = \Pr g\beta (T_{{\text{h}}} - T_{{\text{a}}} )KH/\nu^{2} \\ & {\text{again, Ra}}_{{\text{m}}} = {\text{RaDa,}}\quad {\text{and Ra}} = \Pr g\beta (T_{{\text{h}}} - T_{{\text{a}}} )H^{3} /\nu^{2} \\ \end{aligned} $$

(9)

For the present problem, the buoyancy force drives the fluid flow in the system. Due to the presence of a porous substance, the fluid flow faces more resistance compared to that when the system domain has no porous substance (we can say it as a clear domain). The modified Rayleigh or Darcy–Rayleigh number (Ra_m) can address properly the flow-resisting effect of the porous substance associated with a particular permeability of it (which is addressed by the Darcy number). Therefore, Ra_m is utilized for the buoyancy force term in Eq. (7). The relation of the Darcy–Rayleigh number to the fluid-based Rayleigh number (Ra) is indicated in Eq. (9) as a product of Ra and Da. However, for the simulation of clear domain systems, the Rayleigh number (Ra) is used for the buoyancy term. The continuity and energy equations remain unaltered; however, the momentum equations become more straightforward omitting the porosity (ε) and the Brinkman–Forchheimer–Darcy terms as follows:

$$ \left( {U\frac{\partial U}{{\partial X}} + V\frac{\partial U}{{\partial Y}}} \right) = - \frac{\partial P}{{\partial X}} + \Pr \left( {\frac{{\partial^{2} U}}{{\partial X^{2} }} + \frac{{\partial^{2} U}}{{\partial Y^{2} }}} \right) + {\text{Ha}}^{2} \Pr \left( {V\sin \gamma \cos \gamma - U\sin^{2} \gamma } \right) $$

(10)

$$ \left( {U\frac{\partial V}{{\partial X}} + V\frac{\partial V}{{\partial Y}}} \right) = - \frac{\partial P}{{\partial Y}} + \Pr \left( {\frac{{\partial^{2} V}}{{\partial X^{2} }} + \frac{{\partial^{2} V}}{{\partial Y^{2} }}} \right) + {\text{Ha}}^{2} \Pr \left( {U\sin \gamma \cos \gamma - V\cos^{2} \gamma } \right) + {\text{Ra}}\Pr \theta $$

(11)

The conditions on different boundaries of all the cavities are prescribed for the numerical solutions as follows:

• The zero-velocity condition is implemented at all the walls by U = V = 0.

• The cold and hot walls are, respectively, addressed by θ = 0 and θ = 1 and the adiabatic walls (or portion of walls) by ∂θ/∂N = 0, where N is a normal distance of the immediate node from the respective walls.

• The conditions for the aspiration vents are ∂θ/∂Y = P = 0 and θ = P = 0, respectively, for the top and bottom vents.

The process of ‘breathing in’ is expected to happen through the bottom opening, so θ there is set to 0. The gradient condition is considered at the top opening, which is computed using the upwind scheme. The constant pressure of P = 0 is used at the vents through which the inside and outside fluid interaction could be possible.

The most important parameter of interests, the average Nusselt number (Nu), is estimated using the solved temperature fields. It is computed for all the cavities with aspiration (DHCA, CHCA, and SHCA) and without aspiration (DHC, CHC, and SHC), by integrating the temperature gradient along the hot wall(s) as expressed below:

$$ {\text{Nu}} = \int\limits_{0}^{1} {\left( {\left. { - \frac{\partial \theta }{{\partial X}}} \right|_{X = 0} } \right)} {\text{ d}}Y\quad {\text{for}}\;{\text{DHCA}}\;{\text{and}}\;{\text{DHC}} $$

(12a)

$$ {\text{Nu}} = \int\limits_{0}^{0.5} {\left( {\left. { - \frac{\partial \theta }{{\partial X}}} \right|_{X = 0} } \right)} {\text{ d}}Y + \int\limits_{0}^{0.5} {\left( {\left. { - \frac{\partial \theta }{{\partial Y}}} \right|_{Y = 0} } \right)} {\text{ d}}X\quad {\text{for}}\;{\text{CHCA}}\;{\text{and}}\;{\text{CHC}} $$

(12b)

$$ {\text{Nu}} = \int\limits_{0.25}^{0.75} {\left( {\left. { - \frac{\partial \theta }{{\partial X}}} \right|_{X = 0} } \right)} {\text{ d}}Y + \int\limits_{0.25}^{0.75} {\left( {\left. {\frac{\partial \theta }{{\partial X}}} \right|_{X = 1} } \right)} {\text{ d}}Y\quad {\text{for}}\;{\text{SHCA}}\;{\text{and}}\;{\text{SHC}} $$

(12c)

To understand the overall flow pattern inside the cavities, the stream function ψ is solved iteratively following an integration method [4]. It is given as

$$ - \frac{\partial \psi }{{\partial X}} = V\;{\text{and}}\;\frac{\partial \psi }{{\partial Y}} = U $$

(13)

It is very important to understand the pattern of streamlines in the flow domain as it reflects the associated flow physics with the convective flow. For the present confined domain, the closed-loop streamlines indicate a rotation of fluid mass. This circulation presents important information about the flow. The number of circulations, their shapes and strengths, and the position of individual vortex core become different for the cavities (aspirated and non-aspirated) under different parametric variations. The transport process strongly depends upon these facts. During the presentation of the results, a negative sign (−) before the contour value of ψ in the streamline plots usually suggests a clockwise (CW) rotation, whereas a positive valued ψ appears when the fluid rotates in a counterclockwise (CCW) direction. Stronger fluid flow is indicated by a densely distributed streamlines.

3 Numerical technique

3.1 Code validation

The partial differential equations (PDEs) in their non-dimensional forms, as given in Eqs. (5)–(8), are discretized applying the finite volume method and the appropriate boundary conditions. During the discretization of the PDEs, the diffusion terms are replaced by the second-order central differencing scheme, while the convective term is approximated using QUICK (a second-order upwind differencing scheme). Adopting the SIMPLE algorithm [36], the solutions of the discretized algebraic equations are carried out through the TDMA algorithm. All the computations are performed by an in-house code developed in FORTRAN. The convergence of computation is identified when the maximum residuals (and mass defects) fall below 10⁻⁸ (10⁻¹⁰). For solving the pressure correction, velocity, and temperature equations, the under-relaxation factors are taken as 1.0, 0.4, and 0.9, respectively.

The code has been validated repeatedly and extensively in the context of earlier works [4, 37,38,39]. Biswas et al. [4] have reported the code validation under mixed convective heat transfer through porous media packed in a lid-driven cavity. Still, one more validation study on the MHD effect is conducted here. The accuracy of the present code in solving MHD convection is presented in Tables 1 and 2. For this, the results reported in Ghasemi et al. [40] are utilized for the comparison of Nu and $\left| \psi \right|_{\max }$. Considering Pr = 6.2, the present predictions of the maximum stream function $\left| \psi \right|_{\max }$ and Nu for Ra = 10³–10⁵ and Ha = 0–60 are indicated in Tables 1 and 2. A good agreement is observed with the published results (maximum error < 1.9%).

Table 1 Nu and $\left| \psi \right|_{\max }$ at Ha = 30, Pr = 6.2 and different Ra values

Table 2 Nu and $\left| \psi \right|_{\max }$ at different Ha values, Pr = 6.2 and Ra = 10⁵

3.2 Grid sensitivity analysis

To select an appropriate grid distribution, a grid independence study is performed considering the DHCA case and four different grids (160 × 60, 200 × 100, 242 × 140, and 300 × 200). A nonuniform grid arrangement is applied by refining the mesh size near the walls. As shown in Table 3, the Nu of the heated wall is assessed for Ra_m = 1, 10, 100, 1000 at Da = 10⁻⁴, ε = 0.6, and Ha = 30. With increasing grid size (coarse grid to finer grid), the error percentage (in the brackets) turns to < 1.5% with the grid size 242 × 140. Therefore, this grid size is selected for conducting numerical simulations of all the cavities with and without aspiration. It is noteworthy to mention here that, for each aspiration vent (of the length of 0.1), 54 nodes are considered. In the overall distribution, the dimensionless node spacing is 0.001 and 0.002 as the minimum and maximum sizes, respectively.

Table 3 Nu-based grid analysis of DHCA at Da = 10⁻⁴, ε = 0.6 and Ha = 30

The grid sensitivity analysis based on the aspirated flow rates $(\dot{m}_{{\text{i}}} ,\dot{m}_{{\text{o}}} )$ is also conducted to ascertain the accuracy of the present numerical simulation. Table 4 represents $\dot{m}_{{\text{i}}}$ (inflow), $\dot{m}_{{\text{o}}}$(outflow), and their magnitude difference ($\left| {\dot{m}_{{\text{i}}} - \dot{m}_{{\text{o}}} } \right|$) for different grid sizes. From the table, it is observed that the flow imbalance ($\left| {\dot{m}_{{\text{i}}} - \dot{m}_{{\text{o}}} } \right|$) is very insignificant irrespective of the grid sizes for Ra_m = 1, 10, and 100 and fixed values of Da = 10⁻⁴, ε = 0.6, and Ha = 30. This implies a proper arrangement of nonuniform grid distribution. Considering both the approaches of grid sensitivity analyses (based on the average Nu and the flow balance due to aspiration), 242 × 140 grid size is selected for all the cavities.

Table 4 Aspirated flow-based grid analysis of DHCA at Da = 10⁻⁴, ε = 0.6 and Ha = 30

4 Results and discussion

The present investigation aims to implement free aspiration to explore possible heat transfer augmentation even when the negative dampening forces (evolved from a magnetic field and a porous medium) are present. Three classical cavity problems (DHC, CHC, and SHC–DHC based on differential heating, CHC based on corner heating, and SHC based on split heating as indicated in Fig. 1) are investigated by implementing aspiration facility to the cavities. These basic configurations of the problem are encountered in many familiar applications. The outcomes of the present fundamental study would enrich the knowledge base in this area and could provide valuable information for the improvement of the design and operation of similar thermal systems. The computation is carried out extensively for both the cases of aspiration and no aspiration of three flow configurations considering L = H and Pr = 0.71. For this study, the ranges of operating parameters are chosen as follows: Rayleigh number (Ra = 10³, 10⁴, 10⁵, 10⁶), porosity (ε = 0.1. 0.3, 0.5, 0.6, 0.8, 1), Darcy number (Da = 10⁻⁷, 10⁻⁶, 10⁻⁵, 10⁻⁴, 10⁻³), and Hartmann number (Ha = 0, 10, 30, 50, 70, 100).

It is noteworthy to mention here that, due to the inherent flow-resisting characteristics of the porous matrix, heat transfer reduces substantially. Thus, the finding of any benefit of aspirated porous cavities over non-aspirated porous cavities will be very useful. The same is true with the magnetic field (that dampens the flow velocity through the Lorentz force), and thus, aspiration can be useful to clear-domain applications too. Kee** in mind these facts, the investigation on free aspiration is conducted broadly in two groups: (1) porous cavities with magnetic fields and (2) cavities with a clear domain with magnetic fields. Under each group, the studies are carried out thoroughly in parametric form considering all the involved parameters of fluid flow and heat transfer. This section is organized systematically in more subsections. First of all, the porous cavities are considered to demonstrate the advantage of aspiration for all three cavities (DHC, CHC, and SHC) considering a typical dataset (for Ra_m, ε, Da, Ha) in Sect. 4.1 with three Sects. 4.1.1–4.1.3. Next, the clear-domain cavities with magnetic fields are undertaken (using Ra, Ha, γ) to explore the corresponding impact of aspiration elaborated in Sect. 4.2 with three Sects. 4.2.1–4.2.3. Afterward, the heat transfer enhancement is analyzed for both porous- and clear-domain cavities in Sect. 4.3. The aspirated flow and heat balance analysis are also assessed and included in Sect. 4.4.

4.1 Aspiration to porous thermal cavities (DHC, CHC, and SHC)

4.1.1 Overview of thermal performances and flow structures

In Figs. 2 and 3, the impact of the proposed aspiration on thermal performance and flow structures is explored with the reference to their respective non-aspirated cavities. For a typical dataset (Ra_m = 0.1–10³, Da = 10⁻⁴, ε = 0.6, Ha = 30, and γ = 0°), the trends of average Nusselt number (Nu) are presented in Fig. 2 for both the non-aspirated (DHC, CHC, and SHC) and aspirated cavities (DHCA, CHCA, and SHCA). At a lower value of Ra_m = 0.1, the thermal conduction takes the principal role to drive the flow. The corresponding fluid circulation in the cavities is markedly weak. The free aspiration passing through the cavities is found insignificant to cause any effective difference in Nu of the aspirated and non-aspirated cavities. At Ra_m > 10, when the convection mode of heat transfer plays a dominating role (over thermal conduction), a significantly higher Nu is observed with the aspiration technique. The Nu of aspirated cavities substantially increases due to stronger aspirated flow with the increase in Ra_m. On an overall basis, the thermal performance of SHCA is found to be superior, then DHCA.

Fig. 2

Characteristics of heat transfer with aspiration (DHCA, CHCA, SHCA) and without aspiration (DHC, CHC, SHC) for Ra_m = 0.1–10³ at fixed Da = 10⁻⁴, ε = 0.6, Ha = 30, and γ = 0°. The aspiration remarkably enhances heat transfer at Ra_m> 10 for all the cavities

Fig. 3

Fluid flow (streamlines) and static temperature (isotherms) in cavities at Ra_m = 10², Da = 10⁻⁴, ε = 0.6, Ha = 30, and γ = 0°: a non-aspiration (DHC, CHC, SHC), b aspiration (DHCA, CHCA, SHCA). The average Nu shows significant enhancement in heat transfer (71.99, 61.34, and 230.37% respectively for DHCA, CHCA, and SHCA)

The reason behind the enhanced heat transfer from aspiration can be realized by analyzing the flow structures and static temperature distribution presented in Fig. 3. For this, using a typical dataset of Ra_m = 10², Da = 10⁻⁴, ε = 0.6, Ha = 30, and γ = 0°, the flow structures (indicated by the streamlines) and associated temperature distribution (presented by the isotherms) are shown for the three heating arrangements (DHC, CHC, and SHC) without aspiration (Fig. 3a) and with aspiration (Fig. 3b). The path of aspirated flow (from the entry to exit) is indicated in DHCA, CHCA, and SHCA using the magenta-color dotted line with arrowheads (Fig. 3b). It is noteworthy to mention here that the aspiration as ‘breathing in’ through bottom openings and as ‘breathing out’ through top openings takes place simultaneously in a continuous manner. Consequently, an incessant partial supply (or ‘recharge’) of fresh cold flow together with fractional venting of heated fluid augments the heat transport process in the thermal cavities.

For the case of DHC, the buoyancy-driven flow that originates due to the temperature gradients between the active walls forms a clockwise (CW) circulation in all confined cavities. From the coupled relation among velocity, temperature, and magnetic fields, the CW circulation deflects the upper and lower parts of the isotherms toward the right and left, respectively. When the aspiration is applied to DHC (that is for DHCA), the fresh fluid is partially drawn through the lower opening. The aspirated stream flows over the bottom wall and then along the right hot wall and is finally aired out through the upper port. It leads to a superior rate of heat transfer from the left hot wall, by decreasing the thickness of the thermal boundary layer thereon. The aspirated flow vents most of the heat (which the flow carries) out. It results in a significant rise in Nu (72.09% more) compared to the non-aspirated DHC as indicated below the isotherm plots.

Similarly, in the case of CHC (Fig. 3a), the streamline plot shows a CW circulation in the entire cavity. The static temperature is distributed from the corner heater (located about the lower-left corner). When the aspiration is implemented to CHC (in a similar manner of DHCA), the aspirated stream flows over the bottom wall, and along the left wall, which eventually leaves through the upper opening port (Fig. 3b). The pattern of the streamlines of CHCA is similar to that of DHCA. However, the pattern of isotherms is different due to the corner heating. In this case, the aspiration increases the heat transfer by 61% compared to the non-aspirated CHC.

For the case of split heating, significant changes in flow structures are noted between SHC and SHCA. For the enclosure of SHC (Fig. 3a), due to the presence of a cold bottom wall and a top adiabatic wall, the hot fluid is located in the upper portion of the enclosure indicated by the isotherm contour of 0.9. A stratified distribution of temperature is noted near the bottom cold wall. Furthermore, the configuration of the cold bottom wall and hot sidewalls leads to the formation of two contra-rotating circulations. With this configuration, the heat transfer (as well as $\left| \psi \right|_{\max }$) is found to be the lowest compared to the other two configurations (DHC and CHC). When the aspiration is applied, a dramatic change in the flow patterns is observed in Fig. 3b with SHCA. Fresh cold fluid aspirated through the lower port flows along the hot sidewalls and exits through the upper port. The collection of hot fluid in SHC is not observed for SHCA as it can vent directly hot fluid out. Thus, the distribution of the isotherms (and streamlines too) is found symmetrical about the mid-vertical plane. The Nu of SHCA becomes remarkably higher, compared to the Nu of SHC. Corresponding heat transfer enhancement due to the free aspiration is ~ 231%.

From the findings of Figs. 2 and 3, it is undoubtedly observed that, for all the cases of heating configurations, the rate of heat transfer increases significantly for all aspirated cavities (DHCA, CHCA, SHCA). The main reason behind this is the ingress of cold ambient fluid, which flows over the hot wall(s) and then vents out. A significant part of the heat is rejected during venting (discussed in detail in Sect. 4.4 with Table 7). The flow of aspiration is found to increase with the increase in Ra_m as $\left| \psi \right|_{\max }$ of all the non-aspirated and aspirated cavities increases as presented in Table 5.

Table 5 Gains in maximum stream function ($\left| \psi \right|_{\max }$) with Darcy–Rayleigh number (Ra_m)

The above-discussed facts on free aspiration in connection with Figs. 2 and 3 strongly advocate the use of aspiration in thermal cavities whenever feasible. The implementation of aspiration is very simple and saves energy, as it is free from an electromechanical means. However, one cardinal point about the free aspiration is that the cavity fluid and the ambient fluid must be the same.

4.1.2 Effect of porosity ($\varepsilon$) on aspirated porous cavities

The effect of porosity (ε = 0.1–1.0) on the thermal performance and thermofluid structures is analyzed with the help of Figs. 4 and 5. The average Nu in Fig. 4 shows heat transfer trends at fixed values of Ra_m = 10², Da = 10⁻⁴, Ha = 30, and γ = 0° for both aspirated cavities (DHCA, CHCA, and SHCA) and non-aspirated cavities (DHC, CHC, and SHC). The porosity effect on Nu of non-aspirated cavities is found marginal. However, a growing rate of Nu is observed for all the aspirated cavities. An enhanced heat transfer is found with free aspiration. The thermal performance of SHCA is superior. Kee** Ra_m = 10², Da = 10⁻⁴, and Ha = 30 fixed, the thermofluid structures are examined with ε = 0.3 (Fig. 5a) and 1.0 (Fig. 5b). The increase in the porosity value reduces the resistance to the flowing fluid; the convective flow velocity improves and, in turn, causes a relatively higher heat transfer at a higher value of ε. The relative effect of porosity is also reflected from the values of the maximum stream function (indicated in the cavities) and Nu.

Fig. 4

Influence of porosity on heat transfer characteristics of aspirated cavities (DHCA, CHCA, SHCA) and non-aspirated cavities (DHC, CHC, SHC) at Ra_m = 10², Da = 10⁻⁴, Ha = 30, and γ = 0°. Free aspiration enhances heat transfer significantly for all range of ε

Fig. 5

Fluid flow (streamlines) and temperature distribution (isotherms) in DHCA, CHCA and SHCA at Ra_m = 10², Da = 10⁻⁴, Ha = 30 and γ = 0°: a ε = 0.3, b ε = 1

4.1.3 Effect of Darcy number (Da) on aspirated porous cavities

To comprehend the effect of a porous substance on thermal behavior, the effect of the Darcy number (Da) is investigated for both the non-aspirated and aspirated cavities. The variation of Nu with Da is presented in Fig. 6 considering fixed values of Ra_m = 10², ε = 0.6, Ha = 30, and γ = 0°. In general, as the Da increases, the fluid flow faces less resistance. It increases the flow velocity and, in turn, augments heat transport. In the present case, Ra_m (= RaDa) is kept fixed. Therefore, as Da increases, the fluid-based Ra decreases. As such, in Fig. 6, a trend of Nu is observed almost constant when Da is in the range of 10⁻⁷ to 10⁻⁵. At Da ≤ 10⁻⁵, the permeability of the porous substance is so poor that an increased Ra has almost no effect on Nu. Thereafter, at Da > 10⁻⁵, the Nu is found to be decreasing as the Ra decreases significantly (to maintain a fixed value of Ra_m). It is an interesting observation over the usual concept of an increased Nu at a higher Da. The change in heat transfer is found insignificant with a non-aspiration cavity. However, for the aspirated cavities, the decrement in Nu is higher with the increase in Da value.

Fig. 6

Influence of aspirated flow on heat transfer characteristics of aspirated cavities (DHCA, CHCA, SHCA) over non-aspirated cavities (DHC, CHC, SHC) at Ra_m = 10², ε = 0.6, Ha = 30 and Da = 10⁻⁷–10⁻³. Allowing free aspiration leads to a higher rate of heat transfer as Da decreases

When the structures of flow and static temperature distribution of the aspirated cavities at different values of Da (10⁻⁶, 10⁻³) are compared (kee** fixed Ra_m = 10², ε = 0.6, and Ha = 30) as presented in Fig. 7, it supports the findings of Fig. 6. When Fig. 7a is compared with Fig. 7b, the strength of circulation becomes higher due to fixed Ra_m even with Da = 10⁻⁶ (since Ra increases as Da decreases). Consistent with densely distributed streamlines, a thin thermal boundary layer appears at the hot wall. It increases Nu at Da = 10⁻⁶ (Fig. 7a), compared to Nu at Da = 10⁻³ (Fig. 7b).

Fig. 7

Fluid flow (streamlines) and temperature field (isotherms) in DHCA, CHCA and SHCA at Ra_m = 10², ε = 0.6, Ha = 30 and γ = 0°: a Da = 10⁻⁶, b Da = 10⁻³. At a lower Da, the dense distributions of streamlines and isotherms are noted

4.2 Aspiration to clear-domain thermal cavities (DHC, CHC, and SHC)

After the analysis of porous cavities without and with aspiration, the study of aspiration is extended to a clear-domain condition of the same classical flow geometries. To explore this, the aspiration flow in a clear domain is investigated first without a magnetic field and presented in Figs. 8 and 9; thereafter, the impact of an external magnetic field is analyzed using Figs. 10 and 11. Finally, the orientation of the applied magnetic field is also investigated with the help of Figs. 12 and 13.

Fig. 8

Heat transfer of clear-domain cavities with aspiration (DHCA, CHCA, SHCA) over no aspiration (DHC, CHC, SHC) at different Ra values in the absence of magnetic fields. With aspiration, Nu consistently enhances with increasing Ra. Nu of SHCA is the highest for all Ra

Fig. 9

Fluid flow (streamlines) and temperature distribution (isotherms) in clear-domain cavities without magnetic field at Ra = 10⁵: a non-aspiration (DHC, CHC, SHC), b aspiration (DHCA, CHCA and SHCA). Heat transfer augmentation is significant—about 58.46, 41.20 and 296.18% respectively for DHCA, CHCA and SHCA

Fig. 10

Influence of Ha on heat transfer characteristics for DHCA, CHCA and SHCA over non-aspiration (DHC, CHC, and SHC), indicated by Nu for different Ha values at γ = 0°. With aspiration, Nu consistently decreases with increasing Ha

Fig. 11

Fluid flow (streamlines) and static temperature (isotherms) in clear-domain aspirated cavities at Ra = 10⁵ and γ = 0°: a Ha = 30, b Ha = 50. A strong impact of Ha is observed; higher Ha weakens flow fields and Nu

Fig. 12

Impact of magnetic field inclination angle (γ) on heat transfer (of DHCA, CHCA, SHCA, and DHC, CHC, SHC) at Ra = 10⁵ and Ha = 30

Fig. 13

Fluid flow (streamlines) and temperature distribution (isotherms) in clear-domain aspirated cavities at Ra = 10⁵ and Ha = 30: a $\gamma$ = 30°, b $\gamma$ = 90°

4.2.1 Overview of thermal performances and flow structures without magnetic field

The overall thermal performance with a clear domain is presented in Fig. 8 for Ra = 10³–10⁶ without a magnetic field (Ha = 0). In the figure, the average Nu for with and without aspiration is shown. It is noted that the trend curves of heat transfer are clustered for Ra ≤ 10⁴ and thereafter diverging with the increasing Ra values. The mode of heat transfer is governed by a nearly pure conduction mode when Ra is significantly less (≤ 10³). In contrast, the thermal convection plays a dominating role causing a higher rate of heat transfer at a higher Ra. As the Rayleigh number increases, the thermal convection is progressively strengthened through an enhanced convective velocity. It causes steep temperature gradients and thinner thermal boundary layers near the active walls. Thus, at Ra = 10⁶, the Nu becomes highest for all the cases presented in Fig. 8. This figure interestingly shows the magnitude of heat transfer with the aspiration significantly high compared to the case of no aspiration. The maximum heat transfer is noted for SHCA.

The flow structure and the temperature distribution at Ra = 10⁵ are shown in Figs. 9a and 9b for both the cases of non-aspiration and aspiration, respectively. The streamline plots show that the overall features are not similar to those obtained with a porous medium (Fig. 3). For the case of DHC, one large CW circulation is distinctly observed inside the cavity, in which there also exist two small circulation cells. Similarly, for the case of CHC, the primary clockwise circulating cell covers the entire space inside the enclosure. For the case of SHC, two symmetric circulations are located about the mid-vertical plane. At Ra = 10⁵, the maximum stream function ($\left| \psi \right|_{\max }$) becomes around 4.522, 6.319, and 9.446, respectively, for DHC, CHC, and SHC. At this heightening circulation (compared to their respective porous cavities), the isotherms exhibit different characteristics than those obtained in the presence of a porous substance. The pattern of the isotherms indicates a nonuniform temperature distribution in DHC and CHC. However, for SHC, a better stratification of the temperature field is observed in the bottom region of the cavity. In Fig. 9b, with the introduction of aspiration, both the streamlines and isotherms in the clear-domain cavities change drastically. The aspirated flow takes place vigorously at both suction and venting. It leads to an increase in the maximum stream function ($\left| \psi \right|_{\max }$), which at Ra = 10⁵ becomes approximately 7.116, 6.319, and 9.446 for DHCA, CHCA, and SHCA, respectively. The aspiration flow markedly modifies the temperature distribution compared to no-aspiration cases as well as with the presence of a porous substance. The isotherm lines are mostly localized adjoining to the hot wall(s). Thus, the rate of heat transfer (Nu) markedly increases (as indicated below the isotherm plots). The enhanced behavior in the flow fields and Nu can be attributed to the fact that, as the porous substance is absent, the fluid flow in the cavities takes place freely (without any additional resistance from the porous medium). Furthermore, the aspiration becomes stronger as the basic circulation strength in non-aspirated cavities is inherently very strong. The stronger aspiration causes the swee** of heat from the hot walls, leading to an enhanced heat transfer.

4.2.2 Impact of Hartmann number (Ha) on aspirated cavities

In Figs. 10 and 11, the Nu (Fig. 10) and the streamlines and isotherms (Fig. 11) are presented for the clear-domain cases of DHC, CHC, and SHC. Figure 10 demonstrates the variation of heat transfer characteristics with Ha for both the cases of aspiration and non-aspiration at a fixed Ra = 10⁵. In general, with Ha = 0 (no magnetic field), the rate of heat transfer is maximum (since the evolved Lorentz force dampening the flow velocity is absent). An increase in Ha markedly reduces heat transfer. This is true for aspirated flow as well as non-aspirated flow. However, the magnitude of change in heat transfer is significant with the aspiration. Now, as Ha increases, both the U and V velocity components decrease abiding the mass balance. In turn, the reduced flow velocity takes active participation in heat energy transport as given in Eq. (8). It results in a lesser amount of heat transport in the cavities. Hence, the increase in Ha value leads to a more reduction in heat transfer. In general, for a fixed Ra = 10⁵, when Ha changes from 0 to 100, the performance of the overall heat transfer reduces from 7.166 to 1.938 for DHCA, from 6.319 to 2.555 for CHCA, and from 9.446 to 3.337 for SHCA. Similarly, the reduction in heat transfer is observed with the non-aspirated cases as 4.522 to 1.224 (for DHC), 4.475 to 1.354 (CHC), and 2.384 to 1.354 (SHC).

In Fig. 11, the structures of the thermofluid flow fields at different Hartmann numbers (Ha = 30, 50) are represented at Ra = 10⁵ for only the aspirated cavities (DHCA, CHCA, and SHCA). The streamlines, as well as the isotherms, become different with increasing Ha. Comparing respective plots (Figs. 11a with Fig. 11b), it is clear that the number of streamlines strongly decreases (due to the dampening effect) as the Ha increases. On the other hand, the isotherm plots indicate thicker thermal boundary layers with an increase in Ha. It is important to mention here that, as the magnetic field strength increases, it weakens the strength of basic circulation in the confined/closed cavities. In turn, the aspiration caused partial flow reduces with the increase in Ha.

4.2.3 Impact of magnetic field angle ($\gamma$) on aspirated cavities

Finally, the inclination (γ) effect of the imposed magnetic field is investigated as depicted in Figs. 12 and 13. Figure 12 presents the average Nu distribution with an increasing angle for fixed Ha = 30 and Ra = 10⁵. An increase in γ from 0 to 180° demonstrates a non-monotonic increasing and decreasing variation in heat transfer. The trend curve of the heat transfer gradually increases as the angle γ increases and becomes maximum at γ = 90° for SHCA, γ = 60° for DHCA, and γ = 60° for CHCA. The heightening of heat transfer is more with the case of SHCA. The changes in Nu for a non-aspiration flow with γ are almost insignificant.

However, the shape and the size of the circulation cells in the cavities are strongly influenced by the direction of the imposed magnetic field. It can be realized from Fig. 13 showing the flow structures at γ = 30° and 90° with fixed values of Ha = 30 and Ra = 10⁵. With a change in γ, the CW circulation cells are distorted for both the cases of DHCA and CHCA (the distortion is more comparable to the situation at γ = 0°), whereas, for the case of SHCA, the aspirated stream partly deflects toward the right active wall and partly toward the left active wall at γ = 30°. With such deflections, the maximum stream function $\left| \psi \right|_{\max }$ is increased substantially to 10.679 (DHCA), 12.262 (CHCA), and 12.084 (SHCA) at γ = 30°, compared to that of γ = 0° (as in Fig. 11a). It is attributed to nonuniformly distributed magnetic forces inside the cavities. The corresponding distribution of the isotherms represents a weak variation (compared to that of γ = 0° as in Fig. 11a) for the cases of DHCA and CHCA. For SHCA, the deflection of isotherms is prominently noticeable. However, the distance between the active walls and the isotherm contour of value ‘0.9′ reduces significantly, which leads to an increased value of Nu. A change in γ also affects the aspirated flow path inside the cavities.

4.3 Analysis of heat transfer enhancement

The assessment of the benefit of free aspiration on the thermal performance is carried out for both the porous cavities and the clear-domain cavities as shown in Figs. 14 and 15, respectively. For this assessment, a heat transfer parameter (η) is defined using identical parametric conditions of the aspirated cavities (DHCA, CHCA, and SHCA) and their respective non-aspirated cavities (DHC, CHC, SHC) as given in Eq. (14):

$$ \eta = \frac{{{\text{Nu}}\;{\text{with}}\;{\text{aspiration}}}}{{{\text{Nu}}\;{\text{with}}\;{\text{no } - \text{ aspiration }}}} $$

(14)

Fig. 14

Characteristics of an enhanced heat transfer of aspirated porous cavities (DHCA, CHCA, SHCA): a Darcy–Rayleigh number, b porosity, c Darcy number and d Hartmann number

Fig. 15

Characteristics of an enhanced heat transfer of clear-domain aspirated cavities showing the impacts of a Rayleigh number, b Hartmann number and c magnetic field angle

The percentage enhancement of heat transfer is indicated by (η − 1) × 100%.

In Figs. 14a–d for all the aspirated porous cavities, the heat transfer enhancement of SHCA (split heating case) is found superior under the parametric conditions of Ra_m, ε, Da, and Ha. Except for a very low range of Darcy–Rayleigh number (Ra_m < 10), the heat transfer shows enhancement with the increase in Ra_m (Fig. 14a). A sharp rise in η is found at Ra_m > 100. Here, Da = 10⁻⁴, ε = 0.6, Ha = 30, and γ = 0° are kept constant. The heat transfer augmentation reaches to a maximum of 708% (η = 8.08) even with the presence of the porous substance at Ra_m = 10³, which is a strongly convection-dominated flow regime. For other cavities under the same parametric conditions and Ra_m = 10³, the maximum augmentation becomes 179% (η = 2.79) for DHCA and 56% (η = 1.56) for CHCA. The porosity effect, as indicated at fixed Ra_m = 10², Da = 10⁻⁴, and Ha = 30 in Fig. 14b, also displays a heat transfer enhancement with all ranges of ε; however, the trends of curves here are more or less flat. The ranges of heat transfer enhancement are 65–76% for DHCA, 50–65% for CHCA, and 173–247% for SHCA. In Fig. 14c with Ra_m = 10², ε = 0.6, and Ha = 30, the augmentation of heat transfer by applying aspiration is noted for all Da values. It reveals that η is less sensitive when Da < 10⁻⁴, particularly for DHCA and CHCA. It happens due to a fixed value of Ra_m (= RaDa). When the Da decreases, the Ra (a fluid-based Rayleigh number) increases. It can counterbalance the poor permeability of the porous system at a very low Da by invigorated Ra (which is responsible for a strong convective flow). In Fig. 14d, the influence of the magnetic field strength is presented with the fixed parameters Ra_m = 10², Da = 10⁻⁴, and ε = 0.6. It indicates a minor change in η with Ha for DHCA and CHCA. However, for SHCA, the traditional decreasing trend with the increasing Ha is observed. The aspiration leads to heat transfer enhancement for all the cavities, and the thermal performance of SHCA is found superior.

In the case of a clear domain (absence of the porous substance) in Fig. 15a, the enhancement of heat transfer at Ra = 10⁶ and Ha = 30 is a maximum of 604% (η = 7.04) for SHCA. The maximum enhancements are 114% (η = 2.14) for CHCA and 73% (η = 1.73) for DHCA. For Ra ≤ 10⁴ using aspiration, there is almost no improvement in heat transport. The influence of the magnetic field on the overall heat transfer is indicated in Fig. 15b considering γ = 0. A trend of an increment (up to Ha = 50) and then a decrement in η is observed at Ra = 10⁵. It happens due to a change in flow structures due to aspiration. The maximum enhancements in heat transfer due to the aspiration are observed 74% for DHCA at Ha = 50 (η = 1.74) and 79% for CHCA at Ha = 70 (η = 1.79), whereas an opposite trend of η for SHCA is observed with Ha. A substantial enhancement in heat transfer of 296% ($\eta$ = 3.96) is observed at Ha = 0. It decreases rapidly to a minimum value of 118% ($\eta$ = 2.18) at Ha = 50. Afterward, η monotonously increases up to Ha = 100. For the case of split heating (SHCA), the location of heating sources and the free aspiration are responsible for this enhancement and peculiar trend in η. In Fig. 15c at Ra = 10⁵ and Ha = 30, the enhancement and η are found changing under the variation of the magnetic field angle (γ) for SHCA. At γ = 90°, heat transfer enhancement is a maximum of 269% (η = 3.69), whereas η is almost invariable with γ for DHCA and CHCA.

4.4 Assessment of aspirated flow and associated heat balance

For the completeness of the present investigation, the analysis on the aspirated flow rate ($\dot{m}_{{\text{i}}}$) and associated heat transport is conducted as presented in tabular form in Tables 6 and 7 under the different parametric conditions. It is important to mention here that the rate of the aspiration flow for different cavities is calculated using the simulated velocity fields over the inflow aspiration vents. Table 6 is dedicated to the aspirated flow ($\dot{m}_{{\text{i}}}$). In Table 6a, the effect of porosity (ε) of the porous matrix is presented taking Ra_m = 10², Da = 10⁻⁴, Ha = 30, γ = 0° (fixed) for three geometries (DHCA, CHCA, SHCA). In general, the aspirated flow ($\dot{m}_{{\text{i}}}$) increases with the increase in ε and a maximum $\dot{m}_{{\text{i}}}$ is observed at ε = 1. It is also noted that for any value of the porosity, $\dot{m}_{{\text{i}}}$ is higher for SHCA. It happens due to the presence of temperature gradients on both the vertical hot walls; it supports the free aspiration of the ambient fresh fluid in comparison with other flow configurations (discussed earlier in detail with Fig. 5). Similarly, the effect of the Darcy number at fixed Ra_m = 10², ε = 0.6, Ha = 30, γ = 0° is indicated in Table 6b. Unlike the usual notion, it is interesting to note that a substantial increment in $\dot{m}_{{\text{i}}}$ is observed with the decrement in Da. It happens as the Darcy–Rayleigh number is kept fixed at Ra_m = 100. For a fixed Ra_m (= RaDa), as the Da decreases, the fluid-based Rayleigh number (Ra) increases and it contributes to the enhanced rate of aspiration (more discussion presented earlier with Fig. 7). The aspiration becomes significant at stronger buoyancy for both the porous domain (Ra_m ≥ 100 and fixed Da = 10⁻⁴, ε = 0.6, Ha = 30, γ = 0°) and the clear domain (Ra ≥ 10⁵ and Ha = 0) as, respectively, mentioned in Table 5c and Table 6d. Here, SHCA shows a higher rate of aspiration. The aspiration for the clear-domain cavities under the influence of a magnetic field is indicated in Table 6e for Ra = 10⁵, Ha = 10–100, γ = 0. The significant reduction in the aspiration flow is noted as the magnetic field (Ha) increases. Finally, the impact of the angle γ of the magnetic field is presented in Table 6f using a clear domain, Ra = 10⁵, Ha = 30. It shows a maximum aspiration at γ = 90°. Overall, it can be concluded from Table 6 that the rate of aspiration in the case of SHCA is maximum; CHCA stands next to it, and DHCA shows the lowest rate of aspiration among all three cavities.

Table 6 The analysis of the rate of aspirated flow ($\dot{m}_{{\text{i}}}$) under different parametric conditions

Table 7 Heat balance analysis of three cavities (DHC, CHC, and SHC) without and with aspiration

Adopting the same structure of the aspirated flow analysis, the heat balance analysis is prepared as indicated in Table 7. However, in this analysis, both the non-aspiration and aspiration cavities are included. For each of the non-aspiration cases, the rate of heat input ($\dot{q}_{{\text{h}}}$) to the cavity from the heat source is the same as the heat rejection rate ($\dot{q}_{{\text{c}}}$) through the cold heat sinks (as the steady-state condition is applied during the simulations). For the aspirated cases, the heat balance is made as per Eq. (15) given below:

$$ \dot{q}_{{\text{h}}} = \dot{q}_{{\text{c}}} + \dot{q}_{{\text{a}}} $$

(15)

The heat input ($\dot{q}_{{\text{h}}}$) to the system is released through the cold heat sinks ($\dot{q}_{{\text{c}}}$) and by the aspirated partial venting ($\dot{q}_{{\text{a}}}$) of the hot fluid. In Table 7, the heat transfer is presented as $\dot{q}_{{\text{h}}}$(= $\dot{q}_{{\text{c}}}$) for the non-aspiration conditions, first. Thereafter, the results with the aspiration are shown. From all the parametric conditions, it is evident that the heat input increases substantially with the aspiration and a major share of heat rejection takes place by venting ($\dot{q}_{{\text{a}}}$). In Table 7a, without aspiration, the heat transfer is found to increase with the porosity particularly for DHC and CHC. However, SHC shows an almost insignificant impact on this issue. When aspiration is applied, SHC shows a growing trend of heat transfer against ε (same as the non-aspiration cases). The same is applicable for DHC and CHC with aspiration. In Table 7b–d, respectively, for the variations in Da, Ra_m, and Ra, the consistent pattern of heat transfer under no aspiration and aspiration is observed. Table 7e shows the effect of Ha on the clear domain, which indicates decreasing trends for all the shares of heat transfer for all the cavities. Table 7f presents a minor impact of the magnetic field angle (γ) on the heat balance as found with the aspirated flow in Table 6f. For all the cases, the aspirated heat transport ($\dot{q}_{{\text{a}}}$) is significantly higher compared to their share through the cold walls ($\dot{q}_{{\text{c}}}$). The aspirated flow ($\dot{m}_{{\text{i}}}$) carries a substantial quantity of heat ($\dot{q}_{{\text{a}}}$). The aspiration effect reduces heat rejection significantly through the cold heat sink ($\dot{q}_{{\text{c}}}$). The aspirated cases lead to a higher transfer from the heat source. The reason behind this is that the aspiration flow additionally cools the heated walls. Thus, the flow of aspiration ($\dot{m}_{{\text{i}}}$) causes an enhanced heat transfer under the cases of aspiration.

5 Conclusions

In this study, a novel approach for augmenting heat transfer of cavity-like thermal systems is demonstrated by introducing a free aspiration technique. This new technique is applied to a confined cavity flow considering different heating configurations, porous substance and magnetohydrodynamic thermal convection. Free aspiration through the cavities occurs naturally through some properly located vents by partially exchanging fluid with the immediate surroundings. The investigation is carried out thoroughly on the aspirated cavities (DHCA, CHCA, and SHCA) and their counterpart of non-aspirated cavities (DHC, CHC, and SHC) along with magnetic fields, a porous medium and a clear domain. The salient observations are:

• Without any additional pum** effort and their mounting complexities, the free aspiration can augment heat transfer remarkably. This fact applies to all three configurations of heating examined with DHC, CHC, and SHC in the presence of a magnetic field and a porous substance (both of them generally detrimental to a convective flow).

• From a heat balance analysis, it establishes that the aspirated flow stream plays a major role to remove heat from the heat source of the cavities.

• Among the three heating configurations of cavities, the case of split heating (SHC) demonstrates as a most beneficial configuration. It results in a magnificent heat transfer performance with the aspiration. Compared to other aspirated flow cases, SHCA configuration causes a substantial flow of aspiration, and thus, it leads to better heat transport.

• In general, all the parameters of Ra_m, Da, ε, Ha and γ affect the flow structure, and the thermal patterns inside the cavities are further severely influenced by the free aspiration leading to better thermal performance.

• As the Rayleigh number (Ra) or the Darcy–Rayleigh number (Ra_m) increases, the trend of heat transfer increases appreciably. The permeability of the porous medium (or porosity) and the magnetic field inclination angle have a minor role to modify the transfer characteristics.

• As the strength of the magnetic field (Ha) increases, the buoyancy effect decreases substantially leading to a reduction in heat transfer.

The approach of free aspiration demonstrated in the present work can easily be adapted and extended to other systems (along with other boundary conditions and multi-physical issues of nanofluid, non-Newtonian fluid, etc.)