SpringerLink
Log in

Trilinear equations, Bell polynomials, and resonant solutions

  • Research Article
  • Published:
Frontiers of Mathematics in China Aims and scope Submit manuscript

Abstract

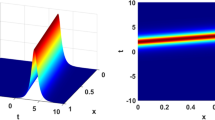
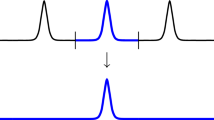
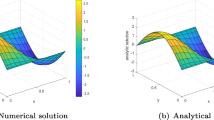
A class of trilinear differential operators is introduced through a technique of assigning signs to derivatives and used to create trilinear differential equations. The resulting trilinear differential operators and equations are characterized by the Bell polynomials, and the superposition principle is applied to the construction of resonant solutions of exponential waves. Two illustrative examples are made by an algorithm using weights of dependent variables.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Bell E T. Exponential polynomials. Ann Math, 1934, 35: 258–277

    Article  Google Scholar 

  2. Bogdan M M, Kovalev A S. Exact multisoliton solution of one-dimensional Landau-Lifshitz equations for an anisotropic ferromagnet. JETP Lett, 1980, 31(8): 424–427

    Google Scholar 

  3. Broer L J F. Approximate equations for long wave equations. Appl Sci Res, 1975, 31(5): 377–395

    Article  MathSciNet  MATH  Google Scholar 

  4. Craik A D D. Prehistory of Faà di Bruno’s formula. Amer Math Monthly, 2005, 112: 217–234

    Article  MathSciNet  Google Scholar 

  5. Delzell C N. A continuous, constructive solution to Hilbert’s 17th problem. Invent Math, 1984, 76(3): 365–384

    Article  MathSciNet  MATH  Google Scholar 

  6. Gilson C, Lambert F, Nimmo J, Willox R. On the combinatorics of the Hirota D-operators. Proc R Soc Lond A, 1996, 452: 223–234

    Article  MathSciNet  MATH  Google Scholar 

  7. Grammaticos B, Ramani A, Hietarinta J. Multilinear operators: the natural extension of Hirota’s bilinear formalism. Phys Lett A, 1994, 190(1): 65–70

    Article  MathSciNet  Google Scholar 

  8. Hietarinta J. Hirota’s bilinear method and soliton solutions. Phys AUC, 2005, 15(part 1): 31–37

    Google Scholar 

  9. Hirota R. Exact solution of the Korteweg-de Vries equation for multiple collisions of solitons. Phys Rev Lett, 1971, 27: 1192–1194

    Article  MATH  Google Scholar 

  10. Hirota R. A new form of Bäcklund transformations and its relation to the inverse scattering problem. Progr Theoret Phys, 1974, 52: 1498–1512

    Article  MATH  Google Scholar 

  11. Hirota R. Soliton solutions to the BKP equations-I. The Pfaffian technique. J Phys Soc Jpn, 1989, 58: 2285–2296

    Article  MathSciNet  Google Scholar 

  12. Hirota R. The Direct Method in Soliton Theory. Cambridge: Cambridge University Press, 2004

    Book  MATH  Google Scholar 

  13. Hu X B, Wang H Y. Construction of dKP and BKP equations with self-consistent sources. Inverse Problems, 2006, 22: 1903–1920

    Article  MathSciNet  MATH  Google Scholar 

  14. Kaup D J. A higher-order water-wave equation and the method for solving it. Progr Theoret Phys, 1975, 54(2): 396–408

    Article  MathSciNet  MATH  Google Scholar 

  15. Lambert F, Springael J. Construction of Bäcklund transformations with binary Bell polynomials. J Phys Soc Jpn, 1997, 66: 2211–2213

    Article  MathSciNet  MATH  Google Scholar 

  16. Lambert F, Springael J, Willox R. On a direct bilinearization method: Kaup’s higherorder water wave equation as a modified nonlocal Boussinesq equation. J Phys A: Math Gen, 1994, 27: 5325–5334

    Article  MathSciNet  MATH  Google Scholar 

  17. Ma WX. Complexiton solutions to the Korteweg-de Vries equation. Phys Lett A, 2002, 301: 35–44

    Article  MathSciNet  MATH  Google Scholar 

  18. Ma W X. Variational identities and Hamiltonian structures. In: Ma W X, Hu X B, Liu Q P, eds. Nonlinear and Modern Mathematical Physics. AIP Conf Proc 1212. Melville: Amer Inst Phys, 2010, 1–27

    Google Scholar 

  19. Ma W X. Generalized bilinear differential equations. Stud Nonlinear Sci, 2011, 2: 140–144

    Google Scholar 

  20. Ma W X. Bilinear equations, Bell polynomials and the linear superposition principle. J Phys: Conf Ser, 2013, 411: 012021

    Article  Google Scholar 

  21. Ma W X, Abdeljabbar A, Asaad M G. Wronskian and Grammian solutions to a (3+1)-dimensional generalized KP equation. Appl Math Comput, 2011, 217: 10016–10023

    Article  MathSciNet  MATH  Google Scholar 

  22. Ma W X, Fan E G. Linear superposition principle applying to Hirota bilinear equations. Comput Math Appl, 2011, 61: 950–959

    Article  MathSciNet  MATH  Google Scholar 

  23. Ma W X, Li C X, He J S. A second Wronskian formulation of the Boussinesq equation. Nonlinear Anal, 2009, 70: 4245–4258

    Article  MathSciNet  MATH  Google Scholar 

  24. Ma W X, You Y. Solving the Korteweg-de Vries equation by its bilinear form: Wronskian solutions. Trans Amer Math Soc, 2005, 357: 1753–1778

    Article  MathSciNet  MATH  Google Scholar 

  25. Ma W X, Zhang Y, Tang Y N, Tu J Y. Hirota bilinear equations with linear subspaces of solutions. Appl Math Comput, 2012, 218: 7174–7183

    Article  MathSciNet  MATH  Google Scholar 

  26. Matsukidaira J, Satsuma J. Integrable four-dimensional nonlinear lattice expressed by trilinear form. J Phys Soc Jpn, 1990, 59(10): 3413–3416

    Article  MathSciNet  Google Scholar 

  27. Matsukidaira J, Satsuma J. Exactly solvable four-dimensional discrete equation expressed by trilinear form. Phys Lett A, 1991, 154: 366–372

    Article  MathSciNet  Google Scholar 

  28. Matsukidaira J, Satsuma J. The trilinear equation as a (2+2)-dimensional extension of the (1+1)-dimensional relativistic Toda lattice. Phys Lett A, 1991, 161: 267–273

    Article  MathSciNet  Google Scholar 

  29. Matsukidaira J, Satsuma J, Strampp W. Soliton equations expressed by trilinear forms and their solutions. Phys Lett A, 1990, 147: 467–471

    Article  MathSciNet  Google Scholar 

  30. Terng C -L, Uhlenbeck K. Geometry of solitons. Notices Amer Math Soc, 2000, 47(1): 17–25

    MathSciNet  MATH  Google Scholar 

  31. Terng C -L, Uhlenbeck K. The n×n KdV hierarchy. J Fixed Point Theory Appl, 2011, 110(1): 37–61

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics and Statistics, University of South Florida, Tampa, FL, 33620-5700, USA

    Wen-**u Ma

Authors
  1. Wen-**u Ma
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Wen-**u Ma.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Ma, WX. Trilinear equations, Bell polynomials, and resonant solutions. Front. Math. China 8, 1139–1156 (2013). https://doi.org/10.1007/s11464-013-0319-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11464-013-0319-5

Keywords

MSC

Search

Navigation