SpringerLink
Log in

New way to construct high order Hamiltonian variational integrators

  • Published:
Applied Mathematics and Mechanics Aims and scope Submit manuscript

Abstract

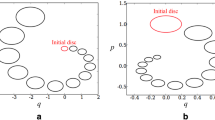
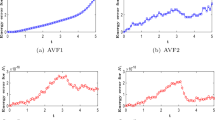
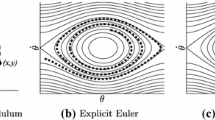
This paper develops a new approach to construct variational integrators. A simplified unconventional Hamilton’s variational principle corresponding to initial value problems is proposed, which is convenient for applications. The displacement and momentum are approximated with the same Lagrange interpolation. After the numerical integration and variational operation, the original problems are expressed as algebraic equations with the displacement and momentum at the interpolation points as unknown variables. Some particular variational integrators are derived. An optimal scheme of choosing initial values for the Newton-Raphson method is presented for the nonlinear dynamic system. In addition, specific examples show that the proposed integrators are symplectic when the interpolation point coincides with the numerical integration point, and both are Gaussian quadrature points. Meanwhile, compared with the same order symplectic Runge-Kutta methods, although the accuracy of the two methods is almost the same, the proposed integrators are much simpler and less computationally expensive.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (France)

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Feng, K. Difference schemes for Hamiltonian formalism and symplectic geometry. Journal of Computational Mathematics, 4, 279–289 (1986)

    MathSciNet  MATH  Google Scholar 

  2. Ruth, R. D. A canonical integration technique. IEEE Transactions on Nuclear Science, 30, 2669–2671 (1983)

    Article  Google Scholar 

  3. Zhong, W. X. and Williams, F. W. A precise time step integration method. Proceedings of the Institution of Mechanical Engineers, 208, 427–430 (1994)

    Google Scholar 

  4. Zhong, W. X. On precise integration method. Journal of Computational and Applied Mathematics, 163, 59–78 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  5. Saito, S., Sugiura, H., and Mitsui, T. Family of symplectic implicit Runge-Kutta formulae. BIT Numerical Mathematics, 32, 539–543 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  6. Sanz-Serna, J. M. and Abia, L. Order conditions for canonical Runge-Kutta schemes. SIAM Journal on Numerical Analysis, 28, 1081–1096 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  7. Abia, L. and Sanz-Serna, J. M. Partitioned Runge-Kutta methods for separable Hamiltonian problems. Mathematics of Computation, 60, 617–634 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  8. Monovasilis, T., Kalogiratou, Z., and Simos, T. E. Symplectic partitioned Runge-Kutta methods with minimal phase-lag. Computer Physics Communications, 181, 1251–1254 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  9. Okunbor, D. and Skeel, R. D. An explicit Runge-Kutta-Nyström method in canonical if and only if its adjointis explicit. SIAM Journal on Numerical Analysis, 29, 521–527 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  10. Franco, J. M. and G´omez, I. Symplectic explicit methods of Runge-Kutta-Nyström type for solving perturbed oscillators. Journal of Computational and Applied Mathematics, 260, 482–493 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  11. Simos, T. E. and Vigo-Aguiar, J. Exponentially fitted symplecitic integrator. Physical Reviwe E, 67, 016701 (2003)

    Article  MathSciNet  Google Scholar 

  12. Simos, T. E. Exponentially-fitted Runge-Kutta-Nyström method for the numerical solution of initial-value problems with oscillating solutions. Applied Mathematics Letters, 15, 217–225 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  13. Marsden, J. E. and West, M. Discrete mechanics and variational integrators. Acta Numerica, 10, 357–514 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  14. Lew, A., Marsden, J. E., Ortiz, M., and West, M. Variational time integrators. International Journal for Numerical Methods in Engineering, 60, 153–212 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  15. Kane, C., Marsden, J. E., and Ortiz, M. Symplectic-energy-momentum preserving variational integrators. Journal of Mathematical Physics, 40, 3353–3371 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  16. Cortés, J. and Martínez, S. Non-holonomic integrators. Nonlinearity, 14, 1365–1392 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  17. Leyendecker, S., Marsden, J. E., and Ortiz, M. Variational integrators for constrained dynamical systems. Journal of Applied Mathematics and Mechanics, 88, 677–708 (2008)

    MathSciNet  MATH  Google Scholar 

  18. Leyendecker, S., Ober-Blöbaum, S., Marsden, J. E., and Ortiz, M. Discrete mechanics and optimal control for constrained systems. Optimal Control Applications and Methods, 31, 505–528 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  19. Kobilarov, M., Marsden, J. E., and Sukhatme, G. S. Geometric discretization of nonholonomic systems with symmetries. Discrete and Continuous Dynamical Systems Series S, 1, 61–84 (2010)

    MathSciNet  MATH  Google Scholar 

  20. Kane, C., Marsden, J. E., Ortiz, M., and West, M. Variational integrators and the Newmark algorithm for conservative and dissipative mechanical systems. International Journal for Numerical Methods in Engineering, 49, 1295–1325 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  21. Bou-Rabee, N. and Owhadi, H. Stochastic variational integrators. IMA Journal of Numerical Analysis, 29, 421–443 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  22. Bou-Rabee, N. and Owhadi, H. Long-run accuracy of variational integrators in the stochastic context. SIAM Journal on Numerical Analysis, 48, 278–297 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  23. Mata, P. and Lew, A. J. Variational integrators for the dynamics of thermo-elastic solids with finite speed thermal waves. Journal of Computational Physics, 257, 1423–1443 (2014)

    Article  MathSciNet  Google Scholar 

  24. Ober-Blöbaum, S., Tao, M., Cheng, M., Owhadi, H., and Marsden, J. E. Variational integrators for electric circuits. Journal of Computational Physics, 242, 498–530 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  25. Webb., S. D. Symplectic integration of magnetic systems. Journal of Computational Physics, 270, 570–576 (2014)

    Article  MathSciNet  Google Scholar 

  26. Lall, S. and West, M. Discrete variational Hamiltonian mechanics. Journal of Physics A, 39, 5509–5519 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  27. Leok, M. and Zhang, J. Discrete Hamiltonian variational integrators. IMA Journal of Numerical Analysis, 31, 1497–1532 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  28. Luo, E., Huang, W. J., and Zhang, H. X. Unconventional Hamilton-type variational principle in phase space and symplectic algorithm. Science China Physics, Mechanics and Astronomy, 46, 248–258 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  29. Gao, Q., Tan, S. J., Zhang, H. W., and Zhong, W. X. Symplectic algorithms based on the principle of least action and generating functions. International Journal for Numerical Methods in Engineering, 89, 438–508 (2012)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Engineering, Sun Yat-sen University, Guangzhou, 510275, China

    Minghui Fu & Kelang Lu

  2. Guangdong Provincial Academy of Building Research Group Company Limited, Guangzhou, 510500, China

    Kelang Lu

  3. College of Electromechanical Engineering, Guangdong Polytechnic Normal University, Guangzhou, 510635, China

    Weihua Li

  4. Faculty of Mechanics and Mathematics, Lomonosov Moscow State University, Moscow, 119992, Russia

    S. V. Sheshenin

Authors
  1. Minghui Fu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Kelang Lu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Weihua Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. S. V. Sheshenin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Weihua Li.

Additional information

Project supported by the National Natural Science Foundation of China (Nos. 11172334 and 11202247) and the Fundamental Research Funds for the Central Universities (No. 2013390003161292)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Fu, M., Lu, K., Li, W. et al. New way to construct high order Hamiltonian variational integrators. Appl. Math. Mech.-Engl. Ed. 37, 1041–1052 (2016). https://doi.org/10.1007/s10483-016-2116-8

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10483-016-2116-8

Key words

Chinese Library Classification

2010 Mathematics Subject Classification

Search

Navigation