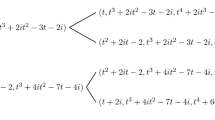Abstract
Let \(\mathbb{K}\) be an algebraically closed field and let \(X\subset\mathbb{K}^m\) be an n-dimensional affine variety. Assume that f1,...,f k are polynomials which have no common zeros on X. We estimate the degrees of polynomials \(A_i\in\mathbb{K}[X]\) such that 1=∑ki=1A i f i on X. Our estimate is sharp for k≤n and nearly sharp for k>n. Now assume that f1,...,f k are polynomials on X. Let \(I=(f_1,\dots,f_k)\subset\mathbb{K}[X]\) be the ideal generated by f i . It is well-known that there is a number e(I) (the Noether exponent) such that √Ie(I)⊂I. We give a sharp estimate of e(I) in terms of n, deg X and deg f i . We also give similar estimates in the projective case. Finally we obtain a result from the elimination theory: if \(f_1,\dots,f_n\in\mathbb{K}[x_1,\dots,x_n]\) is a system of polynomials with a finite number of common zeros, then we have the following optimal elimination:
where \({\deg} f_jg_{ij}\le\prod^n_{i=1}\deg f_i\).
Similar content being viewed by others
References
Brownawell, W.D.: A pure power product version of the Hilbert Nullstellensatz. Mich. Math. J. 45, 581–597 (1998)
Brownawell, W.D.: Bound for the degree in the Nullstellensatz. Ann. Math. 126, 577–591 (1987)
Ein, L., Lazarsfeld, R.: A geometric effective Nullstellensatz. Invent. Math. 137, 427–448 (1999)
Hartshorne, R.: Algebraic Geometry. Berlin, Heidelberg, New York: Springer 1997
Jelonek, Z.: Testing sets for properness of polynomial map**s. Math. Ann. 315, 1–35 (1999)
Jelonek, Z.: Topological characterization of finite map**s. Bull. Pol. Acad. Sci., Math. 49, 375–379 (2001)
Jelonek, Z.: On the Łojasiewicz exponent. To appear
Kollár, J.: Sharp effective Nullstellensatz. J. Am. Math. Soc. 1, 963–975 (1988)
Kollár, J.: Effective Nullstellensatz for arbitrary ideals. J. Eur. Math. Soc. (JEMS) 1, 313–337 (1999)
Perron, O.: Algebra I (Die Grundlagen). Berlin, Leipzig: Walter de Gruyter 1927
Sombra, M.: A sparse effective Nullstellensatz. Adv. Appl. Math. 22, 271–295 (1999)
Author information
Authors and Affiliations
Corresponding author
Additional information
Dedicated to Professor Arkadiusz Płoski
Mathematics Subject Classification (1991)
14D06, 14Q20
Rights and permissions
About this article
Cite this article
Jelonek, Z. On the effective Nullstellensatz. Invent. math. 162, 1–17 (2005). https://doi.org/10.1007/s00222-004-0434-8
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00222-004-0434-8