SpringerLink
Log in

Partition Function Zeros at First-Order Phase Transitions: A General Analysis

  • Published:
Communications in Mathematical Physics Aims and scope Submit manuscript

Abstract

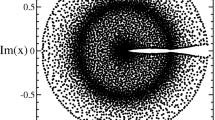
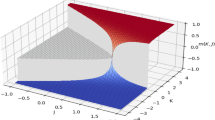
We present a general, rigorous theory of partition function zeros for lattice spin models depending on one complex parameter. First, we formulate a set of natural assumptions which are verified for a large class of spin models in a companion paper [5]. Under these assumptions, we derive equations whose solutions give the location of the zeros of the partition function with periodic boundary conditions, up to an error which we prove is (generically) exponentially small in the linear size of the system. For asymptotically large systems, the zeros concentrate on phase boundaries which are simple curves ending in multiple points. For models with an Ising-like plus-minus symmetry, we also establish a local version of the Lee-Yang Circle Theorem. This result allows us to control situations when in one region of the complex plane the zeros lie precisely on the unit circle, while in the complement of this region the zeros concentrate on less symmetric curves.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Beraha, S., Kahane, J., Weiss, N.J.: Limits of zeroes of recursively defined families of polynomials. In: G.-C. Rota (ed.), Studies in Foundations and Combinatorics (Advances in Mathematics Supplementary Studies, Vol. 1), New York: Academic Press, 1978, pp. 213–232

  2. Biskup, M., Borgs, C., Chayes, J.T., Kleinwaks, L.J., Kotecký, R.: General theory of Lee-Yang zeros in models with first-order phase transitions. Phys. Rev. Lett. 84:21, 4794–4797 (2000)

    Google Scholar 

  3. Biskup, M., Borgs, C., Chayes, J.T., Kotecký, R.: Phase diagrams of Potts models in external fields: I. Real fields. In preparation

  4. Biskup, M., Borgs, C., Chayes, J.T., Kotecký, R.: Phase diagrams of Potts models in external fields: II. One complex field. In preparation

  5. Biskup, M., Borgs, C., Chayes, J.T., Kotecký, R.: Partition function zeros at first-order phase transitions: Pirogov-Sinai theory. J. Stat. Phys. 116, 97–155 (2004)

    Article  Google Scholar 

  6. Borgs, C., Imbrie, J.Z.: A unified approach to phase diagrams in field theory and statistical mechanics. Commun. Math. Phys. 123, 305–328 (1989)

    MathSciNet  MATH  Google Scholar 

  7. Borgs, C., Kotecký, R.: A rigorous theory of finite-size scaling at first-order phase transitions. J. Statist. Phys. 61, 79–119 (1990)

    MathSciNet  Google Scholar 

  8. Chang, S.-C., Shrock, R.: Ground state entropy of the Potts antiferromagnet on strips of the square lattice. Physica A 290, 402–430 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  9. Chang, S.-C., Shrock, R.: T=0 partition functions for Potts antiferromagnets on lattice strips with fully periodic boundary conditions. Physica A 292, 307–345 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  10. Chen, C.-N., Hu, C.-K., Wu, F.Y.: Partition function zeros of the square lattice Potts model. Phys. Rev. Lett.76, 169–172 (1996)

    Google Scholar 

  11. Dobrushin, R.L.: Estimates of semiinvariants for the Ising model at low temperatures. In: R.L. Dobrushin et al. (ed.), Topics in statistical and theoretical physics. F. A. Berezin memorial volume, Transl. Ser. 2, Vol. 177(32), Providence: Am. Math. Soc. (1996) pp. 59–81

  12. Doland, B.P., Johnston, D.A.: One dimensional Potts model, Lee-Yang edges, and chaos. Phys. Rev. E 65, 057103 (2002)

    Article  Google Scholar 

  13. Federer, H.: Geometric Measure Theory. Berlin: Springer-Verlag, 1996

  14. Fisher, M.E.: The nature of critical points. In: W.E. Brittin (ed.), Lectures in Theoretical Physics, Vol 7c (Statistical physics, weak interactions, field theory), Boulder: University of Colorado Press, 1965, pp. 1–159

  15. Friedli, S., Pfister, C.-E.: On the singularity of the free energy at first order phase transitions. Commun. Math. Phys. 245, 69–103 (2004)

    Article  MATH  Google Scholar 

  16. Gamelin, T.W.: Complex Analysis. Undergraduate Texts in Mathematics, New York: Springer-Verlag, 2001

  17. Gibbs, J.W.: Elementary Principles of Statistical Mechanics. In: J.W. Gibbs (ed.), The Collected Works, Vol. II, New Haven, CT: Yale University Press, 1948

  18. Glumac, Z., Uzelac, K.: The partition function zeros in the one-dimensional q-state Potts model. J. Phys. A: Math. Gen. 27, 7709–7717 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  19. Isakov, S.N.: Nonanalytic features of the first-order phase transition in the Ising model. Commun. Math. Phys. 95, 427–443 (1984)

    MathSciNet  Google Scholar 

  20. Janke, W., Kenna, R.: Phase transition strengths from the density of partition function zeroes. Nucl. Phys. Proc. Suppl. 106, 905–907 (2002)

    Article  Google Scholar 

  21. Kenna, R., Lang, C.B.: Scaling and density of Lee-Yang zeros in the four-dimensional Ising model. Phys. Rev. E 49, 5012–5017 (1994)

    Article  Google Scholar 

  22. Kim, S.-Y., Creswick, R.J.: Yang-Lee zeros of the q-state Potts model in the complex magnetic field plane. Phys. Rev. Lett. 81, 2000–2003 (1998)

    Article  MathSciNet  Google Scholar 

  23. Kotecký, R., Preiss, D.: Cluster expansion for abstract polymer models. Commun. Math. Phys. 103, 491–498 (1986)

    MathSciNet  Google Scholar 

  24. Lee, K.-C.: Generalized circle theorem on zeros of partition functions at asymmetric first-order transitions. Phys. Rev. Lett. 73, 2801–2804 (1994)

    Article  Google Scholar 

  25. Lee, T.D., Yang, C.N.: Statistical theory of equations of state and phase transitions: II. Lattice gas and Ising model. Phys. Rev. 87, 410–419 (1952)

    Article  MATH  Google Scholar 

  26. Lieb, E.H., Sokal, A.D.: A general Lee-Yang theorem for one-component and multi-component ferromagnets. Commun. Math. Phys. 80, 153–179 (1981)

    MathSciNet  Google Scholar 

  27. Lu, W.T., Wu, F.Y.: Partition function zeroes of a self-dual Ising model. Physica A 258, 157–170 (1998)

    Article  MathSciNet  Google Scholar 

  28. Matveev, V., Shrock, R.: Complex-temperature properties of the Ising model on 2D heteropolygonal lattices. J. Phys.A: Math. Gen. 28, 5235–5256 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  29. Matveev, V., Shrock, R.: Some new results on Yang-Lee zeros of the Ising model partition function. Phys. Lett. A 215, 271–279 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  30. Milnor, J.W.: Topology from the Differentiable Viewpoint. Princeton Landmarks in Mathematics, Princeton, NJ: Princeton University Press, 1997

  31. Nashimori, H., Griffiths, R.B.: Structure and motion of the Lee-Yang zeros. J. Math. Phys. 24, 2637–2647 (1983)

    Article  Google Scholar 

  32. Newman, C.M.: Zeros of the partition function for generalized Ising systems. Commun. Pure Appl. Math. 27, 143–159 (1974)

    Google Scholar 

  33. Pirogov, S.A., Sinai, Ya.G.: Phase diagrams of classical lattice systems. (Russian), Theor. Math. Phys. 25(3), 358–369 (1975)

    Google Scholar 

  34. Pirogov, S.A., Sinai, Ya.G.: Phase diagrams of classical lattice systems. Continuation. (Russian), Theor. Math. Phys. 26(1), 61–76 (1976)

    Google Scholar 

  35. Ruelle, D.: Extension of the Lee-Yang circle theorem. Phys. Rev. Lett. 26, 303–304 (1971)

    Article  Google Scholar 

  36. van Saarloos, W., Kurtze, D.A.: Location of zeros in the complex temperature plane: Absence of Lee-Yang theorem. J. Phys. A: Math. Gen. 18, 1301–1311 (1984)

    Article  Google Scholar 

  37. Salas, J., Sokal, A.D.: Transfer matrices and partition-function zeros for antiferromagnetic Potts models. I. General theory and square-lattice chromatic polynomial, J. Stat. Phys. 104(3–4), 609–699 (2001)

    Google Scholar 

  38. Shrock, R.: Exact Potts model partition functions on ladder graphs. Physica A 283, 388–446 (2000)

    Article  MathSciNet  Google Scholar 

  39. Shrock, R., Tsai, S.-H.: Exact partition functions for Potts antiferromagnets on cyclic lattice strips. Physica A 275, 429–449 (2000)

    Article  MathSciNet  Google Scholar 

  40. Sokal, A.D.: Bounds on the complex zeros of (di)chromatic polynomials and Potts-model partition functions. Combin. Probab. Comput. 10(1), 41–77 (2001)

    MATH  Google Scholar 

  41. Sokal, A.D.: Chromatic roots are dense in the whole complex plane. Combin. Probab. Comput. 13, 221–261 (2004)

    Article  Google Scholar 

  42. Yang, C.N., Lee, T.D.: Statistical theory of equations of state and phase transitions: I. Theory of condensation. Phys. Rev. 87, 404–409 (1952)

    Article  MATH  Google Scholar 

  43. Zahradník, M.: An alternate version of Pirogov-Sinai theory. Commun. Math. Phys. 93, 559–581 (1984)

    MathSciNet  Google Scholar 

  44. Zahradník, M.: Analyticity of low-temperature phase diagrams of lattice spin models. J. Stat. Phys. 47, 725–455 (1987)

    MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, UCLA, Los Angeles, CA, 90095-1555, USA

    M. Biskup

  2. Microsoft Research, One Microsoft Way, Redmond, WA, 98052, USA

    C. Borgs & J.T. Chayes

  3. Department of Physics, Princeton University, Princeton, NJ, 08544, USA

    L.J. Kleinwaks

  4. Center for Theoretical Study, Charles University, Prague, 110 00, Czech Republic

    R. Kotecký

Authors
  1. M. Biskup
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. C. Borgs
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. J.T. Chayes
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. L.J. Kleinwaks
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. R. Kotecký
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Communicated by M. Aizenman

Reproduction of the entire article for non-commercial purposes is permitted without charge.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Biskup, M., Borgs, C., Chayes, J. et al. Partition Function Zeros at First-Order Phase Transitions: A General Analysis. Commun. Math. Phys. 251, 79–131 (2004). https://doi.org/10.1007/s00220-004-1169-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00220-004-1169-5

Keywords

Search

Navigation