SpringerLink
Log in

Progress in Initial-Boundary Value Problems for Nonlinear Evolution Equations and the Fokas Method

  • Conference paper
  • First Online:
Chaos, Fractals and Complexity (COSA-Net 2022)

Part of the book series: Springer Proceedings in Complexity ((SPCOM))

  • 319 Accesses

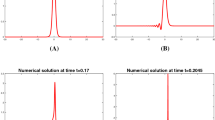
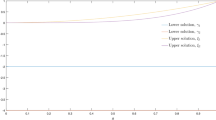
Abstract

The unified transform method (UTM), also known as the Fokas method [13], provides a novel approach for solving initial-boundary value problems (ibvp) for linear and integrable nonlinear partial differential equations. In particular, it gives solution formulas for forced linear ibvp. This motivated the initiation of a new program (by Fokas and collaborators) for studying the well-posedness in Sobolev spaces of ibvp for nonlinear evolution equations by employing this method, which is analogous to the way well-posedness of initial value problems (ivp) are studied. Using the Fokas formula we are able to derive linear estimates in Sobolev, Hadamard and Bourgain spaces. Then, using as an iteration map the one defined by the UTM formula when the forcing is replaced by the nonlinearity, and utilizing the linear estimates in combination with the multilinear estimates suggested by the nonlinearity we show that this map is a contraction in appropriate solution spaces. For the Korteweg-de Vries (KdV) and Nonlinear Schrödinger (NLS) equations this program has been implemented for ibvp in one-space dimension [16, 17, 29] and significant progress has been made in higher dimensions [24]. In this review we will try to present key points of this remarkable story. It is based on collaborative work with A. Fokas, D. Mantzavinos and F. Yan.

Dedicated to Professor Athanassios S. Fokas

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Chapter
EUR 29.95
Price includes VAT (Germany)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
eBook
EUR 149.79
Price includes VAT (Germany)
  • Available as EPUB and PDF
  • Read on any device
  • Instant download
  • Own it forever
Hardcover Book
EUR 192.59
Price includes VAT (Germany)
  • Durable hardcover edition
  • Dispatched in 3 to 5 business days
  • Free ship** worldwide - see info

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Similar content being viewed by others

References

  1. Batal, A., Fokas, A.S., Özsari, T.: Fokas method for linear boundary value problems involving mixed spatial derivatives. Proc. A. 476(2239), 20200076, 15 pp (2020)

    Google Scholar 

  2. Bona, J.L., Sun, S.M., Zhang, B.-Y.: A non-homogeneous boundary-value problem for the Korteweg-de Vries equation in a quarter plane. Trans. Amer. Math. Soc. 354(2), 427–490 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  3. Bona, J.L., Sun, S.M., Zhang, B.-Y.: A nonhomogeneous boundary-value problem for the Korteweg-de Vries equation posed on a finite domain. Commun. Partial Diff. Equ. 28(7–8), 1391–1436 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  4. Bona, J.L., Sun, S.M., Zhang, B.-Y.: Nonhomogeneous boundary value problems of one-dimensional nonlinear Schrödinger equations. J. Math. Pures Appl. 109, 1–66 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  5. Bourgain, J.: Fourier transform restriction phenomena for certain lattice subsets and applications to nonlinear evolution equations. Part II: The KdV equation. Geom. Funct. Anal. 3(3), 209–262 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  6. Bourgain, J.: Fourier transform restriction phenomena for certain lattice subsets and applications to nonlinear evolution equations. Geom. Funct. Anal. 3(2), 107–156 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  7. Colliander, J., Keel, M., Staffilani, G., Takaoka, H., Tao, T.: Sharp global well-posedness for KdV and modified KdV on \(\mathbb{R} \) and \(\mathbb{T} \). J. Amer. Math. Soc. 16(3), 705–749 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  8. Colliander, J.E., Kenig, C.E.: The generalized Korteweg-de Vries equation on the half-line. Commun. Partial Diff. Equ. 27(11–12), 2187–2266 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  9. Deconinck, B., Trogdon, T., Vasan, V.: The method of Fokas for solving linear partial differential equations. SIAM Rev. 56(1), 159–186 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  10. Erdoǧan, M.B., Tzirakis, N.: Regularity properties of the cubic nonlinear Schrödinger equation on the half line. J. Funct. Anal. 271, 2539–2568 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  11. Faminskii, A.V.: An initial boundary-value problem in a half-strip for the Korteweg-de Vries equation in fractional-order Sobolev spaces (English summary). Commun. Partial Diff. Equ. 29(11–12), 1653–1695 (2004)

    Google Scholar 

  12. Figueira, R., Himonas, A., Yan, F.: A higher dispersion KdV equation on the line. Nonlinear Anal. 199, 112055, 38 pp (2020)

    Google Scholar 

  13. Fokas, A.S.: A unified transform method for solving linear and certain nonlinear PDEs. Proc. Roy. Soc. Lond. Ser. A 453(1962), 1411–1443 (1997)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  14. Fokas, A.S.: A new transform method for evolution partial differential equations. IMA J. Appl. Math. 67(6), 559–590 (2002)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  15. Fokas, A.S.: A unified approach to boundary value problems. In: CBMS-NSF Regional Conference Series in Applied Mathematics, 78, xvi+336 pp. Society for Industrial and Applied Mathematics (SIAM), Philadelphia, PA (2008)

    Google Scholar 

  16. Fokas, A.S., Himonas, A.A., Mantzavinos, D.: The Korteweg-de Vries equation on the half-line. Nonlinearity 29(2), 489–527 (2016)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  17. Fokas, A.S., Himonas, A.A., Mantzavinos, D.: The nonlinear Schrödinger equation on the half-line. Trans. Amer. Math. Soc. 369(1), 681–709 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  18. Fokas, A.S., Its, A.R., Sung, L.-Y.: The nonlinear Schrödinger equation on the half-line. Nonlinearity 18(4), 1771–1822 (2005)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  19. Fokas, A.S., Lenells, J.: The unified method: I. Nonlinearizable problems on the half-line. J. Phys. A 45(19), 195201, 38 pp (2012)

    Google Scholar 

  20. Fokas, A.S., Pelloni, B.: Introduction. Unified Transform for Boundary Value Problems, pp. 1–9. SIAM, Philadelphia, PA (2015)

    Google Scholar 

  21. Fokas, A.S., Spence, E.: Synthesis, as opposed to separation, of variables. SIAM Rev. 54(2), 291–324 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  22. Gardner, C.S., Greene, J.M., Kruskal, M.D., Miura, R.M.: Method for solving the Korteweg-de Vries equation. Phys. Rev. Lett. 19(19), 1095–1097 (1967)

    Article  ADS  MATH  Google Scholar 

  23. Himonas, A.A., Mantzavinos, D.: The “good’’ Boussinesq equation on the half-line. J. Differ. Equ. 258(9), 3107–3160 (2015)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  24. Himonas, A.A., Mantzavinos, D.: Well-posedness of the nonlinear Schrödinger equation on the half-plane. Nonlinearity 33, 5567–5609 (2020)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  25. Himonas, A.A., Mantzavinos, D.: The nonlinear Schrödinger equation on the half-line with a Robin boundary condition. Anal. Math. Phys. 11(4), Paper No. 157 (2021)

    Google Scholar 

  26. Himonas, A.A., Mantzavinos, D.: The Robin and Neumann Problems for the Nonlinear Schrödinger Equation on the Half-Plane. Proc. A. 478(2265), Paper No. 279, 20 pp (2022)

    Google Scholar 

  27. Himonas, A.A., Mantzavinos, D., Yan, F.: The Korteweg-de Vries equation on an interval. J. Math. Phys. 60(5), 051507, 26 pp (2019)

    Google Scholar 

  28. Himonas, A.A., Yan, F.: The Korteweg-de Vries equation on the half-line with Robin and Neumann data in low regularity spaces. Nonlinear Anal. 222, 113008, 31 pp (2022)

    Google Scholar 

  29. Himonas, A.A., Yan, F.: A higher dispersion KdV equation on the half-line. J. Diff. Equ. 333(5), 55–102 (2022)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  30. Holmer, J.: The initial-boundary-value problem for the 1D nonlinear Schrödinger equation on the half-line. Diff. Integral Equ. 18(6), 647–668 (2005)

    MATH  Google Scholar 

  31. Holmer, J.: The initial-boundary-value problem for the Korteweg-de Vries equation. Commun. Partial Diff. Equ. 31(8), 1151–1190 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  32. Kenig, C.E., Ponce, G., Vega, L.: On the (generalized) Korteweg-de Vries equation. Duke Math. J. 59(3), 585–610 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  33. Kenig, C.E., Ponce, G., Vega, L.: Oscillatory integrals and regularity of dispersive equations. Indiana Univ. Math. J. 40(1), 33–69 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  34. Kenig, C.E., Ponce, G., Vega, L.: Well-posedness of the initial value problem for the Korteweg-de Vries equation. J. Amer. Math. Soc. 4(2), 323–347 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  35. Kenig, C.E., Ponce, G., Vega, L.: Well-posedness and scattering results for the generalized Korteweg-de Vries equation via the contraction principle. Commun. Pure Appl. Math. 46(4), 527–620 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  36. Kenig, C.E., Ponce, G., Vega, L.: A bilinear estimate with applications to the KdV equation. J. Amer. Math. Soc. 9(2), 573–603 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  37. Kenig, C.E., Ponce, G., Vega, L.: On the ill-posedness of some canonical dispersive equations. Duke Math. J. 106(3), 617–633 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  38. Killip, R., Visan, M.: KdV is well-posed in \(H^{-1}\). Ann. of Math. (2) 190(1), 249–305 (2019)

    Google Scholar 

  39. Korteweg, D.J., de Vries, G.: On the change of form of long waves advancing in a rectangular canal, and on a new type of long stationary waves. Phil. Mag. Ser. 5 39(240), 422–443 (1895)

    Google Scholar 

  40. Lax, P.D.: Integrals of nonlinear equations of evolution and solitary waves. Commun. Pure Appl. Math. 21, 467–490 (1968)

    Article  MathSciNet  MATH  Google Scholar 

  41. Lenells, J.: The KdV equation on the half-line: the Dirichlet to Neumann map. J. Phys. A 46(34), 345203, 20 pp (2013)

    Google Scholar 

  42. Lenells, J., Fokas, A.S.: The unified method: II. NLS on the half-line with \(t\)-periodic boundary conditions. J. Phys. A 45(19), 195202, 36 pp (2012)

    Google Scholar 

  43. Özsari, T., Yolcu, N.: The initial-boundary value problem for the biharmonic Schrödinger equation on the half-line. Commun. Pure Appl. Anal. 18(6), 3285–3316 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  44. Saut, J.-C., Temam, R.: Remarks on the Korteweg-de Vries equation. Israel J. Math. 24(1), 78–87 (1976)

    Article  MathSciNet  MATH  Google Scholar 

  45. Yan, F.: Well-posedness of a higher dispersion KdV equation on the half-line. J. Math. Phys. 61(8), 081506, 29 pp (2020)

    Google Scholar 

  46. Zabusky, N.J., Kruskal, M.D.: Interaction of solitons in a collisionless plasma and the recurrence of initial states. Phys. Rev. Lett. 15(6), 240–243 (1965)

    Article  ADS  MATH  Google Scholar 

Download references

Acknowledgements

The author was partially supported by a grant from the Simons Foundation (#524469 to Alex Himonas).

Author information

Authors and Affiliations

  1. University of Notre Dame, Notre Dame, IN, 46556, USA

    A. Alexandrou Himonas

Authors
  1. A. Alexandrou Himonas
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to A. Alexandrou Himonas .

Editor information

Editors and Affiliations

  1. Mathematics, University of Patras, Patras, Greece

    Tassos Bountis

  2. Geology and Geoenvironment, National and Kapodistrian University of Athens, Athens, Greece

    Filippos Vallianatos

  3. Institute of Nanoscience and Nanotechnology, National Center for Scientific Research Demokritos, Agia Paraskevi, Athens, Greece

    Astero Provata

  4. Electrical and Computer Engineering, Aristotle University of Thessaloniki, Thessaloniki, Greece

    Dimitris Kugiumtzis

  5. School of Applied Mathematical and Physical Sciences, National Technical University of Athens, Athens, Greece

    Yannis Kominis

Rights and permissions

Reprints and permissions

Copyright information

© 2023 The Author(s), under exclusive license to Springer Nature Switzerland AG

About this paper

Check for updates. Verify currency and authenticity via CrossMark

Cite this paper

Himonas, A.A. (2023). Progress in Initial-Boundary Value Problems for Nonlinear Evolution Equations and the Fokas Method. In: Bountis, T., Vallianatos, F., Provata, A., Kugiumtzis, D., Kominis, Y. (eds) Chaos, Fractals and Complexity. COSA-Net 2022. Springer Proceedings in Complexity. Springer, Cham. https://doi.org/10.1007/978-3-031-37404-3_19

Download citation

Publish with us

Policies and ethics

Search

Navigation