Free Vibration Investigation of Single-Phase Porous FG Sandwich Cylindrical Shells: Analytical, Numerical and Experimental Study

Abstract

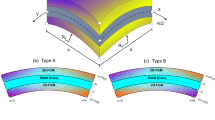
This paper offers new analytical, numerical, and experimental methods for nonlinear free vibration analysis of single-phase functionally graded (FG) porous sandwich panels that are simply supported with cylindrical shell panels using the first-order shear deflection theory. This innovative sandwich shell comprises a single porous polymer core and two uniform skins that have not been previously considered into the vibration analysis, making it highly applicable in diverse fields, such as aircraft structures, biomedical engineering, and defense technology. The properties of the core metal are assumed to depend on the porosity and grade in the direction of thickness, with a power-law distribution concerning the volume fractions of the constituents. This study involved performing free vibration experiments on three-dimensional (3D printed) FGM shells. To validate the analytical solution, a numerical study was carried out employing modal analysis and finite element analysis with the help of ANSYS-2021-R1 software. The objective of this research is to study the impact of various critical factors, including power-law index, porous ratio, FG core thickness, skin thickness, different boundary conditions, and radius of curvature on the natural frequencies and transient deflection response. The findings manifested that the frequency parameter of sandwich shell is positively correlated with both the number of constraints in the boundary conditions and the porosity factor. It is observed that there is an acceptable level of agreement between the suggested analytical procedure and the numerical findings, with a maximum error difference of only 6.7%.

Appendix

$$ \begin{gathered} I_{10} = \frac{{E_{1} }}{{1 - \upsilon^{2} }},I_{20} = \frac{{\upsilon E_{1} }}{{1 - \upsilon^{2} }},I_{30} = \frac{{E_{1} }}{{2\left( {1 + \upsilon } \right)}}, \hfill \\ I_{11} = \frac{{E_{2} }}{{1 - \upsilon^{2} }},I_{21} = \frac{{\upsilon E_{2} }}{{1 - \upsilon^{2} }},I_{31} = \frac{{E_{2} }}{{2\left( {1 + \upsilon } \right)}}, \hfill \\ I_{12} = \frac{{E_{3} }}{{1 - \upsilon^{2} }},I_{22} = \frac{{\upsilon E_{3} }}{{1 - \upsilon^{2} }},I_{32} = \frac{{E_{3} }}{{2\left( {1 + \upsilon } \right)}}, \hfill \\ \end{gathered} $$

(32)

$$ A_{11} = \frac{{I_{10} }}{\Delta },A_{22} = \frac{{I_{10} }}{\Delta },A_{12} = \frac{{I_{20} }}{\Delta },A_{66} = \frac{1}{{I_{30} }}, $$

(33)

$$ \Delta = I_{10}^{2} - I_{20}^{2} ,B_{11} = A_{22} I_{11} - A_{12} I_{21} ,B_{22} = A_{11} I_{11} - A_{12} I_{21} , $$

(34)

$$ \begin{gathered} B_{12} = A_{22} I_{21} - A_{12} A_{12} , \hfill \\ B_{21} = A_{11} I_{21} - A_{12} I_{11} , \hfill \\ B_{66} = \frac{{I_{31} }}{{I_{30} }}, \hfill \\ \end{gathered} $$

(35)

$$ \begin{gathered} D_{11} = I_{12} - B_{11} B_{12} - I_{21} B_{21} , \hfill \\ D_{22} = I_{22} - B_{22} I_{11} - I_{21} B_{12} , \hfill \\ D_{12} = I_{22} - B_{12} I_{11} - I_{21} B_{22} , \hfill \\ D_{21} = I_{22} - B_{21} I_{11} - I_{21} B_{11} , \hfill \\ D_{66} = I_{32} - I_{31} B_{66} , \hfill \\ \end{gathered} $$

(36)

$$ \begin{gathered} {\rm T}_{11} \left( w \right) = K_{s} I_{30} \frac{{\partial^{2} w}}{{\partial x^{2} }} + K_{s} I_{30} \frac{{\partial^{2} w}}{{\partial y^{2} }}, \hfill \\ {\rm T}_{12} \left( {\phi_{x} } \right) = K_{s} I_{30} \frac{{\partial \phi_{x} }}{\partial x}, \hfill \\ {\rm T}_{13} \left( {\phi_{y} } \right) = K_{s} I_{30} \frac{{\partial \phi_{y} }}{\partial y}, \hfill \\ {\rm T}_{21} \left( w \right) = - K_{s} I_{30} \frac{\partial w}{{\partial x}}, \hfill \\ {\rm T}_{22} \left( {\phi_{x} } \right) = D_{11} \frac{{\partial^{2} \phi_{x} }}{{\partial x^{2} }} + D_{66} \frac{{\partial^{2} \phi_{y} }}{{\partial y^{2} }} - K_{s} I_{30} \phi_{x} , \hfill \\ {\rm T}_{23} \left( {\phi_{y} } \right) = \left( {D_{12} + D_{66} } \right)\frac{{\partial^{2} \phi_{y} }}{\partial x\partial y}, \hfill \\ {\rm T}_{33} \left( {\phi_{y} } \right) = D_{22} \frac{{\partial^{2} \phi_{y} }}{{\partial y^{2} }} + D_{66} \frac{{\partial^{2} \phi_{y} }}{{\partial x^{2} }} - K_{s} I_{30} \phi_{y} , \hfill \\ \end{gathered} $$

(37)

$$ \begin{gathered} R_{1} \left( {w,f} \right) = \frac{{\partial^{2} f}}{{\partial x^{2} }}\frac{{\partial^{2} w}}{{\partial x^{2} }} - 2\frac{{\partial^{2} f}}{\partial x\partial y}\frac{{\partial^{2} w}}{\partial x\partial y} + \frac{{\partial^{2} f}}{{\partial x^{2} }}\frac{{\partial^{2} w}}{{\partial y^{2} }} + \frac{1}{R}\frac{{\partial^{2} f}}{{\partial x^{2} }}, \hfill \\ R_{2} \left( f \right) = B_{21} \frac{{\partial^{3} f}}{{\partial x^{3} }} + \left( {B_{11} - B_{66} } \right)\frac{{\partial^{3} f}}{{\partial x\partial y^{2} }}, \hfill \\ R_{3} \left( f \right) = B_{12} \frac{{\partial^{3} f}}{{\partial y^{3} }} + \left( {B_{22} - B_{66} } \right)\frac{{\partial^{3} f}}{{\partial x^{2} \partial y}}, \hfill \\ \end{gathered} $$

(38)

$$ \begin{gathered} t_{11} = - \frac{{\lambda_{m}^{4} }}{{L_{26} R^{2} }},t_{12} = - \lambda_{m}^{2} \frac{{L_{27} }}{{L_{26} R}} - K_{s} I_{30} \lambda_{m} ,t_{13} = - \lambda_{m}^{2} \frac{{L_{28} }}{{L_{26} R}} - K_{s} I_{30} \delta_{n} , \hfill \\ t_{14} = \left( {L_{12} + L_{13} } \right) - \frac{{L_{25} L_{27} }}{{L_{26} }} - \frac{{L_{27} L_{29} }}{{L_{26} }},t_{15} = \left( {L_{14} + L_{15} } \right) - \frac{{L_{25} L_{28} }}{{L_{26} }} - \frac{{L_{28} L_{29} }}{{L_{26} }}, \hfill \\ t_{16} = \left( {L_{23} + L_{24} } \right)\Phi_{1} - K_{s} I_{30} \lambda_{m}^{2} - K_{s} I_{30} \delta_{n}^{2} ,t_{17} = \left( {L_{16} + L_{17} } \right) - \frac{{L_{25} \lambda_{m}^{2} }}{{L_{26} R}} - \frac{{L_{29} \lambda_{m}^{2} }}{{L_{26} R}} - L_{31} , \hfill \\ t_{18} = - \left( {L_{18} + L_{19} } \right) - \frac{1}{16}\left( {\frac{{\lambda_{m}^{2} }}{{A_{22} }} + \frac{{\delta_{n}^{2} }}{{A_{11} }}} \right),t_{21} = - B_{21} \frac{1}{S}\frac{{\lambda_{m}^{2} }}{R}\lambda_{m}^{3} - \left( {B_{11} - B_{66} } \right)\frac{1}{S}\frac{{\lambda_{m}^{2} }}{R}\lambda_{m} \delta_{n}^{2} , \hfill \\ t_{22} = - D_{11} \lambda_{m}^{2} - D_{66} \delta_{n}^{2} - K_{s} I_{30} - B_{21} \frac{\zeta }{S}\lambda_{m}^{3} - \left( {B_{11} - B_{66} } \right)\frac{\zeta }{S}\lambda_{m} \delta_{n}^{2} , \hfill \\ t_{23} = - \left( {D_{12} + D_{66} } \right)\lambda_{m} \delta_{n} - B_{21} \frac{\Psi }{S}\lambda_{m}^{3} - \left( {B_{11} - B_{66} } \right)\frac{\Psi }{S}\lambda_{m} \delta_{n}^{2} , \hfill \\ t_{31} = - B_{12} \frac{1}{S}\frac{{\lambda_{m}^{2} }}{R}\delta_{n}^{3} - \left( {B_{22} - B_{66} } \right)\frac{1}{S}\frac{{\lambda_{m}^{2} }}{R}\delta_{n} \lambda_{m}^{2} , \hfill \\ t_{32} = - \left( {D_{21} + D_{66} } \right)\lambda_{m} \delta_{n} - B_{12} \frac{\zeta }{S}\delta_{n}^{3} - \left( {B_{22} - B_{66} } \right)\frac{\zeta }{S}\lambda_{m}^{2} \delta_{n} , \hfill \\ t_{33} = - D_{22} \delta_{n}^{2} - D_{66} \lambda_{m}^{2} - K_{s} I_{30} - B_{12} \frac{\Psi }{S}\delta_{n}^{3} - \left( {B_{22} - B_{66} } \right)\frac{\Psi }{S}\lambda_{m}^{2} \delta_{n} , \hfill \\ \end{gathered} $$

(39)

$$ \begin{gathered} n_{1} = - K_{s} I_{30} \lambda_{m} ,n_{2} = - \frac{8}{3}\frac{{B_{21} }}{{A_{11} }}\frac{{\delta_{n} }}{ab}, \hfill \\ n_{3} = - K_{s} I_{30} \delta_{n} ,n_{4} = - \frac{8}{3}\frac{{B_{12} }}{{A_{22} }}\frac{{\lambda_{m} }}{ab}, \hfill \\ \end{gathered} $$

(40)

$$ \tilde{\rho }_{1} = \left( {{\rm I}_{2} - \frac{{{\rm I}_{1}^{2} }}{{{\rm I}_{0} }}} \right) $$

(41)

$$ \zeta = B_{21} \lambda_{m}^{3} + \left( {B_{11} - B_{66} } \right)\lambda_{m} \delta_{n}^{2} , $$

(42)

$$ \Psi = B_{12} \delta_{n}^{3} + \left( {B_{22} - B_{66} } \right)\lambda_{m}^{2} \delta_{n} , $$

(43)

$$ S = A_{11} \lambda_{m}^{4} + A_{22} \delta_{n}^{4} + \left( {A_{66} - 2A_{12} } \right)\lambda_{m}^{2} \delta_{n}^{2} , $$

(44)

$$ \begin{gathered} a_{1} = t_{11} + t_{12} \frac{{t_{23} t_{31} - t_{21} t_{33} }}{{t_{22} t_{33} - t_{32} t_{23} }} + t_{13} \frac{{t_{32} t_{21} - t_{22} t_{31} }}{{t_{22} t_{33} - t_{32} t_{23} }}, \hfill \\ a_{2} = t_{12} \frac{{n_{3} t_{23} - n_{1} t_{33} }}{{t_{22} t_{33} - t_{32} t_{23} }} + t_{13} \frac{{n_{1} t_{32} - n_{3} t_{22} }}{{t_{22} t_{33} - t_{32} t_{23} }} + t_{16} , \hfill \\ a_{3} = t_{14} \frac{{t_{23} t_{31} - t_{21} t_{33} }}{{t_{22} t_{33} - t_{32} t_{23} }} + t_{15} \frac{{t_{32} t_{21} - t_{22} t_{31} }}{{t_{22} t_{33} - t_{32} t_{23} }}, \hfill \\ a_{4} = t_{12} \frac{{n_{4} t_{23} - n_{2} t_{33} }}{{t_{22} t_{33} - t_{32} t_{23} }} + t_{13} \frac{{n_{2} t_{32} - n_{4} t_{22} }}{{t_{22} t_{33} - t_{32} t_{23} }}, \hfill \\ a_{5} = t_{14} \frac{{n_{4} t_{23} - n_{2} t_{33} }}{{t_{22} t_{33} - t_{32} t_{23} }} + t_{15} \frac{{n_{2} t_{32} - n_{4} t_{22} }}{{t_{22} t_{33} - t_{32} t_{23} }}, \hfill \\ a_{6} = t_{14} \frac{{n_{3} t_{23} - n_{1} t_{33} }}{{t_{22} t_{33} - t_{32} t_{23} }} + t_{15} \frac{{n_{1} t_{32} - n_{3} t_{22} }}{{t_{22} t_{33} - t_{32} t_{23} }}, \hfill \\ \end{gathered} $$

(45)

$$ \begin{gathered} L_{11} = \left( {A_{11} A_{22} - A_{12}^{2} } \right),L_{12} = \frac{{4\lambda_{m}^{2} \left( {B_{21} A_{12} + A_{11} B_{11} } \right)}}{{\delta_{n} abL_{11} }},L_{13} = \frac{{4\delta_{n} \left( {B_{11} A_{12} + A_{11} A_{22} B_{21} } \right)}}{{abL_{11} }}, \hfill \\ L_{14} = \frac{{4\lambda_{m} \left( {B_{22} A_{12} + A_{11} B_{12} } \right)}}{{abL_{11} }},L_{15} = \frac{{4\delta_{n}^{2} \left( {B_{12} A_{12} + A_{22} B_{22} } \right)}}{{\lambda_{m} abL_{11} }},L_{16} = \frac{{4\lambda_{m} A_{12} }}{{\delta_{n} RL_{11} }},L_{17} = \frac{{4\delta_{n} A_{22} }}{{\lambda_{m} RL_{11} }}, \hfill \\ L_{18} = \frac{{\lambda_{m}^{3} \delta_{n} \left( {\delta_{n}^{2} A_{12} + \lambda_{m}^{2} A_{22} } \right)}}{{8abL_{11} }},L_{19} = \frac{{\delta_{n}^{3} \lambda_{m} \left( {\lambda_{m}^{2} A_{12} + \delta_{n}^{2} A_{22} } \right)}}{{8abL_{11} }},L_{21} = \frac{{\lambda_{m}^{3} \delta_{n} \left( {\delta_{n}^{2} A_{12} + \lambda_{m}^{2} A_{11} } \right)}}{{4abL_{11} }}, \hfill \\ L_{22} = \frac{{\lambda_{m} \delta_{n}^{3} \left( {\lambda_{m}^{2} A_{12} + \delta_{n}^{2} A_{22} } \right)}}{{4abL_{11} }},L_{25} = \frac{{8\lambda_{m} \delta_{n} }}{ab}, \hfill \\ L_{26} = A_{11} \lambda_{m}^{4} + A_{22} \delta_{n}^{4} + \left( {A_{66} - 2A_{12} } \right)\lambda_{m}^{2} \delta_{n}^{2} ,L_{27} = B_{21} \lambda_{m}^{3} + \left( {B_{11} - B_{66} } \right)\lambda_{m} \delta_{n}^{2} , \hfill \\ L_{28} = B_{12} \delta_{n}^{3} + \left( {B_{22} - B_{66} } \right)\lambda_{m}^{2} \delta_{n} ,L_{29} = \frac{{16\lambda_{m}^{2} \delta_{n}^{2} }}{{3mn\pi^{2} }},L_{31} = \frac{{\delta_{n}^{2} }}{{3A_{11} mn\pi^{2} R}},L_{32} = \frac{16}{{mn\pi^{2} }}, \hfill \\ \end{gathered} $$

(46)