SpringerLink
Log in

Fitted Difference Scheme on a Non-uniform Mesh for Singularly Perturbed Parabolic Reaction–Diffusion with Large Negative Shift and Non-local Boundary Condition

  • Original Paper
  • Published:
International Journal of Applied and Computational Mathematics Aims and scope Submit manuscript

Abstract

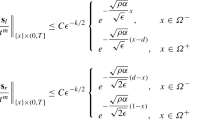
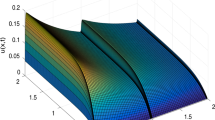
In this paper, we study the numerical solution of singularly perturbed parabolic reaction–diffusion problems with large delay in space, and the right end plane is non-local boundary condition. As the perturbation parameter approaches zero, the solution to this problem exhibits a parabolic boundary layers and an interior layer have been exhibited in the solution domain. To solve these problems, we develop a numerical scheme which combines the cubic spline scheme for the spatial derivatives, and backward difference scheme for the time derivative. To resolve the boundary layers, we use the piecewise uniform Shishkin types mesh (Standard Shishkin mesh, Bakhvalov–Shishkin mesh) for the spatial discretization. To treat the non-local boundary condition,numerical integration method is applied. A priori bounds for the solution and its derivatives of the continuous problem are given, which are necessary to analyze the error. Stability analysis and error estimates are obtained. Some numerical results are considered to support our theoretical result, which shows the \(\varepsilon \)-uniform convergent results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

Data Availability

No external data are used in this article.

References

  1. D’Huys, O., Vicente, R., Erneux, T., Danckaert, J., Fischer, I.: Synchronization properties of network motifs: influence of coupling delay and symmetry. Chaos Interdiscip. J. Nonlinear Sci. 18(3), 037116 458 (2008). https://doi.org/10.1063/1.2953582

    Article  MathSciNet  MATH  Google Scholar 

  2. Gupta, C., López, J.M., Ott, W., Josić, K., Bennett, M.R.: Transcriptional delay stabilizes bistable gene networks. Phys. Rev. Lett. 111, 058104 (2013). https://doi.org/10.1103/PhysRevLett.111.058104

    Article  Google Scholar 

  3. Bratsun, D., Sakharov, A.: Spatial effects of delay-induced stochastic oscillations in a multi-scale cellular system. In: Proceedings of ECCS 2014, European Conference on Complex Systems, pp. 93–103 (2016)

  4. Orosz, G., Wilson, R.E., Stépán, G.: Traffic jams: dynamics and control. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 368, 4455–4479 (1928). https://doi.org/10.1098/rsta.2010.0205

    Article  MathSciNet  MATH  Google Scholar 

  5. Erneux, T., Kalmár-Nagy, T.: Nonlinear stability of a delayed feedback controlled container crane. J. Vib. Control 13(5), 603–616 (2007). https://doi.org/10.1177/1077546307074245

    Article  MATH  Google Scholar 

  6. Szalai, R., Orosz, G.: Decomposing the dynamics of heterogeneous delayed networks with applications to connected vehicle systems. Phys. Rev. E 88, 040902 (2013). https://doi.org/10.1103/PhysRevE.88.040902

    Article  Google Scholar 

  7. Marconi, M., Javaloyes, J., Barland, S., Balle, S., Giudici, M.: Vectorial dissipative solitons in verticalcavity surface-emitting lasers with delays. Nat. Photon 9, 450–455 (2015)

    Article  Google Scholar 

  8. Franz, A.L., Roy, R., Shaw, L.B., Schwartz, I.B.: Effect of multiple time delays on intensity fluctuation dynamics in fibre ring lasers. Phys. Rev. E 78, 16208 (2008)

    Article  Google Scholar 

  9. Mackey, M.C., Glass, L.: Oscillation and chaos in physiological control systems. Sci. New Ser. 197, 287–289 (1977)

    MATH  Google Scholar 

  10. Kuang, Y.: Delay Differential Equations with Applications to Population Biology. Academic Press, New York (1993)

    Google Scholar 

  11. Erneux, T.: Applied Delay Differential Equations. Springer, New York (2009)

    MATH  Google Scholar 

  12. Chakravarthy, P.P., Kumar, S.D., Rao, R.N., Ghate, D.P.: A fitted numerical scheme for second order singularly perturbed delay differential equations via cubic spline in compression. Adv. Differ. Equ. 2015(1), 1–14 (2015)

    MathSciNet  MATH  Google Scholar 

  13. Chakravarthy, P.P., Kumar, S.D., Rao, R.N.: Numerical solution of second order singularly perturbed delay differential equations via cubic spline in tension. Int. J. Appl. Comput. Math. 3(3), 1703–1717 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  14. Kumar, N.S., Rao, R.N.: A second order stabilized central difference method for singularly perturbed differential equations with a large negative shift. Differ. Equ. Dyn. Syst. 1–18 (2020)

  15. Rai, P., Sharma, K.K.: Numerical approximation for a class of singularly perturbed delay differential equations with boundary and interior layer(s). Numer. Algorithms 85(1), 305–328 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  16. Chandru, M., Prabha, T., Shanthi, V.: A hybrid difference scheme for a second-order singularly perturbed reaction-diffusion problem with non-smooth data. Int. J. Appl. Comput. Math 1, 87–100 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  17. Bansal, K., Sharma, K.K.: Parameter-robust numerical scheme for time-dependent singularly perturbed reaction-diffusion problem with large delay. Numer. Funct. Anal. Optim. 39, 127–154 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  18. Chandru, M., Das, P., Ramos, H.: Numerical treatment of two-parameter singularly perturbed parabolic convection diffusion problems with non-smooth data. Math. Methods Appl Sci. (2018). https://doi.org/10.1002/mma.5067

    Article  MathSciNet  MATH  Google Scholar 

  19. Chandru, M., Prabha, T., Das, P., Shanthi, V.: A numerical method for solving boundary and interior layers dominated parabolic problems with discontinuous convection coefficient and source terms. Differ. Equ. Dyn. Syst. (2019). https://doi.org/10.1007/s12591-017-0385-3

    Article  MathSciNet  MATH  Google Scholar 

  20. Kaushik, A., Sharma, N.: An adaptive difference scheme for parabolic delay differential equation with discontinuous coefficients and interior layers. J. Differ. Equ. Appl. 26(11-12), 1450-248, 1470 (2020)

  21. Daba, I.T., Duressa, G.F.: Computational method for singularly perturbed parabolic differential equations with discontinuous coefficients and large delay. Heliyon 8, e10742 (2022). https://doi.org/10.1016/j.heliyon.2022.e10742

    Article  Google Scholar 

  22. Hailu, W.S., Duressa, G.F.: Uniformly convergent numerical method for singularly perturbed parabolic differential equations with non-smooth data and large negative shift. Res. Math. 9:1, 2119677 (2022). https://doi.org/10.1080/27684830.2022.2119677

    Article  MathSciNet  Google Scholar 

  23. Bahuguna, D., Dabas, J.: Existence and uniqueness of a solution to a semilinear partial delay differential equation with an integral condition. Non-linear Dyn. Syst. Theory 8(1), 7–19 (2008)

    MathSciNet  MATH  Google Scholar 

  24. Sekar, E., Tamilselvan, A.: Singularly perturbed delay differential equations of convection-diffusion type with integral boundary condition. J. Appl. Math. Comput. 59(1–2), 701–722 (2019). https://doi.org/10.1007/s12190-018-1198-4

    Article  MathSciNet  MATH  Google Scholar 

  25. Debela, H.G., Duressa, G.F.: Uniformly convergent numerical method for singularly perturbed convection-diffusion type problems with nonlocal boundary condition. Int. J. Numer. Methods Fluids (2020). https://doi.org/10.1002/fld.4854

    Article  MathSciNet  MATH  Google Scholar 

  26. Debela, H.G., Duressa, G.F.: Accelerated fitted operator finite difference method for singularly perturbed delay differential equations with non-local boundary conditio. J. Egypt. Math. Soc. 28(16), 1–16 (2020). https://doi.org/10.1186/s42787-020-00076-6

    Article  MATH  Google Scholar 

  27. Sharma, N., Kaushik, A.: A uniformly convergent difference method for singularly perturbed parabolic partial differential equations with large delay and integral boundary condition. J. Appl. Math. Comput. (2022). https://doi.org/10.1007/s12190-022-01783-2

    Article  MATH  Google Scholar 

  28. Hailu, W.S., Duressa, G.F.: Accelerated parameter-uniform numerical method for singularly perturbed parabolic convection-diffusion problems with a large negative shift and integral boundary condition. Results Appl. Math. 18, 100364 (2023). https://doi.org/10.1016/j.rinam.2023.100364

    Article  MathSciNet  MATH  Google Scholar 

  29. Elango, S., Tamilselvan, A., Vadivel, R.: Finite difference scheme for singularly perturbed reaction diffusion problem of partial delay differential equation with nonlocal boundary condition. Adv. Differ. Equ. 2021, 151 (2021). https://doi.org/10.1186/s13662-021-03296-x

    Article  MathSciNet  MATH  Google Scholar 

  30. Gobena, W.T., Duressa, G.F.: Parameter-uniform numerical scheme for singularly perturbed delay parabolic reaction diffusion equations with integral boundary condition. Int. J. Differ. Equ. Article ID 9993644 (2021) https://doi.org/10.1155/2021/9993644

  31. Gobena, W.T., Duressa, G.F.: Parameter uniform numerical methods for singularly perturbed delay parabolic differential equations with non-local boundary condition. Int. J. Eng. Sci. Technol. 13(2), 57–71 (2021). https://doi.org/10.4314/ijest.v13i2.7

    Article  MATH  Google Scholar 

  32. Hailu, W.S., Duressa, G.F.: Parameter-uniform cubic spline method for singularly perturbed parabolic differential equation with large negative shift and integral boundary condition. Res. Math. 9(1), 2151080 (2022)

    Article  MathSciNet  Google Scholar 

  33. Gobena, W.T., Duressa, G.F.: Exponentially fitted robust scheme for the solution of singularly perturbed delay parabolic differential equations with integral boundary condition. Preprint https://doi.org/10.21203/rs.3.rs-2081265/v1

  34. Gobena, W.T., Duressa, G.F.: Fitted operator average finite difference method for singularly perturbed delay parabolic reaction diffusion problems with non-local boundary conditions. Tamkang J. Math. 1, 1 (2023). https://doi.org/10.5556/j.tkjm.54.2023.4175

    Article  Google Scholar 

  35. Wondimu, G.M., Dinka, T.G., Woldaregay, M.M., Duressa, G.F.: Fitted mesh numerical scheme for singularly perturbed delay reaction diffusion problem with integral boundary condition. Comput. Methods Differ. Equ. (2023). https://doi.org/10.22034/cmde.2023.49239.2054

    Article  MathSciNet  Google Scholar 

  36. Gobena, W.T., Duressa, G.F.: An optimal fitted numerical scheme for solving singularly perturbed parabolic problems with large negative shift and integral boundary condition. Results Control Optim. (2022). https://doi.org/10.1016/j.rico.2022.100172

    Article  Google Scholar 

  37. Roos, H.G.: A second-order scheme for singularly perturbed differential equations with discontinuous source term. J. Numer. Math. 10(4), 275–289 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  38. Selvi, P.A., Ramanujam, N.: An iterative numerical method for singularly perturbed reaction-diffusion equations with negative shift. J. Appl. Comput. Math. 296, 10–23 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  39. Natesan, S., Deb, R.: A robust numerical scheme for singularly perturbed parabolic reaction-diffusion problems. Neural Parallel Sci. Comput. 16, 419 (2008)

    MathSciNet  MATH  Google Scholar 

  40. Rothe, E.: Zweidimensionale parabolische Randwertaufgaben als Grenzfall eindimensionaler Randwertaufgaben. Math. Ann. 102, 650–670 (1930)

    Article  MathSciNet  MATH  Google Scholar 

  41. Ladyzhenskaya, O.A., Solonnikov, V.A., Ural’tseva, N.N.: Linear and Quasi-linear Equations of Parabolic Type, Translations of Mathematical Monographs, vol. 23. American Mathematical Society, Providence (1968)

    Book  MATH  Google Scholar 

  42. Roos, H.G., Stynes, M., Tobiska, L.: Robust Numerical Methods for Singularly Perturbed Differential Equations: Convection Diffusion Reaction and Flow Problems. Springer, Berlin (2008)

    MATH  Google Scholar 

  43. Priyadharshini, R.M., Ramanujam, N., Valanarasu, T.: Hybrid difference schemes for singularly perturbed problem of mixed type with discontinuous source term. J. Appl. Math. Inf. 28, 1035–1054 (2010)

    MathSciNet  MATH  Google Scholar 

  44. Doolan, E.P., Miller, J.J., Schilders, W.H.: Uniform Numerical Methods for Problems with Initial and Boundary Layers. Boole Press, Dublin (1980)

    MATH  Google Scholar 

Download references

Acknowledgements

The authors wish to express their thanks to Jimma University, College of Natural Sciences, for financial support and the authors of the literature for the provided scientific aspects and idea for this work.

Funding

This study did not receive any funding in any form.

Author information

Author notes

  1. Gemechis File Duressa and Lakshmi Sireesha Challa have contributed equally to this work.

Authors and Affiliations

  1. Department of Mathematics, Nekemte College of Teacher Education, 88, Nekemte, Oromia, Ethiopia

    Wakjira Tolassa Gobena & Lakshmi Sireesha Challa

  2. Department of Mathematics, College of Natural Science, Jimma University, 378, Jimma, Oromia, Ethiopia

    Wakjira Tolassa Gobena, Gemechis File Duressa & Lakshmi Sireesha Challa

  3. Department of Mathematics, University College of Science, Saifabad Osmania University, CBIT, Hyderabad, India

    Gemechis File Duressa

Authors
  1. Wakjira Tolassa Gobena
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Gemechis File Duressa
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Lakshmi Sireesha Challa
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Wakjira Tolassa Gobena.

Ethics declarations

Conflict of interest

The authors declare there are no potential conflicts of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Gobena, W.T., Duressa, G.F. & Challa, L.S. Fitted Difference Scheme on a Non-uniform Mesh for Singularly Perturbed Parabolic Reaction–Diffusion with Large Negative Shift and Non-local Boundary Condition. Int. J. Appl. Comput. Math 9, 109 (2023). https://doi.org/10.1007/s40819-023-01553-z

Download citation

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s40819-023-01553-z

Keywords

Mathematics Subject Classification

Search

Navigation