Study on dynamic stability of magneto-electro-thermo-elastic cylindrical nanoshells resting on Winkler–Pasternak elastic foundations using nonlocal strain gradient theory

Abstract

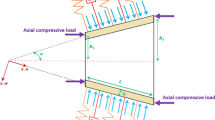
This paper investigated the dynamic stability of magneto-electro-thermo-elastic (METE) cylindrical nanoshells resting on the Winkler–Pasternak elastic foundations in the framework of the nonlocal strain gradient theory and the first-order shear deformation shell theory. Hamilton’s principle is employed to drive the governing differential equations and related boundary conditions. The governing differential equations convert into the form of the Mathieu–Hill equations with the aid of the Navier solution procedure, and then, the boundaries of unstable regions are determined via Bolotin’s method. Results show that the static load factor, electric potential, magnetic potential, temperature change, elastic foundation stiffness have important effects on the dynamic stability of METE nanoshells.

Appendix

$$K_{11} = \left( {\frac{{ - A_{66} n^{2} }}{{L^{2} R^{4} }} - \frac{{A_{11} m^{2} \pi^{2} }}{{L^{4} R^{2} }}} \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(101)

$$K_{12} = \frac{mn\pi }{{L^{3} R^{3} }}\left( {A_{12} + A_{66} } \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(102)

$$K_{13} = \frac{{A_{12} m\pi }}{{L^{3} R^{3} }}\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(103)

$$K_{21} = K_{12} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} K_{13} = K_{31} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} K_{14} = K_{41} = 0,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} K_{15} = K_{16} = K_{17} = 0,$$

(104)

$$K_{22} = \left[ {\frac{{A_{55} k_{S} L^{2} }}{{ - L^{4} R^{4} }}\left( {1 + A_{22} L^{2} n^{2} } \right) - \frac{{A_{66} m^{2} \pi^{2} }}{{L^{4} R^{2} }}} \right]\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(105)

$$K_{23} = - \frac{n}{{L^{2} R^{4} }}\left( {A_{22} + A_{55} k_{S} } \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt}$$

(106)

$$K_{25} = \frac{1}{{L^{2} R^{3} }}A_{55} k_{S} \left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt}$$

(107)

$$K_{24} = 0,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} K_{32} = K_{23} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} K_{52} = K_{25} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} K_{51} = K_{15} ,$$

(108)

$$K_{26} = \frac{1}{{L^{2} R^{3} }}E_{24} n\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(109)

$$K_{27} = \frac{1}{{L^{2} R^{3} }}Q_{24} n\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(110)

$$\begin{aligned} K_{33} & = \frac{{N_{\theta 0} }}{{L^{4} R^{4} }}\left[ {\left( {e_{0} a} \right)^{2} \left( {L^{4} n^{4} + L^{2} m^{2} n^{2} \pi^{2} R^{2} } \right) + L^{4} n^{2} R^{2} } \right] \\ & \quad + \frac{{N_{x0} }}{{L^{4} R^{4} }}\left[ {\left( {e_{0} a} \right)^{2} \left( {L^{2} m^{2} n^{2} \pi^{2} R^{2} + m^{4} \pi^{4} R^{4} } \right) + L^{2} m^{2} \pi^{2} R^{4} } \right] \\ & \quad - \frac{{k_{S} }}{{L^{4} R^{4} }}\left[ {A_{55} \left( {l^{2} L^{4} n^{4} + L^{4} n^{2} R^{2} + l^{2} L^{2} m^{2} n^{2} \pi^{2} R^{2} } \right)} \right. \\ & \quad {\kern 1pt} \left. { + A_{44} \left( {l^{2} L^{2} m^{2} n^{2} \pi^{2} R^{2} + L^{2} m^{2} \pi^{2} R^{4} + l^{2} m^{4} \pi^{4} R^{4} } \right)} \right] \\ & \quad - \frac{{K_{W} }}{{L^{4} R^{4} }}\left[ {L^{4} R^{4} + \left( {e_{0} a} \right)^{2} L^{2} R^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right] \\ & \quad - \frac{{K_{P} }}{{L^{4} R^{4} }}\left[ {L^{4} n^{2} R^{2} + L^{2} m^{2} \pi^{2} R^{4} + \left( {e_{0} a} \right)^{2} L^{2} n^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right. \\ & \quad \left. { + \left( {e_{0} a} \right)^{2} m^{2} \pi^{2} R^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right] - \frac{{A_{22} }}{{L^{4} R^{4} }}\left[ {L^{4} R^{2} + l^{2} \left( {L^{4} n^{2} + L^{2} m^{2} \pi^{2} R^{2} } \right)} \right], \\ \end{aligned}$$

(111)

$$K_{34} = \frac{{ - A_{44} k_{S} }}{{L^{3} R^{2} }}m\pi \left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(112)

$$K_{35} = \frac{{A_{55} }}{{L^{2} R^{3} }}k_{S} n\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(113)

$$K_{41} = K_{14} = 0,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} K_{53} = K_{35} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} K_{43} = K_{34} ,$$

(114)

$$K_{36} = \frac{1}{{L^{4} R^{3} }}\left( {E_{24} L^{2} n^{2} + E_{15} m^{2} \pi^{2} R} \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(115)

$$K_{37} = \frac{1}{{L^{4} R^{3} }}\left( {L^{2} n^{2} Q_{24} + m^{2} \pi^{2} Q_{15} R} \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(116)

$$K_{44} = \left( {\frac{{ - D_{66} n^{2} }}{{L^{2} R^{4} }} - \frac{{A_{44} k_{S} }}{{L^{2} R^{2} }} - \frac{{D_{11} m^{2} \pi^{2} }}{{L^{4} R^{2} }}} \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(117)

$$K_{45} = \frac{mn\pi }{{L^{3} R^{3} }}\left( {D_{12} + D_{66} } \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt}$$

(118)

$$K_{46} = \frac{m\pi }{{L^{3} R^{2} }}\left( {E_{15} + E_{31} } \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(119)

$$K_{47} = \frac{m\pi }{{L^{3} R^{2} }}\left( {Q_{15} + Q_{31} } \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(120)

$$K_{55} = \left( {\frac{{ - D_{22} n^{2} }}{{L^{2} R^{4} }} - \frac{{A_{55} k_{S} }}{{L^{2} R^{2} }} - \frac{{D_{66} m^{2} \pi^{2} }}{{L^{4} R^{2} }}} \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(121)

$$K_{56} = \frac{ - n}{{L^{2} R^{3} }}\left( {E_{32} + E_{24} R} \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(122)

$$K_{57} = \frac{ - n}{{L^{2} R^{3} }}\left( {Q_{32} + Q_{24} R} \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(123)

$$K_{62} = - \frac{{E_{24} n}}{{L^{2} R^{3} }}\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(124)

$$K_{63} = - \frac{1}{{L^{4} R^{3} }}\left( {E_{24} L^{2} n^{2} + E_{15} m^{2} \pi^{2} R} \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(125)

$$K_{64} = \frac{ - m\pi }{{L^{3} R^{2} }}\left( {E_{15} + E_{31} } \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(126)

$$K_{65} = \frac{n}{{L^{2} R^{3} }}\left( {E_{32} + E_{24} R} \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(127)

$$\begin{gathered} K_{66} = \frac{{l^{2} }}{{L^{4} R^{2} }}\left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)\left[ { - m^{2} \pi^{2} X_{11} + L^{2} \left( {n^{4} X_{22} - X_{33} } \right)} \right] \hfill \\ {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} - \frac{1}{{L^{2} }}\left[ {m^{2} \pi^{2} X_{11} + L^{2} \left( {n^{2} X_{22} + X_{33} } \right)} \right], \hfill \\ \end{gathered}$$

(128)

$$K_{67} = \frac{ - 1}{{L^{4} R^{2} }}\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right]\left[ {m^{2} \pi^{2} Y_{11} + L^{2} \left( {n^{2} Y_{22} + Y_{33} } \right)} \right],$$

(129)

$$K_{72} = \frac{ - 1}{{L^{2} R^{3} }}Q_{24} n\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],K_{71} = 0,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} K_{54} = K_{45} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} K_{61} = 0,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt}$$

(130)

$$K_{73} = \frac{ - 1}{{L^{4} R^{3} }}\left( {L^{2} n^{2} Q_{24} + m^{2} \pi^{2} Q_{15} R} \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(131)

$$K_{74} = \frac{ - m\pi }{{L^{3} R^{2} }}\left( {Q_{15} + Q_{31} } \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(132)

$$K_{75} = \frac{n}{{L^{2} R^{4} }}\left( {Q_{32} + Q_{24} R^{2} } \right)\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(133)

$$\begin{gathered} K_{76} = \frac{1}{{L^{4} R^{2} }}\left[ {l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right]\left[ { - m^{2} \pi^{2} Y_{11} + L^{2} \left( {n^{4} Y_{22} - Y_{33} } \right)} \right] \hfill \\ {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} - \frac{1}{{L^{2} }}\left[ {m^{2} \pi^{2} Y_{11} + L^{2} \left( {n^{2} Y_{22} + Y_{33} } \right)} \right], \hfill \\ \end{gathered}$$

(134)

$$K_{77} = \frac{ - 1}{{L^{4} R^{2} }}\left[ {L^{2} R^{2} + l^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right]\left[ {m^{2} \pi^{2} T_{11} + L^{2} \left( {n^{2} T_{22} + T_{33} } \right)} \right],$$

(135)

$$M_{11} = \frac{{I_{0} }}{{L^{2} R^{2} }}\left[ {L^{2} R^{2} + \left( {ea} \right)^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(136)

$$M_{14} = \frac{{I_{1} }}{{L^{2} R^{2} }}\left[ {L^{2} R^{2} + (ea)^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(137)

$$M_{25} = \frac{{I_{1} }}{{L^{2} R^{2} }}\left[ {L^{2} R^{2} + (ea)^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(138)

$$M_{44} = \frac{{I_{2} }}{{L^{2} R^{2} }}\left[ {L^{2} R^{2} + \left( {ea} \right)^{2} \left( {L^{2} n^{2} + m^{2} \pi^{2} R^{2} } \right)} \right],$$

(139)

$$M_{41} = M_{14} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} M_{52} = M_{25} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} M_{22} = M_{11} = M_{33} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} M_{55} = M_{44} ,$$

(140)

$$A_{11} = \int\limits_{ - h/2}^{h/2} {\tilde{c}_{11} } {\text{d}}z,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} A_{12} = \int\limits_{ - h/2}^{h/2} {\tilde{c}_{12} } {\text{d}}z,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} A_{22} = \int\limits_{ - h/2}^{h/2} {\tilde{c}_{22} } {\text{d}}z,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} A_{66} = \int\limits_{ - h/2}^{h/2} {\tilde{c}_{66} } {\text{d}}z,{\kern 1pt}$$

(141)

$$A_{44} = \int\limits_{ - h/2}^{h/2} {\tilde{c}_{44} } {\text{d}}z,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} A_{55} = \int\limits_{ - h/2}^{h/2} {\tilde{c}_{55} } {\text{d}}z,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} D_{11} = \int\limits_{ - h/2}^{h/2} {\tilde{c}_{11} } z^{2} {\text{d}}z,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} D_{12} = \int\limits_{ - h/2}^{h/2} {\tilde{c}_{12} } z^{2} {\text{d}}z,$$

(142)

$$D_{22} = \int\limits_{ - h/2}^{h/2} {\tilde{c}_{22} } z^{2} {\text{d}}z,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} D_{66} = \int\limits_{ - h/2}^{h/2} {\tilde{c}_{66} } z^{2} {\text{d}}z,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} E_{31} = \int\limits_{ - h/2}^{h/2} {\tilde{e}_{31} } z\beta \sin \left( {\beta z} \right){\text{d}}z,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt}$$

(143)

$$E_{32} = \int\limits_{ - h/2}^{h/2} {\tilde{e}_{32} } z\beta \sin \left( {\beta z} \right){\text{d}}z,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} E_{15} = \int\limits_{ - h/2}^{h/2} {\tilde{e}_{15} } \cos \left( {\beta z} \right){\text{d}}z,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} E_{24} = \int\limits_{ - h/2}^{h/2} {\frac{{\tilde{e}_{24} \cos \left( {\beta z} \right)}}{R + z}} {\text{d}}z,{\kern 1pt}$$

(144)

$$X_{11} = \int\limits_{ - h/2}^{h/2} {\tilde{s}_{11} } \left[ {\cos \left( {\beta z} \right)} \right]^{2} {\text{d}}z,{\kern 1pt} X_{22} = \int\limits_{ - h/2}^{h/2} {\tilde{s}_{22} } \left[ {\frac{{\cos \left( {\beta z} \right)}}{R + z}} \right]^{2} {\text{d}}z,{\kern 1pt} {\kern 1pt} Q_{24} = \int\limits_{ - h/2}^{h/2} {\tilde{q}_{24} \frac{{\cos \left( {\beta z} \right)}}{R + z}{\text{d}}z} ,$$

(145)

$$X_{33} = \int\limits_{ - h/2}^{h/2} {\tilde{s}_{33} } \left[ {\beta \sin \left( {\beta z} \right)} \right]^{2} {\text{d}}z,Q_{15} = \int\limits_{ - h/2}^{h/2} {\tilde{q}_{15} \cos \left( {\beta z} \right)} {\text{d}}z,Q_{31} = \int\limits_{ - h/2}^{h/2} {\tilde{q}_{31} \beta z\sin \left( {\beta z} \right)} {\text{d}}z,$$

(146)

$$Q_{32} = \int\limits_{ - h/2}^{h/2} {\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{q}_{32} } \beta z\sin \left( {\beta z} \right){\text{d}}z,Y_{11} = \int\limits_{ - h/2}^{h/2} {\tilde{d}_{11} \cos^{2} \left( {\beta z} \right)} {\text{d}}z,Y_{22} = \int\limits_{ - h/2}^{h/2} {\tilde{d}_{22} \left[ {\frac{{\cos \left( {\beta z} \right)}}{R + z}} \right]}^{2} {\text{d}}z,$$

(147)

$$Y_{33} = \int\limits_{ - h/2}^{h/2} {\tilde{d}_{33} \left[ {\beta \sin \left( {\beta z} \right)} \right]^{2} {\text{d}}z} ,{\kern 1pt} {\kern 1pt} T_{11} = \int\limits_{ - h/2}^{h/2} {\tilde{\mu }_{11} {\text{cos}}^{{2}} \left( {\beta z} \right){\text{d}}z} ,T_{22} = \int\limits_{ - h/2}^{h/2} {\tilde{\mu }_{22} \left[ {\frac{{\cos \left( {\beta z} \right)}}{R + z}} \right]^{2} {\text{d}}z} ,{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt}$$

(148)

$${\kern 1pt} T_{33} = \int\limits_{ - h/2}^{h/2} {\tilde{\mu }_{33} \left[ {\beta {\text{sin}}\left( {\beta z} \right)} \right]^{2} {\text{d}}z} .$$

(149)