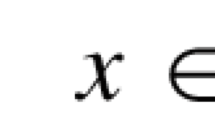SpringerLink
Log in

Existence and Multiplicity Results for Fractional Schrödinger Equation with Critical Growth

  • Published:
The Journal of Geometric Analysis Aims and scope Submit manuscript

Abstract

This paper deals with the following fractional Schrödinger equation with critical growth

$$\begin{aligned} {\left\{ \begin{array}{ll} (-\Delta )^{s}u+V(x)u=|u|^{2_{s}^{*}-2}u, \ \ x\in \Omega , \\ u=0, \ \ x\in {{\mathbb {R}}}^N \setminus \Omega , \end{array}\right. } \end{aligned}$$

where \(\Omega ={{\mathbb {R}}}^N\) or \(\Omega \) is an open bounded domain of \({{\mathbb {R}}}^N\), \(N>2s\) with \(s\in (0,1)\) and V(x) is a sign-changing function. Firstly, using a nonlocal version of the second concentration-compactness principle, we prove the existence of Mountain-Pass solution for the above equation in \({{\mathbb {R}}}^N\). Secondly, we prove the existence of N distinct pairs of nontrivial solutions for the above equation in \({{\mathbb {R}}}^N\) by using a global compactness result and Krasnoselskii’s genus theory, as well as in bounded domains.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Thailand)

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Akgiray, V., Geoffrey Booth, G.: The siable-law model of stock returns. J. Bus. Econ. Stat. 6, 51–57 (1988)

    Google Scholar 

  2. Barletta, G., Candito, P., Marano, S.A., Perera, K.: Multiplicity results for critical p-Laplacian problems. Ann. Mat. Pura Appl. 196, 1431–1440 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  3. Barrios, B., Colorado, E., Servadei, R., Soria, F.: A critical fractional equation with concave-convex power nonlinearities. Ann. Inst. H. Poincaré Anal. Non Linéaire 32, 875–900 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  4. Bonder, J.F., Saintier, N., Silva, A.: The concentration-compactness principle for fractional order Sobolev spaces in unbounded domains and applications to the generalized fractional Brezis-Nirenberg problem. NoDEA 25, Article 52 (2018)

  5. Brasco, L., Squassina, M., Yang, Y.: Global compactness results for nonlocal problems. Discret. Contin. Dyn. Syst. Ser. S. 11, 391–424 (2018)

    MathSciNet  MATH  Google Scholar 

  6. Bucur, C., Valdinoci, E.: Nonlocal Diffusion and Applications. Lecture Notes of the Unione Matematica Italiana, vol. 20. Springer, Cham (2016)

    Google Scholar 

  7. Caffarelli, L., Silvestre, L.: An extension problem related to the fractional Laplacian. Commun. Partial Differ. Equ. 32, 1245–1260 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  8. Caffarelli, L., Silvestre, L.: Regularity theory for fully nonlinear integro-differential equations. Commun. Pure Appl. Math. 62, 597–638 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  9. Caffarelli, L., Silvestre, L.: Regularity results for nonlocal equations by approximation. Arch. Ration. Mech. Anal. 200, 59–88 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  10. Chabrowski, J.: Concentration-compactness principle at infinity and semilinear elliptic equations involving critical and subcritical Sobolev exponents. Calc. Var. Partial Differ. Equ. 4, 493–512 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  11. Chang, X., Wang, Z.-Q.: Ground state of scalar field equations involving a fractional Laplacian with general nonlinearity. Nonlinearity 26, 479–494 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  12. Chen, W., Li, C., Ou, B.: Classifications of solutions for an integral equation. Commun. Pure Appl. Math. 59, 330–343 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  13. Correia, J.N., Figueiredo, G.M.: Existence of positive solution of the equation \((-\Delta )^{s}+a(x)u=|u|^{2_{s}^{*}-2}u\). Calc. Var. Partial Differ. Equ. 58(63), 39 (2019)

    MATH  Google Scholar 

  14. Di Nezza, E., Palatucci, G., Valdinoci, E.: Hitchhiker’s guide to the fractional Sobolev spaces. Bull. Sci. Math. 136, 521–573 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  15. Fall, M.M., Weth, T.: Nonexistence results for a class of fractional elliptic boundary value problems. J. Funct. Anal. 263, 2205–2227 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  16. Felmer, P., Quaas, A., Tan, J.: Positive solutions of the nonlinear Schrödinger equation with the fractional Laplacian. Proc. R. Soc. Edinb. Sect. A 142, 1237–1262 (2012)

    Article  MATH  Google Scholar 

  17. Figueiredo, G.M., Bisci, G.M., Servadei, R.: The effect of the domain topology on the number of solutions of fractional Laplace problems. Calc. Var. Partial Differ. Equ. 57, 103 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  18. Frank, R.L., Lenzmann, E., Silvestre, L.: Uniqueness of radial solutions for the fractional Laplacian. Commun. Pure Appl. Math. 69, 1671–1726 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  19. Ghoussoub, N.: Location, multiplicity and Morse indices of min-max critical points. J. Reine Angew. Math. 417, 27–76 (1991)

    MathSciNet  MATH  Google Scholar 

  20. Giampiero, P., Adriano, P.: Improved Sobolev embeddings, profile decomposition, and concentration-compactness for fractional Sobolev spaces. Calc. Var. Partial Differ. Equ. 50, 799–829 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  21. Guo, L.: Sign-changing solutions for fractional Schrödinger–Poisson system in \({\mathbb{R}}^3\). Appl. Anal. 98, 2085–2104 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  22. Guo, L., Li, Q.: Multiple high energy solutions for fractional Schrödinger equation with critical growth. Calc. Var. Partial Differ. Equ. 61, 26 (2022)

    MATH  Google Scholar 

  23. Guo, Y., Liu, T., Nie, J.: Solutions for fractional Schrödinger equation involving critical exponent via local Pohozaev identities. Adv. Nonlinear Stud. 20, 185–211 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  24. Guo, Y., Li, B., Pistoia, A., Yan, S.: The fractional Brezis–Nirenberg problems on lower dimensions. J. Differ. Equ. 286, 284–331 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  25. Hua, Y., Yu, X.: On the ground state solution for a critical fractional Laplacian equation. Nonlinear Anal. 87, 116–125 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  26. Laskin, N.: Fractional quantum mechanics and Levy path integrals. Phys. Lett. A 268, 298–305 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  27. Laskin, N.: Fractional Schrödinger equation. Phys. Rev. E 66, 056108–056114 (2002)

    Article  MathSciNet  Google Scholar 

  28. Li, Q., Teng, K., Wu, X.: Ground states for fractional Schrödinger equations with critical growth. J. Math. Phys. 59, 12 (2018)

    MATH  Google Scholar 

  29. Lions, P.L.: The concentration-compactness principle in the calculus of variations. The locally compact case. I. Ann. Inst. H. Poincaré Anal. Non Linéaire 1, 109–145 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  30. Lions, P.L.: The concentration-compactness principle in the calculus of variations. The locally compact case. II. Ann. Inst. H. Poincaré Anal. Non Linéaire 1, 223–283 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  31. Lions, P.L.: The concentration-compactness principle in the calculus of variations. The limit case, Part 1. Rev. Mat. Iberoamericana 1, 145–201 (1985)

    Article  MathSciNet  MATH  Google Scholar 

  32. Lions, P.L.: The concentration-compactness principle in the calculus of variations. The limit case, Part 2. Rev. Mat. Iberoamericana 1, 45–121 (1985)

    Article  MathSciNet  MATH  Google Scholar 

  33. Massaccesi, A., Valdinoci, E.: Is a nonlocal diffusion strategy convenient for biological populations in competition? J. Math. Biol. 74(1–2), 113–147 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  34. Miranda, C.: Un’osservazione su un teorema di Brouwer. Boll Unione Mat Ital. 3, 5–7 (1940)

    MathSciNet  MATH  Google Scholar 

  35. MolicaBisci, G., Rădulescu, V.: Ground state solutions of scalar field fractional Schrödinger equations. Calc. Var. Partial Differ. Equ. 54, 2985–3008 (2015)

    Article  MATH  Google Scholar 

  36. Niu, M., Tang, Z.: Least energy solutions for nonlinear Schrödinger equation involving the fractional Laplacian and critical growth. Discret. Contin. Dyn. Syst. 37, 3963–3987 (2017)

    Article  MATH  Google Scholar 

  37. Palatucci, G., Pisante, A.: Improved Sobolev embeddings, profile decomposition and concentration-compactness for fractional Sobolev spaces. Calc. Var. Partial Differ. Equ. 50, 799–829 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  38. Reynolds, A.M., Rhodes, C.J.: The Lévy flight paradigm: random search patterns and mechanisms. Ecology 90(4), 877–887 (2009)

    Article  Google Scholar 

  39. Secchi, S.: Ground state solutions for nonlinear fractional Schröinger equations in \({\mathbb{R}}^N\). J. Math. Phys. 54, 031501 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  40. Servadei, R., Valdinoci, E.: The Brezis-Nirenberg result for the fractional Laplacian. Trans. Am. Math. Soc. 367, 67–102 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  41. Silvestre, L.: Regularity of the obstacle problem for a fractional power of the Laplace operator. Commun. Pure Appl. Math. 60, 67–112 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  42. Yan, S., Yang, J., Yu, X.: Equations involving fractional Laplacian operator: compactness and application. J. Funct. Anal. 269, 47–79 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  43. Zhang, X., Zhang, B., **ang, M.: Ground states for fractional Schrödinger equations involving a critical nonlinearity. Adv. Nonlinear Anal. 5(3), 293–314 (2016)

    MathSciNet  MATH  Google Scholar 

  44. Zhang, X., Zhang, B., Repovs, D.: Existence and symmetry of solutions for critical fractional Schrödinger equations with bounded potentials. Nonlinear Anal. 142, 48–68 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  45. Zou, H.: Existence and non-existence for Schrödinger equations involving critical Sobolev exponents. J. Korean Math. Soc. 47(3), 547–572 (2010)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors are grateful to the referee’s thoughtful reading of details of the paper and valuable comments. Lun Guo was supported by the National Natural Science Foundation of China (No.11901222) and the China Postdoctoral Science Foundation (No.2021M690039).

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, South–Central University for Nationalities, Wuhan, 430074, China

    Lun Guo

  2. School of Mathematical Sciences, Fudan University, Shanghai, 200433, China

    Lun Guo

  3. College of Science & Hubei Province Key Laboratory of Systems Science in Metallurgical Process, Wuhan University of Science and Technology, Wuhan, 430065, China

    Qi Li

Authors
  1. Lun Guo
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Qi Li
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Qi Li.

Ethics declarations

Conflict of interest

The authors don’t have any conflicts of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Guo, L., Li, Q. Existence and Multiplicity Results for Fractional Schrödinger Equation with Critical Growth. J Geom Anal 32, 277 (2022). https://doi.org/10.1007/s12220-022-01011-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s12220-022-01011-0

Keywords

Mathematics Subject Classification

Search

Navigation