SpringerLink
Log in

Solving higher-order nonlinear Volterra integro-differential equations using two discretization methods

  • Original Research
  • Published:
Journal of Applied Mathematics and Computing Aims and scope Submit manuscript

Abstract

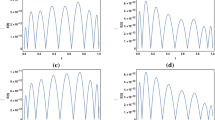
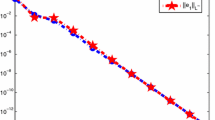
The primary objective of this article is to devis an effective method for solving a high-degree nonlinear Volterra integro-differential equation. First, we conduct a comprehensive literature review to underscore the significance of such mathematical equations in scientific research. Subsequently, we establish sufficient conditions to demonstrate the existence and uniqueness of the exact solution. Then, we employ two different discretization methods, namely Nyström and collocation. Then, we obtain two approximate systems, each of which yields a solution converging to the exact solution. The convergence of these systems is rigorously demonstrated through two theorems. Finally, we present numerical tests to evaluate the effectiveness and simplicity of the proposed numerical processes.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Data availibility

Data sharing is not applicable to this article as no datasets were generated or analyzed during the current study.

References

  1. Alam, M., Rajni, K. P.: Exponentially Fitted Finite Difference Approximation for Singularly Perturbed Fredholm Integro-Differential Equation. ar**v preprint (2024). ar**v:2401.16379

  2. Ali, L.H., Sulaiman, J., Saudi, A., Xu, M.M.: Numerical Solution of Nonlinear Fredholm Integral Equations Using Half-Sweep Newton-PKSOR Iteration. Math. Stat. 10(4), 868–874 (2022). https://doi.org/10.13189/ms.2022.100418

    Article  Google Scholar 

  3. Atkinson, K., Han, W.: Theoretical Numerical Analysis: A Functional Analysis Framework. Springer-Verlag, New York (2001)

    Book  Google Scholar 

  4. Bassi, H.: Learning nonlinear integral operators via recurrent neural networks and its application in solving integro-differential equations. Mach. Learn. Appl. 15, 100524 (2024). https://doi.org/10.1016/j.mlwa.2023.100524

    Article  Google Scholar 

  5. Bounaya, M.C., Lemita, S., Ghiat, M., Aissaoui, M.Z.: On a nonlinear integro-differential equation of Fredholm type. Int. J. Comput. Math. 13(2), 194–205 (2021). https://doi.org/10.1504/IJCSM.2021.114188

    Article  MathSciNet  Google Scholar 

  6. Briani, M., Chioma, C.L., Natalini, R.: Convergence of numerical schemes for viscosity solutions to integro-differential degenerate parabolic problems arising in financial theory. Num. Math. 98(4), 607–646 (2004). https://doi.org/10.1007/s00211-004-0530-0

    Article  MathSciNet  Google Scholar 

  7. Bynum, F. W., Janet, B., Roy, P.: Dictionary of the History of Science. Princeton University Press (2014)

  8. Faria, J.R.: An integro-differential approach to terrorism dynamics. Def. Peace. Econ. 22(6), 595–605 (2011). https://doi.org/10.1080/10242694.2011.627768

    Article  Google Scholar 

  9. Fazeli, S., Hojjati, G.: A class of two-step collocation methods for Volterra integro-differential equations. App. Num. Math. 181, 59–75 (2022). https://doi.org/10.1016/j.apnum.2022.05.017

    Article  MathSciNet  Google Scholar 

  10. Ghiat, M., Guebbai, H.: Analytical and numerical study for an integro-differential nonlinear Volterra equation with weakly singular kernel. Comp. App. Math. 37(4), 4661–4674 (2019). https://doi.org/10.1007/s40314-018-0597-3

    Article  MathSciNet  Google Scholar 

  11. Ghiat, M., Guebbai, H., Kurulay, M., Segni, S.: On the weakly singular integro-differential nonlinear Volterra equation depending in acceleration term. Comp. App. Math. 39(3), 1–13 (2020). https://doi.org/10.1007/s40314-020-01235-2

    Article  MathSciNet  Google Scholar 

  12. Ghiat, M., Tair, B., Guebbai, H., Segni, S.: Block-by-block method for solving nonlinear Volterra integral equation of the first kind. Comp. App. Math. 42(1), 67 (2023). https://doi.org/10.1007/s40314-023-02212-1

    Article  Google Scholar 

  13. Guebbai, H., Aissaoui, M.Z., Debbar, I., Khalla, B.: Analytical and numerical study for an integro-differential nonlinear Volterra equation. App. Math. Comp. 229, 367–373 (2014). https://doi.org/10.1016/j.amc.2013.12.046

    Article  MathSciNet  Google Scholar 

  14. Hernandez, E., Rolnik, V., Ferrari, T.M.: Existence and Uniqueness of Solutions for Abstract Integro-differential Equations with State-Dependent Delay and Applications. Mediterr. J. Math. 19(3), 1–13 (2022). https://doi.org/10.1007/s00009-022-02009-2

    Article  MathSciNet  Google Scholar 

  15. Hossain, M.E.: Numerical investigation of memory-based diffusivity equation: the integro-differential equation. Arab. J. Sci. Eng. 41(7), 2715–2729 (2016). https://doi.org/10.1007/s13369-016-2170-y

    Article  MathSciNet  Google Scholar 

  16. Kanaun, S.: Efficient numerical solution of the volume integro-differential equation for time-varying temperature fields in heterogeneous media. Int. J. Eng. Sci. 173, 103649 (2022). https://doi.org/10.1016/j.ijengsci.2022.103649

    Article  MathSciNet  Google Scholar 

  17. Kerner, H.E.: A statistical mechanics of interacting biological species. Bull. Math. Biol. 19, 121–146 (1957)

    MathSciNet  Google Scholar 

  18. Kumar, P., Suat Erturk, V.: A case study of Covid-19 epidemic in India via new generalised Caputo type fractional derivatives. Math. Meth. App. Sci. 46(7), 7930–7943 (2021). https://doi.org/10.1002/mma.7284

    Article  MathSciNet  Google Scholar 

  19. Laib, H., Bellour, A., Boulmerka, A.: Taylor collocation method for a system of nonlinear Volterra delay integro-differential equations with application to COVID-19 epidemic. Int. J. Com. Math. 99(4), 852–876 (2022). https://doi.org/10.1080/00207160.2021.1938012

    Article  MathSciNet  Google Scholar 

  20. Lakshmikantham, V.: Theory of integro-differential equations. CRC press (1995)

  21. Linz, P.: Analytical and numerical methods for Volterra equations. Society for Industrial and Applied Mathematics (1985)

  22. Lotka, A.: On an integral equation in population analysis. Ann. Math. Stat. 10(2), 144–161 (1939)

    Article  Google Scholar 

  23. Minfu, H.: Linear integro-differential equations with a boundary condition. Trans. Am. Math. Soc. 19(4), 363–407 (1918)

    Article  MathSciNet  Google Scholar 

  24. Mohammad, M., Trounev, A., Cattani, C.: The dynamics of COVID-19 in the UAE based on fractional derivative modeling using Riesz wavelets simulation. Adv. Differ. Equ. 1, 1–14 (2021). https://doi.org/10.1186/s13662-021-03262-7

    Article  MathSciNet  Google Scholar 

  25. Omojola, D.A.F.: Integro-differential equations for light nuclei. J. Phys. A 2(6), 666 (1969). https://doi.org/10.1088/0305-4470/2/6/007

    Article  Google Scholar 

  26. Poincaré, H.: Sur les équations aux dérivées partielles de la physique mathématique. Am. J. Math. 12(3), 211–294 (1890)

    Article  Google Scholar 

  27. Poincaré, H.: Sur le probléme des trois corps et les équations de la dynamique. Acta. Math. 13(1), A3–A270 (1890)

    MathSciNet  Google Scholar 

  28. Pouchol, C., Clairambault, C.J., Alexander, L., Emmanuel, T.: Asymptotic analysis and optimal control of an integro-differential system modelling healthy and cancer cells exposed to chemotherapy. J. de Math. Pures et Appl. 116, 268–308 (2018). https://doi.org/10.1016/j.matpur.2017.10.007

    Article  MathSciNet  Google Scholar 

  29. Salah, S., Guebbai, H., Lemita, S., Aissaoui, M.Z.: Solution of an integro-differential nonlinear equation of Volterra arising of earthquake model. Bol. Soc. Parna. Mate. 40, 1–14 (2019). https://doi.org/10.5269/bspm.48018

    Article  Google Scholar 

  30. Seaton, M. J.: Computer programs for the calculation of electron-atom collision cross sections. II. A numerical method for solving the coupled integro-differential equations. J. Phys. B At. Mol. Opt. Phys. 7(14) , 68-87 (1974).https://doi.org/10.1088/0022-3700/5/12/013

  31. Segni, S., Ghiat, M., Guebbai, H.: New approximation method for Volterra nonlinear integro-differential equation. Asian. Eur. J. Math 2(1), 1950016 (2019). https://doi.org/10.1142/S1793557119500165

    Article  MathSciNet  Google Scholar 

  32. Swan, P.: The relation between zero-energy scattering phase-shifts, the Pauli exclusion principle and the number of composite bound states. Proc. R. soc. Lond. Ser. Math. Phys. 228, 10–33 (1955). https://doi.org/10.1098/rspa.1955.0031

    Article  Google Scholar 

  33. Tair, B., Guebbai, H., Segni, S., Ghiat, M.: Solving linear Fredholm integro-differential equation by Nyström method. J. App. Math. Comp. Mech. 20(3), 53–64 (2021). https://doi.org/10.17512/jamcm.2021.3.05

    Article  Google Scholar 

  34. Tair, B., Guebbai, H., Segni, S., Ghiat, M.: An approximation solution of linear Fredholm integro-differential equation using Collocation and Kantorovich methods. J. App. Math. Comp. 68(3), 1–21 (2021). https://doi.org/10.1007/s12190-021-01654-2

    Article  MathSciNet  Google Scholar 

  35. Tair, B., Segni, S., Guebbai, H., Ghait, M.: Two numerical treatments for solving the linear integro-differential Fredholm equation with a weakly singular kernel. Num. Meth. Progr. 23, 117–136 (2022). https://doi.org/10.26089/NumMet.v23r208

    Article  Google Scholar 

  36. Torkaman, S., Heydari, M., Barid, G.L.: Piecewise barycentric interpolating functions for the numerical solution of Volterra integro-differential equations. Math. Meth. App. Sci. 45(10), 6030–6061 (2022). https://doi.org/10.1002/mma.8154

    Article  MathSciNet  Google Scholar 

  37. Volterra, V.: Variations and fluctuations of the number of individuals in animal species living together. ICES J. Mar. Sci. 3(1), 3–51 (1928). https://doi.org/10.1093/icesjms/3.1.3

    Article  Google Scholar 

  38. Volterra, V.: Theory of functionals and of integral and integro-differential equations. Dover Publications, New York (1959)

    Google Scholar 

  39. Wazwaz, A. M.: Linear and Nonlinear Integral Equations Methods and Applications , Heidelberg Dordrecht (2011)

  40. Yuan, L., Ni, Y.Q., Deng, X.Y., Hao, S.: A-PINN: auxiliary physics informed neural networks for forward and inverse problems of nonlinear integro-differential equations. J. Comp. Phys. 462, 111260 (2022)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

We would like to thank you for following the instructions above very closely in advance. It will definitely save us lot of time and expedite the process of your paper’s publication.

Author information

Authors and Affiliations

  1. Department of Mathematics, 08 Mail 1945 University, Mathematics and Modeling Laboratory B.P. 401, Guelma, Algeria

    Boutheina Tair

  2. National higher school of biotechnology, Université Salah Boubnider, Constantine, 25000, Algeria

    Boutheina Tair

  3. Department of Mathematics, Mohamed Khider University, Biskra, Algeria

    Walid Slimani

Authors
  1. Boutheina Tair
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Walid Slimani
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Boutheina Tair.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Artificial intelligence

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Tair, B., Slimani, W. Solving higher-order nonlinear Volterra integro-differential equations using two discretization methods. J. Appl. Math. Comput. (2024). https://doi.org/10.1007/s12190-024-02075-7

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s12190-024-02075-7

Keywords

Mathematics Subject Classification

Search

Navigation