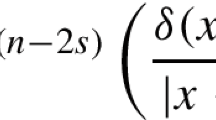Abstract
Our aim in this paper is to analyze the existence and regularity of solutions for the following nonlocal parabolic problem involving the fractional Laplacian with singular nonlinearity
where \(\Omega \) is a bounded domain of class \({\mathcal {C}}^{0,1}\) in \({\mathbb {R}}^{N}\), \(N>2s\) with \(s\in (0,1)\), \(\gamma >0\), \(0<T<+\infty \), \(f\ge 0\), \(f\in L^{m}(\Omega _T)\), \(m\ge 1\), is a non-negative function on \(\Omega _T\) and \(u_0\) is a non-negative function defined on \(\Omega \) belonging to some Lebesgue spaces. The existence and regularity of the very weak and weak solutions are obtained under different assumptions on the summability of the data and on \(\gamma \). One of the difficulties arising in the problem is the proof of the strict positivity of the weak solutions inside the parabolic cylinders. This proof involves the smallest eigenvalue of the fractional Laplacian and the weak comparison principle. We also deal with the case of singular Radon measures data and the asymptotic behavior of the very weak solutions.
Similar content being viewed by others
References
Abdellaoui, B., Attar, A., Bentifour, R., Peral, I.: On fractional \(p\)-Laplacian parabolic problem with general data. Ann. Mat. Pura Appl. (4) 197(2), 329–356 (2018)
Abdellaoui, B., Biroud, K., Laamri, E.-H.: Existence and nonexistence of positive solutions to a fractional parabolic problem with singular weight at the boundary. J. Evol. Equ. 21, 1227–1261 (2021)
Abdellaoui, B., Boucherif, A., Touaoula, T.M.: Fractional parabolic problems with a nonlocal initial condition. Moroccan J. Pure Appl. Anal. 3(1), 116–132 (2017)
Abdellaoui, B., Dall’Aglio, A., Peral, I.: Regularity and nonuniqueness results for parabolic problems arising in some physical models having natural growth in the gradient. J. Math. Pures Appl. (9) 90(3), 242–269 (2008)
Abdellaoui, B., Medina, M., Peral, I., Primo, A.: The effect of the Hardy potential in some Calderón–Zygmund properties for the fractional Laplacian. J. Differ. Equ. 260(11), 8160–8206 (2016)
Abdellaoui, B., Medina, M., Peral, I., Primo, A.: Optimal results for the fractional heat equation involving the Hardy potential. Nonlinear Anal. 140, 166–207 (2016)
Abdellaoui, B., Peral, I., Primo, A., Soria, F.: General KPZ equation with nonlocal diffusion: existence and regularity. Submitted. https://arxiv.org/pdf/1904.04593.pdf
Adimurthi, A., Giacomoni, J., Santra, S.: Positive solutions to a fractional equation with singular nonlinearity. J. Differ. Equ. 265(4), 1191–1226 (2018)
Applebaum, D.: Lévy Processes and Stochastic Calculus, vol. 116. Cambridge Univ. Press, Cambridge, Second edition. Cambridge Studies in Advanced Mathematics (2009)
Badra, M., Bal, K., Giacomoni, J.: A singular parabolic equation: existence, stabilization. J. Differ. Equ. 252(9), 5042–5075 (2012)
Barrios, B., De Bonis, I., Medina, M., Peral, I.: Semilinear problems for the fractional laplacian with a singular nonlinearity. Open Math. 13, 390–407 (2015)
Barrios, B., Medina, M., Peral, I.: Some remarks on the solvability of non-local elliptic problems with the Hardy potential. Commun. Contemp. Math. 16(4), 1350046 (2014)
Barrios, B., Medina, M.: Strong maximum principles for fractional elliptic and parabolic problems with mixed boundary conditions. Proc. R. Soc. Edinb. Sect. A: Math. 150(1), 475–495 (2020)
Bayrami-Aminlouee, M., Hesaaraki, M., Hamdani, M.K., Nguyen, T.C.: Nonlocal Lazer McKenna type problem perturbed by the Hardy potential and its parabolic equivalence. Bound. Value Probl. Paper No. 68, 42 pp (2021)
Biroud, K., Laamri, E.-H.: Fractional \(s(.)\) problem with singular potential and general data. Submitted
Bisci, G.M., Radulescu, V.D., Servadei, R.: Variational methods for nonlocal fractional problems, volume 162 of Encyclopedia of Mathematics and its Applications. Cambridge University Press, Cambridge, 2016. With a foreword by Jean Mawhin
Boccardo, L., Orsina, L.: Semilinear elliptic equations with singular nonlinearities. Calc. Var. Partial. Differ. Equ. 37, 363–380 (2009)
Boccardo, L., Orsina, L., Porzio, M.M.: Existence results for quasilinear elliptic and parabolic problems with quadratic gradient terms and sources. Adv. Calc. Var. 4(4), 397–419 (2011)
Bogdan, K., Jakubowski, T.: Estimates of the green function for the fractional laplacian perturbed by gradient. Potential Anal. 36, 455–481 (2012)
Bougherara, B., Giacomoni, J.: Existence of mild solutions for a singular parabolic equation and stabilization. Adv. Nonlinear Anal. 4(2), 123–134 (2015)
Bucur, C., Valdinoci, E.: Nonlocal Diffusion and Applications. Lecture Notes of the Unione Matematica Italiana, Volume 20, Springer, [Cham], Unione Matematica Italiana, Bologna (2016)
Caffarelli, L., Silvestre, L.: Regularity theory for fully nonlinear integro-differential equations. Commun. Pure Appl. Math. 62(5), 597–638 (2009)
Canino, A., Montoro, L., Sciunzi, B., Squassina, M.: Nonlocal problems with singular nonlinearity. Bull. Sci. Math. 141(3), 223–250 (2017)
Chen, H., Veron, L.: Semilinear fractional elliptic equations involving measures. J. Differ. Equ. 257, 1457–1486 (2014)
Chen, W., Li, Y., Ma, P.: The Fractional Laplacian. World Scientific, New Jersey (2020)
Danielli, D., Salsa, S.: Obstacle problems involving the fractional Laplacian. In: Recent Developments in Nonlocal Theory, pages De Gruyter, Berlin, 81–164 (2018)
Droniou, J., Porretta, A., Prignet, A.: Parabolic capacity and soft measures for nonlinear equations. Potential Anal. 19(2), 99–161 (2003)
de Bonis, I., De Cave, L.M.: Degenerate parabolic equations with singular lower order terms. Differ. Integral Equ. 27(9–10), 949–976 (2014)
De Bonis, I., Giachetti, D.: Singular parabolic problems with possibly changing sign data. Discrete Contin. Dyn. Syst. Ser. B. 19(7), 2047–2064 (2014)
Demengel, F., Demengel, G.: Functional spaces for the theory of elliptic partial differential equations, Universitext. Springer, London; EDP Sciences, Les Ulis, Translated from the 2007 French original by Reinie Erné (2012)
Di Nezza, E., Palatucci, G., Valdinoci, E.: Hitchhiker’s guide to the fractional Sobolev spaces. Bull. Sci. Math. 136(5), 521–573 (2012)
Dipierro, S., Figalli, A., Valdinoci, E.: Strongly nonlocal dislocation dynamics in crystals. Commun. Partial Differ. Equ. 39(12), 2351–2387 (2014)
Giacomoni, J., Mukherjee, T., Sreenadh, K.: Existence and stabilization results for a singular parabolic equation involving the fractional Laplacian. Discrete Contin. Dyn. Syst. Ser. S. 12(2), 311–337 (2019)
Kilbas, A.A., Marichev, O.I., Samko, S.G.: Fractional Integrals and Derivatives. Theory and Applications. Gordon and Breach, Amsterdam (1993)
Kilbas, A.A., Srivastava, H.M., Trujillo, J.J.: Theory and Applications of Fractional Differential Equations. Elsevier, Amsterdam (2006)
Klimsiak, T., Rozkosz, A.: Smooth measures and capacities associated with nonlocal parabolic operators. J. Evol. Equ. 19, 997–1040 (2019)
A. Kufner, John, O., Fučík, S.: Function Spaces, Noordhoff International Publishing, Leyden; Academia, Prague: Monographs and Textbooks on Mechanics of Solids and Fluids. Analysis, Mechanics (1977)
Jiang, R., **ao, J., Yang, D., Zhai, Z.: Regularity and capacity for the fractional dissipative operator. J. Differ. Equ. 259, 3495–3519 (2015)
Leonori, T., Peral, I., Primo, A., Soria, F.: Basic estimates for solutions of a class of nonlocal elliptic and parabolic equations. Discrete Contin. Dyn. Syst. 35(12), 6031–6068 (2015)
Oliva, F., Petitta, F.: A nonlinear parabolic problem with singular terms and nonregular data. Nonlinear Anal. 194, 111472 (2020)
Pierre, M.: Parabolic capacity and Sobolev spaces. SIAM J. Math. Anal. 14(3), 522–533 (1983)
Ponce, A.C.: Elliptic PDEs, Measures and Capacities, From the Poisson Equations to Nonlinear Thomas–Fermi Problems. EMS Tracts in Mathematics, Vol. 23. European Mathematical Society (EMS), Zürich (2016)
Pozrikidis, C.: The Fractional Laplacian. CRC Press, Boca Raton (2016)
Servadei, R.: The Yamabe equation in a non-local setting. Adv. Nonlinear Anal. 2(3), 235–270 (2013)
Servadei, R., Valdinoci, E.: Variational methods for non-local operators of elliptic type. Discrete Contin. Dyn. Syst. 33(5), 2105–2137 (2013)
Silvestre, L.: Regularity of the obstacle problem for a fractional power of the Laplace operator. Commun. Pure Appl. Math. 60(1), 67–112 (2007)
Sire, Y., Valdinoci, E.: Fractional Laplacian phase transitions and boundary reactions: a geometric inequality and a symmetry result. J. Funct. Anal. 256(6), 1842–1864 (2009)
Teng, K., Zhang, C., Zhou, S.: Renormalized and entropy solutions for the fractional \(p\)-Laplacian evolution equations. J. Evol. Equ. 19(2), 559–584 (2019)
Vázquez, J.L.: The Mathematical Theories of Diffusion: Nonlinear and Fractional Diffusion. In: Nonlocal and Nonlinear Diffusions and Interactions: New Methods and Directions, Lecture Notes in Math., Volume 2186, Springer, Cham, pp. 205–278 (2017)
Youssfi, A., Ould Mohamed Mahmoud, G.: On singular equations involving fractional laplacian. Acta Math. Sci. 40B(5), 1289–1315 (2020)
Youssfi, A., Ould Mohamed Mahmoud, G.: Nonlocal semilinear elliptic problems with singular nonlinearity. Calc. Var. Partial Differ. Equ. 60(4), 153 (2021)
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
The authors declare that there is no conflict of interests regarding the publication of this paper.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Abdellaoui, B., Ould Mohamed Mahmoud, G. & Youssfi, A. Fractional heat equation with singular nonlinearity. J. Pseudo-Differ. Oper. Appl. 13, 50 (2022). https://doi.org/10.1007/s11868-022-00484-5
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s11868-022-00484-5
Keywords
- Fractional Laplacian
- Parabolic equations
- Positivity of solutions
- Singular nonlinearity
- Fractional parabolic capacity
- Singular measures