SpringerLink
Log in

Some properties of Melnikov functions near a cuspidal loop

  • Articles
  • Published:
Science China Mathematics Aims and scope Submit manuscript

Abstract

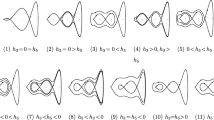
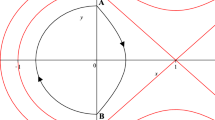
In this paper, we consider the first-order Melnikov functions and limit cycle bifurcations of a near-Hamiltonian system near a cuspidal loop. By establishing relations between the coefficients in the expansions of the two Melnikov functions, we give a general method to obtain the number of limit cycles near the cuspidal loop. As an application, we consider a kind of Liénard systems and obtain a new estimation on the lower bound of the maximum number of limit cycles.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Atabaigi A, Zangeneh H R Z, Kazemi R. Limit cycle bifurcation by perturbing a cuspidal loop of order 2 in a Hamiltonian system. Nonlinear Anal, 2012, 75: 1945–1958

    Article  MathSciNet  Google Scholar 

  2. Blows T R, Lloyd N G. The number of small-amplitude limit cycles of Liénard equations. Math Proc Cambridge Philos Soc, 1984, 95: 359–366

    Article  MathSciNet  Google Scholar 

  3. Christopher C, Lynch S. Small-amplitude limit cycle bifurcations for Liénard systems with quadratic or cubic dam** or restoring forces. Nonlinearity, 1999, 12: 1099–1112

    Article  MathSciNet  Google Scholar 

  4. Han M A. Liapunov constants and Hopf cyclicity of Liénard systems. Ann Differential Equations, 1999, 15: 113–126

    MathSciNet  Google Scholar 

  5. Han M A. On Hopf cyclicity of planar systems. J Math Anal Appl, 2000, 245: 404–422

    Article  MathSciNet  Google Scholar 

  6. Han M A. Bifurcation Theory of Limit Cycles. Bei**g: Science Press, 2013

    Google Scholar 

  7. Han M A, Shu C G, Yang J M, et al. Polynomial Hamiltonian systems with a nilpotent critical point. Adv Space Res, 2010, 46: 521–525

    Article  Google Scholar 

  8. Han M A, Yang J M, Li J B. General study on limit cycle bifurcation near a double homoclinic loop. J Differential Equations, 2023, 347: 1–23

    Article  MathSciNet  Google Scholar 

  9. Han M A, Yang J M, Tarţa A A, et al. Limit cycles near homoclinic and heteroclinic loops. J Dynam Differential Equations, 2008, 20: 923–944

    Article  MathSciNet  Google Scholar 

  10. Han M A, Yu P. Normal Forms, Melnikov Functions and Bifurcations of Limit Cycles. London: Springer, 2012

    Book  Google Scholar 

  11. Han M A, Zang H, Yang J M. Limit cycle bifurcations by perturbing a cuspidal loop in a Hamiltonian system. J Differential Equations, 2009, 246: 129–163

    Article  MathSciNet  Google Scholar 

  12. Hou W W, Liu S S. Melnikov functions for a class of piecewise Hamiltonian systems. J Nonlinear Model Anal, 2023, 5: 123–145

    Google Scholar 

  13. Ilyashenko Y. Centennial history of Hilbert’s 16th problem. Bull Amer Math Soc (NS), 2002, 39: 301–354

    Article  MathSciNet  Google Scholar 

  14. Kazemi R, Zangeneh H R Z, Atabaigi A. On the number of limit cycles in small perturbations of a class of hyper-elliptic Hamiltonian systems. Nonlinear Anal, 2012, 75: 574–587

    Article  MathSciNet  Google Scholar 

  15. Li F, Liu Y R, Liu Y Y, et al. Bi-center problem and bifurcation of limit cycles from nilpotent singular points in Z2-equivariant cubic vector fields. J Differential Equations, 2018, 265: 4965–4992

    Article  MathSciNet  Google Scholar 

  16. Li J, Zhang T H, Han M A. Bifurcation of limit cycles from a heteroclinic loop with two cusps. Chaos Solitons Fractals, 2014, 62–63: 44–54

    Article  MathSciNet  Google Scholar 

  17. Li J B. Hilbert’s 16th problem and bifurcations of planar polynomial vector fields. Internat J Bifur Chaos Appl Sci Engrg, 2003, 13: 47–106

    Article  MathSciNet  Google Scholar 

  18. Li L L, Yang J M. On the number of limit cycles for a quintic Liénard system under polynomial perturbations. J Appl Anal Comput, 2019, 9: 2464–2481

    MathSciNet  Google Scholar 

  19. Liu P, Han M A. Limit cycle bifurcations near a cuspidal loop. Symmetry, 2020, 12: 1425

    Article  Google Scholar 

  20. Llibre J, Mereu A C, Teixeira M A. Limit cycles of the generalized polynomial Liénard differential equations. Math Proc Cambridge Philos Soc, 2010, 148: 363–383

    Article  MathSciNet  Google Scholar 

  21. Tian Y, Han M A. Hopf and homoclinic bifurcations for near-Hamiltonian systems. J Differential Equations, 2017, 262: 3214–3234

    Article  MathSciNet  Google Scholar 

  22. Wei L J, Zhang X. Limit cycles bifurcating from periodic orbits near a centre and a homoclinic loop with a nilpotent singularity of Hamiltonian systems. Nonlinearity, 2020, 33: 2723–2754

    Article  MathSciNet  Google Scholar 

  23. **ong Y Q. Limit cycle bifurcations by perturbing a Hamiltonian system with a cuspidal loop of order m. Internat J Bifur Chaos Appl Sci Engrg, 2015, 25: 1550083

    Article  MathSciNet  Google Scholar 

  24. **ong Y Q, Han M A. New lower bounds for the Hilbert number of polynomial systems of Liénard type. J Differential Equations, 2014, 257: 2565–2590

    Article  MathSciNet  Google Scholar 

  25. Xu W J, Li C P. Limit cycles of some polynomial Liénard systems. J Math Anal Appl, 2012, 389: 367–378

    Article  MathSciNet  Google Scholar 

  26. Yang J M, Han M A. Limit cycles near a double homoclinic loop. Ann Differential Equations, 2007, 23: 536–545

    MathSciNet  Google Scholar 

  27. Yang J M, Yu P, Han M A. Limit cycle bifurcations near a double homoclinic loop with a nilpotent saddle of order m. J Differential Equations, 2019, 266: 455–492

    Article  MathSciNet  Google Scholar 

  28. Yu P, Li F. Bifurcation of limit cycles in a cubic-order planar system around a nilpotent critical point. J Math Anal Appl, 2017, 453: 645–667

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

The first author was supported by National Natural Science Foundation of China (Grant No. 11971145). The second author was supported by National Natural Science Foundation of China (Grant No. 11931016) and the National Key R&D Program of China (Grant No. 2022YFA1005900). The authors thank the referees for their helpful suggestions, which have greatly helped improve the presentation of this paper.

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Hebei Normal University, Shijiazhuang, 050024, China

    Junmin Yang

  2. Department of Mathematics, Zhejiang Normal University, **hua, 321004, China

    Maoan Han

Authors
  1. Junmin Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Maoan Han
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Maoan Han.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yang, J., Han, M. Some properties of Melnikov functions near a cuspidal loop. Sci. China Math. 67, 767–786 (2024). https://doi.org/10.1007/s11425-022-2124-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11425-022-2124-7

Keywords

MSC(2020)

Search

Navigation