Abstract
In this paper, the analytical derivatives of flexible multibody dynamics with the floating frame of reference formulation are derived in a new way using the invariants and their sensitivities. This enables the decoupling of the sensitivity analysis of flexible multibody dynamics from the finite-element solver and guarantees high accuracy and efficiency of the sensitivity computations. The invariants are shown with both consistent and lumped mass approaches. The latter allows generality towards the formulation of a finite-element type, including beams, shells, and solids. The expressions are fully derived with lumped masses, showing for the first time the compensation term of inertia due to the non-consideration of the mass distribution with this approach. It is then shown that the expressions of the system parameters in the lumped case with the newly introduced inertia compensation term correspond to the general case, and, therefore, the derived approach and equations are of general nature. Crucial for the decoupling of the sensitivity analysis are the analytical derivatives of the system parameters that contain the derivatives of the invariants and whose analytical expressions are derived and provided here for the first time. The partial derivatives arise in the sensitivity analysis with both the direct differentiation method and the adjoint variable method, and the former is shown here. In addition, the partial derivatives arise in the Jacobian matrix of the nonlinear solver for the transient solution of flexible multibody systems.


Similar content being viewed by others
Abbreviations
-
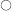 :
: -
zeroth-order tensor or scalar
-
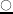 :
: -
first-order tensor or vector
-
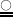 :
: -
second-order tensor or matrix
-
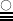 :
: -
third-order tensor or 3D matrix
-
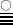 :
: -
fourth-order tensor or 4D matrix
-
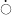 :
: -
first time derivative
-
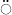 :
: -
second time derivative
-
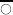 :
: -
expressed in floating coordinates
-
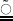 :
: -
skew symmetric matrix
-
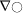 :
: -
total derivative of
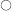 w.r.t. \(\underline{\mathsf{x}}\)
w.r.t. \(\underline{\mathsf{x}}\) -
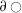 :
: -
partial derivative of
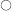 w.r.t. \(\underline{\mathsf{x}}\)
w.r.t. \(\underline{\mathsf{x}}\) -
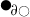 :
: -
partial derivative of
 w.r.t.
w.r.t. 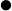
-
 :
: -
Jacobian of
 w.r.t.
w.r.t. 
- \(\underline{\underline{d}}\) :
-
dam** matrix
- \(\underline{e}\) :
-
unit vector
- \(\underline{\underline{e}}\) :
-
unit matrix (identity matrix)
- \(\underline{\underline{k}}\) :
-
stiffness matrix
- \(m\) :
-
mass
- \(\underline{\underline{m}}\) :
-
mass matrix
- \(\underline{q}\) :
-
generalized position vector
- \(\underline{\dot{q}}\) :
-
generalized velocity vector
- \(\underline{\ddot{q}}\) :
-
generalized acceleration vector
- \(\underline{r}\) :
-
position vector to inertial frame expressed in intertial coordinates
- \(t\) :
-
time
- \(\underline{\overline{u}}\) :
-
position vector to floating frame expressed in floating coordinates
- \(\underline{u}\) :
-
position vector to floating frame expressed in inertial coordinates
- \(\underline{\mathsf{x}}\) :
-
vector of design variables
- \(\underline{\underline{A}}\) :
-
rotation matrix
- \(\underline{\underline{B}}\) :
-
Boolean matrix
-
 :
: -
function of
- \(\underline{\underline{G}}\) :
-
angular velocity matrix that relates \(\underline{\omega}\) and \(\underline{\dot{\theta}}\)
- \(\underline{\underline{\mathcal{I}}}\) :
-
invariant (inertia shape integral)
- \(K\) :
-
kinetic energy
- \(\underline{Q}{}_{\mathrm{e}}\) :
-
generalized external force vector
- \(\underline{Q}{}_{\mathrm{v}}\) :
-
quadratic velocity force vector
- \(\underline{\underline{\overline{S}}}\) :
-
matrix of shape functions expressed in floating coordinates
- \(V\) :
-
volume
- \(\underline{\zeta}\) :
-
vector of modal coordinates
- \(\underline{\theta }\) :
-
vector of orientation coordinates
- \(\underline{\lambda }\) :
-
vector of Lagrange multipliers
- \(\rho \) :
-
density
- \(\underline{\tau }\) :
-
position vector of floating frame
- \(\underline{\chi}{}_{\mathrm{o}}\) :
-
center of mass of undeformed body
- \(\underline{\omega }\) :
-
angular velocity vector
- \(\underline{\underline{\Theta}}{}_{\mathrm{o}}\) :
-
inertia tensor of undeformed body
- \(\underline{\Phi }\) :
-
vector of kinematic constraints
- \(\underline{\underline{^{q}\hspace {-2pt}\mathtt{J}\Phi }}\) :
-
Jacobian matrix of kinematic constraints
- \(\underline{\underline{\overline{\Psi}}}\) :
-
modal matrix expressed in floating coordinates
References
Baier, H., Seeßelberg, C., Specht, B.: Optimierung in der Strukturmechanik. LSS Verlag, Dortmund (2006)
Bestle, D.: Analyse und Optimierung Von Mehrkörpersystemen. Springer, Berlin (1994). https://doi.org/10.1007/978-3-642-52352-6
Bestle, D., Eberhard, P.: Analyzing and optimizing multibody systems. Mech. Struct. Mach. 20(1), 67–92 (1992). https://doi.org/10.1080/08905459208905161
Boopathy, K., Kennedy, G.J.: Adjoint-based derivative evaluation methods for flexible multibody systems with rotorcraft applications. In 55th AIAA Aerospace Sciences Meeting. American Institute of Aeronautics and Astronautics, Reston (2017). https://doi.org/10.2514/6.2017-1671
Craig, R.R., Bampton, M.C.C.: Coupling of substructures for dynamic analyses. AIAA J. 6(7), 1313–1319 (1968). https://doi.org/10.2514/3.4741
Dias, J.M.P., Pereira, M.S.: Sensitivity analysis of rigid-flexible multibody systems. Multibody Syst. Dyn. 1(3), 303–322 (1997). https://doi.org/10.1023/A:1009790202712
Fanghella, P., Galletti, C., Torre, G.: An explicit independent-coordinate formulation for the equations of motion of flexible multibody systems. Mech. Mach. Theory 38(5), 417–437 (2003). https://doi.org/10.1016/s0094-114x(02)00131-3
FunctionBay: Multi flexible body dynamics (2022). URL https://functionbay.com/documentation/onlinehelp/default.htm. RecurDyn ducumentation V9R4
Gufler, V., Wehrle, E., Achleitner, J., Vidoni, R.: Flexible multibody dynamics and sensitivity analysis in the design of a morphing leading edge for high-performance sailplanes. In: ECCOMAS Thematic Conference on Multibody Dynamics (2021). https://doi.org/10.3311/ECCOMASMBD2021-203
Gufler, V., Wehrle, E., Vidoni, R.: Multiphysical design optimization of multibody systems: application to a Tyrolean Weir cleaning mechanism. In: Mechanisms and Machine Science, pp. 459–467. Springer, Berlin (2021). https://doi.org/10.1007/978-3-030-55807-9_52
Gufler, V., Wehrle, E., Vidoni, R.: Sensitivity analysis of flexible multibody dynamics with generalized-\(\alpha \) time integration and Baumgarte stabilization. In: Mechanisms and Machine Science, pp. 147–155. Springer, Berlin (2022). https://doi.org/10.1007/978-3-031-10776-4_18
Gufler, V., Wehrle, E., Zwölfer, A.: A review of flexible multibody dynamics for gradient-based design optimization. Multibody Syst. Dyn. 53(4), 379–409 (2021). https://doi.org/10.1007/s11044-021-09802-z
Haftka, R.T., Gürdal, Z.: Elements of Structural Optimization, 3rd Edn. Kluwer, Dordrecht (1992). https://doi.org/10.1007/978-94-011-2550-5
Haug, E.J., Arora, J.S.: Design sensitivity analysis of elastic mechanical systems. Comput. Methods Appl. Mech. Eng. 15(1), 35–62 (1978). https://doi.org/10.1016/0045-7825(78)90004-X
Haug, E.J., Arora, J.S.: Applied Optimal Design: Mechanical and Structural Systems. Wiley, New York (1979)
Held, A., Knüfer, S., Seifried, R.: Structural sensitivity analysis of flexible multibody systems modeled with the floating frame of reference approach using the adjoint variable method. Multibody Syst. Dyn. 40, 287–302 (2017). https://doi.org/10.1007/s11044-016-9540-9
Hurty, W.C.: Dynamic analysis of structural systems using component modes. AIAA J. 3(4), 678–685 (1965). https://doi.org/10.2514/3.2947
MacNeal, R.H.: A hybrid method of component mode synthesis. Comput. Struct. 1(4), 581–601 (1971). https://doi.org/10.1016/0045-7949(71)90031-9
Martins, J.R.R.A., Hwang, J.T.: Review and unification of methods for computing derivatives of multidisciplinary computational models. AIAA J. 51(11), 2582–2599 (2013). https://doi.org/10.2514/1.J052184
Martins, J.R.R.A., Ning, A.: Engineering Design Optimization. Cambridge University Press, Cambridge (2021). https://mdobook.github.io/
MSC Software Corporation: Theory of flexible bodies (2020). URL https://help.mscsoftware.com/bundle/adams_2020/page/adams_help/Adams_Advanced_Package/flex/flex_theory/flex.theory.xhtml. Adams 2020 - Online Help (HTML)
Nachbagauer, K., Oberpeilsteiner, S., Sherif, K., Steiner, W.: The use of the adjoint method for solving typical optimization problems in multibody dynamics. J. Comput. Nonlinear Dyn. 10(6), 061011 (2015). https://doi.org/10.1115/1.4028417
Nejat, A.A., Moghadasi, A., Held, A.: Adjoint sensitivity analysis of flexible multibody systems in differential-algebraic form. Comput. Struct. 228, 106–148 (2020). https://doi.org/10.1016/j.compstruc.2019.106148
Orzechowski, G., Matikainen, M.K., Mikkola, A.M.: Inertia forces and shape integrals in the floating frame of reference formulation. Nonlinear Dyn. 88(3), 1953–1968 (2017). https://doi.org/10.1007/s11071-017-3355-y
Pi, T., Zhang, Y., Chen, L.: First order sensitivity analysis of flexible multibody systems using absolute nodal coordinate formulation. Multibody Syst. Dyn. 27, 153–171 (2012). https://doi.org/10.1007/s11044-011-9269-4
Rubin, S.: Improved component-mode representation for structural dynamic analysis. AIAA J. 13(8), 995–1006 (1975). https://doi.org/10.2514/3.60497
Shabana, A.A.: Dynamics of Multibody Systems, 5th edn. Cambridge University Press, Cambridge (2020). https://doi.org/10.1017/9781108757553
Sherif, K., Nachbagauer, K.: A detailed derivation of the velocity-dependent inertia forces in the floating frame of reference formulation. Journal of Computational and Nonlinear Dynamics 9(4), 044501 (2014). https://doi.org/10.1115/1.4026083
Wehrle, E., Gufler, V.: Lightweight engineering design of nonlinear dynamic systems with gradient-based structural design optimization. In: Proceedings of the Munich Symposium on Lightweight Design 2020, pp. 44–57. Springer, Berlin Heidelberg (2021). https://doi.org/10.1007/978-3-662-63143-0_5
Wehrle, E., Gufler, V.: Analytical sensitivity analysis of dynamic problems with direct differentiation of generalized-\(\alpha\) time integration. Submitted for publication (2022). https://doi.org/10.31224/osf.io/2mb6y
Zhang, M., Peng, H., Song, N.: Semi-analytical sensitivity analysis approach for fully coupled optimization of flexible multibody systems. Mech. Mach. Theory 159, 104256 (2021). https://doi.org/10.1016/j.mechmachtheory.2021.104256
Zwölfer, A., Gerstmayr, J.: Co-rotational formulations for 3D flexible multibody systems: A nodal-based approach. In: Contributions to Advanced Dynamics and Continuum Mechanics, pp. 243–263. Springer, Berlin (2019). https://doi.org/10.1007/978-3-030-21251-3_14
Zwölfer, A., Gerstmayr, J.: A concise nodal-based derivation of the floating frame of reference formulation for displacement-based solid finite elements. Multibody Syst. Dyn. 49, 291–313 (2020). https://doi.org/10.1007/s11044-019-09716-x
Zwölfer, A., Gerstmayr, J.: Consistent and inertia-shape-integrals-free invariants of the floating frame of reference formulation. In: Proceedings of the ASME 2020 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference (2020). https://doi.org/10.1115/DETC2020-22293
Zwölfer, A., Gerstmayr, J.: The nodal-based floating frame of reference formulation with modal reduction. Acta Mech. (2020). https://doi.org/10.1007/s00707-020-02886-2
Zwölfer, A., Gerstmayr, J.: State of the art and unification of corotational formulations for flexible multibody dynamics systems. J. Struct. Dyn. (2022). Submitted for publication
Acknowledgements
This work is supported by the project CRC 2017 – TN2091 doloMULTI \(\underline{D}\)esign \(\underline{o}\)f \(\underline{L}\)ightweight \(\underline{O}\)ptimized structures and systems under \(\underline{MULTI}\)disciplinary considerations through integration of \(\underline{MULTI}\)body dynamics in a \(\underline{MULTI}\)physics framework funded by the Free University of Bozen-Bolzano.
Author information
Authors and Affiliations
Contributions
V.G.: conceptualization, data curation, formal analysis, investigation, methodology, resources, software, validation, visualization, writing – original draft, writing – review & editing. A.Z.: conceptualization, formal analysis, investigation, methodology, supervision, validation, writing – original draft, writing – review & editing. E.W.: conceptualization, funding acquisition, methodology, project administration, supervision, writing – original draft, writing – review & editing.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Additional information
Publisher’s Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Gufler, V., Zwölfer, A. & Wehrle, E. Analytical derivatives of flexible multibody dynamics with the floating frame of reference formulation. Multibody Syst Dyn 60, 257–288 (2024). https://doi.org/10.1007/s11044-022-09858-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11044-022-09858-5




 :
: :
: :
: :
: :
: :
: :
: :
: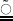 :
: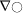 :
: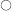 w.r.t.
w.r.t. 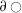 :
: w.r.t.
w.r.t.  :
: w.r.t.
w.r.t. 
 :
: w.r.t.
w.r.t. 
 :
: