SpringerLink
Log in

Contribution of nonlocal integral elasticity to modified strain gradient theory

  • Regular Article
  • Published:
The European Physical Journal Plus Aims and scope Submit manuscript

Abstract

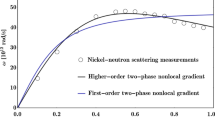
The nonlocal integral elasticity and the modified strain gradient theory are consistently integrated in the framework of the nonlocal modified gradient theory of elasticity. The equivalent differential formulation of the constitutive law, equipped with appropriate nonstandard boundary conditions, is introduced. The size-dependent effects of the dilatation gradient, deviatoric stretch gradient, and symmetric rotation gradient in addition to the nonlocality are beneficially captured in the flexure problem of nano-beams. The well posedness of the proposed nonlocal modified gradient problem is demonstrated via analytical examination of the elastostatic flexure and the wave dispersion phenomenon in nano-beams. The dispersive behavior of flexural waves is verified in comparison with the molecular dynamics simulation. The dominant stiffening effect of the gradient characteristic parameters associated with the nonlocal modified gradient elasticity is confirmed. Both the stiffening and softening responses of nano-structured materials are effectively realized in the framework of the introduced augmented elasticity theory. The conceived nonlocal modified gradient elasticity theory can accordingly provide a practical approach for nanoscopic study of the field quantities.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Canada)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

References

  1. R. Gopalakrishnan, R. Kaveri, Using graphene oxide to improve the mechanical and electrical properties of fiber-reinforced high-volume sugarcane bagasse ash cement mortar. Eur. Phys. J. Plus 136, 202 (2021). https://doi.org/10.1140/epjp/s13360-021-01179-4

    Article  Google Scholar 

  2. M. Mirnezhad, R. Ansari, S.R. Falahatgar, Quantum effects on the mechanical properties of fine-scale CNTs: an approach based on DFT and molecular mechanics model. Eur. Phys. J. Plus 135, 908 (2020). https://doi.org/10.1140/epjp/s13360-020-00878-8

    Article  Google Scholar 

  3. I. Sevostianov, M. Kachanov, Evaluation of the incremental compliances of non-elliptical contacts by treating them as external cracks. Eur. J. Mech. A. Solids 85, 104114 (2021). https://doi.org/10.1016/j.euromechsol.2020.104114

    Article  ADS  MathSciNet  MATH  Google Scholar 

  4. K. Duan, L. Li, F. Wang, S. Liu, Y. Hu, X. Wang, New insights into interface interactions of CNT-reinforced epoxy nanocomposites. Compos. Sci. Technol. 204, 108638 (2021). https://doi.org/10.1016/j.compscitech.2020.108638

    Article  Google Scholar 

  5. M. Shaat, E. Ghavanloo, S.A. Fazelzadeh, Review on nonlocal continuum mechanics: physics, material applicability, and mathematics. Mech. Mater. 150, 103587 (2020). https://doi.org/10.1016/j.mechmat.2020.103587

    Article  Google Scholar 

  6. A. Farajpour, M.H. Ghayesh, H. Farokhi, A review on the mechanics of nanostructures. Int. J. Eng. Sci. 133, 231–263 (2018). https://doi.org/10.1016/j.ijengsci.2018.09.006

    Article  MathSciNet  MATH  Google Scholar 

  7. Z. Li, Y. He, J. Lei, S. Guo, D. Liu, L. Wang, A standard experimental method for determining the material length scale based on modified couple stress theory. Int. J. Mech. Sci. 141, 198–205 (2018). https://doi.org/10.1016/j.ijmecsci.2018.03.035

    Article  Google Scholar 

  8. L. Sun, R.P.S. Han, J. Wang, C.T. Lim, Modeling the size-dependent elastic properties of polymeric nanofibers. Nanotechnol. 19, 455706 (2008). https://doi.org/10.1088/0957-4484/19/45/455706

    Article  ADS  Google Scholar 

  9. D.C.C. Lam, F. Yang, A.C.M. Chong, J. Wang, P. Tong, Experiments and theory in strain gradient elasticity. J. Mech. Phys. Solids 51, 1477–1508 (2003). https://doi.org/10.1016/S0022-5096(03)00053-X

    Article  ADS  MATH  Google Scholar 

  10. R.D. Mindlin, Second gradient of strain and surface-tension in linear elasticity. Int. J. Solids Struct. 1, 417–438 (1965). https://doi.org/10.1016/0020-7683(65)90006-5

    Article  Google Scholar 

  11. R.K. Poonam, K. Sahrawat, K. Kumar, Plane wave propagation in functionally graded isotropic couple stress thermoelastic solid media under initial stress and gravity. Eur. Phys. J. Plus 136, 114 (2021). https://doi.org/10.1140/epjp/s13360-021-01097-5

    Article  Google Scholar 

  12. W. Sae-Long, S. Limkatanyu, J. Rungamornrat, W. Prachasaree, P. Sukontasukkul, H.M. Sedighi, A rational beam-elastic substrate model with incorporation of beam-bulk nonlocality and surface-free energy. Eur. Phys. J. Plus 136, 80 (2021). https://doi.org/10.1140/epjp/s13360-020-00992-7

    Article  Google Scholar 

  13. S.K. Jena, S. Chakraverty, R.M. Jena, Stability analysis of Timoshenko nanobeam with material uncertainties using a double-parametric form-based analytical approach and Monte Carlo simulation technique. Eur. Phys. J. Plus 135, 536 (2020). https://doi.org/10.1140/epjp/s13360-020-00549-8

    Article  Google Scholar 

  14. A. Norouzzadeh, R. Ansari, On the isogeometric analysis of geometrically nonlinear shell structures with the consideration of surface energies. Eur. Phys. J. Plus 135, 264 (2020). https://doi.org/10.1140/epjp/s13360-020-00257-3

    Article  Google Scholar 

  15. M.H. Ghayesh, Viscoelastic dynamics of axially FG microbeams. Int. J. Eng. Sci. 135, 75–85 (2019). https://doi.org/10.1016/j.ijengsci.2018.10.005

    Article  MathSciNet  MATH  Google Scholar 

  16. M.H. Ghayesh, Dynamics of functionally graded viscoelastic microbeams. Int. J. Eng. Sci. 124, 115–131 (2018). https://doi.org/10.1016/j.ijengsci.2017.11.004

    Article  MathSciNet  MATH  Google Scholar 

  17. B. Akgöz, Ö. Civalek, Effects of thermal and shear deformation on vibration response of functionally graded thick composite microbeams. Compos. Part B 129, 77–87 (2017). https://doi.org/10.1016/j.compositesb.2017.07.024

    Article  Google Scholar 

  18. M.A. Wheel, J.C. Frame, P.E. Riches, Is smaller always stiffer? On size effects in supposedly generalised continua. Int. J. Solids Struct. 67–68, 84–92 (2015). https://doi.org/10.1016/j.ijsolstr.2015.03.026

    Article  Google Scholar 

  19. G. Kumar, A. Desai, J. Schroers, Bulk metallic glass: the smaller the better. Adv. Mater. 23, 461–476 (2010). https://doi.org/10.1002/adma.201002148

    Article  Google Scholar 

  20. L.G. Zhou, H. Huang, Are surfaces elastically softer or stiffer. Appl. Phys. Lett. 84, 1940 (2004). https://doi.org/10.1063/1.1682698

    Article  ADS  Google Scholar 

  21. A.C. Eringen, Nonlocal Continuum Field Theories (Springer, New York, 2002). https://springer.longhoe.net/book/https://doi.org/10.1007/b97697

  22. A.A. Pisano, P. Fuschi, C. Polizzotto, Integral and differential approaches to Eringen’s nonlocal elasticity models accounting for boundary effects with applications to beams in bending. ZAMM (2021). https://doi.org/10.1002/zamm.202000152

    Article  Google Scholar 

  23. A.A. Pisano, P. Fuschi, C. Polizzotto, A strain-difference based nonlocal elasticity theory for small-scale shear-deformable beams with parametric war**. Int. J. Multiscale Comput. Eng. 18, 83–102 (2020). https://doi.org/10.1615/IntJMultCompEng.2019030885

    Article  Google Scholar 

  24. P. Fuschi, A.A. Pisano, C. Polizzotto, Size effects of small-scale beams in bending addressed with a strain-difference based nonlocal elasticity theory. Int. J. Mech. Sci. 151, 661–671 (2019). https://doi.org/10.1016/j.ijmecsci.2018.12.024

    Article  Google Scholar 

  25. L. Li, R. Lin, T.Y. Ng, Contribution of nonlocality to surface elasticity. Int. J. Eng. Sci. 152, 103311 (2020). https://doi.org/10.1016/j.ijengsci.2020.103311

    Article  MathSciNet  MATH  Google Scholar 

  26. S.A. Faghidian, Higher-order nonlocal gradient elasticity: a consistent variational theory. Int. J. Eng. Sci. 154, 103337 (2020). https://doi.org/10.1016/j.ijengsci.2020.103337

    Article  MathSciNet  MATH  Google Scholar 

  27. S.A. Faghidian, Two-phase local/nonlocal gradient mechanics of elastic torsion. Math. Methods Appl. Sci. (2020). https://doi.org/10.1002/mma.6877

    Article  Google Scholar 

  28. S.A. Faghidian, Higher-order mixture nonlocal gradient theory of wave propagation. Math. Methods Appl. Sci. (2020). https://doi.org/10.1002/mma.6885

    Article  MATH  Google Scholar 

  29. X.W. Zhu, L. Li, A well-posed Euler–Bernoulli beam model incorporating nonlocality and surface energy effect. Appl. Math. Mech. 40, 1561–1588 (2019). https://doi.org/10.1007/s10483-019-2541-5

    Article  MathSciNet  MATH  Google Scholar 

  30. M. Arefi, G. Ghasemian Talkhunche, Higher-order vibration analysis of FG cylindrical nano-shell. Eur. Phys. J. Plus 136, 154 (2021). https://doi.org/10.1140/epjp/s13360-021-01096-6

    Article  Google Scholar 

  31. S. Aydinlik, A. Kiris, W. Sumelka, Nonlocal vibration analysis of microstretch plates in the framework of space-fractional mechanics-theory and validation. Eur. Phys. J. Plus 136, 169 (2021). https://doi.org/10.1140/epjp/s13360-021-01110-x

    Article  Google Scholar 

  32. H.M. Shodja, H. Moosavian, Weakly nonlocal micromorphic elasticity for diamond structures vis-à-vis lattice dynamics. Mech. Mater. 147, 103365 (2020). https://doi.org/10.1016/j.mechmat.2020.103365

    Article  Google Scholar 

  33. R. Ansari, J. Torabi, A. Norouzzadeh, An integral nonlocal model for the free vibration analysis of Mindlin nanoplates using the VDQ method. Eur. Phys. J. Plus. 135, 206 (2020). https://doi.org/10.1140/epjp/s13360-019-00018-x

    Article  Google Scholar 

  34. S.K. Jena, S. Chakraverty, M. Malikan, Vibration and buckling characteristics of nonlocal beam placed in a magnetic field embedded in Winkler-Pasternak elastic foundation using a new refined beam theory: an analytical approach. Eur. Phys. J. Plus 135, 164 (2020). https://doi.org/10.1140/epjp/s13360-020-00176-3

    Article  Google Scholar 

  35. S.K. Jena, S. Chakraverty, Dynamic behavior of an electromagnetic nanobeam using the Haar wavelet method and the higher-order Haar wavelet method. Eur. Phys. J. Plus 134, 538 (2019). https://doi.org/10.1140/epjp/i2019-12874-8

    Article  Google Scholar 

  36. Ö. Civalek, B. Uzun, M.Ö. Yaylı, B. Akgöz, Size-dependent transverse and longitudinal vibrations of embedded carbon and silica carbide nanotubes by nonlocal finite element method. Eur. Phys. J. Plus 135, 381 (2020). https://doi.org/10.1140/epjp/s13360-020-00385-w

    Article  Google Scholar 

  37. I. Elishakoff, A. Ajenjo, D. Livshits, Generalization of Eringen’s result for random response of a beam on elastic foundation. Eur. J. Mech. A Solids 81, 103931 (2020). https://doi.org/10.1016/j.euromechsol.2019.103931

    Article  ADS  MathSciNet  MATH  Google Scholar 

  38. F. Hache, N. Challamel, I. Elishakoff, Asymptotic derivation of nonlocal beam models from two-dimensional nonlocal elasticity. Math. Mech. Solids. 24, 2425–2443 (2019). https://doi.org/10.1177/1081286518756947

    Article  MathSciNet  MATH  Google Scholar 

  39. E.C. Aifantis, Update on a class of gradient theories. Mech. Mater. 35, 259–280 (2003). https://doi.org/10.1016/S0167-6636(02)00278-8

    Article  Google Scholar 

  40. R. Zaera, Ó. Serrano, J. Fernández-Sáez, Non-standard and constitutive boundary conditions in nonlocal strain gradient elasticity. Meccanica 55, 469–479 (2020). https://doi.org/10.1007/s11012-019-01122-z

    Article  MathSciNet  Google Scholar 

  41. R. Zaera, Ó. Serrano, J. Fernández-Sáez, On the consistency of the nonlocal strain gradient elasticity. Int. J. Eng. Sci. 138, 65–81 (2019). https://doi.org/10.1016/j.ijengsci.2019.02.004

    Article  MathSciNet  MATH  Google Scholar 

  42. H. Babaei, M.R. Eslami, Study on nonlinear vibrations of temperature- and size-dependent FG porous arches on elastic foundation using nonlocal strain gradient theory. Eur. Phys. J. Plus 136, 24 (2021). https://doi.org/10.1140/epjp/s13360-020-00959-8

    Article  Google Scholar 

  43. F. Li, S. Esmaeili, On thermoelastic dam** in axisymmetric vibrations of circular nanoplates: incorporation of size effect into structural and thermal areas. Eur. Phys. J. Plus 136, 194 (2021). https://doi.org/10.1140/epjp/s13360-021-01084-w

    Article  Google Scholar 

  44. R. Barretta, S.A. Faghidian, F. Marotti de Sciarra, M.S. Vaccaro, Nonlocal strain gradient torsion of elastic beams: variational formulation and constitutive boundary conditions. Arch. Appl. Mech. 90, 691–706 (2020). https://doi.org/10.1007/s00419-019-01634-w

    Article  ADS  Google Scholar 

  45. R. Barretta, S.A. Faghidian, F. Marotti de Sciarra, F.P. Pinnola, Timoshenko nonlocal strain gradient nanobeams: variational consistency, exact solutions and carbon nanotube Young moduli. Mech. Adv. Mater. Struct. (2019). https://doi.org/10.1080/15376494.2019.1683660

    Article  Google Scholar 

  46. M. Alakel Abazid, 2D magnetic field effect on the thermal buckling of metal foam nanoplates reinforced with FG-GPLs lying on Pasternak foundation in humid environment. Eur. Phys. J. Plus 135, 910 (2020). https://doi.org/10.1140/epjp/s13360-020-00905-8

    Article  Google Scholar 

  47. I. Elishakoff, Handbook on Timoshenko-Ehrenfest Beam and Uflyand-Mindlin Plate Theories (World Scientific, Singapore, 2019). https://doi.org/10.1142/10890

    Book  Google Scholar 

  48. S.A. Faghidian, Unified formulation of the stress field of saint-Venant’s flexure problem for symmetric cross-sections. Int. J. Mech. Sci. 111–112, 65–72 (2016). https://doi.org/10.1016/j.ijmecsci.2016.04.003

    Article  Google Scholar 

  49. B. Akgöz, Ö. Civalek, Strain gradient elasticity and modified couple stress models for buckling analysis of axially loaded micro-scaled beams. Int. J. Eng. Sci. 49, 1268–1280 (2011). https://doi.org/10.1016/j.ijengsci.2010.12.009

    Article  MathSciNet  MATH  Google Scholar 

  50. M.H. Kahrobaiyan, M. Asghari, M.T. Ahmadian, Strain gradient beam element. Finite Elem. Anal. Des. 68, 63–75 (2013). https://doi.org/10.1016/j.finel.2012.12.006

    Article  MathSciNet  MATH  Google Scholar 

  51. G. Romano, M. Diaco, On formulation of nonlocal elasticity problems. Meccanica (2020). https://doi.org/10.1007/s11012-020-01183-5

    Article  Google Scholar 

  52. S.A. Faghidian, Flexure mechanics of nonlocal modified gradient nano-beams. J. Comput. Des. Eng. (2021). https://doi.org/10.1093/jcde/qwab027

    Article  Google Scholar 

  53. R. Barretta, S.A. Faghidian, F. Marotti de Sciarra, R. Penna, F.P. Pinnola, On torsion of nonlocal Lam strain gradient FG elastic beams. Compos. Struct. 233, 111550 (2020). https://doi.org/10.1016/j.compstruct.2019.111550

    Article  Google Scholar 

  54. A. Shakouri, T.Y. Ng, R.M. Lin, A study of the scale effects on the flexural vibration of graphene sheets using REBO potential based atomistic structural and nonlocal couple stress thin plate models. Phys. E. 50, 22–28 (2013). https://doi.org/10.1016/j.physe.2013.02.024

    Article  Google Scholar 

  55. A. Saha, B. Pradhan, S. Banerjee, Bifurcation analysis of quantum ion-acoustic kink, anti-kink and periodic waves of the Burgers equation in a dense quantum plasma. Eur. Phys. J. Plus 135, 216 (2020). https://doi.org/10.1140/epjp/s13360-020-00235-9

    Article  Google Scholar 

  56. A. Saha, P. Chatterjee, S. Banerjee, An open problem on supernonlinear waves in a two-component Maxwellian plasma. Eur. Phys. J. Plus 135, 801 (2020). https://doi.org/10.1140/epjp/s13360-020-00816-8

    Article  Google Scholar 

  57. D. de Domenico, H. Askes, E.C. Aifantis, Gradient elasticity and dispersive wave propagation: model motivation and length scale identification procedures in concrete and composite laminates. Int. J. Solids Struct. 158, 176–190 (2019). https://doi.org/10.1016/j.ijsolstr.2018.09.007

    Article  Google Scholar 

  58. D. de Domenico, H. Askes, Nano-scale wave dispersion beyond the First Brillouin Zone simulated with inertia gradient continua. J. Appl. Phys. 124, 205107 (2018). https://doi.org/10.1063/1.5045838

    Article  Google Scholar 

  59. L. Wang, H. Hu, Flexural wave propagation in single-walled carbon nanotubes. Phys. Rev. B 71, 195412 (2005). https://doi.org/10.1103/PhysRevB.71.195412

    Article  ADS  Google Scholar 

  60. S.A. Faghidian, Inverse determination of the regularized residual stress and eigenstrain fields due to surface peening. J. Strain Anal. Eng. Des. 50, 84–91 (2015). https://doi.org/10.1177/0309324714558326

    Article  Google Scholar 

  61. S.A. Faghidian, A smoothed inverse eigenstrain method for reconstruction of the regularized residual fields. Int. J. Solids Struct. 51, 4427–4434 (2014). https://doi.org/10.1016/j.ijsolstr.2014.09.012

    Article  Google Scholar 

  62. G.H. Farrahi, S.A. Faghidian, D.J. Smith, Reconstruction of residual stresses in autofrettaged thick-walled tubes from limited measurements. Int. J. Press. Vessels Pip. 86, 777–784 (2009). https://doi.org/10.1016/j.ijpvp.2009.03.010

    Article  Google Scholar 

  63. M.A. Khorshidi, Validation of weakening effect in modified couple stress theory: dispersion analysis of carbon nanotubes. Int. J. Mech. Sci. 170, 105358 (2020). https://doi.org/10.1016/j.ijmecsci.2019.105358

    Article  Google Scholar 

  64. C.A.L. Bailer-Jones, Practical Bayesian Inference: A Primer for Physical Scientists (Cambridge University Press, Cambridge, 2017). https://doi.org/10.1017/9781108123891

    Book  Google Scholar 

  65. M.A. Caprio, LevelScheme: a level scheme drawing and scientific figure preparation system for Mathematica. Comput. Phys. Commun. 171, 107–118 (2005). https://doi.org/10.1016/j.cpc.2005.04.010

    Article  ADS  MATH  Google Scholar 

Download references

Funding

This research did not receive any specific grant from funding agencies in the public, commercial, or not-for-profit sectors.

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering, Science and Research Branch, Islamic Azad University, Tehran, Iran

    S. Ali Faghidian

Authors
  1. S. Ali Faghidian
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to S. Ali Faghidian.

Ethics declarations

Conflict of interest

The author declares that there is not a known competing financial interest or personal relationship that could have appeared to influence the work reported in this paper.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Faghidian, S.A. Contribution of nonlocal integral elasticity to modified strain gradient theory. Eur. Phys. J. Plus 136, 559 (2021). https://doi.org/10.1140/epjp/s13360-021-01520-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1140/epjp/s13360-021-01520-x

Search

Navigation