SpringerLink
Log in

Error Estimate of a Fully Discrete Local Discontinuous Galerkin Method for Variable-Order Time-Fractional Diffusion Equations

  • Original Paper
  • Published:
Communications on Applied Mathematics and Computation Aims and scope Submit manuscript

Abstract

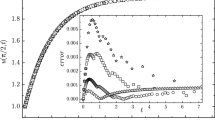
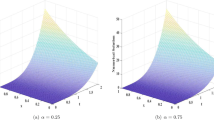
The aim of this paper is to develop a fully discrete local discontinuous Galerkin method to solve a class of variable-order fractional diffusion problems. The scheme is discretized by a weighted-shifted Grünwald formula in the temporal discretization and a local discontinuous Galerkin method in the spatial direction. The stability and the \(L^2\)-convergence of the scheme are proved for all variable-order \(\alpha (t)\in (0,1)\). The proposed method is of accuracy-order \(O(\tau ^3+h^{k+1})\) , where \(\tau\), h, and k are the temporal step size, the spatial step size, and the degree of piecewise \(P^k\) polynomials, respectively. Some numerical tests are provided to illustrate the accuracy and the capability of the scheme.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Germany)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1

Similar content being viewed by others

References

  1. Almeida, R., Tavares, D., Torres, D.: The Variable-Order Fractional Calculus of Variations. Springer, Berlin (2019)

    MATH  Google Scholar 

  2. Baleanu, D., Machado, J., Luo, A.: Fractional Dynamics and Control. Springer Science & Business Media, Berlin (2011)

    Google Scholar 

  3. Burrage, K., Cardone, A., Ambrosio, R., Paternoster, B.: Numerical solution of time fractional diffusion systems. Appl. Numer. Math. 116, 82–94 (2017)

    MathSciNet  MATH  Google Scholar 

  4. Baeumer, B., Kovacs, M., Meerschaert, M.: Numerical solutions for fractional reaction-diffusion equations. Comput. Math. Appl. 55, 2212–2226 (2008)

    MathSciNet  MATH  Google Scholar 

  5. Bhrawy, A., Zaky, M.: Numerical simulation for two-dimensional variable-order fractional nonlinear cable equation. Nonlinear Dyn. 80, 101–116 (2015)

    MathSciNet  MATH  Google Scholar 

  6. Chen, H., Lü, S., Chen, W.: Finite difference/spectral approximations for the distributed order time fractional reaction-diffusion equation on an unbounded domain. J. Comput. Phys. 315, 84–97 (2016)

    MathSciNet  MATH  Google Scholar 

  7. Chen, C., Liu, F., Burrage, K.: Finite difference methods and a Fourier analysis for the fractional reaction-subdiffusion equation. Appl. Math. Comput. 198, 754–769 (2008)

    MathSciNet  MATH  Google Scholar 

  8. Coimbra, C.: Mechanics with variable-order differential operators. Ann. Phys. 12, 692–703 (2003)

    MathSciNet  MATH  Google Scholar 

  9. Cui, M.: Compact alternating direction implicit method for two-dimensional time fractional diffusion equation. J. Comput. Phys. 231, 2621–2633 (2012)

    MathSciNet  MATH  Google Scholar 

  10. Chen, C., Liu, F., Anh, V., Turner, I.: Numerical schemes with high spatial accuracy for a variable-order anomalous subdiffusion equation. SIAM J. Sci. Comput. 32, 1740–1760 (2010)

    MathSciNet  MATH  Google Scholar 

  11. Cao, J., Qiu, Y., Song, G.: A compact finite difference scheme for variable order subdiffusion equation. Commun. Nonlinear Sci. Numer. Simul. 48, 140–149 (2017)

    MathSciNet  MATH  Google Scholar 

  12. Dabiri, A., Moghaddam, B., Machado, J.A.T.: Optimal variable-order fractional PID controllers for dynamical systems. J. Comput. Appl. Math. 339, 40–48 (2018)

    MathSciNet  MATH  Google Scholar 

  13. Deng, W., Hesthaven, J.S.: Local discontinuous Galerkin methods for fractional diffusion equations. ESAIM Math. Model. Numer. Anal. 47, 1845–1864 (2013)

    MathSciNet  MATH  Google Scholar 

  14. Du, Y., Liu, Y., Li, H., Fang, Z., He, S.: Local discontinuous Galerkin method for a nonlinear time-fractional fourth-order partial differential equation. J. Comput. Phys. 344, 108–126 (2017)

    MathSciNet  MATH  Google Scholar 

  15. Evans, K.P., Jacob, N.: Feller semigroups obtained by variable order subordination. (2006). ar**v:math/0608056

  16. Guo, L., Wang, Z., Vong, S.: Fully discrete local discontinuous Galerkin methods for some time-fractional fourth-order problems. Int. J. Comput. Math. 93(10), 1665–1682 (2016)

    MathSciNet  MATH  Google Scholar 

  17. Haq, S., Ghafoor, A., Hussain, M.: Numerical solutions of variable order time fractional (1 + 1)- and (1 + 2)-dimensional advection dispersion and diffusion models. Appl. Math. Comput. 360, 107–121 (2019)

    MathSciNet  MATH  Google Scholar 

  18. Hajipour, M., Jajarmi, A., Baleanu, D., Sun, H.: On an accurate discretization of a variable-order fractional reaction-diffusion equation. Commun. Nonlinear Sci. Numer. Simul. 69, 119–133 (2019)

    MathSciNet  MATH  Google Scholar 

  19. Jiang, Y., Ma, J.: High-order finite element methods for time-fractional partial differential equations. J. Comput. Appl. Math. 235, 3285–3290 (2011)

    MathSciNet  MATH  Google Scholar 

  20. Jiang, W., Liu, N.: A numerical method for solving the time variable fractional order mobile-immobile advection dispersion model. Appl. Numer. Math. 119, 18–32 (2017)

    MathSciNet  MATH  Google Scholar 

  21. Ji, C., Sun, Z.: A high-order compact finite difference scheme for the fractional sub-diffusion equation. J. Sci. Comput. 64, 959–985 (2015)

    MathSciNet  MATH  Google Scholar 

  22. Li, C., Deng, W., Zhao, L.: Well-posedness and numerical algorithm for the tempered fractional differential equations. Discret. Contin. Dyn. Syst. Ser. B 24, 1989–2015 (2019)

    MathSciNet  MATH  Google Scholar 

  23. Li, C., Wang, Z.: The discontinuous Galerkin finite element method for Caputo-type nonlinear conservation law. Math. Comput. Simul. 169, 51–73 (2020)

    MathSciNet  MATH  Google Scholar 

  24. Li, C., Wang, Z.: The local discontinuous Galerkin finite element methods for Caputo-type partial differential equations: mathematical analysis. Appl. Numer. Math. 150, 587–606 (2020)

    MathSciNet  MATH  Google Scholar 

  25. Li, M., Gu, X., Huang, C., Fei, M., Zhang, G.: A fast linearized conservative finite element method for the strongly coupled nonlinear fractional Schrödinger equations. J. Comput. Phys. 358, 256–282 (2018)

    MathSciNet  MATH  Google Scholar 

  26. Lin, R., Liu, F., Anh, V., Turner, I.: Stability and convergence of a new explicit finite-difference approximation for the variable-order nonlinear fractional diffusion equation. Appl. Math. Comput. 212, 435–445 (2009)

    MathSciNet  MATH  Google Scholar 

  27. Moghaddam, B., Machado, J.: Extended algorithms for approximating variable order fractional derivatives with applications. J. Sci. Comput. 71, 1351–1374 (2017)

    MathSciNet  MATH  Google Scholar 

  28. Holte, J.M.: Discrete Gronwall lemma and applications. In: MAA-NCS Meeting at the University of North Dakota (2009)

  29. Liu, Y., Yan, Y., Khan, M.: Discontinuous Galerkin time step** method for solving linear space fractional partial differential equations. Appl. Numer. Math. 115, 200–213 (2017)

    MathSciNet  MATH  Google Scholar 

  30. Liu, Y., Zhang, M., Li, H., Li, J.: High-order local discontinuous Galerkin method combined with WSGD-approximation for a fractional subdiffusion equation. Comput. Math. Appl. 73(6), 1298–1314 (2017)

    MathSciNet  MATH  Google Scholar 

  31. Lorenzo, C., Hartley, T.: Variable order and distributed order fractional operators. Nonlinear Dyn. 29, 57–98 (2002)

    MathSciNet  MATH  Google Scholar 

  32. Mustapha, K., McLean, W.: Superconvergence of a discontinuous Galerkin method for fractional diffusion and wave equations. SIAM J. Numer. Anal. 51(1), 491–515 (2013)

    MathSciNet  MATH  Google Scholar 

  33. Podlubny, I.: Fractional Differential Equations. Mathematics in Science and Engineering, vol. 198. Academic Press, San Diego (1999)

    MATH  Google Scholar 

  34. Shen, S., Liu, F., Chen, J., Turner, I., Anh, V.: Numerical techniques for the variable order time fractional diffusion equation. Appl. Math. Comput. 218, 10861–10870 (2012)

    MathSciNet  MATH  Google Scholar 

  35. Sun, H.G., Chen, W., Wei, H., Chen, Y.Q.: A comparative study of constant-order and variable-order fractional models in characterizing memory property of systems. Eur. Phys. J. Spec. Top. 193, 185–192 (2011)

    Google Scholar 

  36. Sun, Z.Z., Wu, X.: A fully discrete difference scheme for a diffusion-wave system. Appl. Numer. Math. 56, 193–209 (2006)

    MathSciNet  MATH  Google Scholar 

  37. Tavares, D., Almeida, R., Torres, D.: Caputo derivatives of fractional variable order: numerical approximations. Commun. Nonlinear Sci. Numer. Simul. 35, 69–87 (2016)

    MathSciNet  MATH  Google Scholar 

  38. Tarasov, V.: Partial fractional derivatives of Riesz type and nonlinear fractional differential equations. Nonlinear Dyn. 86, 1745–1759 (2016)

    MathSciNet  MATH  Google Scholar 

  39. Wang, H., Zheng, X.: Wellposedness and regularity of the variable-order time-fractional diffusion equations. J. Math. Anal. Appl. 475, 1778–1802 (2019)

    MathSciNet  MATH  Google Scholar 

  40. Wang, H., Zheng, X.: Analysis and numerical solution of a nonlinear variable-order fractional differential equation. Adv. Comput. Math. (2019). https://doi.org/10.1007/s10444-019-09690-0

    Article  MathSciNet  Google Scholar 

  41. Wei, L.L., He, Y.N.: Analysis of a fully discrete local discontinuous Galerkin method for time-fractional fourth-order problems. Appl. Math. Model. 38, 1511–1522 (2014)

    MathSciNet  MATH  Google Scholar 

  42. Wei, L.L.: Analysis of a new finite difference/local discontinuous Galerkin method for the fractional diffusion-wave equation. Appl. Math. Comput. 304, 180–189 (2017)

    MathSciNet  MATH  Google Scholar 

  43. Wei, L.L.: Stability and convergence of a fully discrete local discontinuous Galerkin method for multi-term time fractional diffusion equations. Numer. Algorithms 76, 695–707 (2017)

    MathSciNet  MATH  Google Scholar 

  44. Xu, Q.W., Hesthaven, J.S.: Discontinuous Galerkin method for fractional convection–diffusion equations. SIAM J. Numer. Anal. 52, 405–423 (2014)

    MathSciNet  MATH  Google Scholar 

  45. Xu, Y., Shu, C.-W.: Optimal error estimates of the semidiscrete local discontinuous Galerkin methods for high order wave equations. SIAM J. Numer. Anal. 50(1), 79–104 (2012)

    MathSciNet  MATH  Google Scholar 

  46. Yang, J., Yao, H., Wu, B.: An efficient numerical method for variable order fractional functional differential equation. Appl. Math. Lett. 76, 221–226 (2018)

    MathSciNet  MATH  Google Scholar 

  47. Yaseen, M., Abbas, M., Nazir, T., Baleanu, D.: A finite difference scheme based on cubic trigonometric B-splines for a time fractional diffusion-wave equation. Adv. Differ. Equ. 2017, 274 (2017). https://doi.org/10.1186/s13662-017-1330-z

    Article  MathSciNet  MATH  Google Scholar 

  48. Yu, B., Jiang, X.Y.: Numerical identification of the fractional derivatives in the two-dimensional fractional cable equation. J. Sci. Comput. 68(1), 252–272 (2016)

    MathSciNet  MATH  Google Scholar 

  49. Zayernouri, M., Karniadakis, G.: Fractional spectral collocation methods for linear and nonlinear variable order FPDEs. J. Comput. Phys. 293, 312–338 (2015)

    MathSciNet  MATH  Google Scholar 

  50. Zeng, F., Zhang, Z., Karniadakis, G.: A generalized spectral collocation method with tunable accuracy for variable-order fractional differential equations. SIAM J. Sci. Comput. 37, A2710–A2732 (2015)

    MathSciNet  MATH  Google Scholar 

  51. Zhai, S., Weng, Z., Feng, X.: Fast explicit operator splitting method and time-step adaptivity for fractional non-local Allen–Cahn model. Appl. Math. Model. 40, 1315–1324 (2016)

    MathSciNet  MATH  Google Scholar 

  52. Zhang, M., Liu, Y., Li, H.: High-order local discontinuous Galerkin algorithm with time second-order schemes for the two-dimensional nonlinear fractional diffusion equation. Commun. Appl. Math. Comput. (2020). https://doi.org/10.1007/s42967-019-00058-1

    Article  MathSciNet  Google Scholar 

  53. Zhang, Q., Shu, C.-W.: Stability analysis and a priori error estimates of the third order explicit Runge–Kutta discontinuous Galerkin method for scalar conservation laws. SIAM. J. Numer. Anal. 48, 1038–1063 (2010)

    MathSciNet  MATH  Google Scholar 

  54. Zhang, Q., Shu, C.-W.: Error estimate for the third order explicit Runge–Kutta discontinuous Galerkin method for a linear hyperbolic equation with discontinuous initial solution. Numer. Math. 126, 703–740 (2014)

    MathSciNet  MATH  Google Scholar 

  55. Zhang, H., Liu, F., Jiang, X., Zeng, F., Turner, I.: A Crank–Nicolson ADI Galerkin–Legendre spectral method for the two-dimensional Riesz space distributed-order advection-diffusion equation. Comput. Math. Appl. 76(10), 2460–2476 (2018)

    MathSciNet  MATH  Google Scholar 

  56. Zhao, Y.M., Zhang, Y., Liu, F., Turner, I., Tang, Y., Anh, V.: Convergence and superconvergence of a fully-discrete scheme for multi-term time fractional diffusion equations. Comput. Math. Appl. 73, 1087–1099 (2017)

    MathSciNet  MATH  Google Scholar 

  57. Zhao, L., Deng, W.: A series of high-order quasi-compact schemes for space fractional diffusion equations based on the superconvergent approximations for fractional derivatives. Numer. Methods Partial. Differ. Equ. 31, 1345–1381 (2015)

    MathSciNet  MATH  Google Scholar 

  58. Zheng, M., Liu, F., Turner, I., Anh, V.: A novel high order space-time spectral method for the time-fractional Fokker–Planck equation. SIAM J. Sci. Comput. 37(2), A701–A724 (2015)

    MathSciNet  MATH  Google Scholar 

  59. Zheng, Y.Y., Zhao, Z.G.: The discontinuous Galerkin finite element method for fractional cable equation. Appl. Numer. Math. 115, 32–41 (2017)

    MathSciNet  MATH  Google Scholar 

  60. Zhuang, P., Liu, F., Anh, V., Turner, I.: Numerical methods for the variable-order fractional advection-diffusion equation with a nonlinear source term. SIAM. J. Numer. Anal. 47, 1760–1781 (2009)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

This work was supported by the Fundamental Research Funds for the Henan Provincial Colleges and Universities in Henan University of Technology (2018RCJH10), the Training Plan of Young Backbone Teachers in Henan University of Technology (21420049), the Training Plan of Young Backbone Teachers in Colleges and Universities of Henan Province (2019GGJS094), the innovative Funds Plan of Henan University of Technology,  Foundation of Henan Educational Committee (19A110005), and the National Natural Science Foundation of China (11861068).

Author information

Authors and Affiliations

  1. College of Science, Henan University of Technology, Zhengzhou, 450001, China

    Leilei Wei

  2. School of Mathematical Sciences, Huaqiao University, Quanzhou, 362021, Fujian Province, China

    Shuying Zhai

  3. College of Mathematics Sciences, **njiang Normal University, Urumqi, 830054, China

    **ndong Zhang

Authors
  1. Leilei Wei
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Shuying Zhai
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. **ndong Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Leilei Wei.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wei, L., Zhai, S. & Zhang, X. Error Estimate of a Fully Discrete Local Discontinuous Galerkin Method for Variable-Order Time-Fractional Diffusion Equations. Commun. Appl. Math. Comput. 3, 429–443 (2021). https://doi.org/10.1007/s42967-020-00081-7

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s42967-020-00081-7

Keywords

Mathematics Subject Classification

Search

Navigation