SpringerLink
Log in

Cubic Polynomial Spline Scheme for Fractional Boundary Value Problems with Left and Right Fractional Operators

  • Original Paper
  • Published:
International Journal of Applied and Computational Mathematics Aims and scope Submit manuscript

Abstract

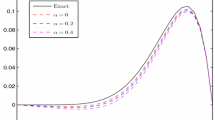
In this paper, cubic polynomial spline based functions are used for the approximate solutions of fractional boundary value problems (FBVPs). Left and right sided Caputo’s fractional approaches are used for the fractional derivative. Convergence analysis of this method is also presented. Numerical examples are given to illustrate the accuracy and efficiency of this method and comparison show that this scheme is more accurate than the existing method (Rehman and Khan in Appl Math Model 36:894–907, 2012).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

References

  1. Diethelm, K., Scalas, E., Trujillo, J.J.: Fractional Calculus Models and Numerical Methods. World Scientific Publishing, New York (2012)

    MATH  Google Scholar 

  2. Diethelm, K., Ford, N.J., Freed, A.D.: Detailed error analysis for a fractional adams method. Numerical Algorithms 36, 31–52 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  3. Diethelm, K.: The Analysis of Fractional Differential Equations, Lecture Notes in Mathematics (2010)

  4. Tarasov, V.E.: Fractional Dynamics: Application of Fractional Calculus to Dynamics of particles, Fields and Media, Springer. Heidelberg; Higher Education Press, Bei**g (2010)

    Book  MATH  Google Scholar 

  5. Su, X.: Solutions to boundary value problem of fractional order on unbounded domains in a Banach space. Nonlinear Anal. 74, 2844–2852 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  6. Torvik, P.J., Bagley, R.L.: On the appearance of the fractional derivative in the behavior of real materials. J. Appl. Mech. 51, 294–298 (1984)

    Article  MATH  Google Scholar 

  7. Marks II, R.J., Hall, M.W.: Differintegral interpolation from a bandlimited signals samples. IEEE Trans. Acoust. Speech Signal Process. 29, 872–877 (1981)

    Article  MATH  Google Scholar 

  8. Olmstead, W.E., Handelsman, R.A.: Diffusion in a semi-infinite region with nonlinear surface dissipation. SIAM Rev. 18, 275–291 (1976)

    Article  MathSciNet  MATH  Google Scholar 

  9. Scalas, E., Gorenflo, R., Mainardi, F.: Fractional calculus and continuous-time finance. Phys. A 284, 376–384 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  10. Ahmad, B., Nieto, J.J.: Existence of solutions for nonlocal boundary value problems of higher-order nonlinear fractional differential equations. Abstr. Appl. Anal. 2009, Article ID 494720, p. 9. doi:10.1155/2009/494720

  11. Liang, S., Zhang, J.: Existence of multiple positive solutions form-point fractional boundary value problems on an infinite interval. Math. Comput. Model. 54, 1334–1346 (2011)

    Article  MATH  Google Scholar 

  12. Bai, Z.B., Sun, W.: Existence and multiplicity of positive solutions for singular fractional boundary value problems Comput. Math. Appl. 63, 1369–1381 (2012)

    MathSciNet  MATH  Google Scholar 

  13. Shuqin, Z.: Existence of solution for boundary value problem of fractional order. Acta Math. Sci. 26, 220–228 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  14. Akram, G., Tariq, H.: An Exponential Spline Technique for Solving Fractional Boundary Value Problem, Calcolo. doi:10.1007/s10092-015-0161-0

  15. Siddiqi, S.S., Akram, G.: Solution of eighth-order boundary value problems using the non-polynomial spline technique. Int. J. Comput. Math. 84(3), 347–368 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  16. Siddiqi, S.S., Akram, G.: Solution of tenth-order boundary value problems using eleventh degree spline. Appl. Math. Comput. 185, 115–127 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  17. Kilbas, A.A., Srivastava, H.M., Trujillo, J.J.: Theory of Application of Fractional Differential Equations, 1st edn. Belarus (2006)

  18. Podlubny, I.: Fractional Differential Equation. Academic, San Diego (1999)

    MATH  Google Scholar 

  19. Lakshmikantham, V., Vatsala, A.S.: Basic theory of fractional differential equations. Nonlinear Anal. 69, 2677–2682 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  20. Rehman, M.U., Khan, R.A.: A numerical method for solving boundary value problems for fractional differential equations. Appl. Math. Model. 36, 894–907 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  21. Usmani, R.A.: Discrete variable methods for a boundary value problem with engineering applications. Math. Comput. 32, 1087–1096 (1978)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, University of the Punjab, Quaid-e-Azam Campus, Lahore, 54590, Pakistan

    Ghazala Akram & Hira Tariq

Authors
  1. Ghazala Akram
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hira Tariq
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ghazala Akram.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Akram, G., Tariq, H. Cubic Polynomial Spline Scheme for Fractional Boundary Value Problems with Left and Right Fractional Operators. Int. J. Appl. Comput. Math 3, 937–946 (2017). https://doi.org/10.1007/s40819-016-0145-z

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40819-016-0145-z

Keywords

Search

Navigation