Effective Behavior Near Clogging in Upscaled Equations for Non-isothermal Reactive Porous Media Flow

Abstract

For a non-isothermal reactive flow process, effective properties such as permeability and heat conductivity change as the underlying pore structure evolves. We investigate changes of the effective properties for a two-dimensional periodic porous medium as the grain geometry changes. We consider specific grain shapes and study the evolution by solving the cell problems numerically for an upscaled model derived in Bringedal et al. (Transp Porous Media 114(2):371–393, 2016. doi:10.1007/s11242-015-0530-9). In particular, we focus on the limit behavior near clogging. The effective heat conductivities are compared to common porosity-weighted volume averaging approximations, and we find that geometric averages perform better than arithmetic and harmonic for isotropic media, while the optimal choice for anisotropic media depends on the degree and direction of the anisotropy. An approximate analytical expression is found to perform well for the isotropic effective heat conductivity. The permeability is compared to some commonly used approaches focusing on the limiting behavior near clogging, where a fitted power law is found to behave reasonably well. The resulting macroscale equations are tested on a case where the geochemical reactions cause pore clogging and a corresponding change in the flow and transport behavior at Darcy scale. As pores clog the flow paths shift away, while heat conduction increases in regions with lower porosity.

Appendix

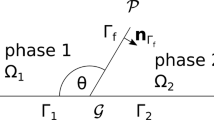
Section 4.1 deals with analytical solutions of an approximate heat conductive cell problem, and we here give the derivation of these solutions. We seek the unknown functions $\Theta _f(y_1,y_1)$ and $\Theta _g(y_1,y_2)$ that should fulfill Eq. (3b) together with periodicity across the external cell boundary:

$$\begin{aligned} \Theta _f(y_1=-1/2) = \Theta _f(y_1=1/2),\ \Theta _f(y_2=-1/2) = \Theta _f(y_2=1/2). \end{aligned}$$

We use polar coordinates and assume separation of variables; hence, the solutions can be written

$$\begin{aligned} \Theta _f(r,\theta ) = F_r(r)F_{\theta }(\theta ),\ \Theta _g(r,\theta ) = G_r(r)G_{\theta }(\theta ). \end{aligned}$$

Then, the model equations from (3b) are

$$\begin{aligned} \frac{1}{r}\frac{\mathrm{d}}{\mathrm{d}r}\left( r\frac{\mathrm{d}F_r}{\mathrm{d}r}\right) F_{\theta }+\frac{1}{r^2}\frac{\mathrm{d}^2F_{\theta }}{\mathrm{d}\theta ^2}F_r&=0,\ y\in Y_0(x,t); \\ \frac{1}{r}\frac{\mathrm{d}}{\mathrm{d}r}\left( r\frac{\mathrm{d}G_r}{\mathrm{d}r}\right) G_{\theta }+\frac{1}{r^2}\frac{\mathrm{d}^2G_{\theta }}{\mathrm{d}\theta ^2}G_r&=0,\ y\in G_0(x,t), \end{aligned}$$

while the interior boundary conditions can be written as

$$\begin{aligned}&\displaystyle \cos \theta +F_r'(R)F_{\theta }=\kappa \cos \theta +\kappa G_r'(R)G_{\theta } \text { at } r=R,&\end{aligned}$$

(8a)

$$\begin{aligned}&\displaystyle F_r(R)F_{\theta }=G_r(R)G_{\theta } \text { at } r=R,&\end{aligned}$$

(8b)

and for all $\theta$. The model equations for $F_{\theta }$ and $G_{\theta }$ reduce to $F_{\theta }'' = -\lambda F_{\theta }$ for some number $\lambda$, which, together with the interior boundary condition (8a), suggest that

$$\begin{aligned} F_{\theta }(\theta )=G_{\theta }(\theta ) = \cos \theta . \end{aligned}$$

The model equations for $F_r$ and $G_r$ are then reduced to $r^2F''_r+rF'_r-F_r=0,$ which have the general solutions

$$\begin{aligned} F_r(r) = b_1r+b_2\frac{1}{r},\ G_r(r) = b_3r+b_4\frac{1}{r}, \end{aligned}$$

where $b_1, b_2, b_3, b_4$ are integration constants. However, our assumption of separation of variables together with the solution in $\theta$ leads to the periodicity requirement on the external boundary not being fulfilled. There is periodicity across the horizontal boundaries, but periodicity across the vertical boundaries is not met. We instead search an approximate solution through alternative boundary conditions for the external boundary and consider two approaches: either drop** the external boundary and allowing $\Theta _f$ to be well defined for all $r>R$; or, kee** the boundary, but neglecting the boundary conditions and using other means to determine the constants $b_1,b_2,b_3,b_4$.

1.1 Alternative I: Infinite Domain

We allow $\Theta _f$ to be defined for all $r>R$; hence, we require it to be finite as $r\rightarrow \infty$. Also, as $\Theta _g$ must be well defined as $r\rightarrow 0$, the general solutions in r are

$$\begin{aligned} F_r(r) = b_2\frac{1}{r},\ G_r(r) = b_3r. \end{aligned}$$

Applying the internal boundary conditions (8) results in $b_2=R^2(1-\kappa )/(1+\kappa )$ and $b_3=(1-\kappa )/(1+\kappa )$. Hence, the solutions of the approximate cell problem are

$$\begin{aligned} \Theta _f(r,\theta )&= R^2\frac{1-\kappa }{1+\kappa }\frac{1}{r}\cos \theta , \\ \Theta _g(r,\theta )&= \frac{1-\kappa }{1+\kappa }r\cos \theta . \end{aligned}$$

The approximate heat conductivity coefficients are then

$$\begin{aligned} {\mathcal {A}}_f&=\int _{Y_0(x,t)}(1+\partial _x\Theta _f)\mathrm{d}y=1-\pi R^2, \\ {\mathcal {A}}_g&=\int _{G_0(x,t)}(1+\partial _x\Theta _g)\mathrm{d}y = \pi R^2\frac{2}{1+\kappa }. \end{aligned}$$

Although $\Theta _f$ is now defined for all $r>R$, the original integration area for ${\mathcal {A}}_f$ is used. As we made an error by allowing $\Theta _f$ to exist as $r\rightarrow \infty$, and as we know the sum of ${\mathcal {A}}_f$ and ${\mathcal {A}}_g$ should be 1 when in the finite pore space domain, our above approximation can be improved by scaling the above coefficients with the same number such that their sum is 1. Hence,

$$\begin{aligned} {\mathcal {A}}_f&=(1-\pi R^2)\frac{1+\kappa }{(1+\kappa )(1-\pi R^2)+2\pi R^2}, \end{aligned}$$

(9a)

$$\begin{aligned} {\mathcal {A}}_g&= \pi R^2\frac{2}{(1+\kappa )(1-\pi R^2)+2\pi R^2}. \end{aligned}$$

(9b)

1.2 Alternative II: No Periodic Boundary Condition

In this approach, we keep the external boundary but do not require periodicity across it. We still require $\Theta _g$ to be well defined as $r\rightarrow 0$, and hence $b_4=0$ and the three remaining constants must be such that they fulfill the two internal boundary conditions (8):

$$\begin{aligned} 1+b_1-\frac{b_2}{R^2}&=\kappa +\kappa b_3,\\ 1+b_1R+\frac{b_2}{R}&= b_3R. \end{aligned}$$

Expressing $b_2,b_3$ as functions of $b_1$ leads to

$$\begin{aligned} b_2&= R^2\frac{1-\kappa }{1+\kappa }(1+b_1),\\ b_3&= \frac{1-\kappa }{1+\kappa } + \frac{2b_1}{1+\kappa }. \end{aligned}$$

The solutions of the (approximate) cell problem are then

$$\begin{aligned} \Theta _f(r,\theta )&= \left( b_1+\frac{R^2}{r^2}\frac{1-\kappa }{1+\kappa }(1+b_1)\right) r\cos \theta ,\\ \Theta _g(r,\theta )&= \left( \frac{1-\kappa }{1+\kappa }+\frac{2b_1}{1+\kappa }\right) r\cos \theta , \end{aligned}$$

where the constant $b_1$ is to be determined later. The heat conductivities are then

$$\begin{aligned} {\mathcal {A}}_f&=\int _{Y_0(x,t)}(1+\partial _x\Theta _f)\mathrm{d}y= (1-\pi R^2)(1+b_1),\\ {\mathcal {A}}_g&=\int _{G_0(x,t)}(1+\partial _x\Theta _g)\mathrm{d}y= \pi R^2\frac{2(1+b_1)}{1+\kappa }. \end{aligned}$$

We now require the sum ${\mathcal {A}}_f + {\mathcal {A}}_g$ to be 1 and use this to determine $b_1$. This way,

$$\begin{aligned} {\mathcal {A}}_f&= (1-\pi R^2)\frac{1+\kappa }{1+\kappa + \pi R^2(1-\kappa )},\end{aligned}$$

(10a)

$$\begin{aligned} {\mathcal {A}}_g&= \pi R^2\frac{2}{1+\kappa +\pi R^2(1-\kappa )}. \end{aligned}$$

(10b)

Although this derivation uses different assumptions than Alternative I, the resulting approximate heat conductivity coefficients ${\mathcal {A}}_f$ and ${\mathcal {A}}_g$ found in (9) and (10) are identical.