SpringerLink
Log in

Fractional centered difference schemes and banded preconditioners for nonlinear Riesz space variable-order fractional diffusion equations

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

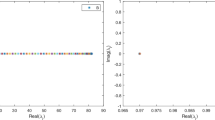
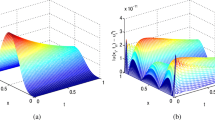
Abstract

In this paper, high-order finite difference methods are proposed to solve the initial-boundary value problem for one- and two-dimensional Riesz space variable-order fractional diffusion equations. We first introduce fractional centered difference (FCD) and weighted and shifted fractional centered difference (WSFCD) schemes for Riesz space variable-order fractional derivatives. Then the Crank-Nicolson (CN) scheme and the linearly implicit conservative (LIC) difference scheme are applied to discretize the time derivative in linear and nonlinear problems, respectively. Thus, we get CN-FCD and CN-WSFCD schemes, and LIC-FCD and LIC-WSFCD schemes, respectively. Theoretical results about the stability and convergence for the above-mentioned schemes are presented and proved. Banded preconditioners are introduced to speed up GMRES methods for solving the discretization linear systems. The spectral property of the preconditioned matrix is analyzed. Numerical results show that the proposed schemes and preconditioners are very efficient.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Data availability

Data sharing not applicable to this article as no datasets were generated or analyzed during the current study.

References

  1. Abirami, A., Prakash, P., Ma, Y.K.: Variable-order fractional diffusion model-based medical image denoising. Math. Probl. Eng. 2021, 1–10 (2021)

    Article  Google Scholar 

  2. Axelsson, O., Kolotilina, L.: Montonicity and discretization error eatimates. SIAM J. Numer. Anal. 27, 1591–1611 (1990)

    Article  MathSciNet  Google Scholar 

  3. Bai, J., Feng, X.C.: Fractional-order anisotropic diffusion for image denoising. IEEE Trans. Image Proc. 16, 2492–2502 (2007)

    Article  MathSciNet  Google Scholar 

  4. Benson, D.A., Wheatcraft, S.W., Meerschaert, M.M.: Application of a fractional advection-dispersion equation. Water Resour. Res. 36, 1403–1412 (2000)

    Article  Google Scholar 

  5. Benson, D.A., Wheatcraft, S.W., Meerschaert, M.M.: The fractional-order governing equation of Lévy motion. Water Resour. Res. 36, 1413–1423 (2000)

    Article  Google Scholar 

  6. Çelik, C., Duman, M.: Crank-Nicolson method for the fractional diffusion equation with the Riesz fractional derivative. J. Comput. Phys. 231, 1743–1750 (2012)

    Article  MathSciNet  Google Scholar 

  7. Ding, H.F., Li, C.P., Chen, Y.Q.: High-order algorithms for Riesz derivative and their applications (I). Abstr. Appl. Anal. 2014, 653797 (2014). https://doi.org/10.1155/2014/653797

    Article  MathSciNet  Google Scholar 

  8. Donatelli, M., Mazza, M., Serra-Capizzano, S.: Spectral analysis and structure preserving preconditioners for fractional diffusion equations. J. Comput. Phys. 307, 262–279 (2016)

    Article  MathSciNet  Google Scholar 

  9. Du, R., Alikhanov, A.A., Sun, Z.Z.: Temporal second order difference schemes for the multi-dimensional variable-order time fractional sub-diffusion equations. Comput. Math. Appl. 79, 2952–2972 (2020)

    Article  MathSciNet  Google Scholar 

  10. Feller, W.: On a generalization of Marcel Riesz’ potentials and the semi-groups generated by them. Medd. Lunds Univ. Mat. Sem. 1952, 72–81 (1952)

    MathSciNet  Google Scholar 

  11. Garrappa, R., Giusti, A., Mainardi, F.: Variable-order fractional calculus: a change of perspective. Commun. Nonlinear Sci. Numer. Simul. 102, 105904 (2021). https://doi.org/10.1016/j.cnsns.2021.105904

    Article  MathSciNet  Google Scholar 

  12. Gu, X.M., Zhao, Y.L., Zhao, X.L., Carpentieri, B., Huang, Y.Y.: A note on parallel preconditioning for the all-at-once solution of Riesz fractional diffusion equations. Numer. Math. Theor. Meth. Appl. 14, 893–919 (2021)

    Article  MathSciNet  Google Scholar 

  13. Guo, L., Zhao, X.L., Gu, X.M., Zhao, Y.L., Zheng, Y.B., Huang, T.Z.: Three-dimensional fractional total variation regularized tensor optimized model for image deblurring. Appl. Math. Comput. 404, 126224 (2021). https://doi.org/10.1016/j.amc.2021.126224

    Article  MathSciNet  Google Scholar 

  14. Henry, B.I., Wearne, S.L.: Fractional reaction-diffusion. Physica A 276, 448–455 (2000)

    Article  MathSciNet  Google Scholar 

  15. Horn, R.A., Johnson, C.R.: Toptics in Matrix Analysis. Academic Press, Cambridge (1994)

    Google Scholar 

  16. Lin, F.R., Liu, W.D.: The accuracy and stability of CN-WSGD schemes for space fractional diffusion equation. J. Comput. Appl. Math. 363, 7–91 (2020)

    Article  MathSciNet  Google Scholar 

  17. Lin, R., Liu, F., Anh, V., Turner, I.: Stability and convergence of a new explicit finite-difference approximation for the variable-order nonlinear fractional diffusion equation. Appl. Math. Comput. 212, 435–445 (2009)

    MathSciNet  Google Scholar 

  18. Lin, F.R., Qiu, Y.F., She, Z.H.: IRK-WSGD methods for space fractional diffusion equations. Appl. Numer. Math. 164, 222–244 (2021)

    Article  MathSciNet  Google Scholar 

  19. Lin, F.R., Qu, H.D., She, Z.H.: DNT preconditioner for one-sided space fractional diffusion equations. BIT 61, 1311–1335 (2021)

    Article  MathSciNet  Google Scholar 

  20. Lin, F.R., Wang, Q.Y., **, X.Q.: Crank-Nicolson-weighted-shifted-Grünwald difference schemes for space Riesz variable-order fractional diffusion equations. Numer. Algor. 87, 601–631 (2021)

    Article  Google Scholar 

  21. Lin, F.R., Yang, S.W., **, X.Q.: Preconditioned iterative methods for fractional diffusion equation. J. Comput. Phys. 256, 109–117 (2014)

    Article  MathSciNet  Google Scholar 

  22. Lorenzo, C.F., Hartley, T.T.: Variable-order and distributed order fractional operators. Nonlinear Dyn. 29, 57–98 (2002)

    Article  MathSciNet  Google Scholar 

  23. Miller, K.S., Ross, B.: An Introduction to the Fractional Calculus and Fractional Differential Equations. John Wiley, New York (1993)

    Google Scholar 

  24. Oldham, K.B., Spanier, J.: The Fractional Calculus. Academic Press, New York (1974)

    Google Scholar 

  25. Ortigueira, M.D.: Riesz potential operators and inverses via fractional centred derivatives. Int. J. Math. Math. Sci. 2006, 1–12 (2006)

    Article  MathSciNet  Google Scholar 

  26. Pang, H.K., Sun, H.W.: A fast algorithm for the variable-order spatial fractional advection-diffusion equation. J. Sci. Comput. 87, 15 (2021). https://doi.org/10.1007/s10915-021-01427-w

    Article  MathSciNet  Google Scholar 

  27. Podlubny, I.: Fractional Differential Equations. Cambridge University Press, New York (1999)

    Google Scholar 

  28. Ruiz-Medina, M.D., Anh, V., Angulo, J.M.: Fractional generalized random fields of variable order. Stochastic Anal. Appl. 22, 775–799 (2004)

    Article  MathSciNet  Google Scholar 

  29. Samko, S.G., Kilbas, A.A., Marichev, O.I.: Fractional Integerals and Derivatives: Theory and Applications. Gordon and Breach Science Publishers, Amsterdam (1993)

    Google Scholar 

  30. Samko, S.G., Ross, B.: Integration and differentiation to a variable fractional order. Integral Transform Spec. Funct. 1, 277–300 (1993)

    Article  MathSciNet  Google Scholar 

  31. Seki, K., Wojcik, M., Tachiya, M.: Fractional reaction-diffusion equation. J. Chem. Phys. 119, 2165–2174 (2003)

    Article  Google Scholar 

  32. She, Z.H.: A class of unconditioned stable 4-point WSGD schemes and fast iteration methods for space fractional diffusion equations. J. Sci. Comput. 92, 18 (2022). https://doi.org/10.1007/s10915-022-01860-5

  33. She, Z.H., Lao, C.X., Yang, H., Lin, F.R.: Banded preconditioners for Riesz space fractional diffusion equations. J. Sci. Comput. 86, 31 (2021). https://doi.org/10.1007/s10915-020-01398-4

    Article  MathSciNet  Google Scholar 

  34. She, Z.H., Qu, H.D., Liu, H.: Stability and convergence of finite difference method for two-sided space-fractional diffusion equations. Comput. Math. Appl. 89, 78–86 (2021)

    Article  MathSciNet  Google Scholar 

  35. Sokolov, I.M., Klafter, J., Blumen, A.: Fractional kinetics. Phys. Today 55, 48–54 (2002)

    Article  Google Scholar 

  36. Tian, W., Zhou, H., Deng, W.: A class of second order difference approximations for solving space fractional diffusion equations. Math. Comput. 84, 1703–1727 (2015)

    Article  MathSciNet  Google Scholar 

  37. Wang, D.L., **ao, A.G., Yang, W.: Maximum-norm error analysis of a difference scheme for the space fractional CNLS. Appl. Math. Comput. 257, 241–251 (2015)

    MathSciNet  Google Scholar 

  38. Zeng, F.H., Zhang, Z.Q., Karniadakis, G.E.: A generalized spectral collocation method with tunable accuracy for variable-order fractional differential equations. SIAM J. Sci. Comput. 37, A2710–A2732 (2015)

    Article  MathSciNet  Google Scholar 

  39. Zhao, X., Sun, Z.Z., Karniadakis, G.E.: Second-order approximations for variable order fractional derivatives: Algorithms and applications. J. Comput. Phys. 293, 184–200 (2015)

    Article  MathSciNet  Google Scholar 

  40. Zhao, Y.L., Zhu, P.Y., Gu, X.M., Zhao, X.L., Jian, H.Y.: A preconditioning technique for all-at-once system from the nonlinear tempered fractional diffusion equation. J. Sci. Comput. 83, 10 (2020). https://doi.org/10.1007/s10915-020-01193-1

    Article  MathSciNet  Google Scholar 

  41. Zhuang, P., Liu, F., Anh, V., Turner, I.: Numerical methods for the variable-order fractional advection-diffusion equation with a nonlinear source term. SIAM J. Numer. Anal. 47, 1760–1781 (2009)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

We thank the referees for providing detailed and valuable comments and suggestions, which are very helpful for improving our paper.

Funding

This research was supported by National Natural Science Foundation of China (11771265), basic research and applied basic research projects in Guangdong Province (Projects of Guangdong-Hong Kong-Macao Center for Applied Mathematics) (2020B1515310018) and key research projects of general universities in Guangdong Province (2019KZDXM034).

Author information

Authors and Affiliations

  1. Department of Mathematics, Shantou University, Shantou, 515063, Guangdong, People’s Republic of China

    Qiu-Ya Wang, Zi-Hang She, Cheng-Xue Lao & Fu-Rong Lin

Authors
  1. Qiu-Ya Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zi-Hang She
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Cheng-Xue Lao
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Fu-Rong Lin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Fu-Rong Lin.

Ethics declarations

Conflict of interest

The authors declare no competing interests.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, QY., She, ZH., Lao, CX. et al. Fractional centered difference schemes and banded preconditioners for nonlinear Riesz space variable-order fractional diffusion equations. Numer Algor 95, 859–895 (2024). https://doi.org/10.1007/s11075-023-01592-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-023-01592-z

Keywords

Mathematics Subject Classification (2010)

Search

Navigation