Magnetic black holes in Weitzenböck geometry

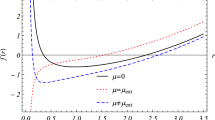
Abstract

We derive magnetic black hole solutions using a general gauge potential in the framework of teleparallel equivalent general relativity. One of the solutions gives a non-trivial value of the scalar torsion. This non-triviality of the torsion scalar depends on some values of the magnetic field. The metric of those solutions behave asymptotically as anti-de-Sitter/de-Sitter spacetimes. The energy conditions are discussed in details. Also, we calculate the torsion and curvature invariants to discuss singularities. Additionally, we calculate the conserved quantities using the Einstein–Cartan geometry to understand the physics of the constants appearing into the solutions.

Appendices

Appendix A: Notation used in the calculations of conserved currents

The indices ${ i, j, \ldots }$ are used for the (co)frame components while $\alpha$, $\beta , \ldots$ label the local holonomic spacetime coordinates. Exterior product is defined as $\wedge$, while the interior is denoted by $\xi \rfloor \Psi$. The vector basis, dual to the 1-forms $\vartheta ^{i}$, is denoted by $e_i$. They satisfy the condition $e_i \rfloor \vartheta ^{j}={\delta _i}^j$. Using the local coordinates $x^\mu$, we have $\vartheta ^{i}={b^i}_\mu dx^\mu$ and $e_i={b_i}^\mu \partial _\mu$ where ${b^i}_\mu$ and ${b_i}^\mu$ are the covariant and contravariant components of the tetrad field. The volume is defined as $\eta :=\vartheta ^{\hat{0}}\wedge \vartheta ^{\hat{1}} \wedge \vartheta ^{\hat{2}}\wedge \vartheta ^{\hat{3}}$ which is a 4-form. Moreover, by using the interior product one can define

$$\begin{aligned} \eta _i:=e_i \rfloor \eta = \ \frac{1}{3!} \ \epsilon _{i j k l} \ \vartheta ^j \wedge \vartheta ^k \wedge \vartheta ^l, \end{aligned}$$

where $\epsilon _{ i j k l}$ is totally antisymmetric with $\epsilon _{0123}=1$.

$$\begin{aligned} \eta _{ i j}:=e_j \rfloor \eta _i = \frac{1}{2!}\epsilon _{i j k l} \ \vartheta ^k \wedge \vartheta ^l,\quad \eta _{i j k}:=e_k \rfloor \eta _{i j}= \frac{1}{1!} \epsilon _{i j k l} \ \vartheta ^l, \end{aligned}$$

that are the bases for 3-, 2- and 1-forms respectively. Finally,

$$\begin{aligned} \eta _{i j k l} :=e_l \rfloor \eta _{i j k}= e_l \rfloor e_k \rfloor e_j \rfloor e_i \rfloor \eta , \end{aligned}$$

is the Levi-Civita tensor density. The $\eta$-forms satisfy the useful identities:

$$\begin{aligned} \vartheta ^i \wedge \eta _j&:= \delta ^i_j \eta , \quad \vartheta ^i \wedge \eta _{j k} := \delta ^i_k \eta _j-\delta ^i_j \eta _k,\nonumber \\ \vartheta ^i \wedge \eta _{j k l}&:= \delta ^i_j \eta _{k l} +\delta ^i_k \eta _{l j}+\delta ^i_l \eta _{ j k}, \nonumber \\ \vartheta ^i \wedge \eta _{j k l n}&:= \delta ^i_n \eta _{j k l}-\delta ^i_l \eta _{j k n }+\delta ^i_k \eta _{ j l n}-\delta ^i_j \eta _{k l n}. \end{aligned}$$

(48)

Appendix B: Calculations of the Weyl and $W^{\mu \nu }$ tensors

The non-vanishing components of Weyl tensor, using solutions (16), have the form:

$$\begin{aligned} C_{0101}= & {} -\,C_{0110} =C_{1010}=-C_{1001}=2C_{0220}=-2C_{0202}=2C_{0330} =-2C_{0303}\nonumber \\= & {} -\,2C_{2020}=2C_{2002} =2C_{3003}=-2C_{3030}= 2C_{1212}=-2C_{1221}=2C_{1313}\nonumber \\= & {} - \,2C_{1331}=-2C_{2112}=2C_{2121}=-2C_{3113}=2C_{3131}= -C_{2323}=C_{2332}\nonumber \\= & {} -\,C_{3232}=C_{3223}=-\frac{c_1}{r^3}, \end{aligned}$$

(49)

and the non-vanishing components of the tensor $W^{\mu \nu }$ take the form

$$\begin{aligned} W^{01}= & {} \frac{c_1}{r^3}(dt \wedge dr),\quad W^{02} =\frac{\sqrt{3c_1-\Lambda r^3}}{2\sqrt{3r^5}}(d\phi \wedge dt),\nonumber \\ W^{03}= & {} \frac{\sqrt{3c_1-\Lambda r^3}}{2\sqrt{3r^5}}(dz \wedge dt), \quad W^{12}=\frac{3c_1}{2\sqrt{3r^3(3c_1-\Lambda r^3)}}(d\phi \wedge dr),\nonumber \\ W^{13}= & {} \frac{3c_1}{2\sqrt{3r^3(3c_1-\Lambda r^3)}}(dr \wedge dz), \quad W^{23}=\frac{c_1(d\phi \wedge dz)}{r}. \end{aligned}$$

(50)