Effective behavior of viscoelastic composites: comparison of Laplace–Carson and time-domain mean-field approach

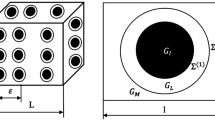
Abstract

The paper focuses on deriving the macroscale viscoelastic constitutive laws using asymptotic expansion method. Both the differential and integral form of the linear viscoelastic constitutive relation of the phases is used in deriving the effective incremental potential and effective constitutive relation, respectively. The integral form is handled by considering the correspondence principle and the Laplace–Carson (LC) transform. A closed-form expression for the effective viscoelastic properties in LC domain is obtained by means of the asymptotic homogenization method (AHM). In addition, AHM coupled with finite element simulation of a representative volume element with periodic boundary conditions is used (AHM + FE). The last step in both approaches is the numerical inversion to the time domain. Solution in time domain is obtained with numerical Laplace inversion algorithms. In case of the differential form, using variational approach, the effective incremental potential in time domain is directly obtained using mean-field method. Different homogenization approaches are exemplified for evaluation of the effective relaxation behavior of composite (viscoelastic matrix reinforced by unidirectional elastic fibers), and they are compared. In the approaches based on LC transform, effective modulus and Poisson’s ratio agree well with each other for any property contrast and fiber volume fraction. However, in case of relatively low property contrast, mean field overpredicts as compared to LC approaches in the fiber direction, whereas at relatively higher property contrast, it is vice versa. The difference increases at higher volume fractions due to synergistic effect of the error due to geometrical assumptions involved in the localization tensor and interaction effects of the fiber inclusions. A good agreement in all directions is observed among the three schemes at intermediate volume fractions and property contrast. This study serves as benchmark for further theoretical improvements and experimental investigations.

Appendices

Appendix

Implementation of IVMFH approach

Figure 

Fig. 11

Flowchart for implementation of the incremental variational-based MFH to compute Σ at current time step t

11 shows the flowchart of the incremental approach to compute the effective response of the composite as described in Sect. 4.2. The core aspect of the algorithm lies in finding the unknown pair of quantities $\left( {\theta^{{\gamma_{\alpha } }} ,\,\;{\varvec{\varepsilon}}_{{\text{ov n}}}^{{\gamma_{\alpha } }} } \right)$ for all N—Maxwell branches using Eq. (24). These are assembled in a column vector X for γ-phase. This vector of unknowns is given as an input to a function specified in the form F(X) = 0 (Fig. 11), which is given as

$${\mathbf{F}}\underbrace {{\left( \begin{gathered} \theta^{{\gamma_{\alpha = 1} }} \\ \vdots \\ \theta^{{\gamma_{\alpha = N} }} \\ {\varvec{\varepsilon}^{\prime}}_{{\text{ov n}}}^{{\gamma_{\alpha = 1} }} \\ \vdots \\ {\varvec{\varepsilon}^{\prime}}_{{{\text{ov }}n}}^{{\gamma_{\alpha = N} }} \\ \end{gathered} \right)}}_{{\left( {\varvec{X}} \right)}} = \left( {\begin{array}{*{20}c} {1 - \sqrt {\frac{{\left\langle {{\varvec{\varepsilon}^{\prime}}_{{\text{v n}}} \cdot {\varvec{\varepsilon}^{\prime}}_{{\text{v n}}} } \right\rangle^{{\gamma_{\alpha } }} - 2 \cdot {\varvec{\varepsilon}^{\prime}}_{{\text{ov n}}}^{{\gamma_{\alpha } }} \cdot \left\langle {{\varvec{\varepsilon}^{\prime}}_{{\text{v n}}} } \right\rangle^{{\gamma_{\alpha } }} + {\varvec{\varepsilon}^{\prime}}_{{\text{ov n}}}^{{\gamma_{\alpha } }} \cdot {\varvec{\varepsilon}^{\prime}}_{{\text{ov n}}}^{{\gamma_{\alpha } }} }}{{\left\langle {{\varvec{\varepsilon}^{\prime}}_{{\text{v}}} \cdot {\varvec{\varepsilon}^{\prime}}_{{\text{v}}} } \right\rangle^{{\gamma_{\alpha } }} - 2 \cdot {\varvec{\varepsilon}^{\prime}}_{{\text{ov n}}}^{{\gamma_{\alpha } }} \cdot \left\langle {{\varvec{\varepsilon}^{\prime}}_{{\text{v}}} } \right\rangle^{{\gamma_{\alpha } }} + {\varvec{\varepsilon}^{\prime}}_{{\text{ov n}}}^{{\gamma_{\alpha } }} \cdot {\varvec{\varepsilon}^{\prime}}_{{\text{ov n}}}^{{\gamma_{\alpha } }} }}} - \,\theta^{{\gamma_{\alpha } }} } \\ {\frac{1}{{\theta^{{\gamma_{\alpha } }} }}\left\langle {{\varvec{{\varepsilon}}^{\prime}}_{{\text{v n}}} } \right\rangle^{{\gamma_{\alpha } }} + \left( {1 - \frac{1}{{\theta^{{\gamma_{\alpha } }} }}} \right)\left\langle {{\varvec{\varepsilon}^{\prime}}_{{\text{v}}} } \right\rangle^{{\gamma_{\alpha } }} - \;{\varvec{\varepsilon}^{\prime}}_{{\text{ov n}}}^{{\gamma_{\alpha } }} } \\ \end{array} } \right) = \underbrace {{\left( \begin{gathered} 0 \hfill \\ \vdots \hfill \\ 0 \hfill \\ \varvec{\it{0}} \hfill \\ \vdots \hfill \\ {\varvec{\it{0}}} \hfill \\ \end{gathered} \right)}}_{{\left( {\mathbf{0}} \right)}}$$

(A.1)

The function is assembled with the pair of unknowns for all N-Maxwell branches using expanded form of Eq. (24). The flowchart also indicates the dependencies of the quantities involved in the computations. The steps for determining the effective response of the composite are summarized as follows:

The first and second moments of the internal strain ($\left\langle {\varvec{\varepsilon}^{\prime}}_{{\text{v}}} \right\rangle^{\gamma }$ and $\left\langle {\varvec{\varepsilon}^{\prime}_{{\text{v}}} \cdot \varvec{\varepsilon}^{\prime}}_{{\text{v}}} \right\rangle^{\gamma }$, respectively) at past time t_n are known for γ-phase. Here, the initial conditions are assumed that $\left\langle {\varvec{\varepsilon}^{\prime}}_{{\text{v}}} \right\rangle^{\gamma } = \it {\varvec{0}}$ and $\left\langle {\varvec{\varepsilon}^{\prime}_{{\text{v}}} \cdot \varvec{\varepsilon}^{\prime}}_{{\text{v}}} \right\rangle^{\gamma } = 0$.

The nonlinear set of equations involved in F(X) is solved for all Maxwell branches. The unknown vector X at t is initialized at ith iteration to the solution vector X_n available at t_n to achieve a faster convergence. However, at t = 0 it is assumed that the shear viscosity in the actual problem and virtual thermoelastic problem is same, and the effective internal variable in the virtual problem is zero. Hence, the pair of unknowns for α-Maxwell element in γ-phase is initialized as $\theta^{{\gamma_{\alpha } }} = 1,\,\;{\varvec{\varepsilon}}_{{{\text{ov }}n}}^{{\gamma_{\alpha } }} = \it \varvec{0}$. Based on this, all the thermoelastic constants for the virtual thermoelastic problem are evaluated using Eq. (27) before entering a Jacobian-free optimization loop:

The first and second moments of the total strain ($\left\langle {\varvec{\varepsilon}} \right\rangle^{\gamma }$ and $\left\langle {{\varvec{\varepsilon}^{\prime}} \cdot {\varvec{\varepsilon}^{\prime}}} \right\rangle^{\gamma } ,$ respectively) in the virtual thermoelastic problem are evaluated using an appropriate elastic MFH scheme such as HSLB or DI method for the considered composite microstructure. These moments depend on the thermoelastic constants which in turn depend on unknown vector X.

Based on step 2.1, the first and second moment of the internal strain for the α-Maxwell element in γ-phase ($\left\langle {\varvec{\varepsilon}^{\prime}_{{\text{v}}} } \right\rangle^{{\gamma_{\alpha } }}$ and $\left\langle {\varvec{\varepsilon}^{\prime}_{{\text{v}}} \cdot \varvec{\varepsilon}^{\prime}}_{{\text{v}}} \right\rangle^{{\gamma_{\alpha } }}$, respectively) is evaluated using local field solution in Eq. (25). These quantities also depend on unknown vector X via $\left\langle {\varvec{\varepsilon}} \right\rangle^{\gamma }$ and $\left\langle {\varvec{\varepsilon}^{\prime} \cdot \varvec{\varepsilon}^{\prime}} \right\rangle^{\gamma }$.

The residual for F(X) is computed. If the residual vector is approximately zero, store the first and second moments of the internal strain and go to step 3, else return to step 2.1. The Jacobian of the F(X) is approximated numerically using finite difference.

The macroscopic stress Σ at t can be obtained by the using the converged solution vector X obtained at i + 1 iteration of the optimization loop via Eqs. (25) and (29) (Fig. 11). If t ≤ T, return to step 1 else the time loop stops.

This scheme is implemented in MATLAB using trust-region-dogleg algorithm available in fsolve function. All the tensor calculations are done in the normalized Voigt notation.

Evaluation of first and second moments of total strain

The first and second moment of the internal strain deviator as required in Eq. (24)₁ depends on the first and second moment of the total strain by use of Eq. (25). These are expressed [52] as

$$\begin{gathered} \left\langle {\varvec{\varepsilon}} \right\rangle^{\gamma } = {\mathbb{A}}^{\gamma } \left[ {\varvec{E}} \right] + {\varvec{a}}^{\gamma } \hfill \\ \left\langle {\varvec{\varepsilon}^{\prime} \cdot \varvec{\varepsilon}^{\prime}} \right\rangle^{\gamma } = \left( {\frac{1}{{c^{\gamma } }}\frac{{\partial \overline{\psi }_{{\text{o}}} \left( {K^{\gamma } ,G^{\gamma } } \right)}}{{\partial G^{\gamma } }}} \right) = \frac{1}{{c^{\gamma } }}\frac{{\overline{\psi }_{{\text{o}}} \left( {K^{\gamma } ,G^{\gamma } + \delta G^{\gamma } } \right) - \overline{\psi }_{{\text{o}}} \left( {K^{\gamma } ,G^{\gamma } - \delta G^{\gamma } } \right)}}{{{2}\left( {\delta G^{\gamma } } \right)}} \hfill \\ \end{gathered}$$

(A.2)

where δG^γ is variation in the shear modulus, ${\mathbb{A}}^{\gamma }$ is the strain localization tensor and a^γ is the thermal strain localization tensor of γ-phase. It is sufficient to define ${\mathbb{A}}^{\gamma }$ for homogenizing a two-phase thermoelastic composite problem (see Eq. (A.5)).

Mean-field solution of a linear thermoelastic homogenization problem

Consider a two-phase linear thermoelastic composite (identified as phase γ = 1 and 2, labeled as “m” and “f,” respectively) defined with the thermoelastic energy function of the local constituents as

$$\psi_{{\text{o}}} = \frac{1}{2}\left( {{\varvec{\varepsilon}} - {\varvec{\varepsilon}}_{\theta } } \right) \cdot {\mathbb{C}}\left[ {{\varvec{\varepsilon}} - {\varvec{\varepsilon}}_{\theta } } \right] = \frac{1}{2}{\varvec{\varepsilon}} \cdot {\mathbb{C}}\left[ {\varvec{\varepsilon}} \right] + {\varvec{\beta}} \cdot {\varvec{\varepsilon}} + h$$

(A.3)

where the local terms ε_θ is the thermal strain and β is the thermal stress. Consider the composite constitutes of fiber stiffness surrounded by the matrix stiffness, i.e., ${\mathbb{C}}^{{\text{f}}}$ and ${\mathbb{C}}^{{\text{m}}}$, respectively, with the thermal stresses β^f and β^m. The effective thermoelastic energy function and effective stress are given as

$$\begin{gathered} \overline{\psi }_{{\text{o}}} = \frac{1}{2}\varvec{\it E} \cdot \overline{\mathbb{C}}\left[ \varvec{\it E} \right] + \varvec{\it B} \cdot \varvec{\it E} + \overline{h} \hfill \\{\varvec{\it \Sigma}}= \frac{{\partial \overline{\psi }_{{\text{o}}} }}{{\partial {\varvec{E}}}} = \overline{\mathbb{C}}\left[ {\varvec{E}} \right] + \varvec{\it B} \hfill \\ \overline{\mathbb{C}} = {\mathbb{C}}^{{\text{m}}} + c^{{\text{f}}} \delta {\mathbb{C}}{\mathbb{A}}^{{\text{f}}} \hfill \\ \varvec{\rm B} = \left\langle {\varvec{\beta}} \right\rangle + c^{{\text{f}}} \delta {\mathbb{C}}\,\left[ {{\varvec{a}}^{{\text{f}}} } \right] \hfill \\ \overline{h} = \left\langle h \right\rangle + c^{{\text{f}}} \delta {\varvec{\beta}} \cdot {\varvec{a}}^{{\text{f}}} \hfill \\ \end{gathered}$$

(A.4)

where B is the effective thermal stress, $\delta {\mathbb{C}} = {\mathbb{C}}^{{\text{f}}} - {\mathbb{C}}^{{\text{m}}}$, δβ = β^f–β^m and ${\mathbb{A}}^{{\text{f}}}$ is the strain localization tensor for the fiber phase. In two-phase composite problem, the following two identities are applicable, i.e., $\left\langle {\mathbb{A}} \right\rangle = {\mathbb{I}}^{{\text{s}}}$ and $\left\langle {\varvec{a}} \right\rangle = \it {\varvec{0}}$. In this problem, it is sufficient to define ${\mathbb{A}}^{{\text{f}}}$ as the thermal strain localization tensor a^f can be expressed in terms of ${\mathbb{A}}^{{\text{f}}}$ [53] as

$${\varvec{a}}^{{\text{f}}} = \left( {{\mathbb{A}}^{{\text{f}}} - {\mathbb{I}}^{{\text{s}}} } \right)\delta {\mathbb{C}}^{ - 1} \left[ {\delta {\varvec{\beta}}} \right]$$

(A.5)

The strain localization tensor for the fiber phase ${\mathbb{A}}^{\text{f}}$ is defined by HSLB elastic homogenization scheme.

4.1 Hashin–Shtrikman lower bound (HSLB)

A closer estimate of effective elastic stiffness tensor can be obtained using a variational principle [54] that yields in estimating the upper and lower bounds of the effective stiffness of composite by the choice of reference medium. If the comparison material corresponds to the matrix material or the compliant medium, it estimates the lower bound of the effective stiffness. The strain localization tensor corresponding to the lower bound is given as

$$\begin{gathered} {\mathbb{A}}_{{{\text{HSLB}}}}^{{\text{f}}} = \left( {c^{{\text{m}}} \left( {{\mathbb{A}}^{{{\text{SIP}}}} } \right)^{ - 1} + c^{{\text{f}}} {\mathbb{I}}^{{\text{s}}} } \right)^{ - 1} \hfill \\ {\mathbb{A}}^{{{\text{SIP}}}} = \left( {{\mathbb{I}}^{{\text{s}}} + {\mathbb{P}}_{{\text{o}}} \left( {\delta {\mathbb{C}}} \right)^{ - 1} } \right)^{ - 1} \hfill \\ \end{gathered}$$

(A.6)

where the strain localization tensor ${\mathbb{A}}^{{{\text{SIP}}}}$ corresponds to a single inclusion problem and $\delta {\mathbb{C}} = {\mathbb{C}}^{\text{f}} - {\mathbb{C}}_{{\text{o}}}$ with ${\mathbb{C}}_{{\text{o}}} = {\mathbb{C}}^{{\text{m}}}$ for HSLB. The Hill’s polarization tensor, ${\mathbb{P}}_{{\text{o}}}$, is a function of the elastic properties of the comparison material, i.e., the matrix material in case of HSLB and the geometrical shape of the inhomogeneity [55]. In case of UD FRP composites, the geometrical shape of the fiber is modeled as a needle-shaped inclusion rather than actually being a cylinder of higher aspect ratio [50].