SpringerLink
Log in

Numerical study of solitary waves and reversible shock structures in tubes with controlled pressure

  • Published:
Computational Mathematics and Mathematical Physics Aims and scope Submit manuscript

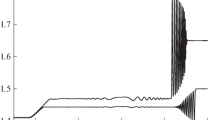
Abstract

Methods for computing and analyzing solutions for a model of a tube with elastic walls in the case of controlled internal pressure is developed. A membrane model or a plate model is used for the tube walls. Numerical methods are applied. The Boussinesq equations are used to describe waves near the transition to the instability zone of homogeneous states and to verify the numerical methods. Solitary waves and soliton shock structures for these equations are studied. The Boussinesq equations are analyzed and generalized. Next, the same methods are applied to the complete equations. Solitary waves and reversible shock structures (generalized kinks) are studied. The stability of the solitary waves is analyzed by finding an eigenfunction. The kinks are studied using general methods of the theory of reversible shocks.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Thailand)

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Y. B. Fu and S. P. Pearce, “Characterization and stability of localized bulging/necking in inflated membrane tubes,” IMA J. Appl. Math. 75, 581–602 (2010).

    Article  MathSciNet  MATH  Google Scholar 

  2. A. G. Kulikovskii, “On the stability of homogeneous states,” Prikl. Mat. Mekh. 30 (1), 148–153 (1966).

    Google Scholar 

  3. I. B. Bakholdin, Nondissipative Shocks in Continuum Mechanics (Fizmatlit, Moscow, 2014) [in Russian].

    Google Scholar 

  4. I. B. Bakholdin and V. Ya. Tomashpol’skii, “Solitary waves in the model of a predeformed nonlinear composite,” Differ. Equations 40 (4), 571–582 (2004).

    Article  MathSciNet  MATH  Google Scholar 

  5. I. B. Bakholdin, “Analysis methods for structures of dissipative and nondissipative jumps in dispersive systems,” Comput. Math. Math. Phys. 15 (2), 317–328 (2005).

    MathSciNet  Google Scholar 

  6. I. B. Bakholdin, “Discontinuities described by generalized Korteweg–de Vries equations,” Izv. Ross. Akad. Nauk, Mekh. Zhidk. Gaza, No. 4, 95–109 (1999).

    MathSciNet  MATH  Google Scholar 

  7. I. B. Bakholdin, “Time-invariant and time-varying discontinuity structures for models described by the generalized Korteweg–Burgers equation,” J. Appl. Math. Mech. 75 (2), 189–209 (2011).

    Article  MathSciNet  MATH  Google Scholar 

  8. I. B. Bakholdin, “Theory and classification of the reversible structures of discontinuities in hydrodynamic-type models,” J. Appl. Math. Mech. 78 (6), 599–612 (2014).

    Article  MathSciNet  Google Scholar 

  9. P. J. Roache, Computational Fluid Dynamics (Hermosa, Albuquerque, 1976; Mir, Moscow, 1980).

    MATH  Google Scholar 

  10. A. A. Samarskii, The Theory of Difference Schemes (Nauka, Moscow, 1977; Marcel Dekker, New York, 2001).

    MATH  Google Scholar 

  11. Y. B. Fu and A. Il’ichev, “Solitary waves in fluid-filled elastic tubes: Existence, persistence, and the role of axial displacement,” IMA J. Appl. Math. 75, 257–268 (2010).

    Article  MathSciNet  MATH  Google Scholar 

  12. A. T. Ilichev and I. B. Fu, “Stability of aneurism solutions in fluid-filled elastic membrane tube,” Acta Mech. Sinica 28, 1209–1218 (2012).

    Article  MathSciNet  Google Scholar 

  13. A. Aydin and B. Karasozen, “Symplectic and multi-symplectic Lobatto methods for the “good” Boussinesq equation,” J. Math. Phys. (2008) 49, 083509; http://dx.doi.org/10.1063/1.297048.

    Article  MathSciNet  MATH  Google Scholar 

  14. J. C. Alexander and R. Sachs, “Linear instability of solitary waves of Boussinesq-type equation: A computer assisted computation,” Nonlinear Word 2, 471–507 (1995).

    MathSciNet  MATH  Google Scholar 

  15. J. L. Bona and R. L. Sachs, “Global existence of smooth solutions and stability of solitary waves for a generalized Boussinesq equation,” Comm. Math. Phys. 118, 15–29 (1988).

    Article  MathSciNet  MATH  Google Scholar 

  16. L. V. Bogdanov and V. E. Zakharov, “The Boussinesq equation revisited,” Phys. D 165, 137–162 (2002).

    Article  MathSciNet  MATH  Google Scholar 

  17. J. W. Miles, “Resonantly interacting solitary waves,” J. Fluid Mech. 79, Part 1, 157–169 (1977).

    Article  MathSciNet  MATH  Google Scholar 

  18. I. B. Bakholdin, “Discontinuities of the variables that characterize the propagation of solitary waves in a fluid layer,” Fluid Dyn. 19 (3), 416–422 (1984).

    Article  Google Scholar 

  19. V. K. Kalantarov and O. A. Ladyzhenskaya, “The occurrence of collapse for quasilinear equations of parabolic and hyperbolic types,” 10 (1), 53–70 (1978).

    Google Scholar 

  20. F. Linares, “Global existence of small solutions for a generalized Boussinesq equation,” J. Differ. Equations 106, 257–293 (1993).

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Keldysh Institute of Applied Mathematics, Russian Academy of Sciences, Miusskaya pl. 4, Moscow, 125047, Russia

    I. B. Bakholdin

Authors
  1. I. B. Bakholdin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to I. B. Bakholdin.

Additional information

Original Russian Text © I.B. Bakholdin, 2015, published in Zhurnal Vychislitel’noi Matematiki i Matematicheskoi Fiziki, 2015, Vol. 55, No. 11, pp. 1921–1936.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bakholdin, I.B. Numerical study of solitary waves and reversible shock structures in tubes with controlled pressure. Comput. Math. and Math. Phys. 55, 1884–1898 (2015). https://doi.org/10.1134/S0965542515110056

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0965542515110056

Keywords

Search

Navigation