SpringerLink
Log in

Sharp Error Analysis for Averaging Crank-Nicolson Schemes with Corrections for Subdiffusion with Nonsmooth Solutions

  • Original Paper
  • Published:
Communications on Applied Mathematics and Computation Aims and scope Submit manuscript

Abstract

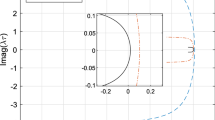
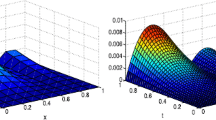
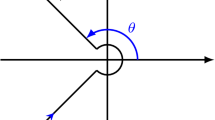
Thanks to the singularity of the solution of linear subdiffusion problems, most time-step** methods on uniform meshes can result in \(O(\tau )\) accuracy where \(\tau \) denotes the time step. The present work aims to discover the reason why some type of Crank-Nicolson schemes (the averaging Crank-Nicolson (ACN) scheme) for the subdiffusion can only yield \(O(\tau ^\alpha )\,\, (\alpha <1)\) accuracy, which is much lower than the desired. The existing well-developed error analysis for the subdiffusion, which has been successfully applied to many time-step** methods such as the fractional BDF-\(p\,\,(1\leqslant p\leqslant 6)\), requires singular points to be out of the path of contour integrals involved. The ACN scheme in this work is quite natural but fails to meet this requirement. By resorting to the residue theorem, some novel sharp error analysis is developed in this study, upon which correction methods are further designed to obtain the optimal \(O(\tau ^2)\) accuracy. All results are verified by numerical tests.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
EUR 32.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or Ebook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Germany)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Data Availability

The data that support the findings of this study are available from the corresponding author, upon reasonable request.

References

  1. Alikhanov, A.A.: A new difference scheme for the time fractional diffusion equation. J. Comput. Phys. 280, 424–438 (2015)

    Article  MathSciNet  Google Scholar 

  2. Barkai, E., Metzler, R., Klafter, J.: From continuous time random walks to the fractional Fokker-Planck equation. Phys. Rev. E 61(1), 132 (2000)

    Article  MathSciNet  Google Scholar 

  3. Chen, H., Stynes, M.: Error analysis of a second-order method on fitted meshes for a time-fractional diffusion problem. J. Sci. Comput. 79, 624–647 (2019)

    Article  MathSciNet  Google Scholar 

  4. Chen, S., Shen, J., Zhang, Z., Zhou, Z.: A spectrally accurate approximation to subdiffusion equations using the log orthogonal functions. SIAM J. Sci. Comput. 42(2), A849–A877 (2020)

    Article  MathSciNet  Google Scholar 

  5. Dimitrov, Y.: Numerical approximations for fractional differential equations. ar**v:1311.3935 (2013)

  6. Gao, G.H., Sun, H.W., Sun, Z.Z.: Stability and convergence of finite difference schemes for a class of time-fractional sub-diffusion equations based on certain superconvergence. J. Comput. Phys. 280, 510–528 (2015)

    Article  MathSciNet  Google Scholar 

  7. Golding, I., Cox, E.C.: Physical nature of bacterial cytoplasm. Phys. Rev. Lett. 96(9), 098102 (2006)

    Article  Google Scholar 

  8. **, B., Lazarov, R., Zhou, Z.: Error estimates for a semidiscrete finite element method for fractional order parabolic equations. SIAM J. Numer. Anal. 51(1), 445–466 (2013)

    Article  MathSciNet  Google Scholar 

  9. **, B., Li, B., Zhou, Z.: Correction of high-order BDF convolution quadrature for fractional evolution equations. SIAM J. Sci. Comput. 39(6), A3129–A3152 (2017)

    Article  MathSciNet  Google Scholar 

  10. **, B., Li, B., Zhou, Z.: An analysis of the Crank-Nicolson method for subdiffusion. IMA J. Numer. Anal. 38(1), 518–541 (2018)

    Article  MathSciNet  Google Scholar 

  11. Kilbas, A.A., Srivastava, H.M., Trujillo, J.J.: Theory and Applications of Fractional Differential Equations, vol. 204. Elsevier, London (2006)

    Book  Google Scholar 

  12. Kopteva, N.: Error analysis of the L1 method on graded and uniform meshes for a fractional-derivative problem in two and three dimensions. Math. Comput. 88(319), 2135–2155 (2019)

    Article  MathSciNet  Google Scholar 

  13. Li, C., Zeng, F.: Numerical Methods for Fractional Calculus, vol. 24. CRC Press, New York (2015)

    Book  Google Scholar 

  14. Li, D., Sun, W., Wu, C.: A novel numerical approach to time-fractional parabolic equations with nonsmooth solutions. Numer. Math. Theor. Methods Appl. 14(2), 355–376 (2021)

    Article  MathSciNet  Google Scholar 

  15. Liao, H.L.: A second-order scheme with nonuniform time steps for a linear reaction-subdiffusion problem. Commun. Comput. Phys. 30(2), 567–601 (2021)

    Article  MathSciNet  Google Scholar 

  16. Liao, H.L., Li, D., Zhang, J.: Sharp error estimate of the nonuniform L1 formula for linear reaction-subdiffusion equations. SIAM J. Numer. Anal. 56(2), 1112–1133 (2018)

  17. Lin, Y., Xu, C.: Finite difference/spectral approximations for the time-fractional diffusion equation. J. Comput. Phys. 225(2), 1533–1552 (2007)

    Article  MathSciNet  Google Scholar 

  18. Liu, Y., Yin, B., Li, H., Zhang, Z.: The unified theory of shifted convolution quadrature for fractional calculus. J. Sci. Comput. 89(1), 18 (2021)

    Article  MathSciNet  Google Scholar 

  19. Lubich, C.: Discretized fractional calculus. SIAM J. Math. Anal. 17(3), 704–719 (1986)

    Article  MathSciNet  Google Scholar 

  20. Magin, R.: Fractional calculus in bioengineering, part 1. Crit. Rev. Bioeng. 32(1), 33 (2004)

    Google Scholar 

  21. Raberto, M., Scalas, E., Mainardi, F.: Waiting-times and returns in high-frequency financial data: an empirical study. Phys. A 314(1–/2/3/4), 749–755 (2002)

  22. Sakamoto, K., Yamamoto, M.: Initial value/boundary value problems for fractional diffusion-wave equations and applications to some inverse problems. J. Math. Anal. Appl. 382(1), 426–447 (2011)

    Article  MathSciNet  Google Scholar 

  23. Shi, J., Chen, M., Yan, Y., Cao, J.: Correction of high-order \(L_k\) approximation for subdiffusion. J. Sci. Comput. 93(1), 31 (2022)

    Article  Google Scholar 

  24. Stynes, M.: Too much regularity may force too much uniqueness. Frac. Calc. Appl. Anal. 19(6), 1554–1562 (2016)

    Article  MathSciNet  Google Scholar 

  25. Stynes, M., O’Riordan, E., Gracia, J.L.: Error analysis of a finite difference method on graded meshes for a time-fractional diffusion equation. SIAM J. Numer. Anal. 55(2), 1057–1079 (2017)

    Article  MathSciNet  Google Scholar 

  26. Sun, Z.Z., Wu, X.: A fully discrete difference scheme for a diffusion-wave system. Appl. Numer. Math. 56(2), 193–209 (2006)

    Article  MathSciNet  Google Scholar 

  27. Wang, J., Wang, J., Yin, L.: A single-step correction scheme of Crank-Nicolson convolution quadrature for the subdiffusion equation. J. Sci. Comput. 87(1), 26 (2021)

    Article  MathSciNet  Google Scholar 

  28. Wang, Y., Yan, Y., Yan, Y., Pani, A.K.: Higher order time step** methods for subdiffusion problems based on weighted and shifted Grünwald-Letnikov formulae with nonsmooth data. J. Sci. Comput. 83(3), 40 (2020)

    Article  Google Scholar 

  29. Yan, Y., Khan, M., Ford, N.J.: An analysis of the modified L1 scheme for time-fractional partial differential equations with nonsmooth data. SIAM J. Numer. Anal. 56(1), 210–227 (2018)

    Article  MathSciNet  Google Scholar 

  30. Yin, B., Liu, Y., Li, H.: Necessity of introducing non-integer shifted parameters by constructing high accuracy finite difference algorithms for a two-sided space-fractional advection-diffusion model. Appl. Math. Lett. 105, 106347 (2020)

    Article  MathSciNet  Google Scholar 

  31. Yin, B., Liu, Y., Li, H., Zhang, Z.: Efficient shifted fractional trapezoidal rule for subdiffusion problems with nonsmooth solutions on uniform meshes. BIT 62(2), 631–666 (2022)

    Article  MathSciNet  Google Scholar 

  32. Zaslavsky, G.M.: Chaos, fractional kinetics, and anomalous transport. Phys. Rep. 371(6), 461–580 (2002)

    Article  MathSciNet  Google Scholar 

  33. Zeng, F., Li, C., Liu, F., Turner, I.: Numerical algorithms for time-fractional subdiffusion equation with second-order accuracy. SIAM J. Sci. Comput. 37(1), A55–A78 (2015)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

This work is supported by the Autonomous Region Level High-Level Talent Introduction Research Support Program in 2022 (No. 12000-15042224 to B.Y.), the National Natural Science Foundation of China (No. 12201322 to B.Y., 12061053 to Y.L., and 12161063 to H.L.), and the Natural Science Foundation of Inner Mongolia, China (No. 2020MS01003 to Y.L. and 2021MS01018 to H.L.).

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Inner Mongolia University, Hohhot, 010021, Inner Mongolia, China

    Baoli Yin, Yang Liu & Hong Li

Authors
  1. Baoli Yin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yang Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Hong Li
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Hong Li.

Ethics declarations

Conflict of Interest

The authors declare that they have no conflict of interest.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yin, B., Liu, Y. & Li, H. Sharp Error Analysis for Averaging Crank-Nicolson Schemes with Corrections for Subdiffusion with Nonsmooth Solutions. Commun. Appl. Math. Comput. (2024). https://doi.org/10.1007/s42967-024-00401-1

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s42967-024-00401-1

Keywords

Mathematics Subject Classification

Search

Navigation